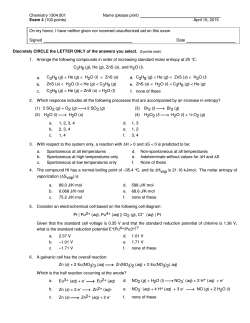
LeChatelier`s Principle LeChatelier`s Principle CO2(g) +
CHEM 107, Spring 2015
Class #35
LeChatelier’s Principle
CHEM 107
L.S. Brown
Texas A&M University
LeChatelier’s Principle
• “When a change is imposed on a
system at equilibrium, the system will
react in the direction that reduces the
amount of change.”
• Adding or removing material, changes
in P or T
CO2(g) + CF4(g) ⇌ 2 COF2(g)
• At equilibrium at 1000°C a 10.0 L vessel
contains 0.990 mol CO2, 0.714 mol CF4
and 0.570 mol COF2
• Remove all of the COF2. What happens
to the amount of CF4?
• Calculate final amount of each gas
© 2015, L.S. Brown
1
CHEM 107, Spring 2015
Using Le Châtelier's Principle
CO(g) + 3H2(g) ⇌ CH4(g) + H2O(g)
• An equilibrium mixture at 1200 K
contains 0.613 mol CO, 1.839 mol H2,
0.387 mol CH4 and 0.387 mol H2O, all
in a 10.0 L vessel. (What is Keq?)
• All of the H2O is removed, and
equilibrium is re-established.
• What will happen to the amount of CH4?
• Set up eqn. for final amount of CH4.
Using Le Châtelier's Principle - Set up
K eq =
[CH 4 ][H 2 O]
[CO][H 2 ]3
CO(g) + 3H2(g) ⇌ CH4(g) + H2O(g)
init. .0613
.1839
.0387 .0387 M
chg. .0613
.1839
.0387
0.0 M
• Equilibrium is re-established by production
of more H2O (let the final [H2O] = x):
eq. .0613-x .1839-3x .0387+x x M
Using Le Châtelier's Principle - Set up
K eq = 3.93 =
(.0387 + x)x
(.1839 - 3x) 3 (.0613 - x)
© 2015, L.S. Brown
2
CHEM 107, Spring 2015
Changes in Pressure
Consider the equilibrium (significant at 700 ˚C):
CaO(s) + CO2(g) ⇌ CaCO3(s)
Le Châtelier’s Principle would suggest:
If the pressure is suddenly increased, say by
suddenly compressing the container, more
CO2 would react with CaO to produce
more CaCO3.
LeChatelier: K and T
• Think of “heat” as a reactant or product
• Exothermic:
A + B ! C + Heat
• Raising T will shift to left
• Endothermic:
A + Heat ! B + C
• Raising T will shift to right
Equilibrium & Thermo
• Equilibrium is an eventual outcome - no
time limit
• Related to thermodynamic properties,
especially ΔG
• Changing T changes the values of ΔG
and Keq
© 2015, L.S. Brown
3
CHEM 107, Spring 2015
ΔG° and Keq
ΔG° = –RT ln Keq
• This relationship lets us find an
equilibrium constant at any temperature
provided that we know or can calculate
the standard free energy change (ΔG° )
• So we can get K values by using the
thermo tables.
Example
CH4(g) + 1/2 O2(g) ⇌ CH3OH(l )
Find Keq at 25°C.
Find Keq at 800°C (for making CH3OH(g))
ΔH°f (kJ/mol) S° (J/K/mol)
ΔG°f (kJ/mol)
CH4(g)
–74.81
186.3
–50.72
O2(g)
0
205.0
0
CH3OH(l )
–238.7
126.8
–166.3
CH3OH(g)
–200.7
239.7
–162.0
Temperature Dependence of K
K = exp{–∆G°/RT}
∆G° = ∆H° – T∆S°
⇔
ln K = –∆G°/RT
ln K = –(∆H°/RT) + (∆S°/R)
If we assume, as before, that ∆H and ∆S don’t
change much as a function of temp. (∆H ≈ ∆H°
and S ≈ ∆S°), then a plot of the temperature
dependence of K will give us ∆H° and ∆S°.
© 2015, L.S. Brown
4
CHEM 107, Spring 2015
2 NO2 ⇌ N2O4, ∆S < 0, ∆H < 0
low T: N2O4 favored; high T: NO2 favored
N2O4: colorless
120 pm
O
N
134˚
O
NO2: brown
ln K vs. 1/T : 2 NO2 ⇌ N2O4
∆H , ∆S?
ln K vs. 1/T : 2 NO2 ⇌ N2O4
ln K = –(∆H°/R)(1/T) + (∆S°/R)
intercept (1/T) → 0 : (∆S°/R) = 21.2
∆S° = (21.2)(8.314) = 176.3 J/mol • K
slope: –(∆H°/R) = 6880 K
∆H° = – (6880)(8.314) = – 57200 J/mol =
= – 57.2 kJ/mol
© 2015, L.S. Brown
5
CHEM 107, Spring 2015
H2O(l ) ⇌ H2O(g)
H2O(l ) ⇌ H2O(g)
Problem: H2O(l ) ⇌ H2O(g)
• Given: 44.01 kJ of heat is required to
boil away 1.0 mol of water at 1.0 atm.
As we know, this happens at 100 ˚C.
• Q: Estimate the boiling point of water on
a planet where the atmospheric
pressure is twice that of earth.
© 2015, L.S. Brown
6
CHEM 107, Spring 2015
CaCO3(s) ⇌ CaO(s) + CO2(g)
• Given:
∆H° = + 178 kJ; at 25 ˚C, KP = 1.39 × 10-23
• Give an estimate for KP at 800 ˚C.
Solubility Equilibrium
The process is
in equilibrium
when rate of
ions (or
molecules)
leaving the
solid = rate
returning to the
solid.
That is, when
dissolution rate
= precipitation
rate
Solubility Products (Problem)
In a saturated aqueous solution of MgF2, the
concentration of Mg2+ ions is 1.14 × 10-3 M.
Write the equilibrium constant expression,
and compute Ksp for MgF2.
What will the concentration of F– ions be in a
saturated solution of MgF2 dissolved in a
0.05 M Mg(NO3)2 solution?
© 2015, L.S. Brown
7
CHEM 107, Spring 2015
Mixed Eq. Const. Example
Limestone caverns are formed by the reaction
of H2O and CO2 with natural deposits of
calcium carbonate (CaCO3):
CaCO3(s) + CO2(g) + H2O(l ) ⇌
Ca2+(aq) + 2HCO3–(aq)
-3
Keq = 1.56 × 10 M3 atm-1
The partial pressure of in the Earth’s
atmosphere is atm. What is the equilibrium
Ca2+ concentration in the groundwater in a
limestone cavern?
© 2015, L.S. Brown
8
© Copyright 2025