Download Report

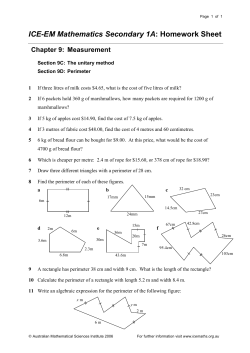
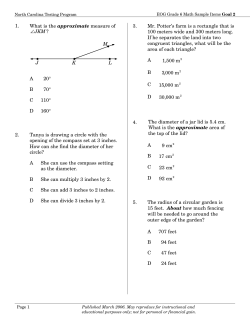
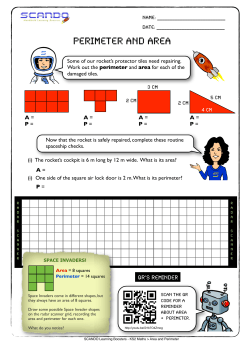
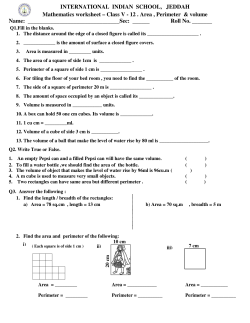
Module 12 Review Questions
____
1. The rule for a pattern is multiply by 2. If the first term in the pattern is 3,
which shows the numbers in the pattern?
A.
B.
C.
D.
____
3, 6, 12, 24, …
8, 16, 32, 64, …
3, 9, 18, 36, …
3, 5, 7, 9, …
2. The input/output table shows the number of monkeys, m, and the number
of bananas, b, that they eat.
If the output is
A.
B.
C.
D.
____
8, 10, 12, 14, …
8, 16, 24, 32, …
8, 12, 16, 20, …
4, 8, 12, 16, …
4. The rule for a pattern is subtract 3. The first term in the pattern is 60. Which
shows the numbers in the pattern?
A.
B.
C.
D.
____
2
36
35
12
3. The first term in a pattern is 8. If the rule for the pattern is skip-count by 4,
which shows the numbers in the pattern?
A.
B.
C.
D.
____
, how many bananas do 7 monkeys eat?
60, 63, 66, 69, …
60, 57, 54, 51, …
60, 30, 10, 5, …
60, 55, 50, 45, …
5. Tiffani makes the input/output table below.
Which could be a rule for her table?
A.
B.
C.
D.
____
The output is
The output is
The output is
Not here
.
.
.
6. The input-output table shows the number of dimes, d, and the value in
cents, c, of that number of dimes.
Input
Output
If the output is d
A.
B.
C.
D.
____
2
20
4
5
7
10, what is the value in cents of 7 dimes?
7 cents
80 cents
70 cents
100 cents
7. The rule for a pattern is add 9. The first term in the pattern is 3. Which
shows the numbers in the pattern?
A.
B.
C.
D.
____
d
c
3, 12, 13, 14, …
3, 12, 22, 33, …
3, 11, 19, 27, …
3, 12, 21, 30, …
8. Jane makes the input-output table below.
Input
Output
b
c
Which could be a rule for her table?
8
2
9
3
10
4
11
5
A.
B.
C.
D.
____
The output is b
The output is b
The output is b
The output is b
6.
1.
4.
6.
9. Cy wants to find the perimeter of this rectangle in meters.
Which expression should he use to find the perimeter?
A.
B.
C.
D.
____ 10. Cliff wants to find the area of a rectangular bulletin board. The bulletin
board is 30 inches long and 14 inches wide.
Which formula should Cliff use to find the area of the bulletin board?
A.
B.
C.
D.
A=l l
A=l w
A=w w
A=l+w
____ 11. A rectangle measures 4 yards by 6 yards. Which expression can be used
to find the area of the rectangle?
A.
B.
C.
D.
(2 4) + (2
(2 4) 6
4 6
4+6
6)
____ 12. Jo wants to find the area of the ceiling in her rectangular bedroom. If she
knows the length and the width, which formula can she use to find the
area?
A.
B.
C.
D.
A = 2 (l + w)
A = (2 w) + (2
A=l+w+l+w
A=l w
l)
____ 13. Mindy puts a rectangular poster of her favorite singer on a wall in her
bedroom.
What is the perimeter of the poster?
A.
B.
C.
D.
100 inches
70 inches
60 inches
50 inches
____ 14. Hiro painted a mural with the dimensions shown.
What is the area of Hiro’s mural?
A.
B.
C.
D.
4 square feet
16 square feet
32 square feet
60 square feet
____ 15. Mrs.Ericson is building a new balcony.
What is the area of Mrs.Ericson’s new balcony?
A.
B.
C.
D.
44 square feet
36 square feet
32 square feet
28 square feet
____ 16. Aidan bought a frame for a photograph that he took.
What was the area of the frame that he bought?
A.
B.
C.
D.
80 square inches
120 square inches
160 square inches
240 square inches
____ 17. A row of lockers covers 160 square feet of space along a wall. If the lockers
are 5 feet tall, what length along the wall do they cover?
A.
B.
C.
D.
800 feet
155 feet
75 feet
32 feet
____ 18. A farmer wants to use 200 feet of fencing for a chicken pen. He wants the
width of the pen to be 20 feet. What is the length of the pen?
A.
B.
C.
D.
10 feet
20 feet
40 feet
80 feet
____ 19. Iris is sewing fringe around the edges of a square tablecloth. Each side of
the tablecloth is 36 inches long. How many inches of fringe will Iris need?
A.
B.
C.
D.
72 inches
108 inches
144 inches
1,296 inches
____ 20. Eric measures a rectangular rug in his home. The rug has a length of 7 feet
and a width of 4 feet.
What is the perimeter of the rug?
A.
B.
C.
D.
28 feet
21 feet
22 feet
24 feet
____ 21. Mr. Douglass wants to build a fence around his backyard. His backyard is a
rectangle 30 yards long by 17 yards wide. How many yards of fence will he
need?
A.
B.
C.
D.
47 yards
84 yards
510 yards
94 yards
____ 22. A small rectangular garden is 18 feet long and 12 feet wide. What is the
perimeter of the garden?
A.
B.
C.
D.
60 feet
36 feet
30 feet
216 feet
Module 12 Review Questions
Answer Section
1. ANS: A
PTS: 1
REF: Lesson 12.1: Number Patterns
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
2. ANS: C
PTS: 1
REF: Lesson 12.1: Number Patterns
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
3. ANS: C
PTS: 1
REF: Lesson 12.1: Number Patterns
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
4. ANS: B
PTS: 1
REF: Lesson 12.1: Number Patterns
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
5. ANS: C
PTS: 1
REF: Lesson 12.2: Find a Rule
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
6. ANS: C
PTS: 1
REF: Lesson 12.1: Number Patterns
7.
8.
9.
10.
11.
12.
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
ANS: D
PTS: 1
REF: Lesson 12.1: Number Patterns
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
ANS: A
PTS: 1
REF: Lesson 12.2: Find a Rule
STA: TEKS.4.5.B Represent problems using an input-output table and
numerical expressions to generate a number pattern that follows a given
rule such as given the rule "Add 3" and the starting number 1, use the
expressions 1 + 3, 2 + 3, 3 + 3, and so forth to generate a table to
represent the relationship of the values in the resulting sequence and their
position in the sequence.
ANS: C
PTS: 1
REF: Lesson 12.3: Model Perimeter Formulas
STA: TEKS.4.5.C Use models to determine the formulas for the perimeter
of a rectangle (l + w + l + w or 2l + 2w), including the special form for
perimeter of a square(4s) and the area of a rectangle (l x w).
ANS: B
PTS: 1
REF: Lesson 12.4: Model Area Formulas
STA: TEKS.4.5.C Use models to determine the formulas for the perimeter
of a rectangle (l + w + l + w or 2l + 2w), including the special form for
perimeter of a square(4s) and the area of a rectangle (l x w).
ANS: C
PTS: 1
REF: Lesson 12.4: Model Area Formulas
STA: TEKS.4.5.C Use models to determine the formulas for the perimeter
of a rectangle (l + w + l + w or 2l + 2w), including the special form for
perimeter of a square(4s) and the area of a rectangle (l x w).
ANS: D
PTS: 1
REF: Lesson 12.4: Model Area Formulas
STA: TEKS.4.5.C Use models to determine the formulas for the perimeter
of a rectangle (l + w + l + w or 2l + 2w), including the special form for
13.
14.
15.
16.
17.
18.
perimeter of a square(4s) and the area of a rectangle (l x w).
ANS: A
PTS: 1
DIF: average
REF: Lesson 12.3: Model Perimeter Formulas
OBJ: Use a formula to find the perimeter of a rectangle.
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
TOP: Solve problems involving measurement and conversion of
measurements from a larger unit to a smaller unit.
KEY: perimeter | length | width
NOT: Measurement and Data
ANS: D
PTS: 1
DIF: average
REF: Lesson 12.4: Model Area Formulas
OBJ: Use a formula to find the area of a rectangle.
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
TOP: Solve problems involving measurement and conversion of
measurements from a larger unit to a smaller unit.
KEY: area | base | height | formula NOT: Measurement and Data
ANS: C
PTS: 1
DIF: average
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
OBJ: Find the area of combined rectangles.
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
TOP: Solve problems involving measurement and conversion of
measurements from a larger unit to a smaller unit.
NOT: Measurement and Data
ANS: A
PTS: 1
DIF: average
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
OBJ: Use the strategy solve a simpler problem to solve area problems.
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
TOP: Solve problems involving measurement and conversion of
measurements from a larger unit to a smaller unit.
NOT: Measurement and Data
ANS: D
PTS: 1
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
ANS: D
PTS: 1
REF: Lesson 12.3: Model Perimeter Formulas
19.
20.
21.
22.
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
ANS: C
PTS: 1
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
ANS: C
PTS: 1
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
ANS: D
PTS: 1
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.
ANS: A
PTS: 1
REF: Lesson 12.5: Problem Solving: Find the Perimeter and Area
STA: TEKS.4.5.D Solve problems related to perimeter and area of
rectangles where dimensions are whole numbers.