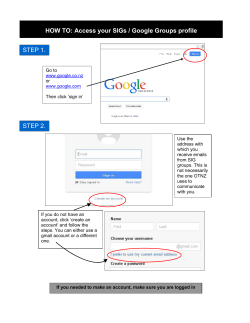
k âε model.
MAPNA Generator Co Lecturer: Ehsan Saadati [email protected] Eddy-viscosity Models: • Zero equation model. • Standard k −ε model. • RNG k −ε model. • Standard k −ω model. • Baseline (BSL) zonal k −ω based model. (This model can only be selected through Expert Control Parameters.) • SST zonal k −ω based model. • (k −ε)1E model.( This model can only be selected through Expert Control Parameters.) • Curvature correction for two-equation models. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Reynolds-Stress Models (RSM): • Launder, Reece and Rodi Isotropization of Production model (LRR Reynolds Stress). • Launder, Reece and Rodi Quasi-Isotropic model (QI Reynolds Stress). • Speziale, Sarkar and Gatski (SSG Reynolds Stress). • SMC-ω model (Omega Reynolds Stress). • Baseline (BSL) Reynolds Stress model. • Explicit Algebraic Reynolds Stress Model (EARSM). MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Laminar flow is governed by the unsteady Navier-Stokes equations. The laminar option does not apply a turbulence model to the simulation and is only appropriate if the flow is laminar. This typically applies at low Reynolds number flows (for example, for pipe flow the laminar flow regime is ~ Re < 1000). Energy transfer in the fluid is accomplished by molecular interaction (diffusion). In the case of high speed flows, the work of the viscous stresses can also contribute to the energy transfer. You should always check in the output file that the maximum Reynolds number is in the laminar flow regime. If you set up a simulation using laminar flow, but the real flow is turbulent, convergence is difficult and the simulation will not reach the correct solution. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The Zero Equation model implemented in CFX is simple to implement and use, can produce approximate results very quickly, and provides a good initial guess for simulations using more advanced turbulence models. In CFX, a constant turbulent eddy viscosity is calculated for the entire flow domain. If you specify an eddy viscosity, this value is used instead of the solver calculated value. You should not use this model to obtain final results. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The turbulence viscosity is modeled as the product of a turbulent velocity scale, Ut, and a turbulence length scale, lt, as proposed by Prandtl and Kolmogorov, μt =ρfμUt lt where f μ is a proportionality constant. The velocity scale is taken to be the maximum velocity in the fluid domain. The length scale is derived using the formula: where VD is the fluid domain volume. This model has little physical foundation and is not recommended. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] One of the most prominent turbulence models, the k −ε (k-epsilon) model, has been implemented in most general purpose CFD codes and is considered the industry standard model. It has proven to be stable and numerically robust and has a well established regime of predictive capability. For general purpose simulations, the k −ε model offers a good compromise in terms of accuracy and robustness. Within CFX, the k −ε turbulence model uses the scalable wall-function approach to improve robustness and accuracy when the near-wall mesh is very fine. The scalable wall functions allow solution on arbitrarily fine near wall grids, which is a significant improvement over standard wall functions. While standard two-equation models, such as the k −ε model, provide good predictions for many flows of engineering interest, there are applications for which these models may not be suitable. Among these are: • Flows with boundary layer separation. • Flows with sudden changes in the mean strain rate. • Flows in rotating fluids. • Flows over curved surfaces. A Reynolds Stress model may be more appropriate for flows with sudden changes in strain rate or rotating flows, while the SST model may be more appropriate for separated flows. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] and the momentum equation becomes: The k-ε model, like the zero equation model, is based on the eddy viscosity concept, so that: μeff=μ+μt where μt is the turbulence viscosity. The k-ε model assumes that the turbulence viscosity is linked to the turbulence kinetic energy and dissipation via the relation: where Cμ is a constant. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The values of k and ε come directly from the differential transport equations for the turbulence kinetic energy and turbulence dissipation rate: where Cε1, Cε2, σk and σε are constants. Pkb and Pεb represent the influence of the buoyancy forces, which are described below. Pk is the turbulence production due to viscous forces, which is modeled using: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The RNG k −ε model is an alternative to the standard k −ε model. In general it offers little improvement compared to the standard k −ε model. The RNG k − ε model is based on renormalization group analysis of the NavierStokes equations. The transport equations for turbulence generation and dissipation are the same as those for the standard k − ε model, but the model constants differ, and the constant Cε1 is replaced by the function Cε1RNG. The transport equation for turbulence dissipation becomes: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Currently, the most prominent two-equation models in this area are the k −ω based models of Menter. The k −ω based Shear-Stress-Transport (SST) model was designed to give a highly accurate predictions of the onset and the amount of flow separation under adverse pressure gradients by the inclusion of transport effects into the formulation of the eddy-viscosity. This results in a major improvement in terms of flow separation predictions. The superior performance of this model has been demonstrated in a large number of validation studies (Bardina et al.) The SST model is recommended for high accuracy boundary layer simulations. To benefit from this model, a resolution of the boundary layer of more than 10 points is required. For free shear flows, the SST model is identical to the k −ε model. The SST model was developed to overcome deficiencies in the k −ω and BSL k −ω models. Therefore, using the SST model over these models is recommended. One of the advantages of the k −ω formulation is the near wall treatment for lowReynolds number computations where it is more accurate and more robust. The convergence behavior of the k −ω model is often similar to that of the k −ε model. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] One of the advantages of the k-ω formulation is the near wall treatment for lowReynolds number computations. The model does not involve the complex nonlinear damping functions required for the k-ε model and is therefore more accurate and more robust. A low-Reynolds k-ε model would typically require a near wall resolution of y+ <0.2, while a low-Reynolds number k-ω model would require at least y+ <2. In industrial flows, even y+ <2 cannot be guaranteed in most applications and for this reason, a new near wall treatment was developed for the k-ω models. It allows for smooth shift from a low-Reynolds number form to a wall function formulation. The k-ω models assumes that the turbulence viscosity is linked to the turbulence kinetic energy and turbulent frequency via the relation: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The starting point of the present formulation is the k-ω model developed by Wilcox .It solves two transport equations, one for the turbulent kinetic energy, k, and one for the turbulent frequency, ω. The stress tensor is computed from the eddy-viscosity concept. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The main problem with the Wilcox model is its well known strong sensitivity to freestream conditions. Depending on the value specified for ω at the inlet, a significant variation in the results of the model canbe obtained. This is undesirable and in order to solve the problem, a blending between the k-ω model near the surface and the k-ε model in the outer region was developed by Menter. It consists of a transformation of the k-ε model to a k-ω formulation and a subsequent addition of the corresponding equations. The Wilcox model is thereby multiplied by a blending function F1 and the transformed k-ε model by a function 1−F1. F1 is equal to one near the surface and decreases to a value of zero outside the boundary layer (that is, a function of the wall distance). At the boundary layer edge and outside the boundary layer, the standard k-ε model is therefore recovered. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The k-ω based SST model accounts for the transport of the turbulent shear stress and gives highly accurate predictions of the onset and the amount of flow separation under adverse pressure gradients. The BSL model combines the advantages of the Wilcox and the k-ε model, but still fails to properly predict the onset and amount of flow separation from smooth surfaces. The reasons for this deficiency are given in detail in Menter .The main reason is that both models do not account for the transport of the turbulent shear stress. This results in an over prediction of the eddy-viscosity. The proper transport behavior can be obtained by a limiter to the formulation of the eddy-viscosity: Again F2 is a blending function similar to F1, which restricts the limiter to the wall boundary layer, as the underlying assumptions are not correct for free shear flows. S is an invariant measure of the strain rate. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The blending functions are critical to the success of the method. Their formulation is based on the distance to the nearest surface and on the flow variables. where y is the distance to the nearest wall, ν is the kinematic viscosity and: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] A disadvantage of standard two-equation turbulence models is the excessive generation of turbulence energy, Pk , in the vicinity of stagnation points. In order to avoid the build-up of turbulent kinetic energy in stagnation regions, a formulation of limiters for the production term in the turbulence equations is available. The coefficient Clim is called Clip Factor and has a value of 10 for ω based models. This limiter does not affect the shear layer performance of the model, but has consistently avoided the stagnation point build-up in aerodynamic simulations. Using the standard Boussinesq-approximation for the Reynolds stress tensor, the production term Pk can be expressed for incompressible flow as: where S denotes the magnitude of the strain rate and Sij the strain rate tensor. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Kato and Launder noticed that the very high levels of the shear strain rate S in stagnation regions are responsible for the excessive levels of the turbulence kinetic energy. Since the deformation near a stagnation point is nearly irrotational, that is the vorticity rate Ω is very small, they proposed the following replacement of the production term: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] One weakness of the eddy-viscosity models is that these models are insensitive to streamline curvature and system rotation. Based on the work of Spalart and Shur a modification of the production term has been derived which allows to sensitize the standard two-equation models to these effects .The empirical function suggested by Spalart and Shur to account for these effects is defined by It is used as a multiplier of the production term and has been limited in ANSYS CFX in the following way: The specific limiter 1.25 provided a good compromise for different test cases which have been considered with the SST model (for example, flow through a Uturn, flow in hydro cyclone, and flow over a NACA 0012 wing tip vortex MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] A very simple one-equation model has been developed by Menter. It is derived directly from the k − ε model and is therefore named the (k − ε)1E model where ∼v is the kinematic eddy viscosity, ∼v t is the turbulent kinematic eddy viscosity and σ is a model constant. The low Reynolds formulation of the (k − ε)1E model requires a near wall mesh resolution of y+ <1. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] In flows where the turbulent transport or non-equilibrium effects are important, the eddy-viscosity assumption is no longer valid and results of eddy-viscosity models might be inaccurate. Reynolds Stress (or Second Moment Closure (SMC)) models naturally include the effects of streamline curvature, sudden changes in the strain rate, secondary flows or buoyancy compared to turbulence models using the eddy-viscosity approximation. You may consider using a Reynolds Stress model in the following types of flow: • Free shear flows with strong anisotropy, like a strong swirl component. This includes flows in rotating fluids. • Flows with sudden changes in the mean strain rate. • Flows where the strain fields are complex, and reproduce the anisotropic nature of turbulence itself. • Flows with strong streamline curvature. • Secondary flow. • Buoyant flow. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Reynolds Stress models have shown superior predictive performance compared to eddy-viscosity models in these cases. This is the major justification for Reynolds Stress models, which are based on transport equations for the individual components of the Reynolds stress tensor and the dissipation rate. These models are characterized by a higher degree of universality. The penalty for this flexibility is a high degree of complexity in the resulting mathematical system. The increased number of transport equations leads to reduced numerical robustness, requires increased computational effort and often prevents their usage in complex flows. Theoretically, Reynolds Stress models are more suited to complex flows, however, practice shows that they are often not superior to twoequation models. An example of this is for wall-bounded shear layers, where despite their (theoretically) higher degree of universality, Reynolds Stress models often prove inferior to two-equation models. For wall-bounded flows try to use the SMC-BSL model. It is based on the ω-equation and automatic wall treatment. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Three varieties of the Reynolds Stress Model are available which use different model constants: • Reynolds Stress Model (LRR-IP) • QI Reynolds Stress Model (LRR-IQ) • SSG Reynolds Stress Model (SSG) In general, the SSG model is more accurate than the LRR versions for most flows. This is particularly true for swirling flows. The SSG model is therefore recommended over the other models, which are there for historic reasons and because they are standard models. It is frequently observed that Reynolds Stress models produce unsteady results, where two-equation models give steady state solutions. This can be correct from a physical standpoint, but requires the solution of the equations in transient mode. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Some of the main deficiencies of the Reynolds Stress models for the simulation of boundary layers are an inheritance from the underlying ε-equation. Particularly the accurate prediction of flow separation is problematic when the ε-equation is used. Furthermore, low-Reynolds number formulations for the ε-equation are usually complex and difficult to integrate, a deficiency which is exaggerated in combination with a Reynolds Stress model formulation. In order to avoid these issues, a Reynolds Stress model has been implemented which uses the ω equation instead of the ε-equation as the scale-determining equation. There are two types of SMC-ω model in CFX: • Omega Reynolds Stress • Baseline (BSL) Reynolds Stress As the free stream sensitivity of the standard k −ω model does carry over to the Reynolds Stress model, the BSL Reynolds Stress Model was developed which is based on the ω-equation used in the BSL model, and is preferred over the Omega Reynolds Stress Model. This is the formulation recommended when using the ωReynolds Stress model. All variants of the ω-Reynolds Stress model are combined with automatic wall treatment, which allows a flexible grid resolution near the wall. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Explicit algebraic Reynolds stress models (EARSM) represent an extension of the standard two-equation models. They are derived from the Reynolds stress transport equations and give a nonlinear relation between the Reynolds stresses and the mean strain-rate and vorticity tensors. Due to the higher order terms many flow phenomena are included in the model without the need to solve transport equations. The EARSM is an extension of the current k −ε and BSL model to capture effects of secondary flows and of flows with streamline curvature and system rotation. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Engineering transition predictions are based mainly on two modeling concepts. The first is the use of low-Reynolds number turbulence models, where the wall damping functions of the underlying turbulence model trigger the transition onset. This concept is attractive, as it is based on transport equations and can therefore be implemented without much effort. However, experience has shown that this approach is not capable of reliably capturing the influence of the many different factors that affect transition, such as free-stream turbulence, pressure gradients and separation. The second approach is the use of experimental correlations. The correlations usually relate the turbulence intensity,Tu , in the free-stream to the momentum-thickness Reynolds number, Reθt, at transition onset. ANSYS has developed a locally formulated transport equation for intermittency, which can be used to trigger transition. The full model is based on two transport equations, one for the intermittency and one for the transition onset criteria in terms of momentum thickness Reynolds number. It is called the ‘Gamma Theta Model’ and is the recommended transition model for general-purpose applications. It uses a new empirical correlation (Langtry and Menter) that has been developed to cover standard bypass transition as well as flows in low free-stream turbulence environments. This built-in correlation has been extensively validated together with the SST turbulence model for a wide range of transitional flows. The transition model can also be used with the BSL or SAS-SST turbulence models as well. A description of the full model can be found in the solver theory chapter. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Because the transition model requires the solution of two extra transport equations, there are additional CPU costs associated with using it. A rough estimate is that for the same grid the transition model solution requires approximately 18 percent additional CPU time compared to a fully turbulent solution. As well, the transition model requires somewhat finer grids than are typically used for routine design purposes. This is because the max grid y+ must be approximately equal to one (that is, wall-function grids cannot be used because they cannot properly resolve the laminar boundary layer) and sufficient grid points in the streamwise direction are needed to resolve the transitional region. For this reason, it is important to be able to estimate when the additional cost of using the transition model in terms of CPU and grid generation time is justified. The relative percentage of laminar flow on a device can be estimated using the following formula, which is based on the Mayle empirical correlation for transition onset. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Where Rext is the transition Reynolds number, Rex is the device Reynolds number, LDevice is the length of the device, V is a representative velocity, and Tu is the freestream turbulence intensity, which can be calculated, as follows: where k is the turbulent kinetic energy. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] For y+ values between 0.001 and 1, there is almost no effect on the solution. Once the maximum y+ increases above 8, the transition onset location begins to move upstream. At a maximum y+ of 25, the boundary layer is almost completely turbulent. For y+ values below 0.001, the transition location appears to move downstream. This is presumably caused by the large surface value of the specific turbulence frequency ω, which scales with the first grid point height. Additional simulations on a compressor test case have indicated that at very small y+ values the SST blending functions switch to k −ε in the boundary layer. For these reasons, very small (below 0.001) y+ values should be avoided at all costs. Effect of increasing y+ for the flat plate T3A test case MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Effect of decreasing y+ for the flat plate T3A test case MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Effect of wall normal expansion ratio for the flat plate T3A test case MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The solution differed significantly from the grid independent one only for the case of 25 streamwise nodes where there was only one cell in the transitional region. Nevertheless, the grid independent solution appears to occur when there is approximately 75 - 100 streamwise grid points. In CFX, when the transition model is active and the high resolution scheme is selected for the hydrodynamic equations, the default advection scheme for the turbulence and transition equations is automatically set to high resolution. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Based on the grid sensitivity study the recommended best practice mesh guidelines are a max y+ of 1, a wall normal expansion ratio of 1.1 and about 75 100 grid nodes in the streamwise direction. Note that if separation induced transition is present, additional grid points in the streamwise direction are most likely needed. For a typical 2D blade assuming an H-O-H type grid, the above guide lines result in an inlet H-grid of 15x30, an O-grid around the blade of 200x80 and an outlet H-grid of 100x140 for a total of approximately 30 000 nodes, which has been found to be grid independent for most turbomachinery cases. It should also be noted that for the surfaces in the out of plane z-direction, symmetry planes should always be used, not slip walls. The use of slip walls has been found to result in an incorrect calculation of the wall distance, which is critical for calculating the transition onset location accurately. Another point to note is that all the validation cases for the transition model have been performed on hexahedral meshes. At this point, the accuracy of the transition model on tetrahedral meshes has not been investigated. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] It has been observed that the turbulence intensity specified at an inlet can decay quite rapidly depending on the inlet viscosity ratio (μt ⁄ μ) (and hence turbulence eddy frequency). As a result, the local turbulence intensity downstream of the inlet can be much smaller than the inlet value .Typically, the larger the inlet viscosity ratio, the smaller the turbulent decay rate. However, if too large a viscosity ratio is specified (that is, >100), the skin friction can deviate significantly from the laminar value. There is experimental evidence that suggests that this effect occurs physically; however, at this point it is not clear how accurately the transition model reproduces this behavior. For this reason, if possible, it is desirable to have a relatively low (that is ≈1 - 10) inlet viscosity ratio and to estimate the inlet value of turbulence intensity such that at the leading edge of the blade/airfoil, the turbulence intensity has decayed to the desired value. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The decay of turbulent kinetic energy can be calculated with the following analytical solution: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Turbulent flows contain a wide range of length and time scales, with large scale motions being generally more energetic than the small scale ones. The prediction of industrially important fluctuating flow problems can be performed using the technique of LES. LES is an approach which solves for large-scale fluctuating motions and uses "sub-grid" scale turbulence models for the small-scale motion. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Before starting an LES simulation, you should consider if it is the most appropriate solution approach. For low Reynolds numbers (Re < 5000) or when it is important to be able to resolve all scales (for example, for transition to turbulent flow), consider DNS if you have a large computer available. For Higher Reynolds numbers, LES might be a suitable option for cases where: The flow is likely to be unstable, with large scale flapping of a shear layer or vortex shedding. The flow is likely to be unsteady with coherent structures (cyclone, flasher). The flow is buoyant, with large unstable regions created by heating from below, or by lighter fluid below heavier fluid (multiphase flows in inclined pipelines). Conventional RANS approach are known to fail (for example due to highly anisotropic turbulence). A good representation of the turbulent structure is required for small-scale processes such as micro-mixing o chemical reaction. The noise from the flow is to be calculated, and especially when the broadband contribution is significant. Other fluctuating information is required (such as fluctuating forces, gusts of winds). You can afford to wait for up to a week for results, using an 8 to 16 processor system. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Geometry for LES Even though there might be symmetries in the geometry and flow, the geometrical model should include the full region, since, while the time averaged flows may be symmetric, the instantaneous flows are not, and restricting the domain could constrain the turbulence. Two-dimensional approximations are particularly poor. Meshing The mesh and timesteps are an inherent part of the model. LES models make use of the grid scale for filtering out the turbulence. If the mesh is anisotropic to resolve a jet, for example, the longer length scale in the flow direction may also have an undue effect on the cross-stream turbulence. For this reason, consider the use of isotropic grids, perhaps using tetrahedral rather than hexahedral elements. Boundary Layers If the boundary layer structure is important, you should resolve it with at least 15 points across the boundary layer and with the first grid point at a position of approximately y+ = 1. Note however, that large aspect rations in the mesh are not suitable for LES. This often results in excessive resolution requirements for boundary layer flows. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Three LES models are available: it is recommended to use the wall-adapted local eddy-viscosity model by Nicoud and Ducros (LES WALE model) as a first choice. This is an algebraic model like the Smagorinsky model, but overcomes some known deficiencies of the Smagorinsky model: the WALE model produces almost no eddy-viscosity in wall-bounded laminar flows and is therefore capable to reproduce the laminar to turbulent transition. Furthermore, the WALE model has been designed to return the correct wall-asymptotic y +3-variation of the subgridscale viscosity and needs no damping functions. In addition to the WALE model, the Smagorinsky model and the Dynamic Smagorinsky-Lilly model (Germano et al. Lilly are available. The Dynamic Smagorinsky-Lilly model is based on the Germano-identity and uses information contained in the resolved turbulent velocity field to evaluate themodel coefficient, in order to overcome the deficiencies of the Smagorinsky model. The model coefficient is no longer a constant value and adjusts automatically to the flow type. However this method needs explicit (secondary) filtering and is therefore more time consuming than an algebraic model. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The representation of the turbulent structures at inlets can be a difficult part of the setup. The detailed properties of the incoming flow can have a strong effect on the development of the downstream solution. This has been observed in turbulent jets, as well as developing boundary layer cases. For other cases, however, the turbulent conditions at the inlet can have relatively little impact on the flow in the device. For example, in a cyclone body, the flow can be essentially determined by very strong anisotropy effects. If the inlet turbulence is felt to play an important role, you should consider: 1. Moving the inlet far upstream of the geometry of interest. This allows the correct turbulent structures to establish themselves before the flow reaches that geometry. Additional obstructions can be placed upstream of geometry to affect the turbulent structures. This procedure, however, will require the simulation of the transition process. ` 2. Using unsteady values computed from a separate LES simulation (pipe flow and channel flows). MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] With the transport of turbulent eddies outside of the computational domain, some recirculation can occur at outlets. Experience shows that if the code is allowed to bring some fluid back into the domain at outlets, it can destabilize the solution. Therefore, you should use outlet boundary conditions rather than openings when using LES. Opening types of boundary conditions allow the flow to come back in, whereas with outlet boundary conditions, the code builds artificial walls at the boundary, when the flow tries to come back in. These artificial walls are later taken away when the flow goes out again. The use of artificial walls at outlets might introduce some un-physical behavior locally, but it increases the robustness of the calculation. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] For simulations initialized with values (rather than from an initial guess field), it is possible to perturb the initial guess by setting an RMS Velocity Fluctuation. This has the effect of kickstarting the solution process. For a velocity distribution Vi, the fluctuation is the quantity: The behavior of the velocity fluctuation is applied according to the following rules when you select Automatic or Automatic with Value as the Initialization option, as well as specify a velocity fluctuation: • If an initial values file is not found, the fluctuation is applied to the initial velocity field. • When an initial values file is used, it is assumed that you are restarting from a previous set of results, and the fluctuation is not applied. If you want to apply a velocity fluctuation on a restart (for example, if the initial guess is a RANS solution), you can override this behavior by setting the expert parameter apply ic fluctuations for les to t. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] On the Solver Control form, it is recommend that you use the Central Difference advection scheme rather than the High Resolution scheme because it is less dissipative and it has provided good answers in CFX. Select the transient scheme as 2nd Order Backward Euler. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] First order fully implicit methods in time are usually too diffusive, and the turbulence is damped out. For highly unstable problems, such as cyclones, lower order methods may work, but the results will be very damped, unless very small timesteps are used. • For accuracy, the average Courant (or CFL) number should be in the range of 0.5-1. Larger values can giv stable results, but the turbulence may be damped. For compressible flows where the acoustic behavior I being modeled (e.g., for noise calculations), this conclusion still holds, but for the CFL number based on the acoustic velocity as well as the convective velocity. • 1,000 - 10,000 timesteps are typically required for getting converged statistics. More steps are required fo second order quantities (for example, variances) than for means. Check the convergence of the statistics. For vortex-shedding problem, several cycles of the vortex shedding are required. • The implicit coupled solver used in CFX requires the equations to be converged within each timestep to guarantee conservation. The number of coefficient loops required to achieve this is a function of the timestep size. Wit CFL numbers of order 0.5-1, convergence within each timestep should be achieved quickly. It is advisable t test the sensitivity of the solution to the number of coefficient loops, to avoid using more coefficient loops (an hence longer run times) than necessary. LES tests involving incompressible flow past circular cylinders indicate that one coefficient loop per timestep is sufficient if the average CFL number is about 0.75. If the physics o geometry is more complicated, additional coefficient loops (3-5) may be required. Timesteps size which require more coefficient loops than this will tend to degrade solution accuracy. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The following values can be written to results files as the solution progresses using the Solver Output tab in CFX-Pre. Although the statistics outlined in the Statistical Reynolds Stresses are useful for any simulation, they are particularly important in the context of LES. Of particular interest, are the Reynolds Stress (RS) components: where ui ′ is the fluctuation of the of the ith velocity component. Statistical Reynolds Stresses In LES runs, Reynolds Stress components are automatically generated using running statistics of the instantaneous, transient velocity field. As outlined above for the calculation of the standard deviation, a Reynolds Stress component can be evaluated using the difference between the running arithmetic average of the instantaneous velocity correlation and the running arithmetic average of the instantaneous velocities as: The noted running averages are also automatically generated for LES runs. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Data sampling for the Statistical Reynolds Stresses begins on the first timestep by default. This is because the transient statistics used in the stress evaluation (that is, the arithmetic averages of velocity and its correlation) start accumulating on the first timestep by default. Sampling for the Statistical Reynolds Stresses is deferred, indirectly, by explicitly creating one of the required transient statistics (that is, the arithmetic averages of velocity or its correlation) and setting the start iteration (the starting timestep index) to a value larger than unity. If different start iterations are set for each of the velocity or velocity correlation averages, then the maximum start iteration set is used for both averages to ensure that the Statistical Reynolds Stresses are correctly evaluated. The Statistical Reynolds Stress variable is evaluated using Equation 4.10 (p. 110) during every timestep, regardless of the start iteration specified for the required velocity-based statistics. This means that if the current timestep is less than the start iteration specified for the velocity-based statistics, then those statistics will be initialized as outlined in Working with Transient Statistics Solver Memory You may find, especially if running averages are to be calculated, that for some cases you will need to increase the amount of memory needed for the calculation by using a memory factor above 1 (typically 1.2). MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] In an attempt to improve the predictive capabilities of turbulence models in highly separated regions, Spalart proposed a hybrid approach, which combines features of classical RANS formulations with elements of Large Eddy Simulations (LES) methods. The concept has been termed Detached Eddy Simulation (DES) and is based on the idea of covering the boundary layer by a RANS model and switching the model to a LES mode in detached regions. Ideally, DES would predict the separation line from the underlying RANS model, but capture the unsteady dynamics of the separated shear layer by resolution of the developing turbulent structures. Compared to classical LES methods, DES saves orders of magnitude of computing power for high Reynolds number flows. Though this is due to the moderate costs of the RANS model in the boundary layer region, DES still offers some of the advantages of an LES method in separated regions. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] It is well known that RANS models does not accurately predict all flow details in massively separated flow regions. In addition, the RANS formulation does not provide any information on turbulent flow structures and spectral distribution, which might be of importance to predict flow-induced noise or vibrations. In these cases, DES can provide valuable details far exceeding RANS simulations. Typical examples of DES simulations are: • Flow around non-aerodynamic obstacles (buildings, bridges, etc.) • Flow around ground transport vehicles with massively separated regions (cars, trains, trucks) • Flow around noise generating obstacles (car side-mirror, etc.) • Massively separated flow around stalled wings MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] On the other hand, DES is a computer intensive method because large (detached) turbulent structures need to be resolved in space and time. While the grid resolution requirements are not significantly higher than RANS for simulations, the time resolution imposes high CPU demands. In CFX, steadystate solutions can typically be obtained within 100-200 iterations. A typical DES simulation requires several thousands timesteps using three coefficient loops each. DES is therefore at least one order of magnitude more computer intensive than RANS models. DES does not allow the use of symmetry conditions, as turbulent structures are usually not symmetric. Two-dimensional and axisymmetric simulations are not feasible, as turbulent structures are always three-dimensional. Hence, you should carefully weigh the need for additional information against the required resources. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The main issue with geometry is that 2D or axisymmetric simulations are not visible. In addition, in most cases, symmetry or periodicity conditions are not allowed. As an example, RANS simulations allow the use of a half model for a car simulation. As the turbulent structures are asymmetric, this is not possible in DES. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] There are many requirements in terms of grid generation, which depend on the selection of the specific formulation of the DES model. The grid resolution requirement in the attached boundary layers is the same as that of a regular RANS solution (10-15 nodes in the boundary layer). In the DES region, the grid has to be designed in all three space dimensions to allow at least the resolution of the largest turbulent structures. As the largest grid spacing is used in the switch for the DES limiter, it is essential to have sufficient resolution in the spanwise direction (if it exists). One of the main issues of DES models is the danger to produce grid-induced separation due to an activation of the DES limiter in the RANS region. The zonal formulation used in CFX helps to avoid the severity of this potential limitation. For a cylinder in cross-flow, 50 nodes were used in the spanwise direction for an extension of 2 diameters. For the Ahmed car body, 70 nodes were used in the cross-flow direction. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Timestep selection is a sensitive issue, as it determines the quality of the resolution of the turbulent structures. In most cases, a typical shedding frequency can be estimated for the largest turbulent structures. An example is a cylinder in cross-flow. For a cylinder, the Strouhal number is of the order of: In the CFX simulation the free stream velocity is U=46 m/s and the diameter is D=0.37 m. This results in a frequency f=24.8 1/s. A timestep of Dt=10−4 s is sufficient to resolve the turbulent structures on the given grid. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] In almost all cases, the same boundary conditions as in RANS simulation can be applied. Exceptions are symmetry or periodicity conditions, which may not be satisfied by the turbulent structures. In most cases, RANS inlet conditions can be applied, particularly for flows without profile distributions at the inlet, as mostly used in industrial flows. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] It is recommended to start the DES simulation from a converged RANS solution. For comparison purposes, it is useful to use the same RANS model as in the DES formulation (in CFX this means the SST model). The RANS solution supplies to the DES formulation important elliptic information on the pressure field, which might take a long time to build up in an unsteady simulation. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Due to high computing costs, it is essential to monitor a DES run to ensure that it approaches an appropriate solution. It is recommended to check the blending function for DES model during the simulation (every 50-100 timesteps). In regions where the function is zero, the LES model is used, and the region where its value is one, the RANS model is activated. Figure 4.6, “Blending Function for DES Model for Flow Around Cube.” shows a typical example for the flow around a cube. The blue region behind the cube is governed by the LES part, and the red region by the RANS model. This is desirable for this case as only the structures behind the obstacle are of interest. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] A second variable to monitor is the invariant: where S is the absolute value of the strain rate (S = Shear Strain Rate in CFD-Post) and Ω is the absolute value of the vorticity. Note that the vorticity has to be activated in order to be available in CFD-Post. The following lines are to be added to the solver CCL: LIBRARY: VARIABLE: vorticity Output to Postprocessor = Yes END END MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] In CFD-Post, you have to define a user variable based on the following expression: LIBRARY: CEL: EXPRESSIONS: Invariant = Vorticity^2 - Shear Strain Rate^2 END END END USER SCALAR VARIABLE:VelGradInvariant Boundary Values = Conservative Expression = Invariant Recipe = Expression END MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 Strain Rate Invariant for Flow Around Cube [email protected] Unsteady flow from DES over backward facing step using insufficient spanwise grid resolution MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The Scale-Adaptive Simulation (SAS) The Detached Eddy Simulation is a combination of RANS and LES methods. In an alternative approach, a new class of URANS models has been developed which can provide a LES-like behavior in detached flow regions, Menter and Egorov, Egorov and Menter. The Scale-Adaptive Simulation (SAS) concept is based on the introduction of the von Karman length-scale into the turbulence scale equation. The information provided by the von Karman length-scale allows SAS models to dynamically adjust to resolved structures in a URANS simulation, which results in a LES-like behavior in unsteady regions of the flowfield. At the same time, the model provides standard RANS capabilities in stable flow regions. isosurfaces where S2−Ω2=105[s^−2] for a cylinder in cross flow (Re=3.6x106), calculated using the SST model (URANS) and the SAS-SST model. The URANS simulation produces only the large-scale unsteadyness, whereas the SAS-SST model adjusts to the already resolved scales in a dynamic way and allows the development of a turbulent spectrum in the detached regions. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Resolved structures for cylinder in cross flow (top: URANS; bottom: SAS-SST) MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The original version of the SST-SAS model has undergone continued evolution. This model is still available in ANSYS CFX, but the latest version of the SAS model is now the default model. The SAS method is an improved URANS formulation, with the ability to adapt the length scale to resolved turbulent structures. In order for the SAS method to produce a resolved turbulent spectrum, the underlying turbulence model has to go unsteady. This is typically the case for flows with a global instability, as observed for flows with large separation zones, or vortex interactions. There are many technical flows, where steady state solutions cannot be obtained or where the use of smaller timesteps results in unsteady solutions. One could argue that the occurrence of unsteady regions in a RANS simulation is the result of a nonlocal interaction, which cannot be covered by the single-point RANS closure. All these flows will benefit from the SAS methodology. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] On the other hand, there are classes of flows where the SAS model will return a steady solution. The two main classes are attached or mildly separated boundary layers and undisturbed channel/pipe flows. These flows (or areas in a complex flow domains) will be covered by the RANS formulation. This behavior of SAS is ideal for many technical applications, as flows for which the model produces steady solutions are typically those, where a well-calibrated RANS model can produce accurate results. Contrary to DES, SAS cannot be forced to go unsteady by grid refinement. However, it has been shown in the backstep simulation above that enforcing unsteadiness by grid refinement does require an in-depth understanding of the flow, the turbulence model and its interaction with the grid to produce reliable results. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Nevertheless, there will be cases where the grid sensitivity of DES will be advantageous, as it allows unsteady simulations where SAS might produce a steady state flow-field. This leads to the following hierarchy of models of increased complexity, which can be used as a guideline in order to estimate the needed effort: 1. URANS 2. SAS 3. DES 4. LES MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Due to the similar functionality of the SAS-SST and DES model, most of the guidelines which have been given for the DES model regarding timestepping, boundary conditions and initialization are also valid for the SAS-SST model. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Buoyancy Turbulence is an option in CFX-Pre that is available for some turbulence models when buoyancy is being modeled. When the option is set to Production, a buoyancy production term is included in the equation for k. When the option is set to Production and Dissipation, a buoyancy production term is included in the equations for k, ε, ω (as applicable). See Buoyancy Turbulence and the accompanying text, which describe the treatment for the k −ε and k −ω models. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Near a no-slip wall, there are strong gradients in the dependent variables. In addition, viscous effects on the transport processes are large. The representation of these processes within a numerical simulation raises the following problems: • How to account for viscous effects at the wall. • How to resolve the rapid variation of flow variables which occurs within the boundary layer region. Experiments and mathematical analysis have shown that the near-wall region can be subdivided into two layers. In the innermost layer, the so-called viscous sublayer, the flow is almost laminar-like, and the (molecular) viscosity plays a dominant role in momentum and heat transfer. Further away from the wall, in the logarithmic layer, turbulence dominates the mixing process. Finally, there is a region between the viscous sublayer and the logarithmic layer called the buffer layer, where the effects of molecular viscosity and turbulence are of equal importance. The figure below illustrates these subdivisions of the near-wall region. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Assuming that the logarithmic profile reasonably approximates the velocity distribution near the wall, it provides a means to numerically compute the fluid shear stress as a function of the velocity at a given distance from the wall. This is known as a ‘wall function' and the logarithmic nature gives rise to the well known ‘log law of the wall.‘ Two approaches are commonly used to model the flow in the near-wall region: • The wall function method uses empirical formulas that impose suitable conditions near to the wall without resolving the boundary layer, thus saving computational resources. All turbulence models in CFX are suitable for a wall function method. The major advantages of the wall function approach is that the high gradient shear layers near walls can be modeled with relatively coarse meshes, yielding substantial savings in CPU time and storage. It also avoids the need to account for viscous effects in the turbulence model. • The Low-Reynolds-Number method resolves the details of the boundary layer profile by using very small mesh length scales in the direction normal to the wall (very thin inflation layers). Turbulence models based on the ω-equation, such as the SST or SMC-ω models, are suitable for a low-Re method. Note that the low-Re method does not refer to the device Reynolds number, but to the turbulent Reynolds number, which is low in the viscous sublayer. This method can therefore be used even in simulations with very high device Reynolds numbers, as long as the viscous sublayer has been resolved. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The computations are extended through the viscosity-affected sublayer close to the wall. The low-Re approach requires a very fine mesh in the near-wall zone and correspondingly large number of nodes. Computer-storage and runtime requirements are higher than those of the wall-function approach and care must be taken to ensure good numerical resolution in the near-wall region to capture the rapid variation in variables. To reduce the resolution requirements, an automatic wall treatment was developed by CFX, which allows a gradual switch between wall functions and low-Reynolds number grids, without a loss in accuracy. Wall functions are the most popular way to account for wall effects. In CFX, Scalable Wall Functions are used for all turbulence models based on the εequation. For k −ω based models (including the SST model), an Automatic nearwall treatment method is applied. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] In CFX-5.4.1 and earlier, standard wall functions were used. These have been replaced by scalable wall functions as the default but standard wall functions are still available for backward compatibility. You should not use standard wall functions, as they offer no advantage over the scalable wall functions. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Scalable wall functions overcome one of the major drawbacks of the standard wall function approach in that they can be applied on arbitrarily fine meshes. If the boundary layer is not fully resolved, you will be relying on the logarithmic wall function approximation to model the boundary layer without affecting the validity of the scalable wall function approach. If you are not interested in the details of the boundary layer, then it may not be worth fully resolving it. However, if you have produced a very fine near-wall mesh to examine details of the boundary layer, then you should use the SST model with Automatic near-wall treatment to take advantage of the additional effect in the viscous sublayer. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Automatic near-wall treatment automatically switches from wall-functions to a low-Re near wall formulation as the mesh is refined. One of the well known deficiencies of the k −ε model is its inability to handle low turbulent Reynolds number computations. Complex damping functions can be added to the k −ε model, as well as the requirement of highly refined near-wall grid resolution (y+ < 0.2) in an attempt to model low turbulent Reynolds number flows. This approach often leads to numerical instability. Some of these difficulties may be avoided by using the k −ω model, making it more appropriate than the k −ε model for flows requiring high near-wall resolution (for example, high wall heat transfer, transition). However, a strict low-Reynolds number implementation of the model would also require a near wall grid resolution of at least y+ < 2. This condition cannot be guaranteed in most applications at all walls. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] For this reason, a new near wall treatment was developed by CFX for the k −ω based models that allows for a smooth shift from a low-Reynolds number form to a wall function formulation. This near wall boundary condition, named automatic near wall treatment in CFX, is used as the default in all models based on the ωequation (standard k −ω, Baseline k −ω, SST, ω-Reynolds Stress). To take advantage of the reduction in errors offered by the automatic switch to a low-Re near wall formulation, you should attempt to resolve the boundary layer using at least 10 nodes when using these models. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] A user-defined wall function transfer coefficient may be applied to any wall boundary condition. This is accomplished by adding the following CCL data to the wall boundary condition, in the HEAT TRANSFER block or ADDITIONAL VARIABLES block, as appropriate: TURBULENT WALL FUNCTIONS: Option = User Defined Wall Function Transfer Coefficient = [expression goes here] END Note that the parameter Wall Function Transfer Coefficient must be a normalized value having dimensions of [L T^-1] (that is, the units of kinematic diffusivity divided by length). The definition of the two different types of wall function transfer coefficient and their normalized values are presented next, followed by examples of CCL implementations. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Heat Flux heat flux = C* (Vertex Temperature - Wall Temperature) where C represents the wall heat flux transfer coefficient before normalization. The Wall Function Transfer Coefficient parameter is then calculated as Wall Function Transfer Coefficient = C / (density * Cp) Additional Variable Flux additional variable flux = C * (Vertex AV - Wall AV) where C represents the wall scalar flux transfer coefficient before normalization. The Wall Function Transfer Coefficient parameter is then calculated as: Wall Function Transfer Coefficient = C / density MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Example of User-Defined Wall Function for Heat Flux In the following example, a user-defined wall function, in the form of a wall function transfer coefficient for heat flux, is defined and applied to a wall boundary condition. LIBRARY : CEL: EXPRESSIONS: sn = 1.E-20 prandtl = viscosity * Cp / cond pryplus = prandtl * yplus beta1 = (3.85 * prandtl^1/3 -1.3) ^ 2 gamma = 0.01 * prandtl * yplus ^ 3 / \ ( 1./(yplus*prandtl^3+sn) + 5.0) tplussub = pryplus tpluslog = max(2.12*loge(pryplus+sn)+beta1,0.) tplus = tplussub*exp(-gamma) + tpluslog*exp(-1./(gamma+sn)) twftfc1 = ustar / (tplus + sn) END END END MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] FLOW DOMAIN: flatplate BOUNDARY : Wall Boundary Type = WALL Coord Frame = Coord 0 BOUNDARY CONDITIONS : MASS AND MOMENTUM : Option = No Slip Wall END WALL ROUGHNESS : Option = Rough Wall Roughness Height = 0.019 [in] END HEAT TRANSFER : Option = Fixed Temperature Fixed Temperature = 100[C] TURBULENT WALL FUNCTIONS: Option = User Defined Wall Function Transfer Coefficient = twftfc1 END END END END END MAPNAEND Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The CCL implementation of a user-defined wall function for an Additional Variable is similar to that shown in the example for heat flux. The CCL block in the boundary condition is (using a specified quantity as an example): FLOW DOMAIN: flatplate BOUNDARY : Wall BOUNDARY CONDITIONS : ADDITIONAL VARIABLE: my_av Wall Function Transfer Coefficient = 10 [m s^-1] END END END END END MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The near wall treatment which has been discussed above (Scalable Wall Functions, Automatic Wall Treatment) is appropriate when walls can be considered as hydraulically smooth. For rough walls, the logarithmic profile exists, but moves closer to the wall. The near wall treatment becomes more complex, since it depends now on two variables: the dimensionless wall distance y+ and the roughness height. The roughness height which must be specified in the wall boundary section ,is the equivalent sand grain roughness height. Note that the sand-grain roughness is not equal to the geometric roughness height of the surface under consideration. Wall friction depends not only on roughness height but also on the type of roughness (shape, distribution, etc.). Therefore, one must determine the appropriate equivalent sand-grain roughness height. Details on the formulation of the rough wall treatment for turbulence models based on the ε and ω-equation can be found in Treatment of Rough Walls in the ANSYS CFXSolver Theory Guide. If the ANSYS-CFX two-equation transition model is used together with rough walls, then the roughness correlation must be turned on in the GUI. This correlation requires the geometric roughness height as input parameter. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Yplus (y+) is the dimensionless distance from the wall. It is used to check the location of the first node away from a wall. This was more important when standard wall functions were used, as you had to avoid y+ values smaller than approximately 20. With the scalable wall functions and the automatic wall treatment, these values are only provided for information on the near wall resolution. Two variables related to y+ are output to the results file, Yplus and Solver Yplus. • The variable Yplus is based on the distance from the wall to the first node and the wall shear stress. This is the usual definition in the CFD community. • The variable Solver Yplus is used internally by the CFX-Solver. It uses different definitions for the wall distance of the first wall point. This variable is available for backwards consistency, but is of little use to you. There is usually no need for you to work with the y+ used internally (Solver Yplus). The information required to judge the near wall mesh spacing should be based on the standard y+ (Yplus) definition. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] One of the most essential issues for the optimal performance of turbulence models is the proper resolution of the boundary layer. In this section, two criteria are suggested for judging the quality of a mesh: • Minimum spacing between nodes in the boundary layer • Minimum number of nodes in the boundary layer These are simple guidelines for the generation of meshes which satisfy the minimal requirements for accurate boundary layer computations. Minimum Node Spacing The goal is to determine the required near wall mesh spacing, Δy, in terms of Reynolds number, running length, and a Δ y+ target value. This target value depends on the flow type and the turbulence model in use, or in other words the near wall treatment in use. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Determination of the Near Wall Spacing The estimates will be based on correlations for a flat plate with a Reynolds number of: with characteristic velocity U∞ and length of the plate L. The correlation for the wall shear stress coefficient, cf , is given by White where x is the distance along the plate from the leading edge. The definition of Δ y+ for this estimate is: with Δy being the mesh spacing between the wall and the first node away from the wall. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Using the definition uτ can be eliminated in Equation 4.15 to yield: cf can be eliminated using Equation 4.14 to yield: Further simplification can be made by assuming that: where C is some fraction. Assuming that C1⁄14≈1 , then, except for very small Rex, the result is: This equation allows us to set the target Δ y+ value at a given x location and obtain the mesh spacing, ΔyCourse-Turbulence-Rev-01-Januray2012 for nodes in the boundary layer. MAPNA Generator Co- CFX Introductory [email protected] Minimum Number of Nodes Goal A good mesh should have a minimum number of mesh points inside the boundary layer in order for the turbulence model to work properly. As a general guideline, a boundary layer should be resolved with at least: where Nnormal, min is the minimum number of nodes that should be placed in the boundary layer in the direction normal to the wall. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] Formulation The boundary layer thickness δ can then be computed from the correlation: to be: The boundary layer for a blunt body does not start with zero thickness at the stagnation point for Rex. It is, therefore, safe to assume that Reδ is some fraction of ReL, say 25%. With this assumption, you get: You would, therefore, select a point, say the fifteenth off the surface (for a low-Re model, or 10th for a wall function model) and check to make sure that: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The near wall treatment which has been discussed above (Scalable Wall Functions, Automatic Wall Treatment) is appropriate when walls can be considered as hydraulically smooth. Surface roughness can have a significant effect on flows of engineering interest. Surface roughness typically leads to an increase in turbulence production near the wall. This in turn can result in significant increases in the wall shear stress and the wall heat transfer coefficients. For the accurate prediction of near wall flows, particularly with heat transfer, the proper modeling of surface roughness effects is essential for a good agreement with experimental data. Wall roughness increases the wall shear stress and breaks up the viscous sublayer in turbulent flows. where B=5.2. The shift ΔB is a function of the dimensionless roughness height, h +, defined as h+ =huτ / υ. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] For sand-grain roughness, the downward shift can be expressed as: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] It has been shown that a technical roughness, which has peaks and valleys of different shape and size, can be described by an equivalent sand-grain roughness Depending on the dimensionless sand-grain roughness hs+, three roughness regimes can be distinguished: MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected] The viscous sublayer is fully established near hydraulically smooth walls. In the transitional roughness regime the roughness elements are slightly thicker than the viscous sublayer and start to disturb it, so that in fully rough flows the sublayer is destroyed and viscous effects become negligible. Rough Wall Treatment for Turbulence Models Based on the Dissipation Equation: The Scalable Wall Function approach neglects the viscous sublayer and limits the value used in the logarithmic formulation by a lower value of y+ =max(y* ,11.06) . This limiter must be combined with the formulation Equation 2.238 (p. 92) for the rough wall treatment and reads: y+=max(y* , hS+/ 2, 11.06) Since the automatic wall treatment works together with the transition model, it is the default rough wall treatment. This default Automatic setting for the Wall Function option can be set on the Fluid Models tab of the Domain details view in CFX-Pre. The CFX-Solver checks internally if a roughness height has been specified for a wall and then uses the proper wall treatment: when a roughness height exists, then the automatic rough wall treatment is used, otherwise the automatic smooth wall treatment. MAPNA Generator Co- CFX Introductory Course-Turbulence-Rev-01-Januray2012 [email protected]
© Copyright 2025