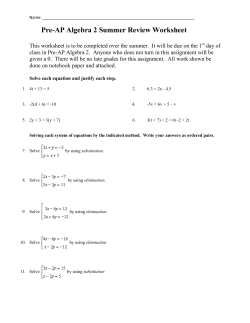
Ch 6 Linear Functions
1
Math 10 C (AP)
Chapter 6: Linear Functions
Page 330
Review:
−42𝑚6 𝑛−3 𝑝5
(5𝑎4 𝑏 6 )(12𝑎𝑏𝑐)0 (−2𝑎2 𝑏𝑐 5 )
Lesson 6.1
6𝑚11 𝑛−5 𝑝5
Slope of a Line
𝑥 6𝑚
2
𝑥4
3
(𝑥 2𝑚+3) (𝑥 𝑚 )
Page 333
The slope – denoted by m – refers to the steepness of the line. The steeper the line, the greater the slope.
In order to calculate the slope (m) of a line we must find the vertical distance (the rise) and the horizontal
distance (the run)
In the following diagram, we are looking to calculate the slope of the line AC. In order to do that, we must first
find the vertical change of the line between points A and points C, which we call the rise. On the diagram, the
rise is drawn between points B and C. Counting the change from point B up to C, we calculate the rise to be 6.
We must then calculate the horizontal distance of the line, which in this case is the distance from point A to B.
This distance is called the run and in our diagram the run is 8.
We can then say that the slope of this line is
slope
rise
run
m
6
8
therefore, m
3
4
Typically we leave the slope as a reduced fraction and not in decimal form.
2
Math 10 C (AP)
Example #1
F
D
C
B
A
When you are given a line, you can select any two points along the line. (Why is it possible to select any two
random points along the line?)
Count the following rise and runs on the graph.
Slope of AB
mAB
rise
run
Slope ofAC
mAC
rise
run
Slope of CD
mCD
rise
run
Slope of AE
mAE
rise
run
Slope of BD
mBD
rise
run
Example #2
What do we notice to be different about the graph?
When we go to calculate the slope and we start at the first point to calculate the
vertical rise, we instead have to count down to the second point, instead of up.
Therefore, when we give the rise, we put is in as a negative.
slope
5
m
4
rise
run
Any line that is decreasing to the right will have a negative slope.
3
Math 10 C (AP)
Example #3
Looking at the first example again–
When we talk about the rise (or the change in vertical distance) we are
essentially looking at the change in the y coordiates of each of the
points. Point A is located at (–3, –2) and point C is located at (5, 4).
So the rise is a change from –2 (the y value of point A) to 4 (the y
value of point c). Finding the difference in these two values, 4 – (–2) =
6, will give us the Rise.
In the same respect finding the difference in the x values, 5– (–3) = 8,
will give you the Run.
Therefore,
rise
run
changeinyvalues
m
changeinxvalues
y
m
x
y y
m 2 1
x2 x1
slope
y2 – the y value of the 2nd point
y1– the y value of the 1st point
x2– the x value of the 2nd point
x1– the x value of the 1st point
Example #4
What do we notice to be different about the graph? Calculate the slope.
Does it matter which point you select as point 1 and which you select as point 2?
4
Math 10 C (AP)
Slope (m) = how steep a line is.
=
rise
run
=
change in y
change in x
=
y 2 y1
for points (x1, y1) and (x2, y2).
x 2 x1
Example #5
Find slopes for each of the following.
a.
b.
c.
d.
e. (6.1, 7.8) & (8.1, 21.2)
f. (2m2, 4m) & (19m2, 7m)
***
The slope of a horizontal line (parallel to the x–axis) is ___________.
***
The slope of a vertical line (parallel to the y–axis) is __________.
***
Lines rising to the right have a _________ slope.
***
Lines falling to the right have a _________ slope.
5
Math 10 C (AP)
Slope of a line or segment.
Slope (m) = how steep a line is.
=
rise
change in y
y y
=
= 2 1 for points (x1, y1) and (x2, y2).
change in x
x 2 x1
run
Example #6
Given (k, 5) and (4, 2) with m =
3
, find k.
5
Example #7
Given (3, 2) and (x, y) and m =
2
, find a possible value for x and y.
5
Example #8
Are the following set of points collinear (lie on the same line)?
a. A (2, 7), B (5, 5), C (1, 19) *** Choose any two pairs.
b. A (2a, 3b), B (12a, 2b), C (10a, 3b), a, b 0.
6
Math 10 C (AP)
Example #9
If (5, 4), (1, 2) and (5, k) are collinear, find the value of k.
Assignment: Pg. 339 # 4, 5, 6, 7ac, 8, 9, 13 i iv, 14, 15, 16, 17
Remember…
x – intercept(s)
This is the point where the function or relation crosses the x-axis.
Can also be referred to as the horizontal intercept
y is always equal to zero. 𝒚 = 𝟎
y – intercept(s)
This is the point where the function or relation crosses the y-axis.
Can also be referred to as the vertical intercept.
x is always equal to zero. 𝒙 = 𝟎
We can determine the rate of change (SLOPE) and y-intercept for a linear function and from those, we can
find the equation that represents the function.
𝑦 = 𝑠𝑙𝑜𝑝𝑒 ∙ 𝑥 + 𝑦 𝑖𝑛𝑡𝑒𝑟𝑐𝑒𝑝𝑡
𝑦 = 𝑚𝑥 + 𝑏
7
Math 10 C (AP)
Slope of a Line (con’t)
Lesson 6.1
Review
Page 332
Express each radical as a power.
3
a)
62
b)
2 5
Meet Slope Man!
When talking about slope, there are only 4 possible slopes,
which Slope Man shows us.
Positive, Negative, Zero and Undefined
Example #1
5
Given point (2, 1) and slope m
, graph the line.
4
plot point (2, 1)
then go down 5 (5) and to the right 4 (4) to get a second point.
then draw line through the two points.
Example #2
Graph line through point (4, 3) with slope 3.
8
Math 10 C (AP)
Example #3
Given equation of line, find the slope.
a. Find slope of 2x + y = 10.
b. Find slope of 3x 2y 14 = 0.
Example #4
For 20 hours of work you were paid $280. For 32 hours of work you were paid $412.
a. What were you paid per hour for the hours between 20 and
32? (This is a great example of slope as rate of change.)
b. Find your salary for 46 hours. (46)
c. Find your fixed salary. 𝑆(0)
d. Find the number of hours worked if your salary is $445. 𝑆(𝑡) = 445
9
Math 10 C (AP)
Slope as a rate of change.
slope of a line shows how the dependent variable changes relative to the independent variable.
Example #5
Determine the rate of change for the graph to the right if the dependent
axis represents distance travelled as a function of time.
Example #6
The value of Canadian Maple products increased from 35.1 million in 1980 to 90.6 million in 1995.
a. Find the average rate of change to the nearest 100 000/y.
b. Predict the value of Canadian Maple products in 2006.
Example #7
Mark’s commission for selling a condo is a percent of the selling price. If he receives $2510 commission for
selling a condo for $48 000 and a $3500 commission for a selling price of $70 000:
a. What is his rate of commission?
c. What is his fixed part of his salary?
b. What is the selling price if the commission is $4490?
10
Math 10 C (AP)
Extra Practice… You Try
1. A line of slope
3
passes through (1, 2) and (s, s + 1). What are the possible values of s?
2
{5}
2. Saskatoon is about 700 km from Winnipeg. The elevation of Saskatoon is 484 m. The elevation of
Winnipeg is 232 m.
a. Write 2 ordered pairs of the form (distance, elevation) to represent the above. {(700, 484), (0, 232)}
b. Assuming the elevation is constant, find the elevation 250 km from Winnipeg. {322 m}
3. A cab company charges $11.50 for a trip of 10 km and $22.75 for a trip of 25 km.
a. Find the charge/km.
{$0.75}
b. How much would you be charged for a trip of 44 km?
{$37}
c. What is the fixed charge?
{$4}
11
Math 10 C (AP)
Review Questions… Keep Going
1. Given A (3, 6) and B (1, 5) find the slope of AB .
2. A line passes through (5, 4) with slope
1
. Find another point on the line.
3
3. Graph the following.
a. passes through (2, 5) with slope undefined.
b. passes through (3, 4) with slope =
5
.
6
4. Determine slopes of the following lines.
a.
ANSWERS: 1.
b. 3y + 2x = 6
1
4
2. (-2, 3)
3.
ASSIGNMENT: Page 340 # 9, 12, 18–22, 23, 24, 27
4. a.
3
7
b.
2
3
12
Math 10 C (AP)
Lesson 6.2 ~ Slope of Parallel and Perpendicular Lines
Page 344
Review: State the domain and range of the following functions in both set notation and interval notation.
Parallel lines ~ Lines on the same flat surface that do not intersect.
They have the same slope.
Perpendicular lines ~ Lines or line segments that intersect at right angles.
The slopes are opposite reciprocals of each other.
Example #1
Find slopes of parallel and perpendicular lines to:
Parallel
m=
3
5
m6
y=
7
x 3
3
m0
4x + 5y 11 = 0
Perpendicular
13
Math 10 C (AP)
Example #2
a) Given A (0, 2), B (3, 4), C (2, 4) and D (8, 1), show that AB CD .
b) Given A (2, 3), B (6, 5), C (1, 4) and D (3, 6), is AB CD ?
Example #3
A line AB passes through the points A (2, 8) and B ( –3, –5).
A. Sketch the line.
B. Sketch a line parallel to line AB.
C. Sketch a line perpendicular to line AB
Example #4
Which of the following lines are:
a. 3x + 4y 24 = 0
i. parallel?
b. 4x + 3y 16 = 0
ii. perpendicular?
c. 3x 4y + 10 = 0
d. 6x + 8y + 15 = 0
14
Math 10 C (AP)
Example #5
Find y in A (3, 8), B (1, 2), C (4, 1) D (2, y) such that:
a. AB CD
b. AB CD
Example #6
Given m1 m 2 , find k.
a.
3 k
,
5 20
b.
k 3
,
5 2
Example #7
For what value of k are the lines 3x 4y + 4 = 0 and kx + 3y + 10 = 0 perpendicular?
Example #8
Find the equation of the line through A (1, 5) which is perpendicular to 3x 2y 12 = 0.
15
Math 10 C (AP)
Example #9
Find the equation of a line which is parallel to 3x + 2y + 8 = 0 and has a y–int of 2.
Assignment: Page 349 ~ #3–11 some from each, #13, 16, 19
and
Page 353 ~ 1 through 8
16
Math 10 C (AP)
Lesson 6.4
Slope-Intercept Form of the Equation for a Linear Function
Review: Factor completely.
a) 9x3 – 36x
b)
27a4 – 147
Page 357
c)
100ab2 – 25a
𝒚 = 𝒎𝒙 + 𝒃
b is y - intercept
m is Slope
Example #1
Find the y–intercept and slope for the following.
a) y = 5x + 4
b) 3x + 2y 12 = 0
Example #2
Determine equations of the following lines given slope and point.
a. m = 2; (0, 3)
b. m = 3; y–intercept is 4
d. m = 5 and same y–intercept as 3x 4y + 32 = 0
c. m =
5
; (0, 6)
6
17
Math 10 C (AP)
Example #3
Given the following equations, find the slope and the y intercept to sketch the graphs.
3
x+1
4
a) y = –5x + 4
b) y =
d) y = –3
e) y = x – 1.25
c) y = –4x
f) x = 12
Example #4
Determine equations of the following.
a.
b. m =
1
2
,b=
3
5
18
Math 10 C (AP)
Example #5
The equation of a line is y = 2x + b. Find b if the line passes through the point:
a. (2, 6)
b. (1, 3)
Example #6
Given the following graphs, find the equation of the lines in slope intercept form.
10
5
-10
-5
0
-5
-10
5
10
19
Math 10 C (AP)
Example #7
Complete the following chart.
Table of Values
x
0
1
2
y
1
3
5
x
y
x
0
2
4
y
–1
0
1
x
y
Graph
Slope (m)
y–intercept (b)
Equation
y = mx + b
y 2 x 1
Assignment ~ Pg. 362 # 4bdef, 5ace, 6ab, 7def, 8, 9, 11, 12cd,13, 14, 15, 16, 17a, 22, 23
20
Math 10 C (AP)
Lesson 6.4 Continued
Example #1 ~ Problem Solving
The SCIL group at Salisbury rent a portable dunk tank as a fundraiser activity. Students pay for the chance to
hit a target and dunk Mr. Chandler into the water. The dunk tank costs $300 to rent and the SCIL group intends
to charge $1.50 per ball at the event.
a) Write an equation for the profit, P dollars, as a function of the sale of balls (b) at the event.
b) Suppose 50 balls were purchased to try and dunk Mr. Chandler. How much profit would the group
raise?
c) If the group raised $350 profit, how many balls were purchased?
d) How many balls need to be purchased in order for the group to break even?
We can graph linear equations using any two points, x & y–intercepts, and slope & point (usually yintercept).
Example #2
Graph using any two points. (Create a small table of values.)
a. y = 2x + 3
x
y
b. 4x y 1 = 0
x
y
c. 3x + 4y + 8 = 0
x
y
21
Math 10 C (AP)
Example #3
Graph using x and y–intercepts.
a. 3x + 4y + 6 = 0
b. 3x y 6 = 0
c. x + 5y 15 = 0
Example #4
Graph using slope and point (usually y–intercept).
a. 3x 4y = 12
Assignment: Pg. 376 ~ 1, 2, 3
b. y = 6x + 5
c. 4x + 2y 5 = 0
22
Math 10 C (AP)
Lesson 6.5: Slope-Point Form of the Equation for a Linear Function
Review ~ If 𝑓(𝑥) = 𝑥 2 − 5, determine…
𝑓(1)
𝑓(−2)
𝑓(𝑥) = 4
Page 365
𝑓(𝑥) = −6
We saw that slope is calculated using the formula
rise
slope
run
change in y values
m
change in x values
y
x
y y
m 2 1
x2 x1
m
If we then multiplied both sides of the equation by x2 x1 , thus not changing the equation only rearranging it,
we would have
( x2 x1 )m ( x2 x1 )(
y2 y1
)
x2 x1
( x2 x1 )m y2 y1
This equation is called the slope–point form of a non–vertical line through point (𝒙𝟏 , 𝒚𝟏 ).
The slope–point form is commonly written as y y1 m x x1 .
(𝑦 − 𝑦1 ) = 𝑚(𝑥 − 𝑥2 )
23
Math 10 C (AP)
Example #1
a) Write the equation of a line through (–2, 5) with a slope of –3.
b) Express the equation in slope–intercept form
Example #2
Use slope–point form to write an equation of the line through (3, –4) and (5, –1).
Convert the above equation to slope / y-intercept form of the equation.
Assignment: Pg. 372 # 4abf, 5cd, 7bc, 8, 9i ii, 10, 11ab, 13, 15, 18, 19, 22, 24
24
Math 10 C (AP)
Lesson 6.6 ~ General Form of the Equation for a Linear Relation
Page 377
Review: Factor fully.
75y2 – 120y + 48
12x – 15xy – 18xy2
The general form of a linear equation is Ax + By + C = 0, where A is a whole number and B and
C are integers.
Get zero on
one side
1, 2, 3, 4, 5… No negatives,
fractions or decimals
You can convert a linear equation from one form to another by applying the rules of algebra.
Example #1
2
Convert y =
x + 6 into general form
3
Example #2
Convert x – 4y + 2 = 0 into slope-intercept form (isolate for y).
Example #3
Convert y + 4 =
3
(x – 3) into general form.
2
25
Math 10 C (AP)
Example #4
For the linear equation 2x – 3y – 6 = 0
a) State the x-intercept of the graph
b) State the y-intercept of the graph
c) Use the intercepts to graph the line
10
5
-10
-5
0
5
10
-5
-10
Example #5
Given the linear equation 3x + 4y + 12 = 0, find the slope and the x and y intercepts.
26
Math 10 C (AP)
Example #6
When an equation is written in general form Ax + By + C = 0, what is the effect on the graph if
a) A = 0
b) B = 0
10
5
-10
-5
0
5
10
-5
-10
Assignment: Pg. 384 # 4, 5ab, 6cd, 7a, 8i ii, 10, 12ac, 13cd, 16, 18ac, 21, 23, 26, 27
27
Math 10 C (AP)
Review
1. Determine equations in the form Ax + By + C = 0 or y = mx + b for the following lines.
a. slope =
4
and passes through (2, 5)
3
b. passing through (1, 3) and (4, 5).
c. slope is undefined and passes through (2, 10).
2
x4.
5
e. same y–intercept as 2x 7y = 14 and perpendicular to 3x + 7y 8 = 0.
d. same x–intercept as 3x 7y + 21 = 0 and parallel to y =
f. parallel to the x–axis and passing through (5, 6)
2. Graph.
a. 2x = 3y 6
(x and y–int)
b. y + 4 = 0
c. 2x + 3y + 7 = 0
(any two points)
3. Quadrilateral ABCD is given by A (5, 1), B (1, 3), C (3, 3) and D (3, 5). Show
whether it is a trapezoid, parallelogram, rhombus, rectangle or square.
Note:
Quadrilaterals
1
2
Trapezoid
3
4
Parallelograms
5
6
Rectangle
Square
Quadrilateral: 4 sides # 1, 2, 3, 4, 5, 6
Trapezoid: at least one set of // sides # 2, 3, 4, 5, 6
Parallelogram: two sets of parallel sides # 3, 4, 5, 6
Rhombus: two sets of parallel sides and all sides equal length 4, 6
Rectangle: two sets of parallel sides, 90 corners 5, 6
Square: two sets of parallel sides, all sides equal, 90 corners 6
4. Are these lines parallel, perpendicular or neither?
a. 2y = 5x 3
10x 4y = 7
b. 3x 4y 9 = 0
3y + 4x = 6
5. a. DE FG . D = (2, 3), E = (1, 4), F = (3, 1) and G = (m, 5). Find m.
b. Find m if . DE FG
28
Math 10 C (AP)
Answers
1. a. 4x + 3y 23 = 0
or
b. 8x + 5y + 7 = 0
or
c. x 2 = 0
or
d. 2x + 5y + 14 = 0
or
e. 7x 3y 6 = 0
or
f. y + 6 = 0
3. square
5. a. m = 11
or
4
23
x
3
3
8
7
y
x
5
5
x=2
2
14
y
x
5
5
7
y x2
3
y = –6
y
a
2.
b
4. a. parallel
b. m =
c
39
7
Test Preparation:
Read Pages 386 and 387
Page 388 to 390 ~ Complete a few questions from each section.
Practice Test on Page 391 ~ complete all questions
b. perpendicular
© Copyright 2025