Lesson 13
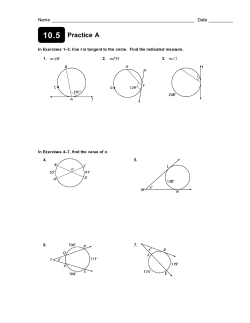
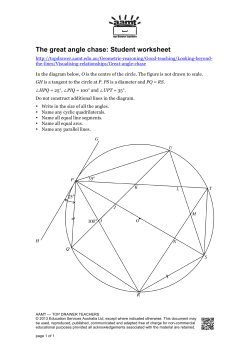
M5
GEOMETRY
Lesson 13: The Inscribed Angle Alternate—A Tangent Angle
Classwork
Opening Exercise
In circle ,
= 56°, and
is a diameter. Find the listed measure, and explain your answer.
a.
b.
c.
d.
e.
f.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.88
Lesson 13
M5
GEOMETRY
= 56°? Explain.
g.
Is the
h.
How do you think we could determine the measure of
?
Exploratory Challenge
Diagram 1
Diagram 2
Examine the diagrams shown. Develop a conjecture about the relationship between and .
Test your conjecture by using a protractor to measure
and .
Diagram 1
Diagram 2
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.89
Lesson 13
M5
GEOMETRY
Do your measurements confirm the relationship you found in your homework?
If needed, revise your conjecture about the relationship between
and :
Now, test your conjecture further using the circle below.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.90
Lesson 13
M5
GEOMETRY
Now, we will prove your conjecture, which is stated below as a theorem.
THE TANGENT-SECANT THEOREM: Let be a point on a circle, let
be a tangent ray to the circle, and let be a point on the
circle such that
is a secant to the circle. If =
and is the angle measure of the arc intercepted by
,
then
=
1
2
.
Given circle with tangent , prove what we have just discovered using
what you know about the properties of a circle and tangent and secant
lines.
a.
Draw triangle
. What is the measure of
b.
What is the measure of
c.
Express the measure of the remaining two angles of triangle
d.
What is the measure of
e.
Explain to your neighbor what we have just proven.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
? Explain.
? Explain.
in terms of
and explain.
in terms of ? Show how you got the answer.
The Inscribed Angle Alternate—A Tangent Angle
S.91
Lesson 13
M5
GEOMETRY
Exercises
Find , , , , and/or .
1.
2.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.92
Lesson 13
M5
GEOMETRY
3.
4.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.93
Lesson 13
M5
GEOMETRY
5.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.94
Lesson 13
M5
GEOMETRY
Lesson Summary
THEOREMS:
CONJECTURE: Let
circle such that
be a point on a circle, let
be a tangent ray to the circle, and let be a point on the
is a secant to the circle. If =
and b is the angle measure of the arc
intercepted by
, then
1
=2 .
THE TANGENT-SECANT THEOREM: Let be a point on a circle, let
a point on the circle such that
is a secant to the circle. If
the arc intercepted by
, then
be a tangent ray to the circle, and let be
=
and is the angle measure of
1
=2 .
Suppose
is a chord of circle , and
is a tangent segment to the circle at point . If
from , then
=
other than or in the arc of on the opposite side of
is any point
.
Problem Set
In Problems 1–9, solve for , , and/or .
1.
2.
3.
4.
5.
6.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
The Inscribed Angle Alternate—A Tangent Angle
S.95
Lesson 13
M5
GEOMETRY
7.
8.
10.
9.
is tangent to circle .
is a diameter. Find the angle measures.
is tangent to circle .
is a diameter. Prove: (i)
a.
b.
c.
d.
e.
11.
Lesson 13:
© 2014 Common Core, Inc. All rights reserved. commoncore.org
=
and (ii)
= .
The Inscribed Angle Alternate—A Tangent Angle
S.96