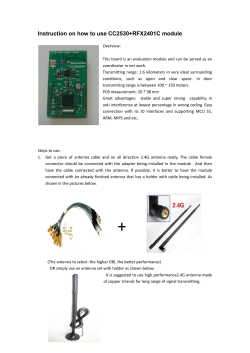
Document
ON THE STUDY OF HIGH GAIN AND STEERABLE MILLIMETRE-WAVE PLASMONIC ANTENNA A dissertation submitted to The University of Manchester for the degree of Master of Science in the Faculty of Engineering and Physical Sciences 2013 SHAKER M.M ALKARAKI SCHOOL OF ELECTRICAL AND ELECTRONIC ENGINEERING Table of Contents LIST OF FIGURES .............................................................................................................................. iv LIST OF TABLES ................................................................................................................................ ix ABSTRACT......................................................................................................................................... x DECLARATION ................................................................................................................................. xi INTELLECTUAL PROPERTY STATEMENT.......................................................................................... xii DEDICATION ...................................................................................................................................xiii ACKNOWLEDGMENT ......................................................................................................................xiv CHAPTER 1 ....................................................................................................................................... 1 INTRODUCTION ................................................................................................................................ 1 1.1 Background ............................................................................................................................ 1 1.2 Aims and objectives ............................................................................................................... 2 1.3 Instrumentation and methodology........................................................................................ 3 1.4 Context ................................................................................................................................... 4 CHAPTER 2 ....................................................................................................................................... 5 LITERATURE REVIEW ........................................................................................................................ 5 2.1 Basics of surface plasmons .................................................................................................... 5 2.2 Existing studies on plasmonic antennas ................................................................................ 7 2.3 Plasmonic antenna beam steering....................................................................................... 10 2.4 Theory of waveguides .......................................................................................................... 12 2.5 Antenna parameters ............................................................................................................ 13 CHAPTER 3 ..................................................................................................................................... 15 MILLIMETRE WAVE PLASMONIC ANTENNA SIMULATION AND ANALYSIS .................................... 15 3.1 Single element patch antenna design .................................................................................. 15 3.2 Millimetre-wave plasmonic antenna design and analysis ................................................... 17 3.3 60 GHz band plasmonic antenna design .............................................................................. 20 3.31 Single element patch antenna design ............................................................................ 20 ............................................................................................................................................... 21 3.32 60 GHz band millimetre-wave plasmonic antenna ........................................................ 21 CHAPTER 4 ..................................................................................................................................... 24 MILLIMETRE WAVE STEERABLE PLASMONIC ................................................................................. 24 ANTENNA DESIGN .......................................................................................................................... 24 4.1 Steer the antenna’s beam by introducing two cross slots on both slit sides ...................... 24 4.2 Steer the antenna’s beam by introducing two rectangular slots ........................................ 26 4.3 Steer the antenna’s beam by introducing three elliptical slots ........................................... 32 ii CHAPTER 5 ..................................................................................................................................... 33 DIRECTLY COUPLED AND STEERABLE MILLIMETRE WAVE PLASMONIC ANTENNA ....................... 33 5.1 Directly coupled and steerable slit grating millimetre-wave plasmonic antenna design .... 33 5.1.1 Directly coupled slit grating millimetre-wave plasmonic antenna design .................... 33 5.1.2 Steer the antenna’s beam by introducing two identical rectangular slots ................... 36 5.2 Bull’s eye millimetre-wave plasmonic antenna with rectangular slit .................................. 39 5.4 Bull’s eye millimetre-wave plasmonic antenna with circular slit ........................................ 41 CHAPTER 6 ..................................................................................................................................... 45 CONCLUSIONS AND FUTURE WORK .............................................................................................. 45 6.1 Conclusions .......................................................................................................................... 45 6.2 Recommendations for future work ..................................................................................... 46 REFERENCES ................................................................................................................................... 47 APPENDIX A .................................................................................................................................... 49 CLAIM OF POSSIBLE PUBLICATION................................................................................................. 49 APPENDIX B .................................................................................................................................... 50 RECTANGULAR PATCH ANTENNA DESIGN ..................................................................................... 50 APPENDIX C .................................................................................................................................... 51 FEASIBILITY STUDY REPORT ........................................................................................................... 51 (Word Count for Thesis‟ Body/Total for thesis: 10977/17092) iii LIST OF FIGURES Figure 1.1: Highly directive plasmonic antenna designed by structuring the metal surface. 2 Figure 2.1: Spoof SPs between metal and dielectric material at metal interface [8]. 5 Figure 2.2: (a) A block of PEC which is periodically cut through by one dimensional slit. (b) Periodic rectangular holes drilled in the PEC, where the length of the hole is, is the width and is the period [10]. 6 Figure 2.3: Plasmonic antenna structures composed of subwavelength apertures. (a) Bull‟s eye structure (b) slit grating structure[11]. 7 Figure 2.4: (a) 1 D Periodic corrugated Structure, (b) Spoof plasmon structure with subwavelength minor grooves in the surface. is the major groove‟s period, is the major groove‟s width and is the major groove‟s depth [6]. 8 Figure 2.5: Aperture of the single slit antenna bounded by two grooves. The EM wave is incident as the rectangular waveguide mode[14]. 8 Figure 2.6: Model of the proposed antenna integrated with the corrugated grooves. (a) Top view. (b) Front view.[15] 9 Figure 2.7: Geometry of the corrugated directive slot antenna, (a) Top view; (b) Side view. P=11.8mm, P1=9.1mm, w=2.4mm, d=2.7mm, t=1.5mm [16]. 9 Figure 2.8: (a) top view of theBull‟s Eye Antenna (b) cross section of the antenna with parameters =12mm , =11.54mm, =17mm, =10mm and =3.4mm[7]. Figure 2.9: Configuration of the slit groove structure [17]. 10 10 Figure 2.10: Electric field radiation from an optical nano-reflector array[17]. (a) and (b) The beam when grooves have different depth. (c) The beam when the grooves filled by different dielectric [17]. 11 Figure2.11: Non-Symmetreical Steerable Plasmonic Antenna[5]. 11 Figure 2.12: Polar coordinate representation of far-field gain radiation pattern for non symmetrical structure[5]. 11 Figure 2.13: Common waveguide structures. (a) Rectangular waveguide. (b) Circular waveguide.[18] 12 iv Figure 3.1: Model of The rectangular patch antenna. 15 Figure 3.2: Simulated 16 parameter of the proposed patch antenna. Figure 3.3: Simulated 3D far-field radiation pattern of the patch antenna. 16 Figure 3.4: Simulated 2D polar coordinate of the far-field of the patch antenna. 16 Figure 3.5: Configuration of the millimetre-wave plasmonic antenna that resonates at 12 GHz. 17 Figure 3.6: Front view of the designed plasmonic antenna. 17 Figure 3.7: Simulated 18 parameter of the plasmonic antenna. Figure 3.8: Simulated 3D far-fieled radiation pattern of the proposed antenna. 18 Figure 3.9: Simulated 2D polar coordinate of the far-field of the plasmonic antenna. 18 Figure 3.10: Simulated 3D far-fieled radiation pattern of the proposed antenna without grooves.19 Figure 3.11: simulated 3D radiation pattern of the proposed antenna with upper grooves only. 19 Figure 3.12: The relationship between the grooves number and the antenna gain. 20 Figure 3.13: Simulated far-field pattern of the 60GHz band patch antenna. (a) 2D polar coordinate. (b) 3D far-field radiation pattern. 21 Figure 3.14: Simulated 22 parameter of the 60 GHz band plasmonic antenna. Figure 3.15: Simulated far-field radiation pattern of the 60GHz band plasmonic antenna. (a) 3D far-field radiation pattern. (b) 2D polar coordinate. Figure 3.16: E-field polarization along the z-axis. 22 22 Figure 3.16: H-field polarization along the z-axis.23 Figure3.17: Distribution of the surface current on the proposed plamonic antenna. 23 Figure 4.1: The configuration of the proposed antenna, C = 3mm , D = 3mm, E = 0.8mm, Cross1 = 7.7mm , Cross2 = 5.3mm. 24 Figure 4.2: Simulated 3D far-field radiation pattern of the proposed steerable plasmonic antenna. 25 Figure 4.3: Simulated 2D polar coordinate of the far-field of the proposed steerable plasmonic antenna. 25 v Figure 4.4: Parametric study for the effect of the position of the 1 st cross on the proposed antenna‟s beam steer-ability. 26 Figure 4.5: Parametric study for the effect of the position of the 2 nd cross on the proposed antenna‟s beam steer-ability. 26 Figure 4.6: The configuration of the proposed antenna, SW1= 40mm, X1=1.9mm , X2= 4mm, E =1mm . 27 Figure 4.7: Simulated 3D far-field radiation pattern of the steerable plasmonic antenna with the two rectangular slots. 27 Figure 4.8: The effect of the position of first rectangualr slot on the antenna‟s resonant frequency. 28 Figure 4.9: The effect of the position of the 2nd rectangualr slot on the antenna‟s resonant frequency. 28 Figure 4.10: Simulated 2D far-field polar coordinate of the proposed antenna radiation pattern. (a) At resonance frequency (b) At 62.5GHz. 28 Figure 4.11: The effect of the position of 1st rectangualr slot on the antenna‟s radiation pattern. 29 Figure 4.12: The effect of the position of 2nd rectangualr slot on the antenna‟s radiation pattern. 29 Figure 4.13: The effect of the width of the two rectangular slot on the antenna radiation pattern.29 Figure 4.14: the proposed antenna‟s simulated gain performance over the operation frequency. 30 Figure 4.15: E-field polarization on the proposed antenna surface. (a) Symmetrical structure with no rectangular slots. (b) Symmetrical structure with presence of two rectangular slots. 30 Figure 4.16: H-field polarization on the proposed antenna with presence of two rectangular slots slots. 31 Figure 4.17: Distribution of the surface current on the proposed antenna. (a) without slots (b)with slots. 31 Figure 4.18: The configuration of the proposed antenna. 32 Figure 4.19: Simulated far-field radiation pattern of the proposed antenna. (a) 3D far-field radiation pattern. (b) 2D polar coordinate. 32 vi Figure 5.1: The slit grating antenna geometry. (a) Front view (b) Top view. 33 Figure 5.2: Simulated 34 parameter of the proposed slit grating plasmonic antenna. Figure 5.3: The simulated far-field radiation pattern of the proposed antenna. (a) 2D polar coordinates. (b) 3D radiation pattern. 34 Figure 5.4: The slit grating antenna‟s simulated gain over the operation bandwidth. 35 Figure 5.5: The geometry of the proposed antenna, X1 = 1.5mm, X2=2.9mm, E=0.55mm, S=40mm,SW=2.35mm,SL=0.2mm. 36 Figure 5.6: Simulated 36 parameter of the proposed antenna. Figure 5.7: The simulated far-field radiation pattern of the proposed antenna design at resonance frequency. (a) 3D radiation pattern. (b) 3D radiation pattern. (c) 2D polar coordinates. 37 Figure 5.8: The steerable slit grating antenna‟s simulated gain over the operation bandwidth. 37 Figure 5.9: The proposed antenna surface current density at resonance frequency. 38 Figure 5.10: The geometry of the proposed antenna C = 6mm, SL =0.2mm, SW= 2.37mm. 38 Figure 5.11: Simulated 2D polar coordinates of the proposed antenna‟s radiation pattern. (a) C = 6mm, (b) C = -6mm. 39 Figure 5.12: The effect of the position of the slit on the antenna‟s far-field radiation pattern. 39 Figure 5.13: Bull Eye millimetre wave antenna. (a) Front view (b) Top view. 40 Figure 5.14: Simulated parameter of the proposed Bull‟s eye antenna. 40 Figure 5.15: The simulated far-field pattern of the Bull Eye antenna at resonance frequency. (a) 3D radiation pattern. (b) 2D polar coordinates. 41 Figure 5.16: The Bull Eye antenna gain performance over the entire operation bandwidth. 42 Figure 5.17: Top of View of the proposed antenna C = 2mm. 42 Figure 5.18: The simulated far-field radiation pattern of Bull‟s eye antenna with unsymmetrical slit position at resonance frequency. (a) 3D radiation pattern. (b) 2D polar coordinates. 42 Figure 5.19: Top view of the proposed Bull‟s eye antenna with circular slit. 43 Figure 5.20: Simulated parameter of the proposed plasmonic antenna between 57GHz and 300GHz. 43 Figure 5.21: The proposed antenna‟s gain performance between 60GHz and 70GHz. 44 vii Figure 5.22: The proposed antenna‟s gain performance between 57 GHz and 300GHz. Figure 5.23: Simulated 44 parameter of the proposed Bull‟s eye antenna between 57GHz and 300GHz. 44 Figure 5.24: The far-field radiation pattern of the Bull‟s eye millimetre-wave plasmonic antenna with circular slit at some selected frequencies.(a) 3D at 90 GHz. (b) 2D at 90 GHz. (c) 3D at 200 GHz. (d) 2D at 200 GHz. (e) 3D at 300 GHz. (f) 2D at 300 GHz. 45 Figure 5.25: The simulated 2D polar coordinates of the far-field radiation pattern of the steerable Bull‟s eye antenna at 64GHz. 46 viii LIST OF TABLES Table 2.1: Values of for TE modes of a circular waveguide [19] 13 Table 3.1: Description of rectangular patch antenna parameters 15 Table 3.2: Description of the plasmonic antenna parameters 17 Table 3.3: description of rectangular patch antenna parameters 20 Table 3.4: Description of the 60 GHz plasmonic antenna parameters 21 Table 4.1: The proposed antenna‟s performance with and without the crosses 25 Table 5.1: The slit grating antenna parameters and specifications 34 Table 5.2: The proposed antenna‟s performance with different feeding methods 35 Table 5.3: The Bull‟s eye plasmonic antenna parameters and specifications 40 Table 5.4: The proposed antenna parameters and specifications 43 Table 5.5: Comparison between the slit grating and Bull‟s eye millimetre-wave plasmonic antennas 45 ix ABSTRACT This research presents an investigation about the design considerations and the beam steer-ability of millimetre-wave plasmonic antennas by modelling and designing steerable slit grating millimetre-wave plasmonic antenna and steerable Bull‟s eye millimetre-wave plasmonic antenna. Millimetre-wave plasmonic antennas are designed by structuring the metal surface with certain patterns that enables the electromagnetic waves to be confined into dimensions smaller than the wavelength and that boost the antenna directivity and gain. Recently, plasmonic antennas have shown promising characteristics that make them an impressive future alternative of phased array antennas for millimetre-wave applications. Plasmonic antennas have the capability to produce directive and steerable beam as efficient as array antennas with lower cost and less complex design. Novel techniques have been implemented to steer the millimetre-wave plamonic antennas beam; meanwhile, these new powerful techniques were able to steer the proposed antennas beam for up to 20˚. Surprisingly, one of the proposed techniques was capable not only to steer the antenna beam, but also to enhance the antenna gain at the same time. Finally, a novel Bull‟s eye millimetre-wave plasmonic antenna has an impedance bandwidth of more than 350% is designed and simulated. The suggested Bull‟s eye antenna bandwidth ranges from 57GHz to more than 300GHz. x DECLARATION I hereby declare that: No portion of the work referred to in the dissertation has been submitted in support of an application for another degree or qualification of this or any other university or other institute of learning. xi INTELLECTUAL PROPERTY STATEMENT I. The author of this dissertation (including any appendices and/or schedules to this dissertation) owns certain copyright or related rights in it (the “Copyright”) and s/he has given The University of Manchester certain rights to use such Copyright, including for administrative purposes. II. Copies of this dissertation, either in full or in extracts and whether in hard or electronic copy, may be made only in accordance with the Copyright, Designs and Patents Act 1988 (as amended) and regulations issued under it or, where appropriate, in accordance with licensing agreements which the University has entered into. This page must form part of any such copies made. III. The ownership of certain Copyright, patents, designs, trade marks and other intellectual property (the “Intellectual Property”) and any reproductions of copyright works in the dissertation, for example graphs and tables (“Reproductions”), which may be described in this dissertation, may not be owned by the author and may be owned by third parties. Such Intellectual Property and Reproductions cannot and must not be made available for use without the prior written permission of the owner(s) of the relevant Intellectual Property and/or Reproductions. IV. Further information on the conditions under which disclosure, publication and commercialisation of this dissertation, the Copyright and any Intellectual Property and/or Reproductions described in it may take place is available in the University IP Policy (see http://documents.manchester.ac.uk/display.aspx?DocID=487), in any relevant Dissertation restriction declarations deposited in the University Library, The University Library‟s regulations (see http://www.manchester.ac.uk/library/aboutus/regulations) and in The University‟s Guidance for the Presentation of Dissertations. xii DEDICATION This work is dedicated to my beloved parents Majdi and Manal Alkaraki. xiii ACKNOWLEDGMENT I would like to express my great gratitude to my supervisor Dr. Zhirun Hu for giving me this opportunity to work on this research under his supervision. I would like to thank him for the endless guidance and support that he has provided to me. I am so thankful for his great attention and help that he has given to tackle all the problems that I faced during my work. I would like to deeply thank Hani Qaddumi Scholarship Foundation (HQSF) for funding my Master‟s study at the University of Manchester, specially Miss. Rana Diab , Mrs. Afifah Kittaneh and Mr. Bassam Shakhshir. Finally, I would also like to thank my parents and members of my family for their prayers. xiv CHAPTER 1 INTRODUCTION 1.1 Background Data are transmitted in the wireless communication systems via electromagnetic waves. The coupling between electronic circuit and electromagnetic waves is achieved by antennas which are the sole interface between the hardware and radio frequency transmission environment. Antenna design proved to have a major impact over the wireless system reliability and cost. Hence, the efficiency of the wireless system will be enhanced by better antenna design that is capable of producing highly directive beam. As wireless technology industry has developed, millimetre and microwave applications have rapidly grown to satisfy the need of this industry. Millimetre wave directive antennas are widely used in in ad hoc network to save energy and to boost the system‟s capacity. The use of the aforementioned antennas will improve the network throughput and will cut the energy dissipation as well [1]. Directive antennas are able to increase the link capacity, enhance the security through decreasing accessibility and boost the bandwidth efficiency in both military and civil mobile networks [2]. The design of millimetre steerable directive antenna is a crucial requirement in the design of radar and imaging systems and such antennas that have high gain and steerable beam capabilities are essential. Particularly, it is vital that the antenna beam is steerable without being physically rotating the antenna itself for many applications. Recently, steerable and directive antennas have been widely employed in biomedical applications such as: cancer detection, because of their high penetration and resolution characteristics [3]. The well-known optical antenna design concepts and procedures can be investigated to be used for the design of millimetre-wave antennas. The main target of any antenna whether operating on radio frequencies (RF) or on optical frequencies is to radiate and receive electromagnetic energy [4]. Plasmonic antennas have been proposed for optical applications and it exploits the surface wave plasmonic phenomenon to produce highly directive radiation beam. Despite the fact that phased array antennas have proven their mettle and capability to provide adaptive beam steering with high gain, the design complexity and high cost of those antennas are its main drawbacks. Hence, the promising characteristics of plasmonic antennas make them an impressive future alternative of phased array antennas for millimetre-wave applications. 1 Plasmonic antennas have the capability to produce directive beam as efficient as array antennas with lower cost and less complex design, but the beam steer-ability of plasmonic antennas remains one of the main challenges in the field of antenna designs. Surface Plasmons (SPs) enable the electromagnetic field to be confined in dimensions that are smaller than the wavelength. SPs have received a great attention from scientists and engineers to design highly directive antennas to operate on optical and microwave frequencies. Generally, plasmonic antennas are designed by structuring the metal surface in certain patterns that boost the antenna directivity and gain as shown in Figure 1.1. Figure 1.1: Highly directive plasmonic antenna designed by structuring the metal surface. 1.2 Aims and objectives The aim of this project is to study and investigate high gain and steer-ability of millimeterwave antennas by controlling and directing radiation at metal surfaces through spoof surface plasmons phenomenon. The main objectives of the project are outlined as follows: To understand the basic concept of „spoof‟ surface plasmon and plasmonic antennas. To design and simulate Bull‟s Eye or other type of plasmonic millimetre-wave antennas. To perform parametric simulation to analyze the performance of beam steering capability of the plasmonic antennas. To investigate the coupling mechanism between the launching antenna and plasmonic one. To investigate the possibility of direct coupling from the circuit or waveguide to the plasmonic antenna. 2 1.3 Instrumentation and methodology The analysis and thorough study of existing research related to plasmonic antenna design to produce directive beam provides basic antenna structure that will be modelled and will be used as a frame work for this research The software package „CST Microwave Studio (CSTMWS)‟ is used to evaluate and test the performance of the suggested antenna. CST is powerful software that enables antenna engineers to simulate antenna performance and various antenna parameters such as: the input reflection coefficient ( ), directivity, gain, 2D and 3D radiation patterns. The parametric sweep facility provided by CST is used to test the effect of different antenna parameters on the proposed design for different geometrical structures. The sequence of simulation and design is as follows: i. An existing and preliminarily design of spoof plasmonic antenna based on existing structures from [5], [6] and [7] will be modelled and simulated using CST to resonate at 12GHz. ii. The initial antenna design which is based on slit grating structure plasmonic fed by patch antenna is optimized by utilizing sweep parameter provided by CST. Optimization will be carried out through extensive parametric study for antenna parameters such as: a. Metal thickness of the plasmonic antenna. b. Distance between the coupling patch and the plasmonic antenna. c. Depth and width of each groove. d. Groove‟s number and period. e. Shape of the grooves. f. iii. Slit dimensions, position and slit shape. The optimized and final antenna design that resonates at 12 GHz will be used as a reference to design millimetre wave plasmonic antenna which operates on the 60 GHz band. iv. Steer the beam of the designed 60GHz slit grating plasmonic antenna by introducing empirical slots on the antenna‟s aperture. v. Designing directly coupled slit grating plasmonic antenna where the coupling is achieved by rectangular waveguide. vi. Steer the beam of the directly coupled antenna by introducing empirical slots on the antenna‟s aperture and by changing the position of the slit. vii. Designing Bull‟s eye millimetre-wave plasmonic antenna with rectangular slit that operate on the 60 GHz band. viii. ix. Steer the beam of the Bull‟s eye antenna by changing the position of the slit. Re-designing the Bull‟s eye antenna with circular slit instead of the rectangular one and steer the beam of the antenna by changing the circular slit position. 3 1.4 Context The report comprises of six chapters as follows: Chapter 1 is an introduction to the project including a relevant background, aims and objectives, methodology, instrumentation and report organization. Chapter 2 contains explores the literature review of the concepts behind plasmonic antenna and fundamentals of surface plasmon phenomenon. Followed by a comprehensive conducted survey about the existing techniques related to the design of directive and steerable millimetre wave antenna is conducted. Chapter 3 presents the designs of two millimetre wave plasmonic antennas based on slit grating structure. The first antenna design resonates on 12 GHz, while the second resonates on the 60 GHz band. Both plasmonic antennas are fed by two rectangular patch antennas. Comprehensive analysis on the radiation mechanism of the proposed antennas is carried out too. Chapter 4 presents novel techniques that are used to steer the beam of the 60 GHz band slit grating plasmonic antenna that was previously designed in chapter 3. Chapter 5 demonstrates the design of directly coupled and steerable plasmonic antennas. Two different antenna structures are presented in this chapter which are slit grating and Bull Eye. Both antennas operate on 60 GHz band and the electromagnetic energy is coupled to both of them using rectangular and circular waveguides. Finally, chapter 6 offers the project conclusion about the design of steerable millimetre wave antenna and shed lights on future work on the study. 4 CHAPTER 2 LITERATURE REVIEW 2.1 Basics of surface plasmons Metals such as silver and copper support spoof surface plasmons (SPs) which are electromagnetic surface waves that propagate between the surface of metal and dielectric as shown in Figure 2.1 .The waves are localized near the surface and they originate from the free electrons of the metal. Surface plasmons have shown potential applications recently in subwavelength optics, biophotonics, microscopy, optical antennas and biological sensors [8] [9]. Figure 2.1: Spoof SPs between metal and dielectric material at metal interface [8]. Usually electromagnetic waves cannot propagate into dimensions that are smaller than the wavelength due to diffraction limit. The localization of incident waves to the metal surface can be controlled by introducing corrugated structures that enable the incident waves to be guided and confined into the slit that has smaller dimensions than the incident wavelength, due to surface the plasmons phenomenon [5]. Zirchao and Ruan have proposed a surface plasmon-like wave guide, at which half of the maximum electric field intensity of the surface plasmon mode wave is confined in a 0.20 0.10 sub-wavelength waveguide, is the wavelength in vacuum. The proposed wave guide is presented in Figure 2.2 [10]. 5 Figure 2.2: (a) A block of PEC which is periodically cut through by one dimensional slit. (b) Periodic rectangular holes drilled in the PEC, where the length of the hole, is the width and is the period [10]. As illustrated in Figure 2.2(b), periodic rectangular holes are drilled in the PEC metal surface and considering the case λ .The guided electric field inside the holes is described in equation 1 and it is zero inside the PEC. [ ] (2.1) Where, x, y, z are the Cartesian coordinates, frequency. √ , is a constant, is the is the wave vector along the z direction and given by [3]: √ Where, and ⁄ (2.2) are the permeability and the permittivity of any material that filling the holes. The periodic rectangular hole acts as a square waveguide with a cut off frequency equals to the surface plasma frequency as shown in equation [5]: (2.3) √ It has been demonstrated that periodic corrugations in metal surface will increase the momentum associated with surface plasmon and will enhance the directivity of the radiated beam due to surface plasmon constructive interference. Surface plasmons enable antenna designer to focus incident beam into an open structure to produce directive beam. So, the ability of surface plasmon to generate higher wave beam than the incident wave is exploited in high gain antenna design. 6 2.2 Existing studies on plasmonic antennas One of the interesting SPs applications is the plasmonic antennas which have great capability of extreme light and electromagnetic beam concentration. Among these antennas, structures that consist of sub-wavelength apertures flanked by surface corrugations which are called as two dimensional slit grating structure and three dimensional Bull‟s eye structure as shown in Figure 2.3[11]. Figure 2.3: Plasmonic antenna structures composed of sub-wavelength apertures. (a) Bull’s eye structure (b) Slit grating structure [11]. The above suggested corrugated structures have a great capability to improve the transmission of electromagnetic waves at microwave and optical frequencies due to constructive interference of resonating spoof plasmonic modes. Besides, these modes can be manipulated by changing the structure parameters such as period and depth of the grooves. The grooves have the ability to redirect incident waves to the sub-wavelength apertures through exciting surface wave in the form of surface plasmons. Then, these waves are refocused by the aperture and the transmission gets extremely enhanced [12]. This approach is well known in the design of optical and THz high gain antennas, but it has been recently exploited to design high gain millimetre microwave antennas. Several studies have been conducted on plasmonic antennas for optical and THz applications; meanwhile, few studies are available on plasmonic antennas for millimetre-wave applications [13]. A study by Haofei et al. has employed spoof surface plasmons phenomenon to design an antenna that operates from 8.2 to 12.5 GHz. Haofei has verified the role of spoof surface plasmon by introducing minor sub-wavelength grooves of period smaller than 1/10th of the operation wavelength. In the designed antenna shown in Figure 2.4, the feed source is converted into radiated wave via the corrugated structure and the antenna‟s far-field properties depends on the diffraction of surface waves [6]. The suggested antenna is based on the following dimensions, =30mm, =5mm , =4.5mm, =1.2mm and =2.38mm. 7 Figure 2.4: (a) 1 D periodic corrugated Structure, (b) Spoof plasmon structure with subwavelength minor grooves in the surface. is the major groove’s period, is the major groove’s width and is the major groove’s depth [6]. The given two expressions represent the far-field scattering properties of the given grating structure: (2.4) (2.5) Where, is the wave vector of the incident wave, wave vector of surface wave and in this case , is the diffraction angle, , and is the are the diffraction orders on each side. According to the authors[6], the radiated enegy is going to be concentrated and maximized when the condition is met, because the diffraction order from both sides will have the same divergence angle. M. Beruete et al have suggested a very low profile corrugated feeder antenna made of sub- wavelength aperture and bounded by two grooves as shown in Figure 2.5. The suggested antenna fed by standard waveguide and it resonates at 16 GHz [14]. Figure 2.5: Aperture of the single slit antenna bounded by two grooves. The EM wave is incident as the 𝑻𝑬𝟎𝟏 rectangular waveguide mode [14]. 8 The antenna gain has been enhanced by 4.3 dB after the grooves addition to reach 10.43 dB at resonance frequency. The groove resonates when its depth is and this explains the enhancement of the antenna gain. One study has proposed a sub-wavelength corrugated grooves antenna as shown in Figure 2.6. The proposed antenna is similar to the slit grating structure previously shown in Figure 2.3(b). The proposed antenna dimensions are: groove period p = 20mm, groove width w = 2.8mm, and groove depth d = 3.6mm. The antenna has four slits which is in size of 11.5mm 2 mm are fed by different four rectangular waveguides [15]. Figure 2.6: Model of the proposed antenna integrated with the corrugated grooves. (a) Top view. (b) Front view. [15] Introducing the corrugated structure has enhanced the antenna gain by about 11 dB and it has reduced the back-lobe level by 10dB. Another study has suggested a highly directive slot antenna with dielectric loaded surface .The proposed antenna has symmetrical periodic grating (grooves) on dual sides of the slot and the slot is directly fed by a waveguide as an exciting source. A dielectric layer with high dielectric constant ( =9.8) is loaded between neighbouring grating in order to reduce the antenna aperture. The proposed antenna is shown in Figure 2.7 and has a gain of 18.1dBi at frequency of 14.5GHz [16]. Figure 2.7: Geometry of the corrugated directive slot antenna, (a) Top view; (b) Side view. P=11.8mm, P1=9.1mm, w=2.4mm, d=2.7mm, t=1.5mm [16]. 9 Another study has proposed a millimetre Bull‟s eye corrugated antenna directly fed by a wave guide feeder. The proposed antenna is presented in Figure 2.8 and it has two resonant frequencies at 16GHz and 12GHz with a gain of 15dBi at 16GHz [7]. Figure 2.8: (a) top view of the Bull’s Eye Antenna (b) cross section of the antenna with parameters 𝒉=12mm, 𝒂=11.54mm, 𝒅=17mm, 𝒍=10mm and 𝒘=3.4mm[7]. The author reported that the slit‟s depth ,h=12mm, determines the longitudinal resonant frequency of the antenna at 16GHz, while the lower resonant frequency at 12GHz is determined by the slit‟s width. The width of every grating and the grating period control the angle where plasmonic like constructive interference takes place, hence both control the antenna‟s gain. Finally, the author demonstrated practically that the same non corrugated flat metallic structure has very low gain with nearly isotropic radiation pattern. 2.3 Plasmonic antenna beam steering Liu et al presented an ultralow profile nano antenna reflectors at which the optical radiation and emission is manipulated through the plasmonic interaction with light. In the proposed reflector design the beam radiation is steered by assigning different sets of groove‟s depths on sliver substrate that is shown in Figure 2.9[17]. Figure 2.9: Configuration of the slit groove structure [17]. 10 The reflector beam is steered to 10 ˚ and 30˚, when the grooves have different depths as shown in Figure 2.10(a) and Figure 2.11(b), however, Filling the grooves by dielectric materials that have different dielectric constant will steer the beam to 10˚ as shown in figure 2.10(c). Figure 2.10: Electric field radiation from an optical nano-reflector array[17]. (a) and (b) The beam when grooves have different depth. (c) The beam when the grooves filled by different dielectric[17]. Finally, the only study which could be found on the design of Millimetre-wave plasmonic antenna has shown that introducing non symmetrical corrugated structure as shown in Figure 2.11 will steer the plasmonic antenna beam by 5˚ as shown in Figure 2.12. The suggested antenna resonates at 9 GHz with linear gain of 14.1(11.5dB) [5]. Figure2.11: Non-Symmetreical Steerable Plasmonic Antenna[5] Figure 2.12: Polar coordinate representation of far-field gain radiation pattern for non-symmetrical structure[5]. In the proposed design, the electromagnetic radiation will be concentrated in the nonsymmetrical side of the antenna because of spoof surface plasmon‟s effect and will focus the radiated beam at that direction. 11 2.4 Theory of waveguides Waveguides are hollow and empty metals that used to transfer (guide) electromagnetic energy from one place to another. Both rectangular and circular waveguides cannot propagate TEM mode, but can propagate TM and TE modes, because of the presence of one conductor only [19]. (a) (b) Figure 2.13: Common waveguide structures. (a) Rectangular waveguide. (b) Circular waveguide.[18] Waveguide acts as a high pass filter with a cut off frequency below which propagation is impossible. The cut off frequency of the rectangular waveguide is given by [19]: √( Where, ) ( ) (2.6) is the velocity of light in vacuum. The waveguide dominant mode is the mode with the lowest cut off frequency lowest occurs for the mode for the rectangular wave guide with , the is given by [19]: (2.7) Thus the mode lowest is the dominant TE mode for rectangular waveguide, while the for the circular wave guide can be given as: (2.8) Where is given in Table 2.1: 12 Table 2.1: Values of for TE modes of a circular waveguide [19] n o 3.832 7.016 10.174 1 1.841 5.331 8.536 2 3.054 6.706 9.970 So the first propagating mode in the circular wave guide is the one that has smallest which is found in table 2.1 to be mode. Thus, waveguide. (In circular waveguide there is no since , is the dominant mode in the circular ). 2.5 Antenna parameters The performance of the designed antennas in this project is going to be analysed in terms of: 1) parameter is the input port voltage reflection coefficient and it is the ratio of the reflected wave to the incident wave. 2) Bandwidth In this research, antenna‟s bandwidth is defined as the range of frequencies that the designed antenna operates successfully according to a predetermined standard which is -10dB. 3) Radiation pattern The antenna radiation pattern is defined as ”the spatial distribution of a quantity that characterises the electromagnetic field generated by antenna” [20]. The antenna radiation pattern is determined in the far-field region and it can be two or three dimensional spatial distribution of power density, gain, directivity etc. 4) Beam width The beam width is the angle around zero point around the main beam. However, half power beam width (3dB) is:”In a radiation pattern cut containing the direction of the maximum of a lobe, the angle between the two directions in which the radiation intensity is one-half the maximum value” [20]. 13 5) Directivity Directivity is a parameter that describes the enhancement of the power density in the maximum radiation direction with respect to the radiation of an isotrope, at distance r in the field region. The directivity of an antenna is defined with respect to the radiated power when it compared to isotrope[21]. 6) Gain Antenna‟s gain is quite similar to the antenna‟s directivity; meanwhile, it is defined with respect to the input power delivered to the antenna. So unlike gain, directivity depends on the structure of the antenna, and does not depend on the efficiency of a given antenna. To find out the amount of power transferred from the antenna to the space, the antenna gain is used rather than the directivity [21]. The gain of the antenna is defined as: G=ηD (2.9) Where, η is the radiation efficiency of the antenna. Gain is always less than directivity because η lies in the range 0 < η < 1. 14 CHAPTER 3 MILLIMETRE WAVE PLASMONIC ANTENNA SIMULATION AND ANALYSIS In this chapter, two slit grating millimetre-wave plasmonic antennas operate at 12GHz and at 60GHz band are designed, optimized and simulated using CST MICROWAVE STUDIO® (CST MWS). CST is powerful software that enables antenna engineers to simulate antenna performance and various antenna‟s parameters such as: return loss ( ), directivity, gain and radiation patterns. Both designed plasmonic antennas are fed by normal rectangular microstrip patch antenna. Moreover, the design considerations and analysis of the proposed antennas are presented and analysed. 3.1 Single element patch antenna design Single element patch antenna is designed based on transmission line model. The patch‟s width has a minor effect on the resonant frequency and it has a major effect on the antenna‟s bandwidth and radiation pattern; meanwhile, the patch‟s length controls the resonant frequency [21].The resonant frequency of the patch antenna is chosen to be 12GHz .The model of the designed antenna is illustrated in Figure 3.1 and detailed antenna‟s parameters are shown in Table 3.1. Figure 3.1: Model of The rectangular patch antenna. Table 3.1: Description of rectangular patch antenna parameters Patch Length L = 4.77mm. Inset depth Patch width W = 5mm. Inset width = 1.5 mm. =1.5mm. Substrate thickness = 1.6 mm. Substrate dielectric constant = 5.2. Feed Line Width = 0.80 mm. Antenna Geometry = 10mm 15 8.5mm. The proposed patch antenna has been optimized using parametric study to resonate accurately at 12GHz. As shown in Figure 3.2 the patch‟s bandwidth is 430MHz and it has a simulated gain of 3.88 (5.89 dB) at 12GHz as presented in Figure 3.3 and Figure 3.4. Figure 3.2: Simulated 𝑺𝟏𝟏 parameter of the proposed patch antenna. Figure 3.3: Simulated 3D far-field radiation pattern of the patch antenna. Figure 3.4: Simulated 2D polar coordinate of the far-field of the patch antenna. 16 3.2 Millimetre-wave plasmonic antenna design and analysis The patch antenna is used to couple electromagnetic energy to the plasmonic antenna as illustrated in Figure 3.5. The geometrical dimensions of the designed antenna structure are elaborated in Table 3.2. The plasmonic antenna can be placed in the near field or in the far-field of the patch antenna[5].In the proposed design, the plasmonic antenna is placed in the near field of the patch antenna with a distance of 3.9 mm ( /6). The bottom structured surface of the plasmonic antenna collects the patch‟s output beam and guide it into the slit. Excitation of the surface plasmon by creating periodic grooves on the bottom and top of the plasmonic helps to confine the patch‟s output beam into the slit. Moreover, the grooves which are on the top of the plasmonic antenna help to produce highly directive beam as shown in Figure 3.8. Figure 3.5: Configuration of the millimetre-wave plasmonic antenna that resonates at 12 GHz. Figure 3.6: Front view of the designed plasmonic antenna. Table 3.2: Description of the plasmonic antenna parameters Dimensions of the whole Geometry W = 240 mm L Distance between patch and plasmonic antenna = 3.7 mm. 100 mm. Dimension of the slit = 2 mm Groove‟s depth Gd = 5 mm. 20 mm. Groove‟s period P = 22.6 mm. Distance between slit and 1st groove A = 10.4 mm. Groove‟s width GW = 5mm. Thickness of the plasmonic antenna T = 12 mm. 17 The designed plasmonic antenna resonates at 11.97 GHz with a bandwidth of 148 MHz and poor parameter, which is equal to -11.4 dB at resonance frequency as shown in Figure 3.7 .The proposed antenna is highly directive with a linear gain of 24.38 (13.9 dB) and with a 3dB angular beam‟s width of 36.4˚ at 11.97GHz as shown in Figure 3.8 and Figure 3.10. Figure 3.7: Simulated 𝑺𝟏𝟏 parameter of the plasmonic antenna. Figure 3.8: Simulated 3D far-fieled radiation pattern of the proposed antenna. Figure 3.9: Simulated 2D polar coordinate of the far-field of the plasmonic antenna. To prove that the high directivity of the antenna is because of the surface plasmon phenomenon, the antenna is simulated without introducing any grooves and one can see that the antenna has a linear gain of 3.049 (4.84 dB) as shown in Figure 3.10. This non-grating structure is fed with the same output beam from the patch antenna that was used to feed the corrugated structure antenna. 18 Figure 3.10: Simulated 3D far-fieled radiation pattern of the proposed antenna without grooves. Figure 3.10 proves that without introducing grooves on the metal‟s surface, the radiated electromagnetic energy from the patch is not well confined into the slit and the remaining confined energy through the slit is not re-directed to produce high directivity. Introducing grooves on the upper part of the antenna as shown in Figure 3.11 will not only enable the electromagnetic energy to be well confined into the slit, but it will also highly produce directive beam and it will rise the antenna gain to be equal to 22.81 (13.6 dB). The final antenna‟s gain has dropped only by 2 linear (0.35 dB), when the bottom grooves are removed as shown in Figure 3.11. Figure 3.11: simulated 3D radiation pattern of the proposed antenna with upper grooves only. Figure 3.12 illustrates the relationship between the antenna gain and number of grooves on each slit‟s side. It can be noticed that the antenna‟s gain increases exponentially as the number of grooves increases up to certain limit which can be called as groove‟s saturation limit. It can be seen that the saturation groove number is five on each antenna side and the antenna gain enhancement is weak beyond this limit. So, there is no need to adopt more grooves after reaching the groove‟s saturation limit to achieve higher gain, since it will increase the antenna physical size and it will extremely reduce the antenna aperture efficiency. 19 Figure 3.12: The relationship between the grooves number and the antenna gain. 3.3 60 GHz band plasmonic antenna design There is a great demand for better performing antennas that work on the free and less congested 60 GHz frequency band. Working on the free 60 GHz band will enable users to use broader bandwidth and higher data transmission rate. As we move up in frequency, many challenges arise for antenna designers, particularly, to achieve broad bandwidth with a reasonable antenna gain [22]. Plasmonic antenna has marvellous characteristics that gives it great capability of providing highly directive gain with a reasonable bandwidth, therefore the possibility of designing 60 GHz directive plasmonic antenna will be investigated in this section. 3.31 Single element patch antenna design Single element patch antenna is designed to resonate at the 60 GHz band using RT Duroid substrate that has a dielectric constant of 2.2. The patch resonates at 60.2 GHz with a bandwidth of 2.592 GHz and a gain of 3.893 (5.9dB) at resonance frequency. The patch‟s parameters are elaborated in table 3.3 and the patch‟s 2D radiation pattern and 3D radiation patterns are shown in Figure 3.13. Table 3.3: description of rectangular patch antenna parameters Patch Length L = 1.73mm. Inset depth Patch width W = 2.13mm. Inset width K = 2 mm. =1 mm. Substrate thickness = 1 mm. Substrate dielectric constant = 2.2. Feed Line Width Antenna Geometry W 20 = 10 µm. L = 2.4mm 2.5 mm. (b) (a) Figure 3.13: Simulated far-field pattern of the 60GHz band patch antenna. (a) 2D polar coordinate. (b) 3D far-field radiation pattern. 3.32 60 GHz band millimetre-wave plasmonic antenna Millimetre-wave plasmonic antenna has been designed and optimized according to the dimensions shown in Table 3.4. The proposed antenna has similar operational principles as the 12GHz antenna that was designed in section 3.2. The antenna is designed to operate at 60 GHz band and it resonates at 62.2 GHz with a bandwidth of 2.47GHz as shown in Figure 3.14. The antenna‟s parameters which described in Table 3.4 have been optimized and have been studied using parametric facility provided by CST to maximize the antenna‟s gain at resonant frequency. Table 3.4: Description of the 60 GHz plasmonic antenna parameters Dimension of the whole Geometry W = 20 mm Distance between patch and plasmonic antenna = L 0.84 mm. 41 mm. Dimension of the slit = 0.6 mm Groove‟s depth Gd = 0.95 mm. 2.65 mm. Groove‟s period P = 4.4 mm. Distance between slit and 1st groove A = 1 mm. Groove‟s width GW = 0.3mm. Thickness of the plasmonic antenna T = 1.2mm. The proposed plasmonic antenna has a simulated gain of 10.4dB (10.99 linear) with a 3dB angular beam‟s width of 31.8˚ at resonant frequency. The patch antenna is placed in the near field of the plasmonic antenna and the distance between the patch and the plasmonic is set to be less than ( /5). The plasmonic antenna parameter is shown in Figure 3.14, while the antenna‟s far-field radiation pattern is shown in Figure 3.15. 21 Figure 3.14: Simulated 𝑺𝟏𝟏 parameter of the 60 GHz band plasmonic antenna. (b) (a) Figure 3.15: Simulated far-field radiation pattern of the 60GHz band plasmonic antenna. (a) 3D far-field radiation pattern. (b) 2D polar coordinate. To understand and analyse the radiation mechanism of the designed antenna, the electric field and magnetic field distribution will be studied at the antenna‟s surface. The designed antenna resonate at 62.2 GHz, thus the operation wavelength λ is 4.82 mm ,while, the slit dimensions are 0.6 mm 2.65 mm which is smaller than λ . It has been found experimentally that the directivity of the antenna is maximized when the groove‟s period P ≈ λ (P=4.4mm). The antenna‟s electric field distribution which is presented in Figure 3.16 shows that the electromagnetic waves is re-emitted and re-directed by the grooves with help of plasmonic waves on both slit sides. Figure 3.16: E-field polarization along the z-axis. 22 When the groove period is closed to the operational wavelength λ, the electromagnetic and plasmonic waves will be re-directed in phase equals to P and constructive interference occurs to cause peak of transmission. It has been noticed experimentally that if the grooves period is much smaller or much bigger than the operational wavelength, the antenna‟s gain will drop exponentially, because of the destructive interference caused by each groove radiation. Moreover, in the proposed design the slit is considered the primary radiation source and the grooves are the other 10 secondary radiation sources. Hence, the energy radiation from the secondary sources is coherently super-positioned with the radiation from the primary source to produce highly directive beam in the zero direction as the antenna has symmetrical physical structure. The magnetic field distribution of the proposed antenna is shown in Figure 3.16 and the surface current density of the antenna is shown in Figure 3.17. It can be noticed that the electric field, magnetic field and surface current are concentrated mainly beside the slit‟s first and second groove side. Besides, the aforementioned fields and current distribution are less concentrated after the first two grooves as illustrated in Figure 3.15, Figure 3.16 and Figure 3.17. However, the antenna is so sensitive to any change of those parameters and that explain why the millimetrewave plasmonic antenna gain is extremely dependent on the 1st and 2nd groove‟s addition as previously shown in Figure 3.12. Figure 3.16: H-field polarization along the z-axis. Figure3.17: Distribution of the surface current on the proposed plamonic antenna. 23 CHAPTER 4 MILLIMETRE WAVE STEERABLE PLASMONIC ANTENNA DESIGN It has been shown that the beam of millimetre-wave plasmonic antenna can be controlled by introducing unsymmetrical corrugated structures on each slit side [1]. This technique has proven its capability to steer the beam of the millimetre-wave plasmonic antenna for a certain limit and it could steer the antenna beam up to 5° [1]. Novel techniques will be presented and implemented in this chapter and the following one to steer the beam of the millimetre -wave plasmonic antenna with a better performance than the aforementioned technique carried out in similar research. The patch antenna designed in section 3.31 is used to feed and couple the electromagnetic energy to the proposed plasmonic antennas presented in this chapter. Besides, all proposed antennas in this chapter operate at the 60GHz band. 4.1 Steer the antenna’s beam by introducing two cross slots on both slit sides Two slots in the shape of cross are introduced on each antenna side to make the antenna‟s beam steerable. Introducing the two crosses steers the antenna‟s beam for up to 10° on the whole antenna‟s bandwidth. The designed antenna resonates at 62.1 GHz and it has a bandwidth of 2.44 GHz with a realized gain of 9.95 dB at resonance frequency. The proposed antenna which is shown in Figure 4.1 has the same dimensions as the antenna presented in section 3.32. In addition, introducing the two crosses slightly changes the antenna‟s resonance frequency and parameter characteristics, while it effectively changes the antenna‟s far-field radiation. Figure 4.1: The configuration of the proposed antenna, C = 3mm , D = 3mm, E = 0.8mm, Cross1 = 7.7mm , Cross2 = 5.3mm. 24 The two crosses have identical dimensions which are: D=3mm , C=3mm , E=0.8mm, and the 1st cross is 7.7mm away from the slit centre while the second is 5.3 mm away from the slit centre. Comparison of the antenna characteristics pre and post adding the two crosses is shown in Table 4.1.The far-field radiation patterns of the proposed antenna are shown in Figure 4.2 and Figure 4.3. It can be seen that the proposed antenna‟s beam is steered by 10˚ as illustrated in Figure 4.3. Table 4.1: The proposed antenna’s performance with and without the crosses Type of the Resonant Bandwidth Simulated Simulated gain Minimum 3dB antenna frequency (GHz) gain at at resonance return Beam resonance (dB) (GHz) (linear) loss width dB Without crosses 62.2 2.47 11.1 10.45 dB -24.67 31.8° With crosses 62.1 2.44 9.89 9.95 dB -34 29° Figure 4.2: Simulated 3D far-field radiation pattern of the proposed steerable plasmonic antenna. Figure 4.3: Simulated 2D polar coordinate of the far-field of the proposed steerable plasmonic antenna. Figure 4.4 illustrates the effect of the position of the 1st cross on the steer ability of the proposed antenna. Figure 4.4 shows that introducing the 1st cross makes the antenna beam steerable by 10°. Introduction and position of the 2nd cross does not affect the beam steerability of the antenna but it reduces the magnitude of the side lobes as shown in figure 4.5. 25 Figure 4.4: Parametric study for the effect of the position of the 1st cross on the proposed antenna’s beam steer-ability. Figure 4.5: Parametric study for the effect of the position of the 2nd cross on the proposed antenna’s beam steer-ability. The two added crosses on each slit side will create unsymmetrical electric and magnetic field distribution along the two slit sides which will make the antenna beam steerable. Addition of crosses will concentrate the electric and magnetic field on one antenna‟s side as will be studied and explained in details at the end of the following section. 4.2 Steer the antenna’s beam by introducing two rectangular slots In the previous section it has been shown that the direction of the beam can be steered for up to 10° by introducing two cross slots on both slit‟s sides. In this section, it will be shown that the direction of the beam pattern can be steered for up to 15° by introducing two identical rectangular slots on any slit‟s side as shown in Figure 4.6. The antenna‟s beam is going to be steered to the opposite direction of the two slots as presented in Figure 4.7. The proposed antenna parameters have the same dimensions as the millimetre-wave plasmonic antenna that was previously designed in section 3.32. 26 Figure 4.6: The configuration of the proposed antenna, SW1= 40mm, X1=1.9mm , X2= 4mm, E =1mm . The proposed antenna resonates at 61.7 GHz with a bandwidth of 2.32 GHz ranging from 60.7 GHz to 63.1 GHz. Adding two rectangular slots does not only make antenna beam steerable, but it also triples the linear antenna gain to 28.2 which is 14.5 dB as shown in figure 4.7. Figure 4.7: Simulated 3D far-field radiation pattern of the steerable plasmonic antenna with the two rectangular slots. Adding the first slot will extremely affect the original antenna‟s resonance frequency, gain and direction of the main beam. After adding the first slot, the antenna‟s resonance frequency is shifted by 500MHz from 62.2 GHz to 61.7GHz and the antenna‟s beam is steered by 15°. The proposed antenna has a good return loss of -24.73 dB at resonance frequency as shown in Figure 4.9. The postion of the 1st rectangular slot affects the behaviour of the antenna as presented in figure 4.8. The resonance frequency is directly proportional to the distance between the slit centre and the postion of the 1st rectangular slot. Addition and position of the second rectangular slot slightly changes the antenna‟s resonance frequency by few MHz. Besides, it increases the antenna gain by 1.5 dB and it reduces the level of side lobes by more than 3dB as will be shown in the next page. 27 Figure 4.8: The effect of the position of 1st rectangualr slot on the antenna’s resonant frequency. Figure 4.9: The effect of the position of the 2nd rectangualr slot on the antenna’s resonant frequency. The proposed antenna has a steerable beam of 15° at the whole antenna‟a operation bandwidth . The proposed antenna‟s polar far-field radiation pattern at some selected frequencies are shown in the Figures 4.10. The simulated antenna 3dB beam width is 21˚ at the resonace frequency with as low as -10.5dB side lobes level. (a) (b) Figure 4.10: Simulated 2D far-field polar coordinate of the proposed antenna radiation pattern. (a) At resonant frequency (b) At 62.5GHz. 28 Simulation results show that the proposed antenna‟s linear gain equals to 21.7 after addition of the 1st rectangular slot. Besides, it is enhanced to 28.2 after the 2nd slot addition as presented in Figure 4.11 and Figure 4.12. Both Figure 4.12 and Figure 4.13 show that the side lobes level is effectively suppressed after addition of the 2nd rectangular slot. Moreover, it can be noticed that the addition of the 2nd rectangular slots did not affect the antenna beam‟s steer-ability. Figure 4.11: The effect of the position of 1st rectangualr slot on the antenna’s radiation pattern. Figure 4.12: The effect of the position of 2nd rectangualr slot on the antenna’s radiation pattern. The parametric study of the effect of the width of the rectangular slots on the designed antenna‟s radiation pattern is shown in Figure 4.13. One can see that the gain of the antenna and its beam steer-ability is directly proportional to the rectangular slots width. The antenna‟s farfield radiation pattern is simulated for different slots width SW1 of 10mm, 20mm, 30 mm and 40mm as shown in Figure 4.14. Figure 4.13: The effect of the width of the two rectangular slot on the antenna radiation pattern. 29 The 60 GHz band plasmonic antenna gain performance over its bandwidth after the addition of both rectangular slots is illustrated in Figure 4.14. Figure 4.14: the proposed antenna’s simulated gain performance over the operation frequency. The electric and magnetic field is uniformly distributed over the antenna‟s surface when there is no rectangular slots as shown in Figure 4.15 (a) and this symmetrical distribution will make the antenna‟s main beam radiated and concentrated in the zero direction. (a) (b) Figure 4.15: E-field polarization on the proposed antenna surface. (a) Symmetrical structure with no rectangular slots. (b) Symmetrical structure with presence of two rectangular slots. 30 Introducing the two rectangular slots will affect the beam steer-ability of the millimetrewave plasmonic antenna because of the asymmetry created in the electric and magnetic fields distribution on both slit‟s sides as shown in Figure 4.15 (b) and Figure 4.16. Creating slots on one slit side will make the electric and magnetic fields concentrated on the opposite side, hence steering the antenna‟s beam to that side. Figure 4.16: H-field polarization on the proposed antenna with presence of two rectangular slots. The proposed antenna‟s surface current is also has symmetrical distribution when the antenna has no slots as shown in Figure 4.17 (a). Asymmetrical distribution of the surface current will be created when the two rectangular slots are introduced as clearly shown in Figure 4.17 (b). In addition, the current density magnitude is increased on the non-slotted side of the antenna as shown in Figure 4.17(b) .The surface current density increment explains why the antenna‟s beam becomes steerable and particularly why the gain has dramatically increased by 4.5 dB after the addition of the two rectangular slots. (a) (b) Figure 4.17: Distribution of the surface current on the proposed antenna. (a) without slots (b)with slots. 31 4.3 Steer the antenna’s beam by introducing three elliptical slots Experimental results show that the millimetre wave plasmonic antenna‟s beam can be steered by using slots in the shape of ellipse as shown in figure 4.18. Three elliptical slots are used to steer the antenna‟s beam and to enhance the antenna‟s gain as well. The antenna‟s beam is steered by 20˚. This techinque is more powerful in terms of the beam steer-ability compared to introducing two rectangulr slots which was only capable to steer the beam up to 15˚. The enhancement of the beam steer-ability was at the cost of gain decrement at resonance frequency. Figure 4.18: The configuration of the proposed antenna. The ellipses dimensions are as follows: A =6.5 mm is the ellipse major axis, B = 0.6mm is the ellipse minor axis, C = 4.6 mm is the horizontal distance between the slit centre and 1st and 2nd ellipse, D = 5.1mm is the vertical distance between the slit‟s centre and the 3rd ellipse and E = 1.85mm is the vertical distance between the slit‟s centre and the 1st and 2nd ellipses. The antenna‟s linear gain at resonance frequency is 17.9 (12.53 dB) as shown in Figure 4.19. (a) (b) Figure 4.19: Simulated far-field radiation pattern of the proposed antenna. (a) 3D far-field radiation pattern. (b) 2D polar coordinate. The proposed antenna resonates at 61.54 GHz with a simulated bandwidth of 2.06GHz ranging from 60.66 GHz to 62.72 GHz. Adding the ellipses has shifted the resonant frequency from 62.1 GHz to 61.54 GHz. The effect of the three elliptical slots on the antenna steer-ability is simillar to the effect of the two rectangular slots introduced in the previous section. Introducing the lowest two ellipses will affect the antenna‟s resonant frequency and the beam steer-ability to 15˚. Besides, introducing the 3rd ellipse will increase the antenna‟s beam steer-ability to 20˚, gain and it will highly reduce the side lobes level gain. 32 CHAPTER 5 DIRECTLY COUPLED AND STEERABLE MILLIMETRE WAVE PLASMONIC ANTENNA Two different steerable and directly coupled plasmonic antenna structures will be presented in this chapter. The first antenna design is based on slit grating structure that has been discussed in the previous two chapters; meanwhile, the second design is based on Bull‟s eye structure. Both antenna structures are designed to work at the 60GHz band. Both suggested structures achieve much higher gain with an excellent steerable beam than the previously proposed antennas. The electromagnetic energy will be coupled to both plasmonic antennas by using rectangular and circular waveguides, instead of normal coupling that uses the patch antenna. The plasmonic antenna beam will be steered and manipulated in the proposed designs by using two different techniques. The first technique is to introduce slots on any slit‟s side to steer the beam to the opposite side, while the second technique is to change the slit position to manipulate the electric field and magnetic field distribution on the antenna‟s surface to steer the beam. 5.1 Directly coupled and steerable slit grating millimetre-wave plasmonic antenna design 5.1.1 Directly coupled slit grating millimetre-wave plasmonic antenna design The proposed slit grating antenna which is shown in Figure 5.1 has optimized dimensions quite similar to the plasmonic antenna fed by patch antenna that was shown in section 3.32. The antenna‟s dimensions are elaborated in Table 5.1. The proposed antenna resonates at 62 GHz with a bandwidth of 8.35 GHz. The electromagnetic energy is coupled to the plasmonic antenna by using rectangular waveguide which operates on the mode. Using a wave guide to couple the electromagnetic energy to the plasmonic enhances the antenna gain at resonance frequency by 4 dB to reach 14.7 at 62GHz. However, the same structure achieved only 10dB of gain when the coupling is achieved by using patch antenna. Figure 5.1: The slit grating antenna geometry. (a) Front view (b) Top view. 33 Table 5.1: The slit grating antenna parameters and specifications Dimension of the whole Geometry W 20 mm L= Wave guide dimensions a 41 mm. 1.3mm b y = 3.2mm 4.5 mm Groove‟s width GW = 0.3 mm. Groove‟s depth Gd = 0.95 mm. Groove‟s period P = 4.4 mm. Distance between slit and 1st groove A = 1.1 mm. Dimension of the slit SL Thickness of the Plasmonic antenna T = 1.2mm. SW = 0.6 2.35 mm. The proposed antenna operates on a range of frequencies between 60.83GHz and 69 GHz as shown in figure 5.2. In addition, the antenna has a linear gain of 29.4 (14.7dB) with a 3dB angular width of 32.1˚ at 62GHz as shown in Figure 5.3. The antenna‟s maximum gain is 15.4 dB at 61 GHz and the antenna‟s gain performance over the antenna‟s operation bandwidth is illustrated in Figure 5.4. Complete comparison between the antenna that was fed by patch that was designed in section 3.42 and the directly coupled antenna is elaborated in Table 5.2. Figure 5.2: Simulated 𝑺𝟏𝟏 parameter of the proposed slit grating plasmonic antenna. (a) (b) Figure 5.3: The simulated far-field radiation pattern of the proposed antenna. (a) 2D polar coordinates. (b) 3D radiation pattern. 34 Figure 5.4: The slit grating antenna’s simulated gain over the operation bandwidth. Table 5.2: The proposed antenna’s performance with different feeding methods Type of the feeding Resonant frequency (GHz) Bandwidth (GHz) Simulated gain at resonance (linear) Simulated gain at resonance (dB) Minimum return loss ( ) (dB) 3dB Beam width Side lobe level (dB) Fed by patch 62.2 2.47 11.1 10.45 -24.68 dB 31.8˚ -7.6 Fed by Waveguide 62.1 8.35 29.4 14.7 -28.45 32.1˚ -7 One of the main advantages of the designed antenna is that the bandwidth and the gain of the antenna can easily be controlled by changing the antenna‟s main parameters. The antenna‟s bandwidth can be varied between 500 MHz and 10GHz by manipulating the slit dimensions. It has been found that the antenna‟s bandwidth is directly proportional to the slit width, while the antenna‟s resonance frequency is inversely proportional to the slit length. The grooves width GW, distance between the 1st groove and slit A and groove‟s period P dimensions have major effect on the antenna‟s radiation pattern and gain characteristics with no major effect on the antenna bandwidth and resonance frequency. Moreover, the position and width of the 1st groove has minor effect on the antenna‟s bandwidth and resonance frequency. Thus, with a constant distance of A „distance between the slit and the 1st groove‟, the antenna‟s bandwidth and resonance frequency can be controlled easily by changing the slit width and length. 35 5.1.2 Steer the antenna’s beam by introducing two identical rectangular slots Introducing two rectangular slots as shown in Figure 5.5 will steer the antenna beam by 20˚ on the entire operation frequencies. The electromagnatic energy is coupled to the antenna using rectangular waveguide that has the same dimensions as the one that has been used in the antenna presented in section 5.1.1. Furthermore, the proposed antenna dimensions are identical to the antenna‟s dimensions that were presented in section 5.1.1. Introducing the two rectangular slots does not only steer the antenna‟s beam, but it also increases the antenna‟s gain at resonance frequency by 2 dB. Figure 5.5: The geometry of the proposed antenna, X1 = 1.5mm, X2=2.9mm, E=0.55mm, S=40mm, SW=2.35mm, SL=0.2mm. The proposed antenna has a bandwidth of 2.9 GHz and resonates at 63 GHz as shown in figure 5.6. The antenna‟s beam is steered by 20˚ with a simulated gain of 42.49 (16.3 dB) at resonance frequency as shown in Figure 5.7. Figure 5.6: Simulated parameter of the proposed antenna. 36 (b) (a) (d) (c) Figure 5.7: The simulated far-field radiation pattern of the proposed antenna design at resonance frequency. (a) 3D radiation pattern. (b) 3D radiation pattern. (c) 2D polar coordinates. The antenna gain performance against the antenna‟s bandwidth is shown in Figure 5.8. Besides, the antenna‟s surface current density is presented in Figure 5.9. It can be seen that the asymmetric current density created by the rectangular slots as shown in Figure 5.9 will steer the antenna beam in a same manner as disscussed in section 4.2. Figure 5.8: The steerable slit grating antenna’s simulated gain over the operation bandwidth. 37 Figure 5.9: The proposed antenna surface current density at resonance frequency. 38 5.2 Bull’s eye millimetre-wave plasmonic antenna with rectangular slit The proposed Bull‟s eye plasmonic antenna is shown in Figure 5.13. The antenna has optimized dimensions that are shown in table 5.3. The suggested antenna resonates at 63GHz with a bandwidth of 9.346GHz as shown in figure 5.14. Figure 5.13: Bull Eye millimetre wave antenna. (a) Front view (b) Top view. Table 5.3: The Bull’s eye plasmonic antenna parameters and specifications Dimension of the whole Geometry W = 34mm W Dimension of the slit SL 34mm. SW = 0.8 mm 2.4 mm. Distance between slit and 1st groove A = Wave guide dimensions a 4.5 mm. 1.8mm b Y = 3.2 mm 4.5mm. Groove‟s depth Gd = 0.94 mm. Groove‟s width GW = 0.15 mm Groove‟s period P = 4 mm. Thickness of the antenna T = 1.2 mm. Figure 5.14: Simulated parameter of the proposed Bull’s eye antenna. 39 The designed Bull‟s eye antenna gain at resonance frequency is 24.1 in linear (13.82 dB) as shown in Figure 5.15. The proposed antenna‟s gain performance over its bandwidth is illustrated in Figure 5.16. (b) (a) Figure 5.15: The simulated far-field pattern of the Bull Eye antenna at resonance frequency. (a) 3D radiation pattern. (b) 2D polar coordinates. Figure 5.16: The Bull Eye antenna gain performance over the entire operation bandwidth. The Bull‟s eye antenna bandwidth can be manipulated in a similar manner to the slit grating structure bandwidth. The slit‟s width is directly proportional to the antenna‟s bandwidth, while the antenna‟s resonance frequency is inversely proportional to the slit length. Similarly to the slit grating structure, simulation results shows that the antenna‟s radiation pattern is a function of groove‟s width GW, groove‟s period P, groove‟s depth Gd and distance between the 1st groove and slit A. Hence, to maximize the antenna‟s far-field performance each parameter should be optimized carefully. 40 5.4 Bull’s eye millimetre-wave plasmonic antenna with circular slit Coupling the electromagnetic energy to the Bull‟s eye antenna by using circular waveguide and circular slit as shown in 5.19 will immensely enhance the antenna‟s impedance bandwidth to be more than 350%. The suggested Bull‟s eye antenna has an endless bandwidth of more than 243 GHz ranging from 57.43GHz to 300 GHz as shown in figure 5.20. Besides, the antenna has a very good simulated gain performance over the entire bandwidth as shown in figure 5.21. Figure 5.19: Top view of the proposed Bull’s eye antenna with circular slit. Table 5.4: The proposed antenna parameters and specifications Dimension of the antenna W radius of the slit r = 1.6 mm. W = 1.6mm. Groove‟s width GW = 0.16 mm. Groove‟s depth Gd = 0.94 mm. Groove‟s period P = 4.5 mm. Thickness of the antenna T = 1.2 mm. Waveguide dimensions r Distance between slit and 1st groove A = Y = 4.072 mm. 1.6mm 5mm. Figure 5.20: Simulated parameter of the proposed plasmonic antenna between 57GHz and 300GHz. 41 The Bull‟s eye with circular slit antenna‟s gain performance between 60 GHz and 70GHz is quite similar to the performance when the slit has rectangular shape as shown in figure 5.21. The antenna has a gain of 13.7 dB at first resonance which is 64 GHz. However, the Bull‟s eye with circular slit gain drops to minimum of 7dB at 75GHz, then it starts to rise steadily as the frequency increases to hit 19.5 dB on 300 GHz as shown in figure 5.22. The antenna parameter‟s performance between 60 GHz and 70 GHz is presented in figure 5.23. Figure 5.21: The proposed antenna’s gain performance between 60GHz and 70GHz. Figure 5.22: The proposed antenna’s gain performance between 57 GHz and 300GHz. Figure 5.23: Simulated parameter of the proposed Bull’s eye antenna between 57GHz and 300GHz. 42 (a) (b) (c) (d) (e) (f) Figure 5.24: The far-field radiation pattern of the Bull’s eye millimetre-wave plasmonic antenna with circular slit at some selected frequencies.(a) 3D at 90 GHz. (b) 2D at 90 GHz. (c) 3D at 200 GHz. (d) 2D at 200 GHz. (e) 3D at 300 GHz. (f) 2D at 300 GHz. 43 Table 5.5 demonstrates a comparison between the performance of the slit grating antenna, Bull‟s eye with rectangular slit and Bull‟s eye with circular slit. One can see that the three antennas have similar gain performance at the resonant frequency, but the Bull‟s eye antenna with rectangular slit has much lower 3dB beam width than the other two proposed antennas. Besides, Bull‟s eye antennas have lower side lobe level than the slit grating antenna. Moreover,both slit grating and Bull‟s eye have similar bandwidth when the slit is rectangular and the feeding is provided by rectangular waveguide, but when the feeding is provided by circular waveguide with circular slit the antenna‟s bandwidth increases dramatically by more than 200 GHz. Table 5.5: Comparison between the slit grating and Bull’s eye millimetre-wave plasmonic antennas Type of the antenna Resonant freque -ncy (GHz) Bandwidth (GHz) Simulated gain at resonance (linear) Simulated gain at resonance (dB) Minimum return loss ( ) (dB) 3dB Beam width Side lobe level (dB) Slit grating 62.1 8.35 29.4 14.7 -28.45 32.1˚ -7 Bull‟s eye with rectangular slit Bull‟s eye with circular slit 63 9.346 24.1 13.82 -27.8 11.5 ˚ -9.7 64 243 23.5 13.7 -20.9 24˚ -8.6 44 CHAPTER 6 CONCLUSIONS AND FUTURE WORK 6.1 Conclusions This research has portrayed novel designs of steerable millimetre-wave plasmonic antennas by controlling and directing radiation at metal surfaces through spoof surface plasmons phenomenon. The proposed antennas characteristics were investigated with various options and were found to operate satisfactory. The designed antennas have excellent beam steer-ability with very high gain performance over the operation bandwidth. The proposed steerable millimetre wave plasmonic antennas presented in this research were based on the two common structures which are: Slit grating structure and Bull‟s eye structures. Two different Slit grating plasmonic antennas resonate on 12 GHz and at the 60 GHz band were designed and were successfully simulated. The two Slit grating plasmonic antennas were simply realised by putting structured metal surface in the near field on top of simple rectangular patch antenna. Placing a structured metal (plasmonic antenna) on top of the patch that resonates at 12 GHz has enhanced the antenna‟s gain by 8.3 dB; meanwhile, placing a structured metal on top of the patch that resonates on the 60 GHz band has enhanced the antenna‟s gain by 4.6 dB. An investigation to steer the slit grating plasmonic antenna that was fed by patch antenna which operates at the 60 GHz has been carried out. It has been found that introducing slots on the plasmonic surface in certain patterns will steer the antenna‟s beam. For example, introducing two slots in shape of crosses on the antenna‟s surface has steered the slit grating millimetre-wave plasmonic antenna by 10˚. Furthermore, it has been found that introducing two rectangular slots has steered the antenna‟s beam by 15˚. It has been proved that coupling the electromagnetic energy to the slit grating millimetrewave plasmonic antenna by using rectangular waveguide has increased the antenna‟s gain by 4.3 dB to reach 14.7 dB. Besides, a simulated gain of 16.3 dB and steerable beam of 20˚ are obtained when the electromagnetic energy is coupled by a rectangular waveguide to the steerable slit grating plasmonic antenna with two rectangular slots. The proposed novel steerable slit grating millimetre-wave plasmonic antenna by introducing slots on the antenna‟s surface is believed to be the first break through of this research. Two millimetre-wave Bull‟s eye plasmonic antennas were some of the fruitful outcomes of this work. The two Bull‟s eye antennas were successfully designed to operate at the 60GHz band with high gain. In addition, it has been demonstrated that changing the Bull‟s eye slit shape and the feeding technique will improve the antenna‟s bandwidth tremendously. For instance, an impedance bandwidth of more than 350% was achieved when the Bull‟s eye millimetre-wave 45 plasmonic antenna slit shape and feeding waveguide was changed from rectangular to circular. An achievement of 350% of impedance bandwidth using the Bull‟s eye structure which is used to have narrow impedance bandwidth prior to this research is believed to be the second breakthrough of this study. 6.2 Recommendations for future work The plasmonic antennas beam were steered in this study by two different methods which are introducing slots on the surface of plasmonic antenna and changing the slit‟s position; meanwhile, further investigation of the possibility of integrating those techniques with other current techniques used to steer the plasmonic antenna to enhance the antenna steer-ability is essential. Bull‟s eye millimetre-wave plasmonic antenna with circular slits and other antennas that presented in this work can be further studied and modelled to support THz communication. Finally, fabrication of the designed antennas is necessarily to validate the simulated results. 46 REFERENCES [1] Ehab A. Omar and Khaled Elsayed. Directional Antenna with Busy Tone for Capacity Boosting and Energy Savings in Wireless Ad-hoc Networks. In: High-Capacity Optical Networks and Enabling Technologies (HONET); 2010 Dec 19-21; Cairo, Egypt. P. 91-95. Available from: IEEE Xplore. [2] Kasch, Burbank J.L and Andrusenko J. The Effects of Antenna Pointing Error on Bit Error Rate Performance in Mobile Directional Antenna Applications. In: CCECE „06; 2006 May; Ontario, Canada. P 1652 – 1657. Available from: IEEE Xplore. [3] 1. Amin M. Abbosh. (2008). Directive antenna for ultra wideband medical imaging Systems International Journal of Antennas and Propagation. Volume 2008. [4] T. D. James, Z. Q. Teo, D. E. Gómez, T. J. Davis and A. Roberts .The plasmonic J-pole antenna. Applied Physics Letter. 2013; 102:033106. [5] Firdaus M, Zhirun Hu and Abdalla M A. Ultra high gain of plasmonic inspired antenna and its beam forming. Antennas and Propagation Conference (LAPC); 2012 Nov 12-13. Loughborough, UK. Available from: IEEE Xplore. [6] Haofei Shi, Xingzhan Wei, Zeyu Zhao, XiaochunDong,Yueguang Lu, and Chunlei Du, “A new surface wave antenna based spoof surface plasmon mechanism”, Microwave AndOptical Technology Letters, October 2010, 52:10. [7] Beruete, M Campillo , Dolado J.S, Rodriguez-Seco, Perea E, Falcone F and Sorolla M. Very low-profile “Bull’s Eye” feeder antenna. Antennas and Wireless Propagation Letters, IEEE.2005; 4: 365 – 368. [8] William L. Barnes, Alain Dereux & Thomas W Ebbesen. Surface plasmon subwavelength optics. Nature. 2003; 424: 824-830 [9] J.B. Pendry, L Martı´n-Moreno, and F.J. Garcia-Vidal, “Mimicking surface plasmons with structured surfaces”, Science, August 2005, 305:847. [10] Zhichao Ruan and Min Qiu, (2007). Slow electromagnetic wave guided in subwavelength region along one dimensional periodically structured metal surface. Applied physics letter. 90, 201906 . DOI: 10.1063/1.2740174. [11]Guangyuan Li, Feng Xiao, Li Kun, Alameh K and Anshi Xu. Theory, Figures of Merit, and Design Recipe of the Plasmonic Structure Composed of a Nano-Slit Aperture Surrounded by Surface Corrugations. IEEE. 2012m, 30 (Issue: 15): 2405 – 2414. [12] Kyoung Youl Park, Meierbachtol C.S., Wiwatcharagoses N and Chahal P. Surface plasmon-assisted terahertz imaging array. In: Electronic Components and Technology Conference (ECTC), 2012 June 1 2012; San Diego, CA. p. 1846 – 1851. Available from: IEEE Xplore [13] Kyoung Youl Park, Meierbachtol C.S., Wiwatcharagoses N and Chahal P. Surface plasmon-assisted terahertz imaging array. In: Electronic Components and Technology Conference (ECTC), 2012 June 1 2012; San Diego, CA. p. 1846 – 1851. Available from: IEEE Xplore. [14] M. Beruete, I. Campillo, J. S. Dolado, J. E. Rodríguez-Seco, E. Perea, F. Falcone. LowProfile Corrugated Feeder Antenna. IEEE Antennas and Wireless Propagation Letters.2005, Vol. 4: 378 – 380. 47 [15] Zhao Zeyo, Huang cheng , Fen Qin and Luo Xiangang. Application of subwavelength corrugated grooves in the antenna field. In: Asia Pasific Microwave Conference (APMC); 2009 Dec 7-10; Singapore. P. 1792 – 1795. Available from: IEEE Xplore. [16] Cheng Huang , Zeyu Zhao and Xiangang Luo. A Highly Directive Slot Antenna with Dielectric Loaded Corrugated Structure. In: International symposium on antenna and propagation (ISAP); 2011, 25 Oct – 28 Oct. Jeju, South Korea. [17] Liu Xing-Xiang and Alu Andrea.Plasmonic low-profile nanoantenna Reflectors.In: Antennas and Propagation Society International Symposium (APSURSI); 2010; 11-17 July 2010. Toronto,Canada. Available from: IEEE Xplore. [18]Figure Retrieved August 10th 2013 from http://www.rfcafe.com/references/electrical/waveguide.htm. [19] David M.Pozar. Microwave engineering,John Wiley & Sons, New York, 2005. [20] IEEE standard definitions of terms for antennas. International standard Std 145-1993. IEEE, Inc.345 East 47th Street, New York, NY 10017-2394, USA. [21] Balanis, C. A. (2005). Antenna Theory : Analysis And Design. Hoboken, New Jersey: John Wiley & Sons, Inc. [22] Aida Vera López, Papapolymerou John, Akira Akiba, Koichi Ikeda and Shun Mitarai,. 60 GHz Micromachined Patch Antenna for Wireless Applications. In: Antennas and Propagation (APSURSI); 2011 July 3-8; Spokane, WA. P. 515 - 518 48 APPENDIX A CLAIM OF POSSIBLE PUBLICATION The dissertation author and the project supervisor believe that the results that were presented in this study worth to be published in two research papers which one of them at least is a journal. We believe that the first publication will be based on the results of the steerable millimetre-wave slit grating antennas that were presented in section 4.2 and section 5.2 and it is expected to be submitted within two week time after the dissertation submission deadline to IET Microwave, Antennas & propagation journal. After that, another research paper will be written based on the Bull‟s eye millimetre-wave plasmonic antenna with circular slit that has very broadband bandwidth. 49 APPENDIX B RECTANGULAR PATCH ANTENNA DESIGN A rectangular patch antenna is designed based on the transmission line model. The patch width has a minor effect on the resonant frequency and major effect on the antenna‟s bandwidth and radiation patterns. However, the Patch length controls the resonant frequency. The design methodology for a patch antenna based on transmission line model is as following [21]: A. Calculation Of The Width And Length Of The Rectangular Patch. Step 1: Calculation of the Width (W) √ Where, c is velocity of light, is the resonant frequency and is the dielectric constant. Step 2: Calculating the Length (L) To calculate the length of the patch, the effective dielectric constant ) should first be calculated. As the frequency of operation increases the effective dielectric constant approaches the value of the dielectric constant of the substrate is given by: ( = ) ( = for ) Then , the actual length is given by the following equation : √ √ –2 √ (3) 50 (1) [ √ ] for (2) APPENDIX C FEASIBILITY STUDY REPORT Course: MSc. Communication System Engineering. Unit: Feasibility Study. Project Title: On the Study of High Gain and Steerable Millimetre-Wave Plasmonic Antenna. Name: Shaker M.M Alkaraki. Student ID: 8437837 Project Supervisor: Dr. Zahirun Hu. Date: 10th May 2013 51 ABSTRACT 56 CHAPTER 1: INTRODUCTION 57 1.1 Background 57 CHAPTER 2: LITERATURE 60 2.1 Previous Studies On Spoof Plasmonic Antennas 60 2.3 Direct Coupling And Beam Steering Possibility Of Millimetre Plasmonic Antenna 62 CHAPTER 3: METHODOLOGY 65 3.1 Background 65 3.2 Preliminary Antenna Design 66 CHAPTER 4: PROJECT PLANNING AND REVIEW OF PROJECT RISK 69 4.1 Project Planning And Milestone Chart 69 4.2 Project Risk 70 REFERENCES 71 APPENDIX A: RISK ASSESSMENT 72 APPENDIX B: RECTANGULAR PATCH ANTENNA DESIGN 76 54 LIST OF FIGURES Figure 1.1: Spoof SPs between metal and dilectric material at Metal interface. Figure 1.2: Metal surface with slit smaller than the incident wave. Figure 2.1: Plasmonic strucures composed of subwavelength apertures. (a) Bull‟s eye structure (b) slit- grating structure. Figure 2.2: Slit grating THz plasmonic antenna. Figure 2.3:Bull‟s Eye THz plasmonic antenna. Figure 2.4: (a) 1 D Periodic corrugated Structure ;(b) Spoof plasmon structure with subwavelength minor grooves in the surface. is the major groove‟s period, is the major groove‟s width and is the major groove‟s depth . Figure 2.5: Farfield radiation in Polar coordinates at 9.0 GHz and 12.0 GHz. Figure 2.6: Geometry of the corrugated directive slot antenna, (a) Top view; (b) Side view. P=11.8mm, P1=9.1mm,w=2.4mm,d=2.7mm,t=1.5mm. Figure 2.7: Electric field distribution of the slot antenna. Figure 2.8: (a) top view of the Bull‟s Eye Antenna (b) cross section of the antenna with parameters =12mm, =11.54mm, =17mm, =10mm and =3.4mm Figure 2.10: Polar coordinate representation of far-field gain radiation pattern for non-symmetrical structure. Figure2.9: Non-Symmetreical Steerable Plasmonic Antenna. Figure 3.1: Steps illustrates the methodology followed in the project. Figure 3.1 : Rectangular Patch antenna Simulated 3D Farfield radiation pattern Figure 3.2: Millimetre wave plasmonic antenna fed by rectangular patch Figure 3.3: of the proposed plasmonic antenna. Figure 3.4: Farfield Radiation pattern of the proposed plasmonic antenna. (a) 3D Farfield Pattern (b) Polar coordinate representation 55 Abstract Many Millimetre wave applications require the design of directive and steerable beam antennas. Recently, plasmonic antenna have shown promising characteristics that makes it an impressive future alternative of phased array antenna for millimetre-wave applications. Plasmonic antennas have the capability to produce directive beam as efficient as array antennas with lower cost and less design complexity. Millimetre-wave plasmonic exploits the surface plasmons(SPs) phenomenon to enable the incidents electromagnetic wave to be confined into dimensions smaller than the wavelength. Surface plasmons localizes the incident wave to the antenna metallic surface and redirect them to produce highly directive beam. It has been shown that introducing corrugation structures on the metallic antenna surface will enable SPs to produce directive and steerable beam. However, this report provides the outline and introduction for the dissertation project which will be detailed study and design high gain and beam steerable millimetre-wave plasmonic antenna through parametric simulation using CST microwave studio software. Finally, an initial Millimetre plasmonic antenna based on existing designs operates at 12GHz with a gain of 13.51Db has been developed and simulated in this report. 56 Chapter 1 Introduction 1.1 Background Wireless communication systems depend on the transmission of information through electromagnetic waves. The coupling between electronic circuits and these electromagnetic waves is achieved by antennas. Thus, proper antenna design would not only enhance the reliability of the wireless communication systems but would reduce the cost as well. However, as wireless technology industry evolves, millimetre and microwave applications have rapidly grown to satisfy the need of this industry. Millimetre Directive antennas is widely deployed in wireless ad hoc network to increase the system capacity and to save energy. The use of such antennas will not only enhance the network throughput, but it will reduce energy dissipation as well [1]. Directive antennas have shown an impressive capabilities in boosting link capacity, enhancing security through decreasing accessibility and boosting bandwidth efficiency through spatial diversity in both military and civil mobile networks[2]. Besides , design of millimetre steerable directive antenna is crucial requirement in the design of radar and imaging systems and such antennas that have high gain and steerable beam capabilities is essential. The main target of any antenna, whether operating in radio frequencies (RF) or optical frequencies is to radiate and receive electromagnetic energy. Thus, well known optical antennas design concepts and procedures can be investigated to be used to design RF antennas and particularly rapidly merging millimetre wave antennas [3]. Plasmonic antennas have been firstly proposed for optical applications and it utilizes plasmonic surface waves to redirect the electromagnetic waves to produce directive beam. The promising characteristics of plasmatic antenna make it an impressive future alternative of phased array antenna for millimetre-wave applications. Plasmonic antennas have the capability to produce directive beam as efficient as array antennas with lower cost and less design complexity. However, the beam steerability of plasmonic antennas is still one of the main challenges in the current antenna designs. Recently, scientist have shown great interest in surface plasmons (SPs), because it explores how electromagnetic fields can be confined in dimensions that is much smaller than the wavelength. 57 Metals such as silver and copper support surface plasmons which are electromagnetic surface waves that propagate on the surface of a metal and dielectric as shown in figure 1.1 .These waves are localized near the surface and originate from the free electrons of the metal. Surface plasmons have shown potential applications recently in subwavelength optics, biophotonics, microscopy, optical antennas and biological sensors [4][5]. Figure 1.1: Spoof SPs between metal and dielectric material at Metal interface[4]. Usually electromagnetic waves cannot propagate into dimensions that are smaller than the wavelength due to diffraction limit. For example, electromagnetic wave will not be confined into slit shown in figure 1.2 if the width of the slit is smaller than the incident wave [6]. Figure 1.2: Metal surface with slit smaller than the incident wave[6] 58 However, the localization of incident wave to the metal surface shown in figure 1.2 can be controlled by introducing corrugated structures that enable the incident waves to be guided and confined into the slit that has dimensions much smaller than the incident wavelength, due to surface plasmons. Besides, It has been demonstrated that periodic corrugation in metal surface will increase the momentum associated with surface plasmon and will enhance the directivity of the radiated beam due to surface plasmon constructive interference. The aim of this project is to study and investigate high gain and steerability of millimeter-wave antennas by controlling and directing radiation at metal surfaces through spoof surface plasmons phenomenon. The main objectives of the project are outlined as follows: To understand the basic concept of „spoof‟ surface plasmon. To practice and familiar with CST Microwave Studio simulation tool To design and simulate Bull‟s Eye or other types of plasmonic millimeter-wave antennas. To perform parametric simulation to analyze the performance of beam steering capability of plasmonic antennas. To investigate the coupling mechanism between the launching antenna and plasmonic one. To investigate the possibility of direct coupling from the circuit or waveguide to the plasmonic antenna. 59 Chapter 2 Literature Review One of the interesting SPs applications is the plasmonic antennas which has a great capability of extreme light and electromagnetic beam concentration. Among these antennas, structures that consist of subwavelength apertures flanked by surface corrugations which called as two dimensional slit-grating structure and three dimensional bull‟s eye structure as shown in figure 2[7]. Figure 2.1: Plasmonic structures composed of subwavelength apertures. (a) Bull’s eye structure (b) slit- grating structure[7]. The above suggested corrugated structures have a great capability to improve the transmission of electromagnetic waves at microwave and optical frequencies due to constructive interference of resonating plasmonic modes. Besides, these modes can be manipulated by changing the structure parameters such as period and depth of the grooves. The grooves have the ability to redirect incident waves to the subwavelength apertures through exciting surface wave in the form of surface plasmons. Then these waves are refocused by the aperture and the transmission is extremely being enhanced [8]. Nevertheless, this approach is well known in design of optical and THz high gain antennas but recently was implemented to design high gain millimetre microwave antennas. 2.1 Previous studies on spoof plasmonic antennas Few studies are available on Plasmonic antennas for millimetre waves. However, several studies have conducted on plasmonic antennas for optical and THz applications, one of those studies suggested two antennas for beam shaping of Terahertz quantum cascade lasers. The proposed antennas operate at frequency of 3 THz and with simulated gain of 16 dBi and 21 dBi respectively. Both antennas are shown in figure 2.2 and 2.3[9]. 60 Figure 2.2: Slit grating THz plasmonic antenna[9]. Figure 2.3:Bull’s Eye THz plasmonic antenna[9]. Haofei et al. has employed spoof surface plasmons phenomenon to design an antenna operates from 8.2 to 12.5 GHz and he has verified the role of spoof surface plasmon by introducing minor sub-wavelength grooves of period smaller than 1/10th of the operation wavelength. In the designed antenna shown in figure 2.4, the feed source is converted into radiated wave via the corrugated structure and the antenna‟s farfield properties depends on the diffraction of surface waves[10]. Figure 2.4: (a) 1 D Periodic corrugated Structure ;(b) Spoof plasmon structure with subwavelength minor grooves in the surface. is the major groove’s period, is the major groove’s width and is the major groove’s depth [10]. The giving two expressions represent the farfield scattering properties of the given grating structure: (2.1) (2.2) 61 Where is the wave vector of the incident wave, vecto of surface wave and in this case , is the diffraction angle, , and is the wave are the diffraction orders on each side. However, according to the author, the radiated enegy is going to be concentrated and maximized when the condition is met, because the diffraction order from both sides will have the same divergence angle. Finally, the simulated farfield radiation patten for the proposed antenna based on the following dimensions, =30mm, =5mm , =4.5mm, =1.2mm and =2.38mm, is presented in figure 2.5. Figure 2.5: Farfield radiation in Polar coordinates at 9.0 GHz and 12.0 GHz[10]. 2.3 Direct coupling and Beam Steering possibility of Millimetre plasmonic antenna A microwave scaled millimetre wave version of optical plasmonic antennas has driven new potential applications recently. Many studies have used a launching antenna to couple electromagnetic energy into the plasmonic antenna. Nevertheless, on study has proposed a highly directive slot antenna with dielectric loaded corrugated structure similar to slit- grating structure previously shown in figure 2. The proposed antenna has symmetrical periodic grating (grooves) on dual side of the slot and the slot is directly fed by a waveguide as exciting source. A dielectric layer with high dielectric constant ( =9.8) is loaded between neighbouring grating to reduce the antenna apertures. The proposed antenna is shown in figure 2.6 and has a gain of 18.1dBi at frequencyof14.5GHz[11]. Figure 2.6: Geometry of the corrugated directive slot antenna, (a) Top view; (b) Side view. P=11.8mm, P1=9.1mm,w=2.4mm,d=2.7mm,t=1.5mm[11]. 62 The improvement mechanism in the antenna directivity is due to constructive superposition of secondary sources radiated from the main source and dielectric layer from the slot antenna. For optimal results the grating period P should be approximately closed to the wavelength and the dielectric thickness t is 1.5mm[11]. The electric field distribution of the proposed antenna is illustrated in figure 2.7. Figure 2.7: Electric field distribution of the slot antenna [11]. Another study has proposed a miliimetre Bull‟s Eye corrugated antenna directly fed by a wave guide feeder. The proposed antenna is presented in figure 9 and it has two resonant frequencies at 16GHz and 12 with a gain of 15dBi at 16GHz [12]. Figure 2.8: (a) top view of the Bull’s Eye Antenna (b) cross section of the antenna with parameters =12mm, =11.54mm, =17mm, =10mm and =3.4mm[12]. The author reported that the slit‟s depth ,h=12mm, determines the longitudinal resonant frequency of the antenna at 16GHz, while the lower resonant frequency at 12GHz is determined by the slit width. The width of every grating and the grating period control the angle where plasmonic like constructive interference take place, hence both controls the antenna‟s gain. Finally, the author demonstrated practically the same non corrugated flat metallic structure has very low gain with nearly isotropic radiation pattern. 63 Firdaus et al. has shown that the plasmonic antenna beam pattern can be controlled such that the antenna become steerable by designing non symmetrical corrugated structure as shown in figure 2.9. The proposed plasmonic antenna resonates at 9GHz with linear gain of 14.1 as shown in figure 2.10[6]. Figure2.9: Non-Symmetreical Steerable Plasmonic Antenna[6] Figure 2.10: Polar coordinate representation of far-field gain radiation pattern for non-symmetrical structure[6]. In the proposed design, the electromagnetic radiation will be concentrated in the non-symmetrical side of the antenna because of the effect of spoof surface plasmon and will focus the radiated beam towards that direction. 64 Chapter 3 Methodology 3.1 Background To accomplish the aim and objectives of this study, millimetre steerable plasmonic antenna needs to be successfully modelled, designed and simulated. The CST Microwave Studio Software package is going to be used to evaluate and test the performance of the suggested antenna. CST is powerful software that enables antenna engineer to simulate antenna performance and various antenna parameters such as: return loss ( ), directivity, gain and radiation patterns. Then, parametric study is going to be conducted to evaluate the effect of different antenna parameters on the proposed design using optimization and sweep facilities provided by CST. The following steps summarize the methodology of this study: i. Literature Review Throughout this research, an extensive study is conducted to understand the theory of surface plasmon radiation and physics behind plasmonic antenna. A further comprehensive investigations need to be conducted to understand the capability of spoof plasmonic antenna to guide and support highly directive and steerable beam. For instance, studies presented in [4] and [5] explain how surface plasmon waves are generated by altering the structure of metals. However, studies presented in [10] ,[11] and [12] illustrate how surface plasmon waves are used to design highly directive millimetre antennas, besides, [10] provides some mathematical analysis that helps in the design of millimetre wave antennas. ii. Preliminary Antenna Design An existing design for spoof plasmonic antenna based on [6], [10] and [12] will be first simulated by using CST and this involves design of Bull‟s eye structure and slit grating structure. Then, this design will be used as a reference to develop a novel millimetre steerable plasmonic antenna. However, at this stage simple slit grating structure millimetre wave plasmonic antenna fed by rectangular patch antenna have been designed to operate at 12GHz and at subsequent stages Bull‟s eye structure will be modelled and designed to achieve the aim of this study. iii. Enhancing and optimizing the preliminary design Further optimization on structures, Bull‟s Eye and Slit grating, need to be done to achieve best possible directivity and bandwidth. Optimization will be done through extensive parametric study for antenna parameters such as: g. Metal thickness of the plasmonic antenna. 65 h. Distance between the coupling patch and the plasmonic antenna. i. Depth and width of each groove. j. Groove‟s number and period. k. Slit dimensions and slit shape, for instance, try to simulate the effect of changing slit shape on the antenna characteristics by simulating different slit shapes such as circular and elliptical. iv. Steerable Antenna Design Investigation of the beam steerability of the designed plasmonic antenna needs to done at this stage. Investigations and parametric simulation will be performed on both designed antennas to achieve beam steerability. The beam pattern of plasmonic antenna can be changed by designing non-symmetrical corrugated structure so that the beam becomes steerable. However, few and limited literature is available on the steerability of the millimetre wave plasmonic antennas, therefore, an extensive efforts to accomplish the steerable antenna design are needed at this stage. v. Investigate the possibility of direct coupling between the antenna and the excitation source The final stage of plasmonic antenna design is to investigate the possibility of achieving direct coupling between plasmonic antenna and feeding method. A patch antenna will be used to couple energy to plasmonic antenna in the initial proposed design. However, at advance stage the possibility of direct feeding from transmission circuit or other feeding method such as using wave guide will be studied and simulated. A summary of the methodology of this project is provided in figure 3.1. comprehensive litreture review Preliminary antenna design Enhancing and optimizing the Preliminary design Steerable plasmonic antenna design directly coupled plasmonic antenna design Figure 3.1: Steps illustrates the methodology followed in the project . 3.2 Preliminary Antenna Design The first step of the plasmonic antenna design is to build a similar antenna structure to the one that presented by Firdaus et al and Haofei et al and in [6] and [10]. The purposes to build such a 66 structure are: to investigate and understand the basic characteristics of Millimetre plasmonic antenna and most importantly to familiarize myself with the CST software and learn its features which will enable me to accomplish the aim and objectives of this research according to the project plan that provided in the following chapter. This basic structure that simulated in this section will be the foundation of the final design that will be presented later on in the dissertation. The resonant frequency of the designed antenna is chosen to be 12GHz and a patch antenna is designed to couple the electromagnetic energy into the plasmonic antenna. The patch antenna can be placed in the near field or in the farfield of the plasmonic antenna[6]. However, the distance between plasmonic and the patch antenna is chosen to be 3.9mm ( /6) .The designed patch antenna is illustrated in figure 10 and detailed design procedure and results are available in appendix B. The proposed patch antenna has bandwidth of 430MHz and has a gain of 3.88 (5.89 Db) at 12GHz as shown in figure 3.1. Figure 3.1: Rectangular Patch antenna Simulated 3D Farfield radiation pattern. Next, the designed patch antenna is used to couple electromagnetic energy into the plasmonic antenna as shown in figure 3.2 and the geometrical dimensions of the designed antenna structure are tabulated in table 3.1. Figure 3.2: Millimetre wave plasmonic antenna fed by rectangular patch 67 Table 3.1: Dimensions of the proposed antenna Groove‟s width = 5mm Dimension of the whole Geometry = 240 mm 100mm Dimension of the slit = Groove‟s period = Groove‟s depth = 5mm 2 mm 21mm Distance between slit and 1st groove = 7.9mm 23mm Distance between Patch and Plasmonic antenna = 3.9 mm Figure 3.3: Return loss ( ) of the proposed plasmonic antenna The proposed plasmonic antenna resonates at 12GHz with a bandwidth of 243MHz and a gain of 22.42 (13.51Db) with a very low side lobe (-9.6 Db) gain as shown figure 3.4. Figure 3.4: Farfield Radiation pattern of the proposed plasmonic antenna. (a) 3D Farfield Pattern (b) Polar coordinate representation 68 Chapter 4 Project Planning and Review of Project Risk 4.1 Project planning and milestone chart The plan that will be followed to achieve the determined aim and objectives of the project based on the methodology described in chapter 3 and ends by submitting the dissertation report on the exact time is shown in the following Gant chart. 69 4.2 Project Risk One of the main expected risks in this project was to delay the submission of the dissertation due to taking much time in learning how to use of the simulation software. However, this has been hardly mitigated during the feasibility study period by learning familiarizing myself with it through designing and simulating initial Millimetre wave plasmonic antenna. Another expected risk during the dissertation might come from any delay caused by losing the whole or part of simulation data because of unexpected machine faults. Thus, backup copies of the simulated data should be saved regularly. In addition to that, a delay might be caused by the difficulty of achieving some of the project objectives specially the steerable and direct antenna coupling parts and this can be mitigated by referring to extra literature, extra investigations and seeking help of the project‟s supervisor. Nevertheless, to eliminate all expected and unexpected risks, an extra float week have been included in the project plan so it will compensate any delay. 70 REFERENCES [1] Ehab A. Omar and Khaled Elsayed. Directional Antenna with Busy Tone for Capacity Boosting and Energy Savings in Wireless Ad-hoc Networks. In: High-Capacity Optical Networks and Enabling Technologies (HONET); 2010 Dec 19-21; Cairo, Egypt. P. 91-95. Available from: IEEE Xplore. [2] Kasch, Burbank J.L and Andrusenko J. The Effects of Antenna Pointing Error on Bit Error Rate Performance in Mobile Directional Antenna Applications. In: CCECE „06; 2006 May; Ontario, Canada. P 1652 – 1657. Available from: IEEE Xplore. [3] T. D. James, Z. Q. Teo, D. E. Gómez, T. J. Davis and A. Roberts . The plasmonic J-pole antenna. Applied Physics Letter. 2013; 102:033106. [4] William L. Barnes, Alain Dereux & Thomas W Ebbesen. Surface plasmon subwavelength optics. Nature. 2003; 424: 824-830 [5] J.B. Pendry, L Martı´n-Moreno, and F.J. Garcia-Vidal, “Mimicking surface plasmons with structured surfaces”, Science, August 2005, 305:847. [6] Firdaus M, Zhirun Hu and Abdalla M A. Ultra high gain of plasmonic inspired antenna and its beam forming. Antennas and Propagation Conference (LAPC); 2012 Nov 12-13. Loughborough, UK. Available from: IEEE Xplore. [7] Guangyuan Li, Feng Xiao, Li Kun, Alameh K and Anshi Xu. Theory, Figures of Merit, and Design Recipe of the Plasmonic Structure Composed of a Nano-Slit Aperture Surrounded by Surface Corrugations. IEEE. 2012m, 30 (Issue: 15): 2405 – 2414. [8] Kyoung Youl Park, Meierbachtol C.S., Wiwatcharagoses N and Chahal P. Surface plasmonassisted terahertz imaging array. In: Electronic Components and Technology Conference (ECTC), 2012 June 1 2012; San Diego, CA. p. 1846 – 1851. Available from: IEEE Xplore. [9] Akalin T, Beruete M, Navarro-Cia, Ducournau G, Lampin J.F. and Sorolla M. Plasmonic antenna for beam-shaping of Terahertz Quantum Cascade Lasers. In: Antennas and Propagation (EUCAP). 2011 April 11-15; Rome, Italy. P.3329 – 3330. Available from: IEEE.Xplore. [10] Haofei Shi, Xingzhan Wei, Zeyu Zhao, XiaochunDong,Yueguang Lu, and Chunlei Du, “A new surface wave antenna based spoof surface plasmon mechanism”, Microwave And Optical Technology Letters, October 2010, 52:10. [11] Cheng Huang , Zeyu Zhao and Xiangang Luo. A Highly Directive Slot Antenna with Dielectric Loaded Corrugated Structure. In: International symposium on antenna and propagation (ISAP); 2011, 25 Oct – 28 Oct. Jeju, South Korea. [12] Beruete, M Campillo , Dolado J.S, Rodriguez-Seco, Perea E, Falcone F and Sorolla M. Very low-profile “Bull‟s Eye” feeder antenna. Antennas and Wireless Propagation Letters, IEEE.2005; 4: 365 – 368. [13] Balanis C.A, “Antenna theory: analysis and design”, New Jersey: John Wiley & Sons, 2005, 71 APPENDIX A RISK ASSESMENT 73 74 75 Appendix B Rectangular Patch Antenna Design A rectangular patch antenna is designed based on the transmission line model. The patch width has a minor effect on the resonant frequency and major effect on the antenna‟s bandwidth and radiation patterns. However, the Patch length controls the resonant frequency. The design methodology for a patch antenna based on transmission line model is as following [13]: B. Calculation Of The Width And Length Of The Rectangular Patch. Step 1: Calculation of the Width (W) √ Where, c is velocity of light, is the resonant frequency and is the dielectric constant. Step 2: Calculating the Length (L) To calculate the length of the patch, the effective dielectric constant ) should first be calculated. As the frequency of operation increases the effective dielectric constant approaches the value of the dielectric constant of the substrate is given by: ( = ) ( = for (1) ) [ √ ] for (2) Then , the actual length is given by the following equation : √ √ –2 √ (3) Where L is given by : ( ) ( ) The final dimension of the optimized antenna are illustrated in table 1. 76 (4) Figure 1: Model of Rectangular patch antenna Table 1: description of rectangular patch antenna parameters Patch Length L = 4.77mm Patch width W= 5mm Inset depth Inset width = 1.5 mm =1.5mm Substrate thickness = 1.6 mm Feed Line Width Substrate dielectric constant = 5.2 Antenna Geometry = 10mm = 0.80 mm 8.5mm The designed patch antenna has a bandwidth of 430MHz and has a gain of 3.88 (5.89 dB) at 12GHz . parameter of the proposed antenna is shown in figure 2 and the farefield radiation patterns are shown in figure 3 and for simultaneously . Figure 2 : simulated parameter of the proposed patch antenna 77 Figure 3: Simulated farfield of the proposed patch antenna. (a) polar coordinate representation (b) 3D representation. 78
© Copyright 2025