1. No category

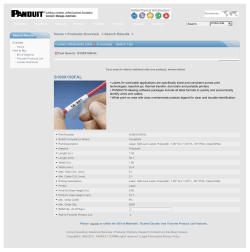
2015 ME Graduate Student Conference
April , 2015
STUDY OF RADIATION HEAT TRANSFER OVER A POWDER BED FOR SELECTIVE
LASER MELTING PROCESS USING A MULTI-LAYER MODEL.
Manish Patil
M.S. Candidate
Faculty Advisor: Dr. Shengmin Guo
ABSTRACT
Use of the laser energy to melt the metallic and non metallic
powders in order to build the components of different
shapes is a commonly used process nowadays in the
industry. In Selective Laser Melting process (SLM), the
energy transferred from the laser beam is utilized in melting
of the metallic powdered bed. The interaction of laser bed
and the powder metal becomes quite complicated because
of an inhomogeneous and a porous nature of a powdered
bed.
Several attempts have been made to evaluate the Radiative
heat transfer in absorbing emitting and scattering medium
for a SLM process. In most cases, the previously developed
models treat the bed as a bulk material [2 ] [3]. and assign
the same physical properties throughout the depth of the
bed. But in reality, the absorbed, the scattered, the emitted
and the reflected laser beam interacts differently with the
metal particles as it propagates further down into the
powdered bed. When the depth of the bed is much greater
than the wavelength of incident radiation, then treating the
power bed as the homogeneous bulk material can no longer
be accurate to capture the physics of the process. In our
model, we segment the powder bed in to multiple
homogeneous sub layers of very small thickness, and treat
the individual layer with a different physical properties so
that we can essentially go much closer to the realty. [see
Fig. 1 ]
In the typical process, the thickness of powdered bed is
taken around 50 to 100 microns, the wave length of the
monochromatic laser heat source caries from 1 to 5
microns. Radiation heat transfer in the porous bed is largely
affected by the physical and optical properties of the
material such as shape and sizes of the particles in the
powdered bed, wavelength of incident radiation,
absorbance, reflectance, scattering coefficients, refractive
index and porosity of the bed which is a function of a depth.
The famous radiative heat transfer equation for a emitting,
absorbing and scattering medium written in the following
form (eqn.1 )
(1)
The equation gives us a change energy per unit volume
when an incident radiation with an intensity
propagates through an absorbing, emitting and a scattering
medium with an observation direction and along the path
(s). The gain or loss in the energy per unit volume is a
summation of energy during emission, absorption and
scattering processes which depends on the absorption (
and the scattering coefficients
of the given material.
The equation is simplified using
which is a spectral
extinction coefficient (
) and
is a spectral albedo.
Fig. 1
Further, this integro-differential equation is solved for a one
dimensional parallel case. Two flux method is effective in
order to evaluate this simple case. This technique is also
39
used by Gusrov A.V. and his team for his model for a bulk
material[3] [11]. The positive and the negative fluxes
represents the incoming and outgoing radiation in a semi
transparent slab. Our multi layer approach tracks down the
path of individual ray as it travel in different layers of the
slab. [See Fig. 2]
This will generates the sets of connected linked integro
differential equations for each layer. The semitransparent
boundaries are considered for all inner sublayers. in order to
solve these coupled equations. The final energy flux density
is calculated by the sum of ∑ I+ and ∑ I -. This approach is
novel approach is a hybrid method which combines
analytical method and ray tracing method for a radiation
heat transfer in a inhomogeneous porous material.
REFERENCES
Fig. 2
The incoming and outgoing fluxes from the other layers are
also taken into account while calculating the total flux for
each independent layer. For n-number of layers the positive
fluxes (outgoing) are calculated as a sum of the number of
rays reflected from surface 2 and a number of rays scattered
from surface 2
+
.
Whereas the negative fluxes ( Incoming) are given by the
sum of the number of rays reflected from surface 1 and a
number of rays scattered from surface 1
+
.
The unique number which is assigned to the fluxes I(m,n,l),
represents the layer number, absorbed or reflected and
scattered count.
[see Fig 3]
Fig 3.
[1] Vandenbroucke B, Kruth J-P. Selective laser melting of
biocompatible metals for rapid manufacturing of
medical parts. Rapid Prototyping J 2007;13/4.
[2] Kruth J-P, Vandenbroucke B, Van Vaerenbergh J, Naert
I. Digital manufacturing of biocompatible metal
frameworks for complex dental prostheses by means of
sls/slm. In: Proceedings of 2nd international conference
on advanced research in virtual and rapid prototyping
(VRAP2005), Leiria, Portugal. Balkema: Taylor &
Francis; September–October 2005. p. 139–45, ISBN 0415-39062-1.
[3] Gusarov AV, Yadroitsev I, Bertrand Ph, Smurov I.
Model of radiation and heat transfer in laser–powder
interaction zone at selective laser melting. J Heat Transf
2009;131:072101.
[4] Ranner LE. Efficient cooling of injection molding tools
with conformal cooling channels, an introductory
analysis. In: international conference on advanced
research in virtual and rapid prototyping 2003; 2003.
[5] Kruth J-P, Levy GN, Klocke F, Childs THC.
Consolidation phenomena in laser and powder-bed
based layered manufacturing. Ann CIRP 2007;56/2:730–
59.
[6] Zhang Y, Faghri A, Buckley CW, Bergman TL. Threedimensional sintering of two-component metal powders
with stationary and moving laser beams. Trans ASME
2000;122:150–8.
[7] Chen T, Zhang Y. Numerical simulation of twodimensional melting and resolidification of a twocomponent metal powder layer inselective laser sintering
process. Num Heat Transf A 2004;46: 633–49.
[8] Chen T, Zhang Y. Three-dimensional modeling of
selective laser
sintering of two-component metal
powder layers. J Manuf Sci Eng 2006;128:299–306.
[9] Chen T, Zhang Y. A partial shrinkage model for selective
laser sintering of a two-component metal powder layer.
Int J Heat Mass Transf 2006;49:1489–92.
[10] Gusarov AV, Laoui T, Froyen L, Titov VI. Contact
thermal conductivity of a powder bed in selective laser
sintering. Int J Heat Mass Transf 2003;46:1103–9.
[11] Gusarov AV, Kruth J-P. Modelling of radiation transfer
in metallic powders at laser treatment. Int J Heat Mass
Transf 2005;48: 3423–34.
40