1. No category

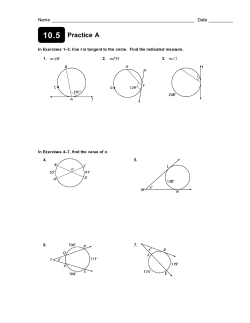
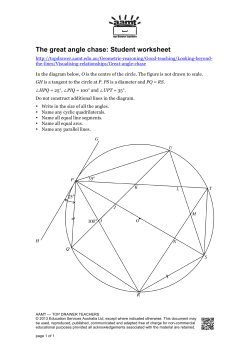
DO NOT EDIT--Changes must be made through “File info”
CorrectionKey=NL-A;CA-A
Name
Class
Date
15.3 Tangents and Circumscribed
Angles
Essential Question: What are the key theorems about tangents to a circle?
Explore
Resource
Locker
Investigating the Tangent-Radius Theorem
A tangent is a line in the same plane as a circle that intersects the circle in exactly one
point. The point where a tangent and a circle intersect is the point of tangency.
P
In the figure, the line is tangent to circle C, and point P is the point of tangency.
You can use a compass and straightedge to construct a circle and a line tangent to it.
Use a compass to draw a circle. Label the center C.
B
Mark a point P on the circle. Using a straightedge, draw a
tangent to the circle through point P. Mark a point Q at a
different position on the tangent line.
D
© Houghton Mifflin Harcourt Publishing Company
Q
A
C
C
P
C
_
Use a straightedge to draw the radius CP.
Use a protractor to measure ∠CPQ. Record the result in the table. Repeat the process two
more times. Make sure to vary the size of the circle and the location of the point of tangency.
Vehicle 1
Measure of ∠CPQ
Circle 1
Circle 2
Circle 3
90°
90°
90°
Reflect
1.
Make a Conjecture Examine the values in the table. Make a conjecture about
the relationship between a tangent line and the radius to the point of tangency.
A tangent line and the radius to the point of tangency are perpendicular to each other.
2.
Discussion Describe any possible inaccuracies related to the tools you used
in this Explore.
It is difficult to accurately construct the tangent using just a straightedge and a point on
the circle. This may cause the angle measurements to vary slightly from 90°.
Module 15
GE_MNLESE385801_U6M15L3.indd 805
805
Lesson 3
02/04/14 11:44 AM
DO NOT EDIT--Changes must be made through “File info”
CorrectionKey=NL-A;CA-A
Explain 1
DO NOT
Correcti
Proving the Tangent-Radius Theorem
The Explore illustrates the Tangent-Radius Theorem.
Tangent-Radius Theorem
If a line is tangent to a circle, then it is perpendicular to a radius
drawn to the point of tangency.
m
Example 1 Complete the proof of the Tangent–Radius Theorem.
Given: Line m is tangent to circle C at point P.
P
¯⊥ m
Prove: CP
C

¯ is not perpendicular to line m.
Use an indirect proof. Assume that CP
¯ ⊥ m.
There must be a point Q on line m such that CQ
¯ ⊥ m, then △CQP is a right triangle, and CP > CQ because
CQ
¯
hypotenuse of the right triangle.
CP
is the
If

C
m are in the exterior of the circle.
This means point Q is in the exterior of the circle. You can conclude
that CP < CQ because
¯
CP
is a radius of circle C.
© Houghton Mifflin Harcourt Publishing Company

P
Since line m is a tangent line, it can intersect circle C at only point P ,
and all other points
of line

m
Q
This
_ contradicts the initial assumption that a point Q exists such that
CQ ⊥ m, because that meant that CP > CQ. Therefore, the assumption
¯
is false and CP must be perpendicular to line m.
Reflect
3.
_
Both lines in the figure are tangent to the circle, and AB is a diameter. What can
you conclude about the tangent lines?
Since the tangent lines are both perpendicular to ¯
AB, they are
A
parallel to each other.
B
Module15
GE_MNLESE385801_U6M15L3.indd 806
806
Lesson3
3/24/14 11:06 PM
DO NOT EDIT--Changes must be made through “File info”
CorrectionKey=NL-A;CA-A
The converse of the Tangent-Radius Theorem is also true. You will be asked to prove
this theorem as an exercise.
Converse of the Tangent-Radius Theorem
If a line is perpendicular to a radius of a circle at a point on the circle, then it is
tangent to the circle at that point on the circle.
Explain 2
Constructing Tangents to a Circle
From a point outside a circle, two tangent lines can be drawn to the circle.
Example 2 Use the steps to construct two tangent lines from a point outside a circle.
 Use a compass to draw a circle. Label the center
C.
A
C
M
X
B
 Mark a point
X outside
_the circle and use a
straightedge to draw CX.
 Use a compass and_straightedge to construct
the midpoint of CX and label the midpoint M.
 Use a compass to construct a circle with center
M and radius CM.
of circle C and circle M as A and B.
 Label the points of intersection
‹ ›
‹ ›
−
−
© Houghton Mifflin Harcourt Publishing Company
Use a straightedge to draw XA and XB . Both lines are tangent to circle C.
Reflect
4.
5.
_
‹ ›
‹ ›
−
−
How can you justify that XA (or
CA on the diagram.)
_XB ) is a tangent line? (Hint: Draw
_
The endpoints of diameter CX lie on the inscribed angle ∠CAX of circle M. By the
_
Angle Inscribed in a Semicircle Theorem, ∠CAX is a right angle. By the Converse of the
‹ ›
−
Tangent-RadiusTheorem, XA is a tangent line to circle C. The same reasoning shows
‹ ›
−
that XB is a tangent line.
_
_
Draw CA and CB on the diagram. Consider quadrilateral CAXB. State any conclusions
you can reach about the measures of the angles of CAXB.
The sum of the measures of the angles is 360°. There are two right angles, ∠CAX and ∠CBX.
∠AXB and ∠ACB are supplementary.
Module15
GE_MNLESE385801_U6M15L3.indd 807
807
Lesson3
23/03/14 2:00 AM
DO NOT EDIT--Changes must be made through “File info”
CorrectionKey=NL-A;CA-A
Explain 3
DO NOT
Correcti
Proving the Circumscribed Angle Theorem
A circumscribed angle is an angle formed by two rays from a common
endpoint that are tangent to a circle.
Circumscribed Angle Theorem
A circumscribed angle of a circle and its associated central angle
are supplementary.
Example 3 Prove the Circumscribed Angle Theorem.
Given: ∠AXB is a circumscribed angle of circle C.
A
Prove: ∠AXB and ∠ACB are supplementary.
_
_
Since ∠AXB is a circumscribed angle of circle C, XA and XB are
C
X
tangents to the circle. Therefore, ∠XAC and ∠XBC are
B
right angles by the Tangent-Radius Theorem .
In quadrilateral XACB, the sum of the measures of its four angles is 360° .
Since m∠XAC + m∠XBC = 180° , this means m∠AXB + m∠ACB = 360° - 180° = 180° .
So, ∠AXB and ∠ACB are supplementary by the definition of supplementary angles .
Reflect
6.
Is it possible for quadrilateral AXBC to be a parallelogram? If so, what type of parallelogram must it be? If
not, why not?
Yes. If AXBC is a parallelogram, it must be a square.
→
→
‾_and KN
‾ are tangent to circle C. Explain how to show that
1. KM
_
KM ≅ _
KN, using congruent triangles.
Draw KC. This creates two right triangles, △KCM and
_
_ _
△KCN.Since ¯
CM ≅ CN andCK ≅ CK, the triangles are
congruent by the Hypotenuse-Leg Theorem.
_
It follows that ¯
KM ≅ KN by CPCTC.
2.
M
C
K
N
Essential Question Check-In What are the key theorems regarding tangent lines to a circle?
If a line is tangent to a circle, it is perpendicular to the radius drawn to the point of
© Houghton Mifflin Harcourt Publishing Company
Elaborate
tangency. Conversely, if a line is perpendicular to a radius of a circle at a point on the
circle, it is tangent to the circle. Also, a circumscribed angle of a circle and its associated
central angle are supplementary.
Module15
GE_MNLESE385801_U6M15L3.indd 808
808
Lesson3
23/03/14 1:59 AM