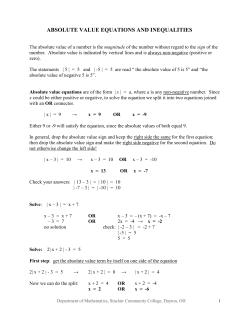
Solving Inequalities
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
Solving Inequalities
Inequalities are solved for x using the same steps as equations.
Clear parenthesis using distribution.
Combine variable terms.
To keep from having to multiply or divide by a negative number, make sure the
final variable term has a positive coefficient.
Add/subtract to get constant terms isolated.
Multiply/divide to isolate the variable. If you multiply or divide by a negative number
you must flip the inequality sign.
For final numeric solutions to match the direction of the graphic solution, the variable
should be on the left side of the inequality. “Flip” the inequality if needed. Make sure to
keep the correct order of the inequality.
If 3
x, then x
3.
3
Solutions can be written verbally, symbolically, and graphically.
Justify solutions algebraically by testing a value in the selected interval solution or by
using the graphing calculator to analyze tables and graphs.
Sample Solutions
Verbal
Symbolic
x is less than two
x is fewer than two
x
2
x is less than or equal to two
x is no more than two
x is at most two
x
2
x is greater than two
x is more than two
x
2
x
2
x is greater than or equal to two
x is no less than two
x is at least two
Graphic
2
2
2
2
Symbols used in Special Case examples
means all real numbers work, shown graphically as
means no solution, no graph since no numbers work
©2012, TESCCC
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
Solving Inequalities
Sample Problems
Solve the following inequalities, giving both a symbolic and number line solution.
Use algebraic methods.
Check solutions for reasonableness
1. 2(2 – x) < x – 2
Algebraic Method
©2012, TESCCC
Justification by Graphing and Table
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
Solving Inequalities
2.
5x 4
6
Algebraic Method
Justification by Graph and Table
3. 6(x + 2) – 4x 8
Algebraic Method
Justification by Graph and Table
4. 5 9
x
4 x 18
Window: {x: -10, 10, 1} {y: 0, 80, 10}
Algebraic Method
©2012, TESCCC
Justification by Graph and Table
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
Solving Inequalities
5. 4 x
7
4
4x
x Special Case
Algebraic Method
Justification by Graph and Table
6. 5x 7 5x 8 Special Case
Algebraic Method
Justification by Graph and Table
What do both special cases have in common?
How do you know if the answer is empty set or all real numbers?
©2012, TESCCC
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
Solving Inequalities
Class-Fun/Home-Fun
Name:____________________________
Block:_____ Date:_________________
Directions: Solve each inequality as indicated, giving the solution in both symbolic and number
line form. Show all work and solutions on paper.
Use a graph and table to solve.
1. 3(x + 4) – 5(x – 1) 5
Use algebraic properties to solve. (Justify solutions by graphs and tables.)
1
2.
x 3 9
5
3. 8
3
x
5
4. 5x
23
5x 11
5. 13 17 x
©2012, TESCCC
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
Algebra 1
HS Mathematics
Unit: 03 Lesson: 01
6. 5(x + 3) – 2x
21
7. 3(3x + 1) – (x – 1)
6(x + 10)
8. 4(2x + 1) < 2( x – 1) + 6(x + 2)
Write and solve an inequality that models each situation.
9. Beatriz is in charge of setting up a banquet hall. She has five tables that will seat six
people each. If no more than 62 people will attend, how many more tables seating four
people each will she need?
10. The student council is sponsoring a concert as a fund raiser. Tickets are $3 for students
and $5 for adults.The student council wants to raise at least $1000. If 200 students attend,
how many adults must attend?
©2012, TESCCC
Editable Resource Document (ERD)–Content may be modified (DULA terms and conditions still apply)
© Copyright 2025