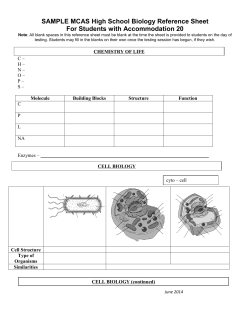
10 Grade Includes: GLENCOE
GLENCOE MATHEMATICS Grade Includes: • West Virginia Content Standards and Objectives, Tenth Grade, Correlated to Glencoe Algebra 1 and Glencoe Geometry • Student Recording Chart • Diagnostic Test • Numerous Practice Questions for Each Objective • Full-Size Sample Test 10 Test-Taking Tips • Go to bed early the night before the test. You will think more clearly after a good night’s rest. • Read each problem carefully and think about ways to solve the problem before you try to answer the question. • Relax. Most people get nervous when taking a test. It’s natural. Just do your best. • Answer questions you are sure about first. If you do not know the answer to a question, skip it and go back to that question later. • Think positively. Some problems may seem hard to you, but you may be able to figure out what to do if you read each question carefully. • If no figure is provided, draw one. If one is furnished, mark it up to help you solve the problem. • When you have finished each problem, reread it to make sure your answer is reasonable. • Become familiar with a variety of formulas and when they should be used. • Make sure that the number of the question on the answer sheet matches the number of the question on which you are working in your test booklet. Copyright © by The McGraw-Hill Companies, Inc. All rights reserved. Printed in the United States of America. Except as permitted under the United States Copyright Act, no part of this book may be reproduced in any form, electronic or mechanical, including photocopy, recording, or any information storage or retrieval system, without prior written permission of the publisher. Send all inquiries to: The McGraw-Hill Companies 8787 Orion Place Columbus, OH 43240-4027 ISBN: 0-07-868587-7 WESTEST Grade 10 Practice and Sample Test Workbook 1 2 3 4 5 6 7 8 9 10 079 13 12 11 10 09 08 07 06 05 04 Contents Student Recording Chart . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . iv West Virginia Content Standards and Objectives, Tenth Grade, Correlated to Glencoe Algebra 1 and Glencoe Geometry . . . . . . . . . . v Formulas . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . viii Test Practice Diagnostic Test . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1 Standards Practice 10.1 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 11 10.2 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 16 10.3 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 27 10.4 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 34 10.5 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 40 Test Practice Sample Test . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 46 How to Use This Workbook The material in this booklet is designed to help you prepare for the West Virginia Educational Standards Test (WESTEST), Grade 10. Copyright © Glencoe/McGraw-Hill Diagnostic Test This test will help you identify any weaknesses you may have as you prepare to take the WESTEST 10. Once you’ve taken the test and it’s been graded, complete the Student Recording Chart that is found on page iv. Mark an × in the square for each question that they answered incorrectly. Practice If you missed one or two of the questions for a particular objective, you could probably use some extra practice with that objective. The Student Recording Chart lists practice pages for each objective. Complete the appropriate practice pages. If you are unsure about how to do some of the problems, you may want to refer to your mathematics book. Sample Test After you have completed your practice worksheet(s), take the Sample Test on pages 46 to 55. WESTEST, Grade 10 iii Student Recording Chart Directions Mark an × by each question from the Diagnostic Test that you answered incorrectly. If there are one or two ×s marked for a SOL, write Yes in the Need Practice? box. Then complete the practice pages for that standard. Standard 10.1.1 10.1.2 10.1.3 10.2.1 10.2.2 11 ■ 19 ■ 4 ■ 33 ■ 41 ■ 33 ■ 18 ■ 1 ■ 23 ■ 31 ■ 44 ■ 11, 14–15 12, 14–15 13–15 16, 25–26 17, 25–26 10.2.3 10.2.4 10.2.5 10.2.6 10.2.7 7 ■ 39 ■ 14 ■ 16 ■ 13 ■ 29 ■ 27 ■ 36 ■ 18, 25–26 19, 25–26 20, 25–26 21, 25–26 22, 25–26 10.2.8 10.2.9 10.3.2 10.3.3 10.3.4 24 ■ 10 ■ 21 ■ 6 ■ 26 ■ 20 ■ 34 ■ 15 ■ 40 ■ 42 ■ 33 ■ 23, 25–26 24–26 27, 32–33 28, 32–33 29, 32–33 10.3.5 10.4.1 10.4.2 10.4.4 3 ■ 30 ■ 42 ■ 33 ■ 8 ■ 38 ■ 25 ■ 43 ■ 2 ■ 12 ■ 28 ■ 32 ■ 43 ■ 48 ■ Practice Pages 30–33 34, 38–39 35, 38–39 36–39 Standard 10.5.1 10.5.2 10.5.3 10.5.4 17 ■ 22 ■ 45 ■ 33 ■ 5■ 35 ■ 45 ■ 9 ■ 37 ■ 45 ■ 33 ■ 40, 44–45 41, 44–45 42, 44–45 43–45 Test Questions Need Practice? Practice Pages Standard Test Questions Need Practice? Practice Pages Standard Test Questions Need Practice? Practice Pages Standard Test Questions Test Questions Need Practice? Practice Pages iv WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill Need Practice? West Virginia Content Standards and Objectives, Tenth Grade, Correlated to Glencoe Algebra 1 and Glencoe Geometry Lessons in which the standards are a primary focus are indicated in bold. Algebra 1 Lesson(s) Objectives Geometry Lesson(s) Standard 1: Number and Operations Students will: • demonstrate understanding of numbers, ways of representing numbers, and relationships among numbers and number systems; • demonstrate meanings of operations and how they relate to one another; and • compute fluently and make reasonable estimates through communication, representation, reasoning and proof, problem solving, and making connections within and beyond the field of mathematics. 10.1.1 Solve computational and practical problems using 1-2, 1-5, 1-6, 2-1, 2-2, 1-2, 1-3, 1-6, 3-3, 3-5, properties of numbers, order of operation, 2-3, 2-4, 2-7, 10-6, computation, and estimation with decimals, 11-1, 11-2 fractions, integers, and mixed numbers, including ratio, proportion, and percents. (AM1.1, AGP.1, AGP.2, AGP.17) 10.1.2 Write numbers involving scientific notation and 4-1, 4-3, 4-4, 4-7, 6-1, 6-2, 6-3, 6-4, 7-2, 8-2, 11-1 8-3 combine numbers written in scientific notation to solve practical problems. (AM1.2) 10.1.3 Estimate and simplify square roots into both 2-7, 11-1, 11-2 exact and approximate forms. (AM1.14, A1.16) PS10, 1-3, 7-2, 7-3, 15-3 Standard 2: Algebra (MA.S.2) Students will: • demonstrate understanding of patterns, relations, and functions; • represent and analyze mathematical situations and structures using algebraic symbols; • use mathematical models to represent and understand quantitative relationships; and • analyze change in various contexts through communication, representation, reasoning and proof, problem solving, and making connections within and beyond the field of mathematics. Copyright © Glencoe/McGraw-Hill 10.2.1 Define variables and solve multi-step linear equations and one-variable inequalities, interpret results on a number line and apply the skills toward solving practical problems. (AM1.10, AM1.11, AGP.18, A1.2, A1.3) 10.2.2 Solve literal equations for a given variable and 1-3, 3-4P, 3-4, 3-5, 1-2, 1-3, 1-4, 1-5, 1-6, 3-6, 3-7, 3-8, 6-1, 6-2, 3-2, 3-6, 4-6, 5-1, 5-4, 6-3, 6-4, 6-5 5-5, 6-1, 6-3, 7-4, 8-1, 8-4, 10-3, 10-4, 10-6, PS6, PS7 3-8 1-2 apply the skills toward solving practical problems. (AM1.8, A1.4) 10.2.3 Solve practical problems using a four-step problem 3-1, 3-4P, 3-4, 3-5, solving approach, justifying steps based on the properties of real numbers. (AM1.9, AM1.7) 2-5, 2-6 3-6 P = Preview Lesson, F = Follow-Up Lesson, PS = Prerequisite Skill Lesson, RM = Reading Math WESTEST, Grade 10 v Algebra 1 Lesson(s) Objectives 10.2.4 Evaluate and simplify algebraic expressions using 1-1, 1-2, 1-4, 1-5, grouping symbols, order of operations, properties of 1-6, 8-1, 8-2, 8-3, real numbers with justification of steps, and the laws 8-6, 8-7, 8-8 of exponents. (AGP.17, AM1.5, AM1.15, A1.7) 10.2.5 Solve absolute value equations in one variable and 6-5 Geometry Lesson(s) PS5, PS10, PS11, PS12 1-3 interpret the results on a number line. (AM1.12, A1.6) 10.2.6 Analyze a given set of data for the existence of a pattern numerically, algebraically and graphically. (AM2.1, A1.6) 10.2.7 Determine the slope of a line given an equation of a line, the graph of a line and two points to be identified. (AM2.2, A1.8) 10.2.8 Write and graph linear equations. (AM2.3, AM2.4, A1.9, A1.10) 4-7P, 4-7, 4-8, RM4, 2-1 10-7 5-1, 5-2, 5-3, 5-3F, 5-4, 5-5, 5-6 3-3, 3-4, 3-5, 3-6 4-5, 4-5F, 4-8, 5-2, 3-4, 3-5, 3-6 5-3P, 5-3, 5-3F, 5-4, 5-5, 5-6 10.2.9 Factor and perform basic operations on simple 8-5P, 8-5, 8-6, 8-7P, 10-8, PS10, PS11, polynomials. (AM2.7, AM2.8, AM2.9, A1.13, A1.14, 8-7, 8-8, 9-2P, 9-2, PS12, PS13 A1.15) 9-3P, 9-3, 9-4, 9-5, RM9, 9-6, 12-3, 12-4, 12-5 10.3.1 Use appropriate tools to make geometric 1-2, 1-3, 1-4, 1-5P, 3-5, 4-4, 4-5, 5-1P, 6-4, 8-4, 8-5, 8-6, 10-3, 10-5P, 10-5, 10-8 constructions. (AGP.9) 10.3.2 Identify angle relationships and apply in solving 1-5, 2-8, 3-1, 3-2, 3-5, 6-3, 6-4 problems (complementary, supplementary, vertical and adjacent as well as relationships formed by parallel lines cut by a transversal). (AGP.13, AGP.14) 10.3.3 Investigate similar figures and apply proportions in problem solving situations. (AGP.15) vi WESTEST, Grade 10 11-3, 11-7P, 11-7 6-2, 6-3, 6-4, 6-5, 6-6, 7-1, 9-5, 13-4 Copyright © Glencoe/McGraw-Hill Standard 3: Geometry Students will: • analyze characteristics and properties of two- and three-dimensional geometric shapes and develop mathematical arguments about geometric relationships; • specify locations and describe spatial relationships using coordinate geometry and other representational systems; • apply transformations and use symmetry to analyze mathematical situations; and • solve problems using visualization, spatial reasoning, and geometric modeling through communication, representation, reasoning and proof, problem solving, and making connections within and beyond the field of mathematics. Algebra 1 Lesson(s) Objectives 10.3.4 Explore circle relationships, emphasizing the vocabulary of circles. (AGP.16) 10.3.5 Solve right triangle problems using the Geometry Lesson(s) 1-1, 3-8, 8-2, 11-3, PS 10-2, 10-3, 10-4, 10-5, 10-6 11-4 1-3, 7-2, 7-3, 9-6 Pythagorean Theorem. (MA8.4.4) Standard 4: Measurement Students will: • demonstrate understanding of measurable attributes of objects and the units, systems, and processes of measurement; and • apply appropriate techniques, tools and formulas to determine measurements through communication, representation, reasoning and proof, problem solving, and making connections within and beyond the field of mathematics. 10.4.1 Calculate the missing measures of angles and 8-5, 11-7P, 11-7, 14-3 1-6, 8-1, 11-3 lengths of sides of a polygon from given data, using formulas. 10.4.2 Estimate, measure, and perform operations 1-2, 13-1, 13-2, 13-3, 13-4, PS22 involving length, mass, and capacity using customary and metric units. (AGP.7) 10.4.3 Use appropriate tools to measure geometric 1-2 figures. (AGP.8) 10.4.4 Develop and apply formulas for area, perimeter, 3-1, 8-1, 8-1F, 8-7, surface area, and volume and apply them in solving practical problems. (AGP.10, AGP.11, AGP.12) 12-5, PS 6-2, 6-5, 11-1, 11-2, 11-3, 11-5, 12-3, 12-4, 12-5, 12-6, 12-7, 13-1, 13-2, 13-3, 13-4, PS14 Copyright © Glencoe/McGraw-Hill Standard 5: Data Analysis and Probability Students will: • formulate questions that can be addressed with data, and collect, organize, and display relevant data to answer them. • select and use appropriate statistical methods to analyze data; develop and evaluate inferences and predictions that are based on models; and • apply and demonstrate an understanding of basic concepts of probability through communication, representation, reasoning and proof, problem solving, and making connections within and beyond the field of mathematics. 10.5.1 Collect, organize, interpret data, and predict 1-9, 2-5, RM2, 13-1, outcomes using the mean, mode, median, range, 13-3, 13-4, 13-5, and standard deviation. (AM1.13, AGP.5) 13-5F 10.5.2 Find the probability of conditional events and 14-3, 14-4 10-1 2-6, 2-6F 1-2F, 11-5, 13-2, 13-3 13-3, 13-5, PS 3-4, 10-8, PS1 mutually exclusive events. (AGP.6) 10.5.3 Predict the outcomes of simple events using the rules of probability. (AM1.16) 10.5.4 Read, interpret and construct graphs to solve problems. (AGP.4) WESTEST, Grade 10 vii Formulas Triangle Rectangular Prism h A 1–2bh h V wh w b Rectangle Pythagorean theorem w A w c b a2 b2 c 2 a Parallelogram h A bh (n 2) n 180 Measure of each interior angle of regular polygon (n number of sides) b Circle r A r 2 180 distance rate time V 4–3 r 3 180 y mx b V r 3h y x2 x1 2 1 r Slope-intercept form of linear equation Cylinder r h viii WESTEST, Grade 10 y y Slope of line Copyright © Glencoe/McGraw-Hill Sphere Name Date Diagnostic Test Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 1 Jeremy is a salesman at an audio-video store. He is paid a base salary of $220 a week, plus a commission of 4% on the items he sells. If he earned a total of $650 one week, what was the total value of the items he sold that week? 10.2.1 A $1,720 B $8,600 C $10,750 D $16,250 1 C 2 The figure shows the running track at Gita’s high school. The ends of the track are semicircles. To the nearest meter, how much distance will Gita cover if she jogs 10 laps around the outer edge of this track? 10.4.4 2 B 3 C 4 Which gives the number 0.00004056 in scientific notation? 10.1.2 A 40.56 106 B 4.056 105 C 4.056 104 D 4.056 105 4 B 5 The table gives West Virginia population data from the 2000 U.S. Census. In 2000, which was closest to the probability that a resident of West Virginia lived in Huntington or Parkersburg? 10.5.2 5 C 5m 48 m A 1,160 m C 1,745 m B 1,274 m D 4,800 m 3 Which is closest to the height of this ramp? 10.3.5 12.3 m 11.9 m Copyright © Glencoe/McGraw-Hill A 9.7 m C 3.1 m B 6.9 m D 0.4 m West Virginia Huntington Morgantown Parkersburg 1,808,344 51,475 26,809 33,099 A 1.8% C 4.7% B 2.8% D 6.2% Go on WESTEST, Grade 10 1 Name Diagnostic Test Date (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 6 D 7 D 8 B 9 The circle graph shows how Adam and Lauren Strauss have budgeted Housing Food their after-tax income. If their 35% 20% monthly after-tax income is Entertainment $2,450, how much is their monthly 5% 10% Other Clothing budget for transportation and 25% Transportation entertainment? 10.5.4 A $122.50 B $245.00 C $367.50 D $612.50 9 C 10 Which polynomial represents the product (z 2)(z2 2z 4)? 10.2.9 A z3 4z2 4z 8 B z3 8 C z3 2z2 4z D z3 8 10 B 6 Lines p and q are parallel. Which is a pair of corresponding angles? 10.3.2 A 4 and 8 B 2 and 6 C 3 and 5 D 5 and 7 2 3 4 6 5 1 7 8 p 7 Marla and Jesse are raking the leaves in their grandmother’s backyard. It would take Marla 221 hours to do this job working alone, while it would take Jesse 3 hours working alone. If x represents the number of hours it will take them to rake the leaves working together, which equation can be solved to find x? 10.2.3 2.5 3 A x 2 B 1 x 13 21.5 1 C x 2.5 3 D 1 x 13 21.5 8 The figure shows the shape of the Schneider family’s dining room. If mB 142°, what is mD? 10.4.1 A 152° B 128° C 120° D 76° 2 WESTEST, Grade 10 C D B E A F Go on Copyright © Glencoe/McGraw-Hill q Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 11 What is the value of 5(8) (4)2 ? 10.1.1 2 3 6 24 A 14 B 6 C 172 D 6 12 Sam is planning to replace the floor molding in the living room of his house. The figure shows the dimensions of this room and the size of the opening to the kitchen, where there is no wall. How many feet of molding will Sam need to purchase if the home improvement store where he buys it will only sell him a whole number of feet? 10.4.4 11 D 12 B 13 C 14 D 15 A 7 ft 6 in. 10 ft 2 in. 18 ft 10 in. A 47 ft C 58 ft B 51 ft D 192 ft 13 The table shows the monthly rents that Sean and Mary Simonds paid for the first four years they rented their apartment. If Sean and Mary keep this apartment and this pattern of annual rent increases continues, what will be the total amount of rent they will pay during the 6th year? 10.2.6 Year 1 2 3 4 Monthly Rent $475 $505 $535 $565 Copyright © Glencoe/McGraw-Hill A $3,300 C $7,500 B $6,250 D $7,860 14 What is the value of 5x3y0z2 if x 2, y 5, and z 4? 10.2.4 A 640 B 52 C 0 D 5 2 15 Which term describes a chord that passes through the center of a circle? A diameter 10.3.4 B radius C secant D tangent Go on WESTEST, Grade 10 3 Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 16 D 17 A 18 C 19 Kara drove from Huntington to Wheeling, a distance of 230 miles, on 9.2 gallons of gas. If she gets the same gas mileage, how much gas will she need to drive from Wheeling to Charleston, a distance of 179 miles? (Round your answer to the nearest tenth of a gallon.) 10.1.1 A 6.9 gal B 7.2 gal C 8.5 gal D 11.8 gal 19 B 20 Which statement is true? 10.3.3 A All squares are similar. C All hexagons are similar. 20 A 21 B 16 Which graph shows all solutions of | 5 2x | 3? 10.2.5 A 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 B C D 17 The table shows Mark’s test scores in his English and biology classes. Which statement is true about these scores? 10.5.1 English Biology 78 86 84 91 67 75 89 82 75 74 A In English, his mean score is higher than his median score, but in biology, his median score is higher than his mean score. B His median score is the same in both classes. C His median score is higher in English than in biology. D The range of his scores is higher in biology than in English. 27 ? 10.1.3 18 Which is equivalent to 300 B 37 D 273 B All rectangles are similar. D All parallelograms are similar. 21 Which is a factor of 6x2 x 35? 10.2.9 A 3x 5 B 3x 7 C 2x 5 D 3x 7 4 WESTEST, Grade 10 Go on Copyright © Glencoe/McGraw-Hill A 310 27 C 73 Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 22 A 23 D 24 Which is an equation of the line with slope 13 and passing through the point at (4, 5)? 10.2.8 A x 3y 11 B 3x y 7 C 3x y 17 D x 3y 19 24 D 25 Justin cut the rope into 3 equal pieces. What is the length of each piece? 25 C 26 B 22 Jonathan is a student who works part-time as a cashier in a coffee shop. He earns $5.75 an hour, but the number of hours he works varies from week to week. The table shows the number of hours he worked each week over a four-week period. How many hours would Jonathan need to work during the following week in order to earn an average (mean) of $115 per week for 5 weeks? 10.5.1 Week Hours Worked 1 20 2 19 3 22 4 25 A 14 C 20 B 17 D 21.5 23 Which graph shows the solution set of 6 2x 4(x 3)? 10.2.1 A 5 4 3 2 1 0 1 2 3 4 5 B 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 C D Copyright © Glencoe/McGraw-Hill 5 4 3 2 1 0 1 2 3 4 5 10.4.2 14 ft 3 in. A 4 ft 1 in. C 4 ft 9 in. 26 What is the value of y? 10.3.2 A 70 B 65 C 50 D 25 B 4 ft 8 in. D 4 ft 10 in. x y (3x 10) Go on WESTEST, Grade 10 5 Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 27 Which could be the slope of this line? 10.2.7 y 27 D 28 C 29 A 30 C A 53 B 35 C 3 5 D 5 3 O x 28 To the nearest square inch, how much gift wrap will Tiffany need to wrap a gift that is placed in this container? (Assume that each surface is covered with no overlap.) 10.4.4 8 in. 15 in. 377 in2 427 in2 478 in2 1,156 in2 29 How many dots are needed to draw the 12th figure in this pattern? 10.2.6 Figure 1 A B C D Figure 2 Figure 3 Figure 4 45 49 144 145 30 Anna and Jim went hiking at Cedar Creek State Park. From the parking lot, they hiked 2.6 miles west and 1.8 miles north, and then stopped at a picnic table for lunch. What is the shortest distance between the picnic table and the parking lot? (Round your answer to the nearest tenth of a mile.) 10.3.5 A 4.4 mi B 3.5 mi C 3.2 mi D 2.1 mi 6 WESTEST, Grade 10 Go on Copyright © Glencoe/McGraw-Hill A B C D Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 31 A 32 A 33 When Saturn is farthest from the Sun, its distance from the Sun is about 936.2 million miles. Which of the following gives the approximate distance from Saturn to the Sun and back to Saturn? 10.1.2 A 1.8724 109 mi B 4.681 108 mi C 9.362 108 mi D 18.724 1016 mi 33 A 34 Haley, who is a 4th grader, is standing in her school playground on a sunny day. She is 4 feet 3 inches tall, and her shadow is 2 feet 6 inches long. If the shadow of the school flagpole at the same time is 30 feet 6 inches, which is closest to the height of the flagpole? 10.3.3 34 B 31 The formula P 2L 2W can be used to find the perimeter of a rectangle with length L and width W. How can this formula be rewritten to find the length of a rectangle if its perimeter and width are known? 10.2.2 P 2W A L 2 B L P2 2W C L 12 (P W) D L 2P 2W W L 32 A sporting goods store sells exercise balls in two sizes, large and jumbo. Which is closest to the amount of air needed to fully inflate one ball of each size for a store display? 10.4.4 20 in. 24 in. Copyright © Glencoe/McGraw-Hill A 11,400 in3 C 6,400 in3 B 8,600 in3 D 4,100 in3 h 4 ft 3 in. 2 ft 6 in. 30 ft 6 in. Go on A 18 ft B 52 ft C 55 ft D 76 ft WESTEST, Grade 10 7 Name Date Diagnostic Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 35 If the probability that it will rain tomorrow is 0.32, what is the probability that it will not rain? 10.5.3 A 0.18 B 0.32 C 0.64 D 0.68 35 D 36 What is the slope of the line 7x 4y 28? 10.2.7 36 A 37 C 38 Which could be the lengths of the three sides of a triangle? 10.4.1 A 1 cm, 3 cm, 5 cm B 12 cm, 12 cm, 24 cm C 15 cm, 17 cm, 32 cm D 16 cm, 25 cm, 32 cm 38 D 39 Andrea wants to rent a car for a holiday weekend in order to visit her friend Kaitlyn in Martinsburg. Andrea can rent the car she wants from Rent-for-Less for $20 a day plus 24 cents a mile, or she can rent the same car from Car-4-U for $35 a day with unlimited mileage. If she plans to rent the car for 3 days, how many miles will she need to drive for Car-4-U to be the better deal? 10.2.3 A at least 188 mi B at most 187 mi C at least 64 mi D at most 63 mi 39 A 40 B A 74 B 47 C 4 7 D 7 4 10.5.4 60 50 40 30 20 10 0 42 35 28 18 21–30 31–40 41–50 Age A 21–30 C 41–50 51–60 B 31–40 D 51–60 40 In circle O, if mSOT 71° and mRQ 57°, what is mRUQ? 10.3.4 A 57° B 64° C 71° D 85° 8 WESTEST, Grade 10 S R Q U O T Go on Copyright © Glencoe/McGraw-Hill Number of Employees 37 The histogram shows the age distribution of the employees of a small company. Which interval contains the median age of the employees? Name Date Diagnostic Test (continued) Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. 41 The diameter of a red blood cell is 0.0000084 meter. Write a paragraph to explain how to rewrite this number in scientific notation. Then use the process that you have described to write the number in scientific notation. 10.1.2 Sample answer: In scientific notation, the decimal place goes directly to the right of the first nonzero digit, which is 8. I need to move the decimal point 6 places to the right. Because I have to move the decimal point to the right, the exponent will be negative, and because I need to move it 6 places, I know that the exponent on the 10 will be 6. 0.0000084 8.4 106 42 Find the radius of circle O. Explain your reasoning. 10.3.4, 10.3.5 A 12 cm B Sample answer: B is an inscribed angle 5 cm in the circle. I know it is a right angle O C because it intercepts a semicircle, so its 1 measure is 2 (180°) 90°. Since B is a right angle, ABC is a right triangle with hypotenuse AC . By the Pythagorean Theorem (or using a Pythagorean triple), 122 52 169 13 cm. Since AC is a diameter and OA is a radius, AC 1 1 13 OA 2 (AC) 2 (13) 2 or 6.5 cm. The radius of circle O is 6.5 cm. Copyright © Glencoe/McGraw-Hill 43 What is the volume of this cylindrical drum? (Round your answer to the nearest tenth of a cubic foot.) 10.4.2, 10.4.4 32 in. 21–2 yd Sample answer: All of the measurements need to be in the same units, and I need to give my answer in cubic feet, so I changed the given measurements to feet. The diameter of the cylinder is 32 in., so the radius is 4 1 5 15 16 in. 1 ft 4 in. 3 ft. The height of the cylinder is 22 yd 2 (3 ft) 2 ft. The formula for the volume of a cylinder is V r2h, so the 4 2 15 Go on volume of the drum is 3 2 41.9 ft3. WESTEST, Grade 10 9 Name Date Diagnostic Test (continued) Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. 44 The formula F 95C 32 can be used to convert temperatures from degrees Celsius to degrees Fahrenheit. 10.2.2 Part A Solve this equation for C to obtain a formula that can be used to convert temperatures from degrees Fahrenheit to degrees Celsius. Sample answer: 9 F 5C 32 9 F 32 5C 5(F 32) 9C 5(F 32) 9 C or C 5(F 32) 9 Part B The highest temperature ever recorded in West Virginia was 112°F on July 10, 1935 in Martinsburg. Use your formula from Part A to convert this temperature to degrees Celsius. Sample answer: C 5(F 32) 9 5(112 32) 9 44 The record high temperature is about 44°C. 45 The stem-and-leaf plot shows the scores for the students in Mr. Roland’s 1st period biology class on a 100-point chapter test. 10.5.1, 10.5.3, 10.5.4 Part A For these data, place these measures of central tendency in increasing order: the median, the mode, and the mean. Stem 3 4 5 6 7 8 9 10 | Leaf |2 |14 |578 |122 |023 |235 |358 |0 5 8 6 9 9 5 5 7 9 Copyright © Glencoe/McGraw-Hill Sample answer: There are 28 scores, so 3 | 2 32 the median is the average of the 14th 73 76 and 15th scores, which is 2 74.5. The mode is the most common score, which is 85, the only score that appears 3 times. To find the mean, I added up all the 2,046 scores and divided by 28: 28 73.1. In increasing order, the measures are mean, median, mode. Part B What is the probability that a randomly chosen student who took this test got an A or B (a score of 80 or above) on the test? Sample answer: There are 11 scores of 80 or above, so the 11 probability is 28 0.393. STOP 10 WESTEST, Grade 10 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.1.1 Solve computational and practical problems using properties of numbers, order of operation, computation, and estimation with decimals, fractions, integers, and mixed numbers, including ratio, proportion, and percents. D 2 Megan bought 3 CDs for $11.99 each and 4 DVDs for $14.99 each. How much did she spend, not including sales tax? A $118.86 B $95.93 C $94.43 D $92.93 2 B 3 Dylan and Rachel Lincoln are spending a long weekend at Kanawha State Forest, which is near Charleston. They want to hike a total of 30 miles on Saturday, Sunday, and Monday. If they hike 814 miles on Saturday and 1112 miles on Sunday, how far will they have to hike on Monday to make their goal? 3 A 4 Pedro drove from Beckley to Parkersburg, a distance of 135 miles, on 5.8 gallons of gas. If he gets the same gas mileage, how much gas will he need to drive from Wheeling to White Sulphur Springs, a distance of 260 miles? (Round your answer to the nearest tenth of a gallon.) A 9.6 gal B 10.6 gal C 11.2 gal D 22.3 gal 4 C 5 Kayla bought a dress from a rack that displayed the sign at the right. If the original price of the dress she chose was $65, how much did Kayla pay for it, not including sales tax? A $52.00 B $46.80 C $45.50 D $35.00 5 B A 2 C Copyright © Glencoe/McGraw-Hill (8)(4) (2)4 ? 5 4 7 32 1 1 What is the value of 2 3 B 48 131 D 2 A 1014 mi B 11 mi C 1434 mi D 1934 mi SPRING CLEARANCE Price on tag is 20% off original price. Additional 10% markdown taken at register. WESTEST, Grade 10 11 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.1.2 Write numbers involving scientific notation and combine numbers written in scientific notation to solve practical problems. 1 The population of West Virginia in 2000, according to the U.S. Census, was about 1,808,000. Which gives this number in scientific notation? B 18.08 105 A 1,808 103 C 1.808 106 D 1.808 107 1 C 2 Which is another way to write the number 3.45 106 ? A 3,450,000 B 0.000345 C 0.00000345 D 0.000000345 2 C 3 When Jupiter is closest to the Sun, its distance from the Sun is about 460.3 million miles. Which of the following gives the approximate distance from Jupiter to the Sun and back to Jupiter when Jupiter is closest to the Sun? B 9.206 108 mi A 4.603 106 mi C 9.206 109 mi D 4.603 1012 mi 3 B 4 D 5 The speed of light is approximately 1.86 105 miles per second, and the average distance between Earth and the Sun is approximately 9.3 107 miles. About how long does it take sunlight to reach Earth? A 8 hr 20 min B 1 hr 23 min 20 sec C 8 min 20 sec D 18 sec 5 C 6 The diameter of a single human hair is approximately 2.0 105 meter. What is the approximate width of 5,000 hairs if they are placed side by side with no space between them? A 1m B 10 cm C 1 cm D 1 mm 6 B A 1.6 109 12 C 1.6 10 5 12 WESTEST, Grade 10 (4.0 106)(1.2 102) ? 3.0 105 B 1.6 101 D 1.6 109 Copyright © Glencoe/McGraw-Hill 4 Which is equivalent to Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.1.3 Estimate and simplify square roots into both exact and approximate forms. 1 B 2 If 52 is placed on a number line, between which two points will it be located? A 8 and 7 B 7 and 6 C 6 and 5 D 5 and 4 2 A 3 Which is closest to the length of the diagonal of this square? A 11 cm B 12 cm C 14 cm D 20 cm 3 C 4 D 5 C 6 B 7 B ? 1 Which is closest to 130 A 11.3 C 12.0 B 11.4 D 13.0 10 2 cm 10 cm 10 cm ? 4 Which is equivalent to 98 A 27 C 9.8 B 7.2 D 72 5 Which is equivalent to 125 45 ? A 55 53 B 52 D 80 Copyright © Glencoe/McGraw-Hill C 25 6 Which is the reciprocal of 5? A 5 5 5 C 25 7 What is another way to write 5 B 5 D 5 2 ? 5 3 A 2(5 3 ) B 5 3 C 5 3 5 3 D 2 WESTEST, Grade 10 13 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.1.1, 10.1.2, 10.1.3 1 A cookie recipe calls for 221 cups of flour to make 4 dozen cookies. For her sister’s graduation party, Elizabeth wants to make 14 dozen cookies from this recipe. How much flour will she need? 10.1.1 Sample answer: I wrote a proportion and solved it using cross multiplication to get the answer. Let x the number of cups of flour needed to make 14 dozen cookies. 1 22 cups 4 dozen x cups 14 dozen 1 22 x 14 4 1 22 (14) 4x 35 4x x 35 4 3 84 3 She will need 84 cups of flour. 2 The land area of West Virginia is 15,410,560 acres. Write a paragraph to explain how to rewrite this number in scientific notation. Then use the process that you have described to express this area in scientific notation. Sample answer: The way the number is written now, the decimal point is at the end of the number, even though it isn’t shown. Place a decimal point there. In scientific notation, the decimal point has to go right after the first nonzero digit, so mark the spot between the 1 and the 5 at the beginning of the number. Move the decimal point to this spot and count how many places you moved it. Count that you moved it 7 places to the left. This gives the exponent on the 10. 15,410,560 15,410,560. 1.541056 107 3 Write 98 200 288 as a single term involving a simplified radical. Show each step of your work. 10.1.3 Sample answer: 98 200 288 49 2 100 2 144 2 72 102 122 (7 10 12)2 52 14 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill 10.1.2 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.1.1, 10.1.2, 10.1.3 (continued) 4 An electronics store marks up all laptop computers 20% above cost. The cost to the store for one of their most popular models is $1,450. 10.1.1 Part A Mark works at this store and is responsible for putting price tags on all the items before they go on the floor. What price should he put on the tag for this computer? Explain in words how you figured this out and show all your work. Sample answer: Marking up a price by 20% means adding 20% of the cost of the item to its cost. The markup is 20% of $1,450 0.20($1,450) $290. The price should be $1,450 $290 $1,740. Part B Store records showed that this computer wasn’t selling well, so the manager decided to put it on sale at 20% off. What will be the sale price? Explain in words how you figured this out and show all your work. Sample answer: Reducing or marking down a price by 20% means subtracting 20% of the price of the item from its price. The markdown is 20% of $1,740 0.20($1,740) $348. The sale price should be $1,740 $348 $1,392. Copyright © Glencoe/McGraw-Hill 5 The diameter of Mercury at its equator is about 4.8794 million meters, while the diameter of Jupiter at its equator is about 142.98 million meters. 10.1.1, 10.1.2 Part A Write each of these numbers in scientific notation. Explain your reasoning. Sample answer: One million 1,000,000 106, so 4.8794 million 4.8794 106. 142.98 million 142.98 106 1.4298 108. Part B Find the ratio of the diameter of Jupiter at its equator to the diameter of Mercury. About how many times as great is the diameter of Jupiter as the diameter of Mercury? Show all your calculations. Sample answer: 1.4298 108 4.8794 106 1.4298 4.8794 108 6 0.293 102 29.3. The diameter of Jupiter is about 29.3 times the diameter of Mercury. WESTEST, Grade 10 15 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.1 Define variables and solve multi-step linear equations and one-variable inequalities, interpret results on a number line, and apply the skills toward solving practical problems. 1 If Heather has x quarters, y dimes, z nickels, and w pennies in her wallet, how much money does she have in dollars? A 0.25x 0.10y 0.05z 0.01w B 25x 10y 5z w C xyzw D 0.025x 0.010y 0.005z 0.001w 1 A 2 Which equation has no solution? A x55x C 5x 2 8x 2 2 D 3 C 4 B 5 A 6 D B 3(x 7) 3x 21 D 4(x 5) 2x (6x 12) 3 What are all solutions of 32x 56 x 13? A 12 B 12, 12 C 1 2 D 2 4 Rita has 15 coins, of which q are quarters and the rest are nickels. How many nickels does she have? A q 15 B 15 q C 15 q D 15 q 5 Which graph shows the solution of 5 3x 14? A 5 4 3 2 1 0 1 2 3 4 5 B 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 D 5 4 3 2 1 0 1 2 3 4 5 6 Nicole is trying to choose a long-distance telephone plan. She is considering two plans: Plan M has a monthly fee of $3.95 and charges 6 cents per minute. Plan R charges a flat rate of $25.95 a month for unlimited long-distance calling. How many minutes of long-distance calls would Nicole need to make during an average month for Plan R to be the better choice? A under 498 min B over 498 min C under 366 min D over 366 min 16 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill C Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.2 Solve literal equations for a given variable and apply the skills toward solving practical problems. 1 A 2 B 3 The formula A P Prt gives the amount A, in dollars, in a savings account paying simple interest if a principal of P dollars is invested at an annual interest rate of r% for t years. How much must Amanda deposit in an account paying 4% simple interest in order to have $900 in her account five years after she opens it? (Assume that she makes no further deposits and withdrawals during the five-year period.) A $865.39 B $820 C $750 D $720 3 C 4 In physics, the formula 1f p1 q1 describes the relationship between the focal length of a lens f, the distance between the object and the lens p, and the distance between the lens and the image q. How can Luis rewrite this formula to give the distance between the lens and its image if he knows the focal length of the lens and the distance between the object and the lens? A qfp B q fp p 4 D 1 The formula A 12 (B b)h can be used to find the area of a trapezoid with bases of lengths B and b and height h. How can this formula be rewritten to give B in terms of A, b, and h? b h B 2A bh A B h 2A b B B h Ab C B 2h D B 12(A b)h Copyright © Glencoe/McGraw-Hill 2 Sean is planning to drive from his home in Huntington to Kevin’s house in Wheeling, a distance d of 230 miles. In order to tell Kevin when to expect him, Sean wants to estimate the time t it will take him to get to Wheeling. He knows the formula d rt. How should he rewrite this formula so that he can use it to find how long the trip will take him at a specific average speed? A t rd B t dr C t dr D tdr pf C q fp fp D q pf WESTEST, Grade 10 17 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 1 Brandon is 8 years less than twice the age of his brother Steven. The sum of their ages is 28. If s represents Steven’s age, which equation describes this situation? A (2s 8) s 28 B (2s 8) s 28 C 2s 8 28 D (2s 8) s 28 1 D 2 Kelsey and Allison went shopping together at the Martinsburg Mall. They decided to buy some clothes at a store that was running a special sale in which all shorts were sold at one price and all jeans at another price. Kelsey spent $92 for 2 pairs of jeans and 3 pairs of shorts, while Allison spent $81 for 1 pair of jeans and 4 pairs of shorts. What was the sale price for a pair of shorts? (Ignore sales tax.) A $14 B $15.75 C $19.50 D $25 2 A 3 In a chemistry lab, Letisha must mix 10 liters of 15% alcohol solution with a 25% alcohol solution to get an 18% solution. If x represents the amount of 25% solution she will need, which equation can she solve to find this amount? A 0.15x 0.25(10) 0.18(10 x) B 0.15(10) 0.18x 0.25(10 x) C 0.15(10) 0.25x 0.18(10 x) D x 0.15(10) 0.25x 3 C 4 Craig received scores of 72, 85, and 79 on his first 3 biology tests. To figure out what score he would need on his 4th test to give him a test average of at least 80, he wrote the inequality 4 D 72 85 79 x 4 80. (1) Then, as his first step in solving this inequality, he wrote 72 85 79 x 320. (2) What property of real numbers did he use to get from inequality (1) to inequality (2)? A Addition Property of Inequality B Associative Property of Multiplication C Distributive Property D Multiplication Property of Inequality 18 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill OBJECTIVE 10.2.3 Solve practical problems using a four-step problem solving approach, justifying steps based on the properties of real numbers. Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.4 Evaluate and simplify algebraic expressions using grouping symbols, order of operations, properties of real numbers, and laws of exponents. 1 What is the value of 3(x y) 5(x y) if x 12 and y 8? A 88 B 40 C 40 D 88 1 A 2 What is the value of 5mn 2m2p 3p3 if m 4, n 3, and p 2? A 100 B 20 C 20 D 28 2 B 3 Which property of real numbers allows you to rewrite 5(a2 2ab b2) as 5a2 10ab 5b2? A Associative Property of Addition B Associative Property of Multiplication C Commutative Property of Multiplication D Distributive Property 3 D 4 Which expression is equivalent to (4x2y)3? A 64x6y3 B 64x5y4 C 12x6y3 D 64x5y3 4 A 5 What is the value of 3p0r3s2 if p 5, r 2, and s 3? 5 B 6 D 7 B A 135 8 Copyright © Glencoe/McGraw-Hill C 216 6 What is another way to write 27 8 B D 1,080 2x2y3 4 3x y 5 2 A 2x12y 20 3 B C 81 1 16x 2y20 D ? 16y 20 81x12 81x12y 20 16 7 Which expression is equivalent to (3r 2s4)(2r 3s4)2? A 12r 5 C 6r 8 s4 B 12r 8 s4 D 12r 8s4 WESTEST, Grade 10 19 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.5 Solve absolute value equations in one variable and interpret the results on a number line. 1 What are all solutions of | 2x 5 | 7? A 6 and 1 B 1 and 6 C 6 D No solutions 1 B 2 What are all numbers on the real number line that are 5 units from 3.5? A 8.5 and 1.5 B 8 and 2 C 6 and 1 D 1.5 2 A 3 Which graph shows all solutions of | 5 x | 3? A 3 D 4 D 5 B 6 C 7 D 10 8 6 4 2 0 2 4 6 8 10 10 8 6 4 2 0 2 4 6 8 10 10 8 6 4 2 0 2 4 6 8 10 10 8 6 4 2 0 2 4 6 8 10 B C D 4 Which equation has exactly one solution? A | 3x 5 | 5 B | 3x 5 | 5 C | 3x 5 | 0.1 D | 3x 5 | 0 A 7 6 B 1 C 1 9 7 D 36 6 For which of the following equations does the graph show all solutions? 5 4 3 2 1 0 1 2 3 4 5 A | 2x 7 | 3 C | 2x 7 | 3 7 Which equation has no solutions? A |5 4x| 6 C |5 4x| 0 20 WESTEST, Grade 10 B | 2x 7 | 3 D | x 3.5 | 1 B |5 4x| 0.6 D |5 4x| 6 Copyright © Glencoe/McGraw-Hill 5 What is the sum of the solutions of x 12 23? Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.6 Analyze a given set of data for the existence of a pattern numerically, algebraically, and graphically. 1 C 2 What is the 8th term of the sequence 1, 2, 4, 8, …? A 128 B 64 C 64 D 128 2 A 3 The table shows the number of bacteria in a colony at several times on one day. If the population of the colony continues to follow this pattern of growth, which is the best estimate of the number of bacteria at 8:00 P.M. on the same day? 3 D 4 B 1 The table shows Trevor’s hourly wage during his first four years on his job. If Trevor stays on this job and this pattern of annual raises continues, what will be his hourly wage during his 7th year on the job? Year 1 Hourly Wage $5.50 2 $5.80 3 $6.10 A $7.60 C $7.30 4 $6.40 B $7.40 D $7.00 Copyright © Glencoe/McGraw-Hill Time Number of Bacteria 8:00 A.M. 0,500 10:00 A.M. 0,750 Noon 1,125 2:00 P.M. 1,688 A B C D 2,400 2,900 3,800 5,700 4 How many dots are needed to draw the 10th figure in the pattern? Figure 1 A B C D Figure 2 Figure 3 110 55 45 21 WESTEST, Grade 10 21 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.7 Determine the slope of a line given an equation of a line, the graph of a line, or two points. 1 What is the slope of the line y 4x 5? A 5 B 4 C 4 8 3 B 5 3 C 3 5 2 C 3 A 4 B 5 D 6 A 7 C D 85 3 Which of the following is an equation of a line with slope 27? A 2x 7y 14 C 2x 7y 14 B D 5 2 What is the slope of the line 3x 5y 8? A 1 B 7x 2y 14 D 7x 2y 14 4 Which is the slope of this line? A 2 y B 12 C O 1 2 x D 2 5 What is the slope of the line that contains the points at (4, 6) and (6, 2)? 5 4 B C 12 1 2 D 45 6 The points shown in this table lie on a line. What is the slope of this line? x y 4 2 20 15 6 5 A 2.5 C 0.4 7 Which line has slope 0? A xy C y0 22 WESTEST, Grade 10 B 0.4 D 2.5 B xy0 D x0 Copyright © Glencoe/McGraw-Hill A Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.8 Write and graph linear equations. 1 Which is an equation of the line that passes through the points at (4, 0) and (0, 3)? A 3x 4y 12 B 3x 4y 12 C 4x 3y 12 D 4x 3y 12 1 B 2 Which is an equation of the line with slope 35 and passing through the point at (2, 6)? A 3x 5y 24 B 5x 3y 8 C 5x 3y 28 D 3x 5y 36 2 D 3 Which is an equation of the horizontal line through the point at (4, 7)? A x4 B x y 3 C y 7 D x y 11 3 C 4 Which could be an equation for this graph? 4 A 5 D 6 A y A y 32 x 32 B y 23 x 23 x O C y 23 x 23 D y 32 x 32 Copyright © Glencoe/McGraw-Hill 5 Which could be the graph of the equation y 2x 3? A B y y x O C D y x O y x O O x 6 Which is an equation of the vertical line through the point at (6, 3)? A x60 B x60 C y30 D xy3 WESTEST, Grade 10 23 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.2.9 Factor and perform basic operations on simple polynomials. 1 D 2 Which is the completely factored form of 2x2 18x 36? A (2x 6)(x 6) B (2x 12)(x 3) C 2(x 9)(x 2) D 2(x 3)(x 6) 2 D 3 Which is the completely factored form of 9x2 30xy 25y 2? A (3x 5y)2 B (3x 5y)2 C (3x 5y)(3x 5y) D (3x)2 (5y)2 3 A 4 Which expression gives the difference (8y2 5y 6) (8y2 5y 9)? A 16y2 10y 15 B 15 C 10y 15 D 10y 3 4 C 5 Which expression is equivalent to 3(2z2 4z 5) 2(4z2 z 1)? A 14z2 10z 13 B 2z2 10z 13 2 C 2z 10z 13 D 2z2 10z 13 5 D 6 Which polynomial represents the product (3r 2s) (9r 5s)? A 27r 2 3rs 10s2 B 27r 2 3rs 10s2 C 27r 2 33rs 10s2 D 27r 2 3rs 10s2 6 A 7 What is the quotient when 6x2 11x 4 is divided by 2x 1? A 3x 4 B 3x 4 C 3x 2 D 4x2 10x 5 7 A 8 C 8 What is another way to write A B C D 24 3x2 5xy 10x2 20xy 3x2 5xy 1 3x2 5xy 1 WESTEST, Grade 10 B x8 D x1 15x3y 25x2y 2 5xy ? 5xy Copyright © Glencoe/McGraw-Hill 1 Which is a factor of x2 7x 8? A x4 C x2 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.2.1, 10.2.2, 10.2.3, 10.2.5, 10.2.6, 10.2.8 1 Chris bought a jacket on sale for $48. The jacket was on sale at 25% off the original price. What was the original price of the jacket? Write an equation that you can use to solve this problem and show all your work to solve it. 10.2.1, 10.2.3 Sample answer: Let x the original price of the jacket. 25% is 0.25, so the sale price can be written as x 0.25x, so my equation is x 0.25x 48. x 0.25x 48 0.75x 48 x 48 0.75 x 64 The original price of the jacket was $64. 2 Solve | 2x 1 | | 4x 3 |. Show all your steps. Explain properties you use that are special properties of absolute value. 10.2.5 Sample answer: | 2x 1 | | 4x 3 |, so 2x 1 4x 3 or 2x 1 (4x 3) 4x 3 because 2 numbers or expressions can only have the same absolute value if they are equal or opposites. This gave me 2 linear equations that I solved to get the two solutions of the equation. 2x 1 4x 3 2x 1 4x 3 2x 4 6x 2 1 x 2 x Copyright © Glencoe/McGraw-Hill 3 3 Graph 2x y 4. Explain how you chose the points you used to draw the line. Identify the points you used on the graph. 10.2.8 y O x Sample answer: I used the x- and y-intercepts. If x 0, the equation becomes y 4, or y 4, so the line goes through the point (0, 4). If y 0, the equation becomes 2x 4, or x 2, so the line goes through the point (2, 0). As a check, I found a third point (3, 2). I plotted these 3 points and drew the line through them. WESTEST, Grade 10 25 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.2.1, 10.2.2, 10.2.3, 10.2.5, 10.2.6, 10.2.8 (continued) 4 The relationship between the time it takes two people to complete a job working together and the times it takes them to do the same job working separately can be described by the equation 1t 1a 1b, where t is the time required working together, and a and b are the times required working separately. 10.2.2 Part A Solve this equation for t in terms of a and b. Show all your steps. 1 t 1 t Sample answer: tab 1 a 1 b 1 1 a b tab ab tb ta ab t(b a) t ab ba Part B Ryan and Tyler are students at West Liberty State College. Their dorm room needs cleaning. If Ryan can clean the room in 3 hours working alone, and Tyler can clean the room in 4 hours working alone, how long will it take them to clean the room working together? Use your equation from Part A to solve this problem. Sample answer: t t h 5 7 4 60 min 43 min Working together, it will take them about 1 h 43 min. 5 The table shows the first 4 terms Term Number 1 2 3 of a certain sequence of numbers. 2 3 4 10.2.6 Term 3 5 7 4 5 9 Part A Explain in words any patterns you see in the terms of the sequence and how each term is related to the term number. Sample answer: To get from one term to the next, add 1 to the numerator and add 2 to the denominator. All the denominators are odd numbers. The numerator is always 1 more than the term number. To get the denominator, double the term number and add 1. Part B Use the patterns you found in Part A to write an expression for the nth term (or general term) of the sequence. Sample answer: The nth term is 26 WESTEST, Grade 10 n1 . 2n 1 Copyright © Glencoe/McGraw-Hill 5 7 ab ; a 3, b ba 12 5 34 1 7 7 34 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.3.2 Identify angle relationships and apply in solving problems (complementary, supplementary, vertical, and adjacent, as well as relationships formed by parallel lines cut by a transversal). 1 What is the measure of the complement of a 39° angle? A 141° B 129° C 61° D 51° 1 D 2 Which angles in the figure are both adjacent and supplementary? A 2 and 3 B 2, 3, and 4 C 1 and 2, 1 and 5 D 4 and 5 2 C 3 C 4 A 5 B 1 2 Copyright © Glencoe/McGraw-Hill 3 What is the value of y? A 45 B 120 C 135 D 150 4 Lines s and t are parallel. Which is a pair of alternate exterior angles? A 2 and 8 B 1 and 8 C 3 and 5 D 4 and 7 5 If line m is parallel to line n , what is the value of x? A 135 B 125 C 55 D 35 x 1 2 4 5 4 3 3x y s 3 5 6 8 t 7 55 x m n WESTEST, Grade 10 27 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.3.3 Investigate similar figures and apply proportions in problem solving situations. 1 C 2 Which statement is true? A All right triangles are similar. B All isosceles triangles are similar. C All obtuse triangles are similar D All equilateral triangles are similar. 2 D 3 Kristi is using a road map of West Virginia on which 1 inch represents 20 miles. She measures a straight-line distance of 734 inches between Charleston and Morgantown. Which is closest to the actual distance between these cities? A 140 mi B 145 mi C 155 mi D 160 mi 3 C 4 Kyle is standing in a park on a sunny day. He is 5 feet 6 inches tall, and his shadow is 3 feet 3 inches long. If a nearby tree has a shadow that is 10 feet 9 inches long at the same time, which is closest to the height of the tree? 5 ft 6 in. A 18 ft 2 in. 3 ft 3 in. B 17 ft 10 in. C 13 ft 0 in. D 6 ft 4 in. 4 A 5 B 1 In the figure, LMN PQR. What are the values of x and y? M Q 14.4 y L 8 N A x 6.4, y 11 C x 9.6, y 9 R 5 6 P B x 9, y 11.4 D x 10, y 8 5 If HJK RST, which of the following must be true? A HJ RS B mK mT C 28 HK RT JRKS WESTEST, Grade 10 D mJ mR Copyright © Glencoe/McGraw-Hill x 10 ft 9 in. Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.3.4 Explore circle relationships, emphasizing the vocabulary of circles. Q in circle O? 1 Which term describes P A chord B diameter C radius D tangent P O A 2 B 3 D 4 D 5 C Q 2 R, S, and T are points on circle O. If mRTU 56°, what is mRST ? R S O T U A 124° C 292° B 248° D 304° 3 Which could be the measure of a minor arc of a circle? A 350° B 200° C 180° D 90° is tangent to circle O at R. If is tangent to circle O at Q, and PR 4 PQ mQSR 215°, what is mP? Copyright © Glencoe/McGraw-Hill 1 Q S O P R A 70° C 40° B 55° D 35° 5 Which term describes a line that intersects a circle in 2 different points? A chord B radius C secant D tangent WESTEST, Grade 10 29 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.3.5 Solve right triangle problems using the Pythagorean Theorem. 1 B 2 C 3 Which could be the lengths of the three sides of a right triangle? A 1 in., 1 in., 2 in. B 8 ft, 9 ft, 10 ft C 12 m, 12 m, 12 m D 10 cm, 26 cm, 24 cm 3 D 4 Miriam and Ed Rose spent Labor Day weekend hiking, swimming, and boating at Blackwater Falls State Park in the Potomac Highlands. On Sunday, they hiked 1.5 miles east, then 1.9 miles south, then 1.2 miles east, and then stopped for a picnic lunch. To the nearest tenth of a mile, what was the distance between their starting point and their picnic spot? A 4.6 mi B 3.3 mi C 3.1 mi D 2.7 mi 4 B 5 The advertised size of a television set is the length of the diagonal of the screen. Natalie bought a 13-inch TV for her dorm room at Fairmont State College. If the screen is 11 inches across, which is closest to its height? A 7 in. B 8 in. C 10 in. D 12 in. 5 A 1 What is the length of the longer leg of this right triangle? 17 cm 8 cm A 17 cm C 12.5 cm B 15 cm D 9 cm 2 Benjamin is using a 10-foot ladder to paint his bedroom. If he places the ladder 5 feet from the base of a wall, about how far up the wall will the ladder reach? 10 ft 5 ft 30 WESTEST, Grade 10 B 7 ft 9 in. D 11 ft 2 in. Copyright © Glencoe/McGraw-Hill A 5 ft C 8 ft 8 in. Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.3.5 (continued) 6 Which is closest to the length of the diagonal of this square? A 12 cm B 10 cm C 8.5 cm D 8.0 cm Forest St. 7 D 8 C 9 A School 50 yd Stone Park Home C 6 cm 7 Danielle can walk from her apartment to her school either by walking north on Forest Street and then east on Linden Street, or by cutting diagonally across Stone Park. Which is closest to the distance she will save by walking through the park? Linden St. 6 100 yd A 150 yd C 50 yd B 119 yd D 38 yd 8 Allison, who lives in Buckhannon, is planning to ship a birthday present to her cousin Maria, who lives in Knoxville, Tennessee. In order to know if the gift she has bought will fit in the carton shown in the figure or if she will need to use a larger one, Allison needs to know the length RS, which is the length of the body diagonal of the carton. Which is closest to this length? Copyright © Glencoe/McGraw-Hill S 6 in. R 8 in. 12 in. A 13.5 in. C 15.6 in. B 14.4 in. D 26.0 in. 9 Which could be the lengths of the sides of an isosceles right triangle? A 5 cm, 52 cm, 5 cm C 1 cm, 2 cm, 3 cm B 5 cm, 5 cm, 5 cm D 5 cm, 5 cm, 53 cm WESTEST, Grade 10 31 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.3.2, 10.3.3, 10.3.4, 10.3.5 1 On a school field trip, Samantha visited West Virginia Independence Hall in Wheeling and bought a postcard of this building. On the postcard, the building is 10 centimeters wide and 8 centimeters tall. She decided to use the postcard to make a scale model of Independence Hall for a class project. If her model is 36 centimeters wide, how tall should it be? (Round your answer to the nearest centimeter.) 10.3.3 Sample answer: Let x the height of the model. 36 cm 10 cm x cm 8 cm 10x 8 36 288 x 28.8 29 Samantha’s model should be about 29 cm tall. 9.2 RU 84 The length of RU is 84 cm, which is approximately 9.2 cm. 3 The ratio of the measures of two supplementary angles is 8:7. What are the measures of these angles? 10.3.2 Sample answer: Let 8x and 7x be the angle measures. Since the measures of supplementary angles add up to 180°, my equation is 8x 7x 180. 8x 7x 180 15x 180 x 180 15 12 8x 8(12) 96, and 7x 7(12) 84, so the angle measures are 96° and 84°. 32 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill S 2 In RST, what is the length of R U , the altitude 10 cm ? (Write your answer in exact radical form to ST R 8 cm U and then as a decimal rounded to the nearest tenth of a centimeter.) 10.3.2, 10.3.5 T Sample answer: Because S T, I know that RST is isosceles with legs R S and R T, and base S T. In an isosceles triangle, the altitude to the base is also the median, so SU UT 4 cm. Since R U ⊥ ST , I can use the Pythagorean Theorem in RSU to find RU. (RU)2 (SU)2 (RS)2 (RU)2 (RS)2 (SU)2 102 42 100 16 84 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.3.2, 10.3.3, 10.3.4, 10.3.5 (continued) and RU are chords of circle O. 10.3.3, 10.3.4 4 SV Part A Complete this similarity statement for the two triangles in the figure. RST ______ Explain how you know that the triangles are similar. S 5 8 R 3 U T x O V Sample answer: RST VUT S U because they are inscribed angles that intercept the same arc. R V for the same reason, so the triangles are similar by the AA Similarity Postulate. (I could also use the vertical angles as one of the angle pairs.) Part B Use the similar triangles to find x. Sample answer: I can write a proportion that involves corresponding sides of the triangles. RT VT 8 x ST UT 5 3 5x 24 24 4 x 5 or 45 is tangent to circle O 5 In the figure, QS at Q and to circle P at S. 10.3.4, 10.3.5 S 6 Copyright © Glencoe/McGraw-Hill O R P Part A Look at OQR and PSR. What 3 4 kind of triangles are these? What Q is the relationship between the two triangles? Explain how you know. Sample answer: They are both right triangles and they are similar. I know they are right triangles because a radius of a circle is perpendicular to a tangent at the point of tangency, which tells me that OQR and PSR are both right angles. Using these right angles and the vertical angles, ORQ and PRS, I know the triangles are similar by the AA Similarity Postulate. Part B Find the distance between the centers of the circles. R and P R are the hypotenuses of the right triangles. I can use Sample answer: O the Pythagorean Theorem or the 3-4-5 Pythagorean triple to find that OR 5. PS 6 2, PR 2(OR) 10, so the Since the triangles are similar, and OQ 3 distance between the centers is OR PR 5 10 15. WESTEST, Grade 10 33 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.4.1 Calculate the missing measures of angles and lengths of sides of a polygon from given data, using formulas. 1 D 2 A 3 What is the measure of each interior angle of a regular pentagon? A 72° B 108° C 120° D 144° 3 B 4 Which could not be the lengths of the 3 sides of a triangle? A 5 cm, 5 cm, 5 cm B 12 cm, 18 cm, 15 cm C 7 cm, 24 cm, 25 cm D 12 cm, 20 cm, 7 cm 4 D 5 Sonja is a student at Mountain State University in Beckley. She hung this college pennant in her dorm room. If m3 38°, what is m1? 5 C 1 What is the measure of the largest angle in this triangle? (3x 16) (x 8) (4x 20) A 72° C 108° B 92° D 112° 2 The figure shows the shape of Melissa’s flower garden. What is the measure of the largest angle? x (4x ) B 135° D 90° 12 in. 1 MSU 2 3 12 in. A 52° C 71° 34 WESTEST, Grade 10 B 62° D 104° Copyright © Glencoe/McGraw-Hill A 144° C 120° Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.4.2 Estimate, measure, and perform operations involving length, mass, and capacity using customary units and metric units. 1 Which is the most appropriate unit for measuring the distance between 2 cities? A millimeter B centimeter C meter D kilometer 1 D 2 Which is the most appropriate unit for measuring the capacity of a car’s gas tank? A milliliter B centiliter C liter D kiloliter 2 C 3 Joshua cut a piece off this log that was 3 feet 5 inches long. What is the length of the remaining portion of the log? 3 B 4 Zachary’s doctor prescribed an antibiotic that he must take for 10 days to treat an infection. The prescription specifies that he is to take a 250-milligram tablet 3 times a day. How many grams of medication will he take over the full course of treatment? A 7.5 g B 75 g C 750 g D 7,500 g 4 A 5 Briana is making punch for a party. She is using a recipe that calls for 5 cups of lemonade when making punch to serve 8 people. How many quarts of lemonade will she need to make this recipe for 56 people? 5 D 6 A 9 ft 2 in. 8 in. Copyright © Glencoe/McGraw-Hill A 5 ft 1 in. C 6 ft 3 in. A 1712 qt B 5 ft 9 in. D 6 ft 9 in. B 14 qt C 10 qt D 834 qt 6 Emma Jaynes weighed 7 pounds 13 ounces at birth. If she exactly tripled her birth weight during her first year, how much did she weigh on her first birthday? A 23 lb 7 oz B 22 lb 3 oz C 21 lb 13 oz D 15 lb 10 oz WESTEST, Grade 10 35 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.4.4 Develop and apply formulas for area, perimeter, surface area, and volume and apply them in solving practical problems. 1 C 2 The Grave Creek Mound at Moundsville, West Virginia, is the largest conical-type burial mound in the United States. It was built by the Adena people, who were Native Americans who lived in this area over 2,000 years ago. The mound is 62 feet in height and 240 feet in diameter. Which is closest to the area of its base? B 45,240 ft2 A 750 ft2 C 46,750 ft2 D 892,800 ft2 2 B 3 Which is closest to the amount of air needed to inflate this beach ball? A 201 in3 B 1,206 in3 C 1,608 in3 D 2,145 in3 3 D 4 B 1 How much cardboard is needed to construct this carton? 10 cm 36 cm 25 cm B 9,000 cm2 D 1,510 cm2 16 in. 4 Mr. and Mrs. Myers plan to fence a portion of their backyard as a play area for their children. One side of the play area will be directly behind the house, so it will not need to be fenced. How much fencing will they need to buy? House 15 ft 37 ft A 52 ft C 107 ft 36 WESTEST, Grade 10 B 67 ft D 555 ft Copyright © Glencoe/McGraw-Hill A 18,000 cm2 C 3,020 cm2 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.4.4 (continued) 5 About how much water will be needed to fill this wading pool 34 of the way full? 5 A 6 B 7 D 8 A 31–2 ft 20 in. A 48 ft3 C 72 ft3 B 69 ft3 D 96 ft3 6 Claudia has chosen a portion of her backyard for planting a vegetable garden. To the nearest square foot, what is the area that she will have available for planting? 10.6 ft 5.4 ft 5.9 ft 8.2 ft Copyright © Glencoe/McGraw-Hill A 30 ft2 C 53 ft2 B 51 ft2 D 60 ft2 7 The soup can and the ice cream cone have the same height, and their bases have the same radius. What is the ratio of the volume of the ice cream cone to the volume of the soup can? A 3:1 B 2:1 C 1:2 D 1:3 8 Tom Ross is a landscaper who is designing a garden to surround a circular fountain in a park in Monongalia County. To the nearest square foot, what is the area Tom will have available for planting? A 302 ft2 B 384 ft2 C 452 ft2 D 1,206 ft2 r r h Garden Fountain 10 ft 22 ft WESTEST, Grade 10 37 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.4.1, 10.4.2, 10.4.4 1 The measures of the four angles of a quadrilateral are in the ratio 6:5:4:3. What are these angle measures? Your solution should include an equation and a check of your answer. 10.4.1 Sample answer: Let 6x, 5x, 4x, and 3x be the angle measures. Since the sum of the measures of the angles of any quadrilateral is 360°, my equation is 6x 5x 4x 3x 360. 6x 5x 4x 3x 360 18x 360 x 20 6x 6(20) 120, 5x 5(20) 100, 4x 4(20) 80, and 3x 3(20) 60, so the angle measures are 120°, 100°, 80°, and 60°. Check: 120° 100° 80° 60° 360° 2 What is the area of this isosceles trapezoid? 14 in. 10.4.2, 10.4.4 Sample answer: All of the measurements need to be in the same units, so I changed the measurements that are given in yards and feet to inches. 2 5 13 ft 3 (12 in.) 20 in.; 1 4 A 1 (B b)h 2 1 (14 20)(9) 2 yd 12–3 ft 1 yd 4 (36 in.) 9 in. Now I can use the formula A A 1– 4 1 (B 2 b)h to find the area of the trapezoid. 153 3 A ball has a radius of 10 centimeters. A cylindrical container has a radius of 10 centimeters and a height of 10 centimeters. Will the ball or the container hold more water? 10.4.4 10 cm 10 cm 10 cm Sample answer: Ball: The formula for the volume of a sphere is 4 4 4,000 V 3 r 3 3 (10)3 3 in3. Container: The formula for the volume of a 4,000 1 cylinder is V r 2h (10)2(10) 1,000 in3. Since 3 1,3333, 4,000 4,000 1,000, so in3 1,000 in3. The ball has the greater volume, 3 3 so it will hold more water. 38 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill The area of the trapezoid is 153 in2. Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.4.1, 10.4.2, 10.4.4 (continued) 4 The figures show an equilateral triangle and a square, each with one exterior angle drawn at each vertex. In each part, explain your reasoning and show any formulas you used to get your answers. 10.4.1 1 1 2 4 3 2 3 Part A Find the measure of each exterior angle of the triangle and the sum of the measures of these angles. Then do the same for the square. Sample answer: The measure of each (interior) angle of an equilateral triangle 180° is 3 60°. Each exterior angle and its adjacent interior angle form a linear pair, so they are supplementary. Therefore, each exterior angle measures 180° 60° 120°, and the sum of the three exterior angles is 3(120°) 360°. The measure of each (interior) angle of a square is 90°. Each exterior angle measures 180° 90° 90°, and the sum of the four exterior angles is 4(90°) 360°. Part B In a certain regular polygon, the measure of each exterior angle is 18°. How many sides does this polygon have? Sample answer: The sum of the exterior angles (1 at each vertex) of any polygon is 360°, and in a regular polygon, each of these angles has the same 360° 360° measure, so the measure of each exterior angle is n. If n 18°, then n 20, so the polygon has 20 sides. Copyright © Glencoe/McGraw-Hill 5 The Lots-a-Fun Toy Company makes hollow blocks and balls. Their most popular products are a block that is 12 centimeters on each side and a ball with a diameter of 16 centimeters. 10.4.4 Part A Find the volume and surface area of the block and the ball. Show all formulas that you use. Sample answer: The block is a cube. V s3 123 1,728 cm3; SA 6s2 6(12)2 864 cm2 4 4 The ball is a sphere. V 3 r3 3 (8)3 2,145 cm3; SA 4r2 4(8)2 804 cm2 Part B Which takes more material to make, the block or the ball? Explain your reasoning. Sample answer: Both objects are hollow, so the material needed to make them is measured by the surface area. Even though the sphere has the greater volume, the block has the greater surface area, so the block requires more material. WESTEST, Grade 10 39 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.5.1 Collect, organize, interpret data, and predict outcomes using the mean, mode, median, range, and standard deviation. 1 Mr. Martinez gave his 5th period Spanish class a five-point vocabulary quiz. The tally shows the students’ scores. What is the mode for these scores? A 3 B 3.13 C 4 D 5 Score Number of Students || 0 ||| 1 |||| 2 |||| || 3 |||| ||| 4 |||| | 5 2 The table shows Veronica’s test scores in her math and history classes. Which statement is true about these scores? Math 83 History 75 A B C D 77 81 86 78 73 90 C 2 D 3 A 91 86 Her median score is higher in history than in math. The range of her scores is greater in history than in math. In math, her mean and median scores are the same. Her mean scores are the same in both classes. 3 The graphs show the distributions of 10th grade standardized math test scores for two high schools. The highest possible scores on both tests is 200, and the scores for both schools are normally distributed. Which conclusion can you draw by comparing these graphs? 50 100 150 200 Score 0 50 100 150 200 Score A The mean score is the same for both schools, but the standard deviation is greater for Southeast High School. B The mean score is the same for both schools, but the standard deviation is greater for Northwest High School C The standard deviation is the same for both schools, but the mean is greater for Southeast High School. D The standard deviation is the same for both schools, but the mean is greater for Northwest High School. WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill 0 Southeast High School Frequency Frequency Northwest High School 40 1 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.5.2 Find the probability of conditional events and mutually exclusive events. 1 The table gives population data from the 2000 U.S. Census. In 2000, which is closest to the probability that a resident of West Virginia lived in Wheeling? 1 B 2 D 3 C 4 B United States West Virginia Charleston, WV Wheeling, WV 284,796,887 1,808,344 53,421 31,419 A 0.17% C 3.0% B 1.7% D 17% 2 The table shows the enrollment at Fox Ridge High School at the beginning of the 2003–2004 school year by grade level and gender. Which is closest to the probability that a randomly chosen female student is a junior or senior? Freshman Sophomore Female 175 183 Male 162 179 Junior 169 182 A 0.531 C 0.482 Senior 147 154 B 0.499 D 0.469 Copyright © Glencoe/McGraw-Hill 3 If Jason draws a marble at random from a bag containing 5 red marbles, 4 blue marbles, 3 yellow marbles, and 6 white marbles, what is the probability that he will draw a marble that is either blue or white? A 4 9 B 1 2 C 5 9 D 2 3 4 The table shows the probability of each sum that can be obtained when a pair of standard 6-sided dice is rolled. On a random throw of a pair of dice, what is the probability that Alexis will roll a sum that is at least 9? Sum Probability 2 3 4 5 6 7 8 9 10 11 12 1 36 1 18 1 12 1 9 5 36 1 6 5 36 1 9 1 12 1 18 1 36 A 1 6 B 5 18 C 4 11 D 5 6 WESTEST, Grade 10 41 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.5.3 Predict the outcomes of simple events using the rules of probability. 1 C 2 Which of the following cannot be the probability of an event? A 1.001 B 1 C 0.001 D 0 2 A 3 Cameron is a college basketball player who made 35 of his last 48 free throws. Which is closest to the probability that he will miss the basket on his next free throw? A 27% B 39% C 50% D 73% 3 A 4 River Branch High School has a total enrollment of 1,265 students. In the Venn diagram, B represents the event, “plays in the band,” and C represents the event, “sings in the chorus.” Which is closest to the probability that a randomly selected River Branch student is a member of the chorus, but not a member of the band? 4 B 5 C 1 Amber tossed a fair penny 4 times, and it landed heads up each time. If she tosses this penny a 5th time, what is the probability that she will get heads again? A 0 B B 178 1 5 C 1 2 D 4 5 C 35 A 14.1% C 20.6% B 17.9% D 27.4% 5 In a standard deck of 52 playing cards, there are 4 suits (spades, hearts, clubs, and diamonds), with the same number of cards in each suit. Within each suit, the jack, king, and queen are called “face cards.” If Erin draws a card at random from a standard deck, what is the probability that she will get a face card? 42 A 10 13 B 4 13 C 3 13 D 3 52 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill 826 Name Date Standards Practice Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. OBJECTIVE 10.5.4 Read, interpret, and construct graphs to solve problems. 1 The students in Mr. Schmidt’s 5th Number of Flavor period class are learning how to Students gather and organize data and to Chocolate 10 display the data in various types of Vanilla 05 graphs. The table shows the results Chocolate Chunk 08 of a class survey of the students’ Other 07 favorite ice cream flavors. If the students make a circle graph to display the data, how many degrees of the circle should they use for the Chocolate Chunk section? A 120° B 96° C 84° D 48° 1 B 2 The table shows the population of West Virginia every 10 years from 1950 through 2000 according to the U.S. Census. Which type of display would be most appropriate to show how the state population changed over this time period? A circle graph B double bar graph C line graph D stem-and-leaf plot 2 C 3 D Year Population 1950 2,005,552 1960 1,860,421 1970 1,744,237 1980 1,949,644 1990 1,793,477 2000 1,808,344 2,000 1,600 1,200 800 400 0 20 19 99 –2 0 00 00 –2 20 0 01 01 –2 20 0 02 02 –2 20 0 03 03 –2 00 4 First Day Enrollment Copyright © Glencoe/McGraw-Hill 3 The bar graph shows first-day enrollment at West Valley High School for several recent school years. Between which two school years did enrollment at this school change the most? School Year A B C D 1999–2000 to 2000–2001 2000–2001 to 2001–2002 2001–2002 to 2002–2003 2002–2003 to 2003–2004 WESTEST, Grade 10 43 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.5.1, 10.5.2, 10.5.3, 10.5.4 1 The stem-and-leaf plot shows the scores for the students in Mrs. Olsen’s fifth-period geometry class on a 100-point chapter test. For these scores, which is greater, the mean or the median? By how many points do these measures of central tendency differ? 10.5.1, 10.5.4 Stem 3 4 5 6 7 8 9 10 | Leaf |5 |27 |368 |122 |025 |244 |358 5 7 9 9 5 6 Sample answer: There are 25 test scores. |0 3 | 5 35 I listed them in increasing order: 35, 42, 47, 53, 56, 58, 61, 62, 62, 65, 70, 72, 75, 77, 79, 79, 82, 84, 84, 85, 86, 93, 95, 98, and 100. To find the mean, I added all these numbers on my calculator 1,800 and divided by 25: 25 72. Since there are 25 scores, the median will be the 13th score from either the lowest or highest score, which is 75. The median test score is 3 points greater than the mean. 2 The following list gives the ages of the people who attended the Watkins family reunion at Beech Fork State Park in southwestern West Virginia: 25, 32, 14, 6, 35, 42, 48, 63, 44, 67, 2, 11, 21, 19, 41, 68. Make a histogram for this data, using 10-year age intervals starting with 0–9. 3 For an annual report, the financial officer of a company made a list of the salaries of all of the employees of the company. Do you think the mean or median would best represent this data? Explain your reasoning. 10.5.1 Sample answer: I think the median is best because half of the people earn above that amount and half of the people below, so it gives me a good idea of how much money the average employee is making. The mean isn’t good because a few high salaries, like that of the president of the company, would affect it too much. 44 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill 10.5.4 Name Date Standards Practice Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. OBJECTIVES 10.5.1, 10.5.2, 10.5.3, 10.5.4 (continued) 4 Eric tossed a penny 3 times and recorded whether he got heads or tails each time. 10.5.2, 10.5.3 Sample answer: Part A Draw a tree diagram that shows the equally likely outcomes of this experiment. Show the probability of each outcome on your diagram. Part B What is the probability that Eric will get heads on exactly 2 of the tosses? Use your tree diagram to answer this question. Sample answer: I see that 3 of the 8 possible outcomes on my tree diagram have 2 heads: HHT, HTH, and THH. Since the 8 outcomes are equally likely, the 3 probability of getting exactly 2 heads is 8. Part C If the 1st penny lands heads up, what is the probability that Eric will get heads on exactly 2 of the 3 tosses? If your answers for Parts B and C are different, explain why. Copyright © Glencoe/McGraw-Hill Sample answer: I only need to look at the outcomes that list H first. There are 4 of these, and 2 of them, HHT and HTH, have 2 heads, so I know that the probability of getting exactly 2 heads if I know the first coin landed heads up 2 1 is 4 2. This answer is different from my answer in Part B because Part C involves conditional probability and uses a reduced sample space. 5 There are 480 students at West River Middle School. The circle graph shows the results of a survey in which all students at the school were asked their favorite sport to watch on television. 10.5.2, 10.5.3, 10.5.4 Basketball 136 Other 80 Football 144 48 72 Hockey Baseball Part A What is the probability that a randomly selected student chose hockey? Sample answer: Since 72 out of 480 students chose hockey, the probability is 72 3 or 0.15 or 15%. 480 20 Part B If you know that a certain student chose either baseball or football, what is the probability that this student chose baseball? Sample answer: There were 48 144 192 students who picked either baseball or football, and 48 of those students chose baseball, so the 48 1 or 0.25 or 25%. probability is 192 4 WESTEST, Grade 10 45 Name Date Sample Test Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 1 Mike is using a road map of West Virginia on which 1 inch represents 22 miles. He measures a straight-line distance of 8.9 inches between Parkersburg and White Sulphur Springs. Which is closest to the actual distance between these cities? 10.3.3 A 205 mi B 196 mi C 190 mi D 180 mi 1 B 2 Which is the solution of 25 34x 58x 13? 10.2.1 2 D 3 B 95 is placed on a number line, between which two points will it be 4 If located? 10.1.3 A 10 and 9 B 9 and 8 C 8 and 9 D 9 and 10 4 A 5 The table shows the enrollment in a high school on the first day of school for several recent years. If enrollment at this school continues to follow this pattern of growth, which is the best estimate of the enrollment on the first day of school of the 2005–2006 school year? 10.2.6 A 1,095 B 1,110 C 1,150 D 1,205 5 C 96 11 A 24 B C 32 D 96 3 All sides of this stop sign are the same length. What is the measure of each interior angle of this polygon? 10.4.1 STOP 46 WESTEST, Grade 10 B 135° D 100° Year Enrollment 2000–2001 0,900 2001–2002 0,945 2002–2003 0,992 2003–2004 1,042 Go on Copyright © Glencoe/McGraw-Hill A 144° C 120° Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 6 What is the value of 4r 2s 10st 6t 3 if r 3, s 6, and t 2? A 288 B 144 10.2.4 C 48 D 288 6 B 7 Mrs. Stevens gave her third-period Geometry class a ten-point quiz. The tally shows the students’ scores on this quiz. What was the mean quiz score? 10.5.1 7 A 8 If two vertical angles are complementary, what are their measures? A 30° and 60° B 45° and 45° 10.3.2 C 60° and 120° D 90° and 90° 8 B 9 Which is closest to the amount of paper needed to make the label for this soup can? 10.4.4 A 86 cm2 B 128 cm2 C 256 cm2 D 299 cm2 9 C 10 D Score Number of Score Number of Students Students | |||| 0 06 |||| || 1 07 | |||| ||| 2 08 || |||| 3 09 || 4 10 ||| 5 A 6.75 B 7.0 C 7.5 D 8.0 7.4 cm 11 cm Copyright © Glencoe/McGraw-Hill 10 Which could be the graph of the equation 3x 4y 12? 10.2.8 A B y y x x O C O D y y x O O x Go on WESTEST, Grade 10 47 Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 11 Austin spent $42 (not including sales tax) for a jacket that was on sale for 25% off. What was the original price of the jacket? 10.1.1 A $168 B $67 C $56 D $33.60 11 C 12 What is the slope of the line that passes through the points at (6, 5) and (3, 11)? 10.2.7 12 B 13 A 14 Geoff and Chris went mountain biking on the North Bend Rail Trail in north central West Virginia. Chris started on the trail at noon and rode at an average speed of 16 miles per hour. Geoff started from the same spot half an hour later and rode at an average speed of 20 miles per hour. If neither of them stopped before they met, at what time did Geoff catch up with Chris? 10.2.3 A 12:54 P.M. B 2:00 P.M. C 2:30 P.M. D 3:00 P.M. 14 C 15 How much water would be needed to fill this fish tank 23 of the way to the top? 10.4.4 15 B A 32 C B 23 2 3 D is 13 If mQS 138°, mQR 148° and PS tangent to circle O at S, what is mP? 3 2 Q 10.3.4 O 32° 37° 46° 69° S R P 12 in. 9 in. 20 in. A B C D 48 2,160 in3 1,440 in3 1,056 in3 704 in3 WESTEST, Grade 10 Go on Copyright © Glencoe/McGraw-Hill A B C D Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 16 D 17 A 18 A 19 The thickness of a red blood cell is approximately 2.4 106 meters. Which is closest to the thickness of 8,000 red blood cells stacked on top of each other, if they could be placed so that there is no space between them? 10.1.2 A 0.000192 m B 0.00192 m C 0.0192 m D 0.192 m 19 C 20 Which is the completely factored form of x4 16? 10.2.9 A (x 2)4 B (x 2)2(x 2)2 C (x2 4)(x2 4) D (x 2)(x 2) (x2 4) 20 D 16 Which graph shows the solution of 3(4 x) 7x 4? 10.2.1 A 5 4 3 2 1 0 1 2 3 4 5 B 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 C D 5 4 3 2 1 0 1 2 3 4 5 17 Jasmine took a survey of 50 students in her middle school in which she asked them to name their favorite class. The table shows the results of her survey. If Jasmine makes a circle graph to display this data, how many degrees of the circle should she use for the Math section? 10.5.4 A 64.8° B 40.0° C 32.4° D 18.0° Number of Students Art 5 English 7 Math 9 Music 8 Science 7 Social Studies 8 Other 6 Class 18 If LMN QTR, which of the following must be true? 10.3.3 A mM mT B L R Copyright © Glencoe/McGraw-Hill C LM QT MN QR D LN QR Go on WESTEST, Grade 10 49 Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 21 D 22 Mrs. Fox takes a 600-milligram calcium tablet every morning. How many grams of calcium does she take each week? 10.4.2 A 0.42 g B 4.2 g C 42 g D 420 g 22 B 23 The formula y mx b gives the equation of a line with slope m and y-intercept b. How can this formula be rewritten to give the x-coordinate of a specific point (x, y) on the line if the y-coordinate of the point, the slope, and the y-intercept are known? 10.2.2 23 A 24 C 25 C 21 Which graph shows all solutions of |x 6| |3x 3|? 10.2.5 A 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 5 4 3 2 1 0 1 2 3 4 5 B C D yb yb A x m B x m ym by D x m 24 The table shows the probability of each sum that can be obtained when a pair of standard 6-sided dice is rolled. On a random throw of a pair of dice, what is the probability that Lindsay will get a sum that is a multiple of 3? 10.5.2 Sum Probability 2 3 4 5 6 7 8 9 10 11 12 1 36 1 18 1 12 1 9 5 36 1 6 5 36 1 9 1 12 1 18 1 36 A 1 4 B 5 18 C 1 3 D 4 11 25 If line m is parallel to line n, what is the value of x? 10.3.2 A 132 B 42 C 33 D 12 50 WESTEST, Grade 10 48 m 4x n Go on Copyright © Glencoe/McGraw-Hill C x b Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. Temperature (F) 26 The line graph shows the average daily high temperature in Charleston. In which month is the average daily high temperature closest to that in April? 10.5.4 90 80 70 60 50 40 0 26 C 27 C 28 A 29 C 30 A J F M A M J J A S O N D J Month A May C October B September D November 27 P and Q are points on circle O. Which term describes PQ? 10.3.4 A chord B diameter C secant D tangent 28 What is another way to write P O Q 4 ? 10.1.3 10 6 A 10 6 B 10 6 10 6 C 4 Copyright © Glencoe/McGraw-Hill D 1 29 Which is closest to h, the length of the altitude to the base of this isosceles triangle? 10.3.5 A 6.6 cm B 7.0 cm C 10.9 cm D 11.0 cm 30 Which expression is equivalent to A y12z4 x6 B x6 1 y 2z4 12 cm h 10 cm x2y3z0 2 ? 10.2.4 xy3z2 C z4 2 x D y36z4 x8 Go on WESTEST, Grade 10 51 Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. 31 What are the quotient and remainder when 8x2 6x 36 is divided by 2x 5? 10.2.9 A quotient: 4x 7; remainder: 1 B quotient: 4x 7; remainder: 1 C quotient: 4x 7; remainder: 1 D quotient: 4x 7; remainder: 1 31 C 32 If the circumference of a fully inflated beach ball is 46 centimeters, which is closest to the amount of air that was needed to inflate it? 10.4.4 A 305,700 cm3 B 50,950 cm3 C 38,200 cm3 D 6,650 cm3 32 B 33 The table shows the enrollment at Fairways Middle School on the 1st day of the 2003–2004 school year by grade level and gender. Which is closest to the probability that a randomly chosen boy enrolled in this school on that day was a 7th grader? 10.5.2 33 D 34 Danielle spent $17.34 at the Philippi Post Office for a combination of 37-cent and 23-cent stamps. If x represents the number of 23-cent stamps and she bought a total of 62 stamps, which equation describes this situation? 10.2.3 A 0.23x 0.37(x 62) 17.34 B 23x 37(62 x) 17.34 C 23x 37(x 62) 17.34 D 0.23x 0.37(62 x) 17.34 34 D 35 In parallelogram PQRS, what is mS? 10.4.1 35 B Grade 6th Grade 7th Grade 8th Grade Boys 106 112 095 Girls 098 108 103 B 0.333 D 0.358 Q (3 x 23) P (x 15) A 108° C 128° 52 WESTEST, Grade 10 R S B 118° D 135° Go on Copyright © Glencoe/McGraw-Hill A 0.180 C 0.350 Name Date Sample Test (continued) Read each question and choose the best answer. Then write the letter for the answer you have chosen in the blank at the right of each question. (2)5 4(3) 5254 36 A 37 D 38 A 39 Which is an equation of the line that passes through the point at (3, 7) and is parallel to the x-axis? 10.2.8 A x 3 B y7 C xy4 D x y 10 39 B 40 Lisette Tompkins is a landscaper who is designing a garden for a park in Monroe County. She has decided that the garden will have decorative stones in the center, surrounded by plants. To the nearest square foot, what is the area that Lisette will have available for planting? 10.4.4 A 763 ft2 B 243 ft2 C 191 ft2 D 127 ft2 40 C 36 What is the value of 2 ? 10.1.1 44 19 A 20 B C 290 D 20 37 What is the probability that a randomly chosen two-digit positive integer will be a multiple of both 3 and 5? 10.5.3 8 15 B 1 3 C 1 8 D 1 15 Copyright © Glencoe/McGraw-Hill 38 To walk from his home to his school, Frank can either walk south on Elm Street and then west on Second Avenue, or he can cut diagonally across an empty lot. How much distance will School he save by walking across the empty lot? 10.3.5 A 440 ft B 660 ft C 770 ft D 1,100 ft Home 660 ft Elm St. A 880 ft Second Ave. Plants Stones 9 ft 18 ft Go on WESTEST, Grade 10 53 Name Date Sample Test (continued) Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. 4 as a single term involving a simplified radical. 10.1.3 41 Write 75 3 Sample answer: 4 25 75 3 3 3 15 3 23 3 4 3 53 2 3 53 2 3 3 3 23 53 3 13 3 3 42 On an algebra quiz, Maria had to solve 4 2x 3(x 2). Here is what she wrote on her quiz paper. 4 2x 3(x 2) 4 2x 3x 6 2x 3x 10 5x 10 x2 Maria’s teacher did not give her credit for this problem. Explain Maria’s error and show how to complete the solution correctly. 10.2.1 Sample answer: Maria’s work was correct until the last step, but then she forgot that when you multiply or divide both sides of an inequality by a negative number, you have to reverse the inequality symbol. Since she divided both sides by 5 in the last step, the final inequality should be x 2. 2– 3 ft 14 in. 13–4 ft Sample answer: To find the amount of cardboard needed to construct this carton, I need to find its surface area. To do this, all of the measurements must be in the same units, and I need to first give my answer in square inches, so I convert the measurements that are given in feet to inches. 2 3 ft 2 (12 3 3 in.) 8 in., and 14 ft 1.75(12 in.) 21 in. The carton is a rectangular prism, so its surface area is given by the formula S 2LW 2LH 2WH 2(21 in.)(14 in.) 2(21 in.)(8 in.) 2(14 in.)(8 in.) 1,148 in2. There are 122 144 in2 in a square foot, so the number of square 1,148 8. feet is 144 Go on It would take 1,148 in2 or about 8 ft2 of cardboard to construct the carton. 54 WESTEST, Grade 10 Copyright © Glencoe/McGraw-Hill 43 How much cardboard is needed to construct this carton? First give your answer in square inches and then give it to the nearest square foot. 10.4.2, 10.4.4 Name Sample Test Date (continued) Read each problem carefully and write your answer in the space provided. Show all your work and use complete sentences to answer all questions. is 44 PR is tangent to circle O at R, and PS tangent to circle O at S. 10.3.4, 10.3.5 R O P Part A Look at PRO and PSO. What kind of triangles are these? What is the relationship between the two S triangles? Explain how you know. Sample answer: They are both right triangles and they are congruent. I know they are right triangles because a radius of a circle is perpendicular to a tangent at the point of tangency, which tells me that PRO and PSO are both right O and S O are radii, and all radii of the same circle are congruent, angles. Since R I know that RO SO . Also, O P is common to both triangles and is the hypotenuse for both of them. So I know that PRO PSO by the Hypotenuse-Leg congruence theorem. Part B If the radius of circle O is 8 centimeters and OP 17 centimeters, what is the length of the tangent segment PS ? is the hypotenuse. I used the Sample answer: In right triangle PSO, OP Copyright © Glencoe/McGraw-Hill 10 8 6 4 2 0 0– 10 9 –1 20 9 –2 30 9 –3 40 9 –4 50 9 –5 60 9 –6 70 9 –7 80 9 –8 9 45 The histogram shows the age distribution of the people who attended the Fox family reunion that was held at Watoga State Park in the mountains of Pocahontas County. 10.5.2, 10.5.3, 10.5.4 Number of People 172 82 225 15 cm. Pythagorean Theorem to find PS: PS Part A What is the probability that a Age randomly selected person who attended the reunion was 40–49 years old? Sample answer: Since 8 out of 50 people were ages 40 to 49, the probability is 8 4 or 0.16 or 16%. 50 25 Part B If you know that a certain person at the reunion is under age 50, what is the probability that this person is at least 40 years old? Sample answer: A man who is aged at least 40, but less than 50, must be 40–49 years old. There were 4 7 5 10 8 34 people under age 50, 8 4 and 8 of these people were in the 40s, the probability is 3 1 or about 4 7 0.235 or 23.5%. Part C If your answers in Parts A and B are different, explain why. Sample answer: Part B involves conditional probability and uses a reduced sample space. STOP WESTEST, Grade 10 55
© Copyright 2025