
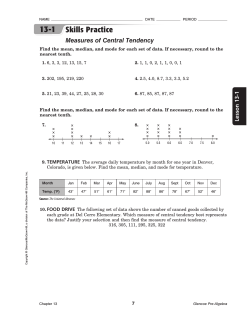
Document 423679
Exercises 4
C (3 x − x 2 ),0 ≤ x < 4
1. Consider the function f(x)=
0Otherwise
could f(x) be a probability density function for any value of C? Suppose we require the function to be a
probability density function when 0≤x≤3. Find the value of C.
2. The probability density function of X, the lifetime of a certain type of electronic device (measured in
hours, is given by
15 / x 2 , x > 15
0, x ≤ 15
f(x)=
(i) Find P(X>30), (ii) What is the cumulative distribution function of X?
(iii) What is the probability that of 6 such types of devices at least 3 will function for at least 15 hours?
What assumptions are you making?
3. For a random variable Y, show that
E(Y) =
∫
∞
0
∞
P(Y ≥ y )dy − ∫ P(Y < − y )dy .
0
4. Let X be a random variable that takes on values between 0 and c. That is
P{0≤X≤c} = 1 Show That
Var(X) ≤ c2/4
Hint: One approach is to first argue that
E[x2] ≤ cE[x]
Then use this to show that
Var(X) ≤ c2[α (1-α) ] where α= E[X]/c
5. This question is the continuous version of Q5 of Exercises3.
Show that for a non-negative random variable Y, E(Y)=
∞
∫ P(Y > t )dt .
0
Use this result to show that for a non-negative random variable X , E[Xn] =
Hint: Start with E[Xn] =
∫ P (X
∞
0
n
)
> t dt .
∫
∞
0
nx n −1 P( X > x )dx
6. The number of minutes of playing time of a certain high school basketball players in a randomly
chosen game is a random variable whose probability density function is given in the following figure.
Find the probability that the player plays
(a) over 15 minutes;
(b) between 20 and 35 minutes;
(c) less than 30 minutes;
(d) more than 36 minutes.
7. Suppose that the travel time from your home to your office is normally distributed with mean 40
minutes and standard deviation 7 minutes. If you want to be 95 percent certain that you will not be late
for an office appointment at 1 PM, What is the latest time that you should leave home?
8. The median of a continuous random variable having distribution function F is that value m such that
F(m) =1/2. That is, a random variable is just as likely to be larger than its median as it is to be smaller.
Find the median of X if X is
(a) uniformly distributed over (a, b),
(b) normal with parameters (µ, σ2),
(c) exponential with mean λ
9. If X has hazard rate function λx(t), compute the hazard rate function of a X where a is a positive
constant.
10. The lung cancer hazard rate of a t-year-old male smoker, A(t), is such that
λ(t) = .027 + .00025(t-40)2
t ≥40
Assuming that a 40-year-old male smoker survives all other hazards, what is the probability that he
survives to (a) age 50 and (b) age 60 without contacting lung cancer?
11. If X is uniformly distributed over (-1, 1), find
(a) P{|X|>1/2};
(b) the density function of the random variable |X|.
12. The number of years a radio functions is exponentially distributed with parameter λ =1/8. If Jones
buys a used radio, what is the probability that it will be working after an additional 8 years?
© Copyright 2024










![1. (1) Suppose Xn ⥠0 and Xn â X a.s. If E[Xn] â E[X], show that E](http://cdn1.abcdocz.com/store/data/001458375_1-37d39cb06fc416578282896c02eb6044-250x500.png)









