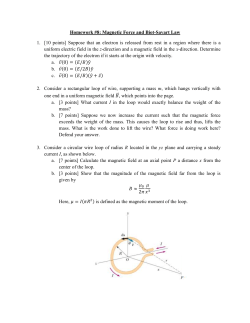
Homework 10 – Magnetization
1. Suppose that a magnetic dipole is located at the origin with the dipole moment vector ⃗⃗
pointed in the positive z-direction.
a. [4 points] Show that the magnetic field for the dipole in Cartesian coordinates
is given by
⃗
[(
)̂
(
)̂
(
) ̂]
b. [4 points] Use a graphing utility (e.g. Mathematica, MATLAB, etc.) to graph
the magnetic field in Cartesian coordinates. Discuss the properties of the
magnetic dipole field. What direction does the field point on the x-axis, y-axis,
and z-axis? How is this field similar to the field of an electric dipole?
c. [2 points] Show that the magnetic field of a dipole can be written in
coordinate-free form
⃗
[ ( ⃗⃗
̂) ̂
⃗⃗ ]
Answer:
(a) Below is a schematic of a magnetic dipole located at the origin with the dipole moment
vector ⃗⃗ pointed in the positive z-direction
As shown in class, the vector potential of a magnetic dipole can be written as
⃗⃗
̂
The magnitude of the vector potential is given by
| ⃗⃗
| |
Since
̂|
√
is tangent to a horizontal circle around the z-axis, its components are
(
)
√
(
√
Since ⃗
)
, then each component of ⃗ is given by
[
[
(
)
(
)
( )
(
)
(
)
( )
(
)
( )
(
(
)]
)
[(
)( )
[
]
⃗
[(
(
)( )
]
]
[
Therefore, we have
)̂
(
)̂
(
) ̂]
(b) The plot of the magnetic dipole in the xz plane is given below
]
As see here, the magnetic field points upward along the z-axis and downward along the x-axis.
The plot of magnetic dipole in the yz plane is given below.
The full 3D picture of the magnetic monopole is given below
(c) Since the field of the magnetic dipole is identical to the field of an electric dipole, we can
write the magnetic dipole field in spherical coordinates, which gives
̂)
(
̂
⃗
First, we note that
( ⃗⃗
̂) ̂
( ⃗⃗
̂
⃗⃗
̂
̂ ) ̂ . Therefore,
̂
̂
Therefore, the electric field of a dipole can be written as
⃗
( ⃗⃗
̂) ̂
⃗⃗
̂
̂
2. Multipole expansion for a volume current
a. [2 points] Derive an expression for the multipole expansion for a volume current
distribution .
b. [4 points] Explicitly show that the monopole potential vanishes. Does the
monopole term vanish outside of magnetostatics? Defend your answer.
c. [4 points] Show that the magnetic dipole moment for a volume current
distribution is given by
⃗⃗
∫(
)
Answer:
(a) Recall that the vector potential for a volume current distribution is given by
∫
( )
Based on our multipole expansion in class, we can write
(
∑( )
)
Therefore, our vector potential becomes
( )
∫
∫( )
∑
(
) ( )
(b) The monopole term in the above expression is given by
∫ ( )
By the continuity equation, we can write the volume current density in terms of the
volume charge density
∫(
)
∫(
)
∫(
)
∫(
)
The RHS of the above expression is zero in magnetostatics; thus, in magnetostatics, we
have
∫(
)
We can examine this for each individual component. For the x-component, we can use
the product rule to give
∫(
)
∫
( )
∫
∫
( )
∫
Since is entirely inside the volume, applying the divergence theorem on the first term
gives zero. Therefore, we have
∫
∫
Therefore, for magnetostatics, we have
∫ ( )
In general, this term does not vanish outside of magnetostatics
(c) By definition, the magnetic dipole moment is given by
⃗⃗
∮
To prove the last line, we can draw a cone subtended by the loop at the origin. If we
divide the conical surface into infinitesimal triangular wedges, each with vertex at the
origin and opposite side
is:
, then we have the area of each infinitesimal triangular wedge
( )( ). By definition the vector area is perpendicular to the surface. Thus, the
vector area is half of the area of the parallelogram spanned by the vectors
on the geometric interpretation of the cross product we have
∮
Applying this to a volume current distribution gives
⃗⃗
∮(
)
and
. Based
3. Consider an arbitrarily shaped loop carrying current in the presence of a uniform
magnetic field directed in the positive ̂ direction. The force operating on a current
element is given by
⃗
a. [6 points] Prove that the torque operating on this current-carrying loop in the presence
of the uniform magnetic field ⃗ is given by
⃗
( ∮
⃗
)
⃗
⃗⃗
b. [4 points] Verify the relationship in (a) for the case of a torque on a rectangular
current loop in a uniform field as shown below.
Answer:
(a) By the Lorentz force law, we have
⃗
⃗)
(
Integrating over the entire loop (assuming that
⃗
∮
) gives
⃗)
(
According to Problem 1 from Homework 1, we have the following vector identity
[
(
⃗ )]
[⃗
(
)]
[
(⃗
)]
This implies that
⃗
∮
(
⃗)
∮(
)
⃗
∮( ⃗
)
( )
Since the magnetic field is uniform and points in the positive ̂ direction, we can
explicitly compute these two integrals in Cartesian coordinates. For the first integral on the RHS
of (1), we have
⃗
)
∮(
)̂
∮[(
(
) ̂]
∮[
̂
̂]
In the last line, we have used
∮ (
)
∮
∮
∮ (
)
∮
∮
For the second integral on the RHS of (1), we have
∮( ⃗
)
)̂
∮[(
(
)̂
(
) ̂]
)̂
∮[(
(
) ̂]
In the last line, we have used
∮ (
)
)
∮(
Therefore, evaluating the RHS of (1) has shown us that
∮( ⃗
)
)
∮(
⃗
Therefore, the torque on the arbitrary current loop is given by
⃗
∮(
)
⃗
∮( ⃗
)
⃗
)
∮(
∮(
)
⃗
⃗⃗
⃗
(b) From the figure above, the loop has a magnetic moment of magnitude
. The
forces on the sloping sides cancel each other and produce no torque because they pass
through a common origin. Thus, the torque on the loop arises from the forces that act on
the horizontal sides. Each of these forces has a magnitude
and the magnitude of
the position vector from the axis of rotation is ( )
. Therefore, the magnitude of the
torque on the loop is
(
)( )
(
)
The torque acts in a direction to bring the magnetic moment parallel to the magnetic field
and thus it points in the positive x-direction. Therefore, the net torque is
⃗
̂
⃗
⃗⃗
⃗
4. Consider an arbitrarily shaped, infinitesimal loop with dipole moment ⃗⃗ in the presence
of an inhomogeneous magnetic field ⃗ .
a. [6 points] Prove that the magnetic force is given by
( ⃗⃗ ⃗ )
b. [4 points] Verify the expression in (a) for a circular wire of radius R, carrying a
current I, suspended above a short solenoid, as shown below.
Answer:
(a) Let’s assume that the dipole is an infinitesimal square current loop as shown below
The Lorentz force for this scenario is given by
around in the direction of the current, we have
⃗ . Starting from the origin and going
{(
̂)
⃗(
)
{ (
̂)
{ (
̂)
(
[⃗ (
)
⃗
⃗
{ ̂
⃗(
̂)
̂
(
⃗
)
)}
̂) ⃗ (
(
) ⃗(
)]}
̂) [ ⃗ (
⃗
⃗
{ (̂
)
( ̂
) }
(
⃗(
)]
⃗
̂)
⃗(
̂)
}
)
(
}
The total force is given by
∮{ ̂
⃗
̂
[̂ (
⃗
}
⃗
{ ̂
)
⃗
̂
̂
Since ⃗⃗ points in the positive x-direction, then we have
generalized to three-dimensions.
}
̂]
[
(
̂
)
̂
( ⃗⃗
̂]
⃗ ). This can be
(b) According to the figure, the magnetic field has an outward radial component everywhere
around the ring. If the current flows in the direction indicated, each element of the loop
must experience a downward force of the magnitude
. If
has the same magnitude
at all points on the ring, as it must since it comes from a solenoid, the total force will
(
)
have the magnitude
⃗
Now
can be directly related to the gradient of . Since
at all points, the net
flux of magnetic field out of any volume is zero. If we replace the ring with a circular
slab of thickness
as shown above, we note that the outward flux from the side is
( )
[
( )
and the net outward flux from the end surfaces is
(
)]
(
). Since the total outward flux is zero, we have
Therefore, the downward force has the magnitude
(
Since
)
(
)
points in the vertical direction, we have
(
)
( ⃗⃗
⃗)
5. Consider a sample of an arbitrary substance, contained in a test tube suspended by a
spring, in the presence of an intense magnetic field produced by a solenoid, as shown
below
a. [3 points] Explain why the maximum force is located on the edge of coil. What is
the magnetic force at the center of the ring? Defend your answers.
b. [3 points] Explain why the substance in the above figure would be attracted into
(pushed out) the solenoid if it contained magnetic dipoles that are parallel
(antiparallel) to the field.
c. [4 points] Suppose that the total strength of the magnetic dipole moments were
proportional to field strength of the solenoid, prove that the force on the substance
must be proportional to the square of the solenoid current. [This is the observed
behavior in the case of the diamagnetic and paramagnetic substances]
Answer:
(a) The presence of the magnetic field magnetizes the substances in the coil. At distances far
from the substance, the field of the magnetized substance is approximately the same as a
dipole. This means that the force on the coil is given by
( ⃗⃗ ⃗ ), as derived in
Problem 4. Since the force is proportional to the gradient of the magnetic field, the
strongest force will be felt at the edge of the coil. Moreover, since the magnetic field in
the center of the solenoid is constant, the force felt at the center of the ring is zero.
(b) Since the magnetic force is given by
( ⃗⃗ ⃗ ), this implies that there will be an
attractive force if the magnetic dipole moment is aligned parallel to the external magnetic
field. This is the effect of paramagnetism. However, if the magnetic dipole moment of the
substance is aligned antiparallel to the external magnetic field, this induces a negative (or
repulsive) force on the substance. This is the effect of diamagnetism.
(c) If the magnetic dipole moment is proportional to the field strength, then we have
( ⃗⃗
⃗)
Since the magnetic field inside the solenoid is directly proportional to the solenoid
current, then we have that
Bonus: [8 points] Average magnetic fields
(a) Prove that the average magnetic field, over a sphere of radius R, due to steady currents
within the sphere is
⃗⃗
⃗
(b) Show that the average magnetic field due to steady currents outside the sphere is the
same as the field they produce at the center.
Answer:
(a) By definition the average magnetic field over a sphere is given by
⃗
∫⃗
∫(
)
∮ (∫
)
∮
Since depends only on the source point, we can rewrite the above expression as
⃗
∮ (∫
)
∮
(∫
)
To evaluate the surface integral, let’s assume that the source point lies on the z-axis. By
symmetry, the surface integral will only have contributions in the z-direction and we have
∫
̂ ∫
̂
√
̂ ∫
( )
̂∫
( )
√
̂∫
( )
√
( )
√
̂
{ ( )
For
̂
, we have
⃗
Since ⃗⃗
∫
(
)
∫(
)
∫(
)
, we have
⃗⃗
⃗
(b) Here, we have
⃗
and thus, we have
(
)
∫(
( )
)
∫
̂
Therefore, the average magnetic field due to steady currents outside the sphere is the same as the
field they produce at the center.