ABC
docz
Explore
Log in
Create new account
Download
Report
home and garden
home improvement and repair
plumbing
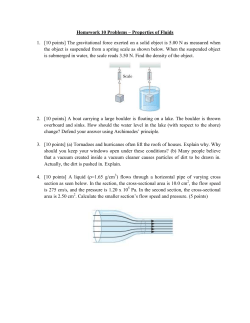
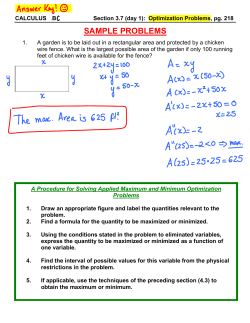
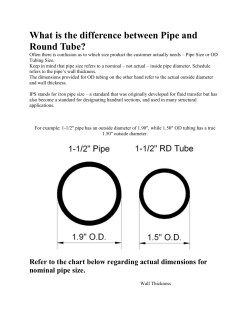
Document 439904
Homework 10 Problems – Properties of Fluids
How to Read a Performance Pipe Gas Printline
SAMPLE PROBLEMS
What is the difference between Pipe and Round Tube?
ENS1101 - Engineering Mechanics Assignment Lecturer: Dr Shuo LI
FR Pipe MEGA www.marleypipesystems.co.za
Sample HW08 - PHYS 21800
How to calculate theoretic weight of a steel pipe and tube
“Multiblock T” Connection fittings for two pipe heating systems
IGEM2014 Seminar by Japan System Planning from corrosion and leakage
© Copyright 2026
About abcdocz
DMCA / GDPR
Report
