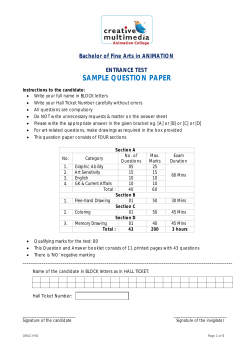
SCALE DRAWING - Rosshall Academy
©TeeJay Publishers General Homework for Book 3G Ch 5 - scale drawings Homework Chapter 5 Scale Drawings / Enlargements Enlargements Exercise 1 1. Make a neat “two-times” enlargement of each of these shapes :- (each box = 1 cm) (a) (b) (c) (d) 5 cm 3 cm (e) 0·5 cm 6 cm 1 cm 1 cm 8 cm 1·5 cm 7 cm 2. Make enlargements of the following using the given scale :(a) (b) 2 cm 1 cm 4 cm 4 cm make a three times enlargement. 4 cm make a two times enlargement. this is Chapter Five page 25 ©TeeJay Publishers General Homework for Book 3G (c) Ch 5 - scale drawings (d) 2 cm 5 cm 4 cm 3 cm 8 cm reduce this shape to half its size. reduce this shape to half its size. Exercise 2 1. This drawing of the side of a wooden crate is done using a scale :- 1 cm = 10 cm (a) Calculate the REAL width of the crate (... x 10) (b) Calculate the REAL height of the crate. (c) Calculate the length of the real diagonal support bar. 2. 10 cm 6 cm 8 cm This cabinet has been drawn to a scale of :- 1 cm = 20 cm 4 cm (a) Calculate the real height of the cabinet. (b) Calculate the real width of the cabinet. 2·5 cm 3. This rectangular piece of land has been purchased by Tisco. A new superstore has to be constructed on it. The scale is :(a) (b) 1 cm = 25 metres. Calculate the real length and the real breadth of the land. 5 cm TISCO Calculate the perimeter of the rectangular piece of land. 8 cm this is Chapter Five page 26 cont’d ....... ©TeeJay Publishers General Homework for Book 3G 4. Ch 5 - scale drawings When this caravan is drawn to a scale of :- 1 cm = 0·5 metres , its length is 9 cm. Calculate the length of the real caravan. 9 cm 5. When this lighthouse is drawn to a scale of :- 1 cm = 2·5 metres , 18 cm its height is 18 cm. Calculate the real height of the lighthouse. 6. When this cello is drawn to a scale of :- 1 cm = 0·2 metres its length is 6 cm. 6 cm Exercise 3 Calculate the real length of the cello. You will need a ruler in this exercise 3 metres 1. This is a “rough” sketch of Edward’s bedroom. 2 metres Make an accurate scale drawing of his room using a simple scale of :- 1 cm = 1 metre. 2. This door frame measures 150 centimetres by 90 centimetres. Make a scale drawing of the door frame using a scale :- 150 cm 1 cm represents 30 cm. 90 cm this is Chapter Five page 27 cont’d ...... ©TeeJay Publishers 3. General Homework for Book 3G Shown is a metal support for a hanging . flower basket. It is in the shape of a right angled triangle. Ch 5 - scale drawings 24 cm Make a neat scale drawing of the support using a scale of :- 64 cm 1 cm = 8 cm. 4. This sketch shows the plan of the side wall of a small theatre. THEATRE Make a scale drawing of it using a scale of :- 1 cm represents 2 metres. (Show the door in your drawing) 1m 3m 2m 30 m 5. (a) Make a scale drawing of this gymnastic arena. S P 25 m R 40 m Q (b) Helga somersaults from corner S to corner Q. Show this as a dotted line on your drawing. (c) Measure the length of the dotted line on your drawing in centimetres. (d) Calculate the real distance somersaulted by Helga in metres. Exercise 4 1. scale :- 1 cm = 5 metres. You will need a ruler and protractor in this exercise H The sketch shows a flag pole supported by a wire (AH). The distance from A to B is 4 metres. ∠HAB = 50°. (a) Make a scale drawing using a scale :- 1 cm = 2 metres. (b) Use your drawing to calculate the real height of the flag pole. this is Chapter Five page 28 A 50° 4m B 12 m ©TeeJay Publishers 2. (a) General Homework for Book 3G Ch 5 - scale drawings T Make a scale drawing to show this tree as it is viewed from point A. scale :- 1 cm = 2 metres. (b) Now, find the height of the real tree. A 30° B 20 metres 3. For both of these sketches :– (i) (ii) Make a scale drawing using the given scale. Calculate the real height of the given object. (a) (b) Kite monument scale :1 cm = 5 m rope scale :1 cm = 20 m 40° 55° 100 metres 20 metres N Exercise 5 1. Make a copy of this compass rose and fill in the eight directions shown. 2. How many degrees are there from :- Copy (a) North to South (clockwise) (b) North to West (clockwise) (c) North to North-West (clockwise) (d) North West to South (clockwise) (e) South-West to South-East (clockwise) (f) N.W. to S.W. (anti-clockwise) ? 3. (a) Joe was facing South-East. He then made a In which direction is Joe now facing ? 1 4 turn clockwise. (b) Helen and Dave were facing North West. They then turned through 90° anti-clockwise. In which direction did they end up facing ? this is Chapter Five page 29 ©TeeJay Publishers 4. General Homework for Book 3G Ch 5 - scale drawings A racing yacht and a cruise liner leave harbour (H) at the same time. yacht 30 km H • 60 km cruise liner The yacht travels 30 kilometres North West. The liner sails 60 kilometres due East. (a) Make a scale drawing of the two journeys. scale 5. 1 cm = 10 km. (b) Measure the distance between the two boats in centimetres. (c) Now calculate the real distance between them in kilometres. Two helicopters leave Stanstead Airport in London. N Stanstead Airport 80 km 120 km One flies South West for 80 kilometres. The other flies South East for 120 kilometres. (a) Make a scale drawing of the two flights. scale 1 cm = 20 km. (b) Measure the distance between the two helicopters, in centimetres. (c) Now calculate the real distance between the two helicopters, in kilometres. this is Chapter Five page 30 ©TeeJay Publishers General Homework for Book 3G Ch 5 - scale drawings Exercise 6E 1. 2. 3. Look at these “scales”. (a) 1 cm to 20 cm. What would you MULTIPLY any measurement on your scale drawing by to obtain the real length ? (b) 1 cm to What would you MULTIPLY any measurement on your scale 1 2 m drawing by to obtain the real length ? (c) 1 : 15 What would you MULTIPLY any measurement on your scale drawing by to obtain the real length ? (d) 1 cm to 10 cm What would you DIVIDE a REAL length by to decide what its length should be in your scale drawing ? (e) 1 cm to 5 m What would you DIVIDE a REAL length by to decide what its length should be in your scale drawing ? (careful !) Measure these lines with a ruler and use the given scales to find the “real life” distance the lines represent :– (a) Scale 1 cm to 50 cm. (b) Scale 1 cm to 2 m. (c) Scale 1 : 5000. This model bicycle was made to a scale of 1 cm to 25 cm. (a) Measure the length of the bike in the drawing above. (b) Use the scale (1 cm = 25 cm) to find the length of the real bicycle. 4. The drawing of a football pitch is done to a scale of 1 cm to 20 metres. (a) Measure the length of the pitch. (b) Calculate the length of the real pitch. this is Chapter Five page 31 ©TeeJay Publishers General Homework for Book 3G Ch 5 - scale drawings Exercise 7E 1. Calculate the REAL lengths/heights of these models, given their model lengths or heights along with their scale factors :– (a) A model train, length 9 cm. (scale factor 100). (b) A model yacht, length 11·5 cm. (scale factor 50). (c) A model spaceship, height 12·8 cm. (scale factor 200). 2. A model of a Tyrannosaurus is 20 centimetres long. The scale of the model is 1 : 40. What is the real length of the Tyrannosaurus ? 20 cm 3. Drawings are made of some objects. Calculate the heights of the objects in the drawings given their real heights and scale factors :– (a) A flagpole 8 metres high. 1 (scale factor 100 ) (i.e. 800 cm ÷ 100) 1 (scale factor 50 ) (b) A house 450 centimetres high 1 (scale factor 200 ) (c) A set of ladders 620 cm long. 4. A model of a toy soldier has a model sword. 120 cm The scale of the model is 1 : 15. If the length of his real sword is 120 cm, what is the length of the model sword ? 5. The elephant in this picture is to be drawn 1 25 of its real life size. If the actual elephant is 3·5 metres tall, what will the height of the elephant be in the picture ? 6. These two arrows are SIMILAR. (a) Write down the scale factor from the smaller arrow to the larger one. (it is not a whole number) € (b) Use this information to find the height of the larger arrow given the height of the smaller one is 5 cm. € this is Chapter Five page 32 8 cm 12 cm
© Copyright 2025