G.C.2 Wkst 1
G.C.2 STUDENT NOTES WS #1
1
Circle Basics
Thus far we have done a number of things involving circles such as circumference, area, sectors, constructions,
and regular polygons and yet we haven’t looked specifically at the properties and relationships found in a
circle. The first step in this process is to define the circle.
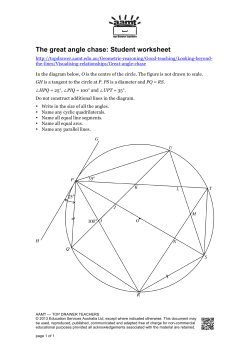
A circle is the locus of all points that are equidistant from a given point,
called the center. The distance from the center to its points is called the radius.
The word locus can be translated to mean ‘the set of’. Thus a circle is the set of all
points that are equidistant from a given point. When we name a circle we refer to it
by its center, so for example the circle to the right is called Circle A ( ⊙ A ).
r
A
The radius of the circle is the fixed distance from the center to the edge of the circle,
so for example the circle to the right has a radius of r.
A circle is quite unique because only a single value defines it shape even through it’s
a two dimensional object. Since all aspects of a circle’s size depends on the length
of its radius, we can change the size of any circle by simply dilating the radius by a
scale factor to either make it bigger or smaller.
There are a number of specific terms that are related to circles that we need to also define and clarify.
Circumference – We have already looked at this in an earlier objective but the circumference of a circle is the
distance around the circle and is calculated by C = πd or C = 2πr.
B
F
C
D
A
Chord -- A chord is a line segment that has both endpoints on the circle. The
longest chord possible in a circle is the one that passes through the center of the
circle and is called the diameter of the circle. A diameter always divides a circle
into two congruent arcs. These congruent arcs are called semi-circles. A semicircle has an arc measure of 180°. A radius is NOT a chord because it does not
have both of its endpoints on the circle.
Chords -- AB , CD , EF
E
Diameter -- EF
Two other important objects that deal with a circle are tangents and secants. These
are two specific types of lines that interact with the circle. A tangent line intersects the
circle EXACTLY ONCE at a point known as the point of tangency. A secant line on the
other hand intersects the circle EXACTLY TWICE forming a chord inside the circle.
B
A
E
D
Secant AB
Chord AB
Tangent CE
Point of Tangency D
C
D
E
F
Arc – An Arc is a piece or portion of the circumference of a circle. Arcs are classified into
two types, major and minor arcs. Major arcs have a value greater than 180° and minor
arcs have a value less than 180°. These arcs also have naming conventions; minor arcs are
named using its two endpoints, such as DE or FD while major arcs include a third point
between its two endpoints to help distinguish the direction and size of the arc, such as
DEF or EDF .
G.C.2 STUDENT NOTES WS #1
2
Semi-Circle – You might have noticed that major and minor arcs were either bigger or
smaller than 180°. So how do we refer to the arc when it is exactly 180°? It is called a
semi-circle. A semi-circle is an arc that is exactly half of the circle and has an arc measure
of 180°. There is no distinction as to whether semi-circles should be written as a major
H
I
M
or minor arc - actually either is acceptable, such as GH , GMH , GIH . The reason you
might use three letters is to help distinguish which half of the circle you are referring to.
Major Arc, ABC
Minor Arc, AC
B
G
Semi-Circle, AED
B
D
Arc Addition
95°
E
G
H
C
65°
C
F
A
A
A
Greater than 180°
Less than 180°
Match the following terms.
a. AC Radius
Equal to 180°
f. CB
Tangent
b. DE
Diameter
g. Point A
Center
c. CD
Chord
h. Point G
Exterior Point
mFG + mGH = mFH
65° + 95° = 160°
G
C
E
A
d. DF
Secant
i. DEF
Major Arc
e. CD
Minor Arc
j. DCE
Semi-Circle
B
D
F
Circles A and D have radii of 4 cm & 1 cm respectively. Use this
information to determine the missing values.
C
G
a) BF = 8 cm because it is a diameter 2r = 2(4)
b) AD = 5 cm because it is the sum of the two radii.
c) CD = 3 cm because
42
+
x2
=
B
52
F
A
D
E
d) CG = 2 cm because 3 – 1 = 2
e) Perimeter of ∆ACD = 3 + 4 + 5 = 12 cm
Draw the following relationships.
a) Secant line AB intersects ⊙ M
at points A and B .
b) Secant line MN intersects
tangent line TM on Circle R.
N
A
M
A
G
T
B
c) Diameter AB intersects tangent
line GB on circle M.
M
R
M
B
G.C.2 WORKSHEET #1
Name: ________________________________ Period ______
1
1. Match the following for Circle A (use each item once).
a. _____ Major Arc
f. _____ Interior Point
1. EG
6. Point I
b. _____ Diameter
g. _____ Secant line
2. Point H 7. FC
c. _____ Chord
h. _____ Exterior Point
3. GE
d. _____ Minor Arc
i. _____ Center
e. _____ Tangent line
j. _____ Semi-Circle
J
B
C
8. CBF
A
I
G
4. Point A 9. CEG
5. FD
H
D
E
F
10. IJ
2. Using the diagram in question #1, name objects that meet the description.
a. Chords
______ , ______, ______, ______
b. Radii ______ , ______, ______, ______
c. Central ∠
__________ , __________ , __________
d. Exterior Points ______ , ______
3. Jeff was a little confused by the lesson about the circle basics. When he looked back at his notes he had
written down that radii and diameters of circles are not chords. Is this correct? Explain.
4. A textbook had the following true and false question.
Two radii always form a diameter.
T or F
The answer is false.
a) Can you find the counter example to this statement to establish it be false.
b) Most of students put true. What makes this statement confusing?
5. Circles B and C are congruent. Circle B has a radius of 4 cm and ∠AEC
is a right angle. Use this information to determine the missing values.
a) BC = _________
b) AD = _________
E
A
B
C
c) Perimeter of Quad. BECF = _________
d) AC = _________
e) AE = ___________
F
f) Perimeter of ∆BED = _________
6. After completing this question a very observant students states, “Hey ∆AEC must be a special right
triangle, 30°°-60°°-90°°.” She is correct, what did she see that helped her come to this conclusion?
D
G.C.2 WORKSHEET #1
2
7. Secant GE goes through Circle F’s center and intersects at points G and E.
a) What is the name of the diameter formed by the secant? __________
b) Name the two radii formed by the secant. ________, _______.
8. Circles B and E have radii of 8 cm & 6 cm respectively, and CD
is 3 cm. Use this information to determine the missing values.
G
a) BC = _________
b) EA = _________
A
B
c) Perimeter of Quad. BGEH = _________
C
D
E
F
H
d) AF = _________
e) Perimeter of ∆BGE = _________
9. Draw the following relationships.
a) Tangent line GE has a point of
tangency at Point F on Circle M.
b) Secant line HT intersects
tangent line JT on Circle R.
c) Radius AB intersects tangent
line GE on circle A.
10. Determine the radius of the circle.
a) Area = 36π r = _________
d) r = _________
b) C = 10π
r = _________
e) r = _________
c) d = 7 cm
r = _________
f) r = _________ (E)
12 2 cm
5 cm
12 cm
6 cm
Square inscribed
g) r = _________
A regular hexagon with
a side of 8 cm
h) r = _________
A regular equilateral ∆ with an
apothem of 5 cm.
i) r = _________ (E)
A regular hexagon with an
apothem of 21 cm
Name: \/'t-tx.
G.C.2 WORKSHEET #7
period
1. Match the following for Circle A (use each item once).
I
a. 0
b. 1
c. I
d. {
Major
Arc
t.
g._
h._
i._
j._
Diameter
Chord
Minor Arc
e. /D Tangent line
2.
lnterior point
L.
Secant line
2. Point
Exterior Point
3,
Center
EG
s.
H
GE
4. Point
Semi-Circle
6. Point
fr
I
7. FC
8, CBF
-
A g. m
Lo.7i
Using the diagram in question #1, name objects that meet the description.
fuz , --_-__, ,+U) ,
d. Exterior points
, T
a. Chords
c. Central
b.
, lC*D
Z
,
_
Radii
little confused by the lesson about the circle basics. When he looked back at his notes he had
written down that radii and diameters of circles are not chords. ls this correct? Exptain.
Aadi; aulz- rto F de-.{.e"
d;a,rw- 'le-< yCI^DJS )..tt<botq- e*l&4>r,nk ck^tt) exa- C,vr)a-"
4. A textbook had the following true and false question.
3. Jeff was a
@
, o@
Two radii always form a diameter.
The answer is false.
a) Can you find the counter example to this statement to establish it be false.
?.e-sz-
*r'a r.-til do 9-t- G*^' a'- {;c"r^-Tv-
b) Most of students put true. what makes this statement confusing?
5. circles B and c are congruent. circle B has a radius of 4 cm and IAEC
is a right angle. use this information to determine the missing vatues.
a) BC
= \"*
b)AD=
c) Perimeter of Quad. BECF
d)AC
= ffCu
f) Perimeter of ABED
=
fb cuo
e)AE =
=
I
t tqG c*,
6. After completing this question
a very observant students states, "Hey AAEC must be a speciat right
triangle, 30o-60o-90o." she is correct, what did she see that helped her come to this conclusion?
G.C.2 WORKSHEET #7
7. Secant
E
2
goes through Circle F's center and intersects at points G and
a) What is
E.
the name of the diameter formed by the secant?
b) Name the two radii formed by the
secant. f 6
8. Circles B and E have radii of 8 cm & G cm respectively, and CD
is 3 cm. Use this information to determine the missing values.
b)rR=
a)BC=
c) Perimeter of Quad. BGEH
d)nr=
= /6 ctw
2{cx
e) Perimeter of ABGE =
9. Draw the following relationships.
E
of
M.
a)Tangent line
has a point
tangency at Point F on Circle
Ei intersects
tangent line V on Circle R.
b) secant line
c) Radius
line
dE
lB
intersects tangent
on circle A.
10. Determine the radius of the circle.
a)Area=36n
d)
r= b.x
b)C=L0n
Qr=
r=
tz.ll c-
r=
3.{cux
c)d=7cm
6.{c*
f) r=
7-+,
+'t7
5
.Y-
15 =Y
Square inscribed
8)r=
{cu
A regular hexagon with
\N/asideor8cm
h)
r=
A regular equilateral A with an
apothem of 5 cm.
ir= *l 6
(E)
A regular hexagon with an
apothem of 2L cm
© Copyright 2025