Answer
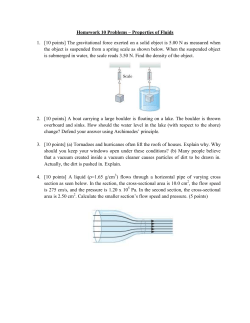
THE SCIENCE OF MUSIC (PC1327/GEK1519) Mid-Term Class Test, Semester 2, 2014/15 This is an open book test. The test is one hour long. Give your answers to ALL 25 questions on the computer-readable sheet provided, using a soft (2B) pencil to shade the appropriate choice for each question. 1. Which of the following is the most appropriate example of a scientific activity? (a) A physicist playing a Schubert sonata on a new kind of electronic piano using physics principles to generate sound. (b) A chemist playing a Mozart concerto on a violin which has just been varnished with a new chemical formulation for violin varnish. (c) A timpani (tuned orchestral kettledrum) player investigating how different materials for the drum skin affect the loudness of the drum. (d) An organist playing a Bach chorale prelude on a pipe organ which has a set of pipes made of a new type of metal alloy. Answer: (c) The physicist, chemist and organist are all performing essentially musical activities. The timpani player is the only one performing an essentially scientific activity. 2. Which of the following is the least appropriate example of a technological activity? (a) An oboe player inventing a new machine which produces oboe reeds for her instrument. (b) A guitarist devising a new type of guitar which is foldable and compact enough to be carried in her handbag. (c) A bassoon player inventing a new type of bassoon which has a pitch range going much higher than a normal bassoon. (d) An electrical engineer playing a harp concerto on a harp which has a new type of pedal mechanism. Answer: (d) The oboe player, guitarist and bassoon player are all performing essentially technological activities. The electrical engineer is the only one performing an essentially musical activity. 3. Which of the following objects is the least appropriate example of an object which is undergoing a vibration? (a) (b) (c) (d) A woman’s head nodding up and down repeatedly during a lecture. A block of wood sliding slowly to a stop on a newly polished floor. The pendulum of a wall clock swinging from side to side repeatedly. A little girl jumping up and down repeatedly to try to reach a bar of chocolate on a shelf. Answer: (b) The woman’s head, pendulum and little girl are each undergoing a repetitive movement and hence can be said to be undergoing a vibration. The block of wood is undergoing a single motion and therefore cannot be said to be undergoing a vibration. 4. A coconut tree and a cherry tree next to each other in a park are each swaying from side to side repeatedly during a thunderstorm. The cherry tree undergoes 6 complete cycles during the same duration in which the coconut tree undergoes 5 complete cycles. If the cherry tree undergoes 4 complete cycles in 9 seconds, what is the time duration in which the coconut tree would undergo 7 complete cycles? 1 (a) (b) 189 seconds. 10 189 seconds. 20 56 seconds. 15 (c) (d) None of the above. Answer: (a) The cherry tree undergoes 4 cycles in 9 seconds, and hence the time taken by one cycle is given by 94 seconds. The time taken by the cherry tree to undergo 6 cycles is given by 94 seconds times 6 cycles i.e. 54 seconds. In this same duration, the coconut tree undergoes 4 seconds divided by 5 i.e. 54 seconds. 5 cycles so the duration of each of its cycles is equal to 54 4 20 Therefore the time duration in which the coconut tree completes 7 cycles is equal to 54 seconds 20 189 378 times 7 i.e. 20 seconds, which can be simplified to 10 seconds. 5. A jewellery designer draws a design for a brooch, which is then given to a jewellery craftsman to make the brooch. A young man sees the completed brooch in a jewellery shop and buys it for his girlfriend, who then wears the brooch. A songwriter writes the musical score of a new song, and a pop singer reads the musical score and performs the song at a pop concert at the Indoor Stadium. A very enthusiastic audience enjoys the song and cheers the singer. Which of the following has the same relationship to the songwriter as the jewellery craftsman has to the jewellery designer? (a) (b) (c) (d) The The The The musical score. pop singer. audience. song produced by the singer. Answer: (b) The singer reads the musical score created by the songwriter and performs the song, thus bringing the song into existence as musical sound by singing it. The jewellery craftsman follows the plans of the jewellery designer and brings the brooch into existence by making the brooch. Hence the pop singer has the same relationship to the songwriter as the jewellery craftsman has to the jewellery designer. 6. A children’s choir is having a rehearsal for a concert to celebrate Singapore’s 50th Anniversary. They start singing the song “Stand Up for Singapore”, just as a child who is a choir member arrives late for the rehearsal. In order not to produce counterpoint, which of the following songs should the latecomer sing as she enters the rehearsal room? (a) (b) (c) (d) “Happy Birthday”. “We are Singapore”. “Count on Me Singapore”. “Stand Up for Singapore”. Answer: (d) In order not to produce counterpoint, the latecomer should sing the same melody as the one which the other choir members are singing, and hence she should also sing “Stand Up for Singapore”. 7. You are in the audience of a concert to celebrate Singapore’s 50th Anniversary, and a band which starts the concert registers a reading of 93 dB on a sound level meter which you are holding in your hand. The next musical item in the concert is a choir which produces 100 times less sound power reaching your sound level meter as compared to that from the band. What is the reading on the sound level meter? (Assume that the sound level meter readings are due only to the band and the choir.) 2 (a) (b) (c) (d) 73 dB. 63 dB. 83 dB. None of the above. Answer: (a) Since a decrease in sound power of 10 times results in a decrease in the sound level meter reading of 10 dB, a decrease in sound power of 100 times, i.e. a decrease of 10 times 10 times in sound power, would result in a decrease in the sound level meter reading of 10 dB plus 10 dB i.e. 20 dB. The sound level meter reading when the choir is performing should be 20 dB less than the reading of 93 dB for the band. Therefore the sound level meter reading will be given by 93 dB minus 20 dB i.e. 73 dB. 8. During a symphony orchestra performance, a violin plays a musical note which has a frequency of 2,640 Hz. A trombone then plays a musical note which has a frequency of 55 Hz. Which of the following correctly describes the number of complete octaves between the note played by the trombone and the note played by the violin? (a) (b) (c) (d) Greater Greater Greater Greater than than than than 7 6 5 4 complete complete complete complete octaves octaves octaves octaves but but but but less less less less than than than than 8 7 6 5 complete complete complete complete octaves. octaves. octaves. octaves. Answer: (c) The interval of an octave has a ratio of 12 i.e. 2, which means that we will go up by the interval of an octave by multiplying the frequency by 2. If we start with the bassoon note’s frequency of 55 Hz, successively multiplying by 2 results in the following frequencies: 55 Hz, 110 Hz, 220 Hz, 440 Hz, 880 Hz, 1,760 Hz and finally 3,520 Hz after 6 multiplications. Since 3,520 Hz is greater than the violin note’s frequency of 2,640 Hz, the interval from 55 Hz to 2,640 Hz is greater than 5 complete octaves but less than 6 complete octaves. 9. The A string just above Middle C of a piano which is tuned to Equal-tempered tuning is tuned to a frequency of 442 Hz. A certain note on this piano has a frequency of approximately 1,403.28 Hz. Of the following notes on the piano, which one is most likely to be the note having this frequency? (Take the ratio of an Equal-tempered semitone to be equal to 1.05946 for your calculations.) (a) (b) (c) (d) G6. Fsharp6. F6. None of the above. Answer: (c) The A string just above Middle C is the note A4, and since this has the frequency 442 Hz, the note A6 two octaves higher on this piano has a frequency equal to 442 Hz multiplied by 2 twice i.e. 1,768 Hz. Dividing 1,768 Hz by the ratio of an Equal-tempered semitone or by 1.05946 to go down by an Equal-tempered semitone, a frequency of approximately 1,668.775 is obtained, and dividing by 1.05946 another three times, we will arrive at a frequency of approximately 1,403.279 Hz. Hence the frequency is 4 Equal-tempered semitones below the note A6 on this piano, and is thus the note F6. 10. A melody for bassoon has a musical score which has a time signature of 17/8 at its beginning. A particular bar of this melody begins with a crotchet rest and ends with a dotted quaver and 5 semiquavers. Which of the following combinations of notes would fit exactly into the middle of this bar? 3 (a) (b) (c) (d) 5 quavers, 5 semiquavers and a minim. 2 dotted crotchets, a quaver and 8 semiquavers. A dotted minim and 9 semiquavers. None of the above. Answer: (b) Since the musical score has a time signature of 17/8, every bar of the melody has to contain the duration equivalent of 17 quavers or 34 semiquavers. At the start of the bar is a crotchet rest which is equivalent to 4 semiquavers, and at the end are a dotted quaver which is equivalent to 3 semiquavers and 5 semiquavers, making a total of 12 semiquavers in all. Therefore the middle of the bar needs another 22 semiquavers to make up 34 semiquavers. Two dotted crotchets are equivalent to 12 semiquavers and together with a quaver (or 2 semiquavers) and 8 semiquavers, will make up a total of 22 semiquavers. 11. Starting from the note A5 on the keyboard of a standard grand piano, you move downwards by one and one-third octaves to arrive at a second note. You then start from this second note and you move upwards by one and one-sixth octaves to arrive at a third note. You then start from this third note and move downwards by two and one-quarter octaves to arrive at the fourth and final note. What is the correct letter name of this fourth note? (a) (b) (c) (d) E4. F3. E3. None of the above. Answer: (c) Going down by an octave from A5, you reach A4 and as an octave consists of 12 semitones, going down by one-third of an octave is a movement downwards by 4 semitones to arrive at F4. From F4, going up by an octave arrives at the note F5, and up by one-sixth of an octave or 2 semitones reaches the note G5. From G5, going down by two octaves you arrive at the note G3, and a further movement downwards by one-quarter of an octave or 3 semitones means that the fourth note on which you finally arrive is E3. 12. A piece of music for solo trumpet has a musical score consisting of a single musical staff which has a treble clef at its beginning. The first note of a certain bar of this piece is written on the middle line of the five lines of the staff, the second note is written on the second highest space of the four spaces of the staff, the third note is written on the lowest space of the staff, and the fourth note is written on the highest line of the staff. Which of the following gives the letter names in the right order of these four notes, from the first note to the fourth note? (a) (b) (c) (d) B4, C5, F4 and F5. B4, D5, F4 and F5. B4, C5, E4 and F5. None of the above. Answer: (a) The treble clef indicates that the second lowest line of the staff is the note G4. The note on the middle line of the five lines is thus the note B4, the note on the second highest space of the four spaces is the note C5, the note on the lowest space must be the note F4, and the note on the highest line must be the note F5. 13. A piano which is tuned normally in agreement with the Equal-tempered scale has its A4 note tuned to a frequency of 440 Hz. The frequency of the A string of a ’cello is exactly one octave below the frequency of the piano’s A4 string, and all the four strings of the ’cello are tuned 4 relative to each other in Just fifths. Which of the following is closest to the ratio of the interval between the frequency of the note from the open G string of the ’cello and the frequency of the C3 note of the piano? Open string means that the notes are not played with a finger on the ’cello’s fingerboard, i.e. with the full length of the respective string vibrating. (Take the ratio of an Equal-tempered semitone to be equal to 1.05946 for your calculations.) (a) (b) (c) (d) 1.338. 1.417. 2.835. 5.670. Answer: (a) The note C3 on the piano is three Equal-tempered semitones above the frequency of its A2 note, which is two octaves below the A4 note and hence has a frequency given by 440 Hz divided by 2 twice i.e. 110 Hz. The frequency of the C3 note is thus given by 110 Hz times 1.05946 three times i.e. approximately 130.81 Hz. The G string of the ’cello has a frequency two Just fifths below its A string which has a frequency of 220 Hz. The frequency of the G string is thus given by 220 Hz divided by 32 twice, which is the same as 220 Hz times 32 twice i.e. approximately 97.78 Hz. The interval from 97.78 Hz to 130.81 Hz is thus equal to 130.81 Hz divided by 97.78 Hz i.e. approximately 1.338. 14. Starting from a first note, you go up by an interval of an octave and a Just sixth and arrive at a second note. From this second note, you then go down by the interval of two octaves and a Pythagorean third to arrive at a third note. From this third note, you then go up by a Just seventh to arrive at a fourth note. Which of the following gives the ratio of the interval between the first note and the fourth note? (a) 54 . (b) 200 . 81 100 . 81 (c) (d) None of the above. Answer: (c) The ratio of a Just sixth is 53 , so going up an octave and a Just sixth, we first 81 multiply the frequency by 2 and then multiply by 53 . The ratio of a Pythagorean third is 64 so going down by two octaves and a Pythagorean third means dividing by 4 and then dividing by 81 . Finally, going up by a Just seventh which has the ratio 15 means multiplying by 15 . Thus 64 8 8 5 we can calculate the ratio from the first to the fourth note by mulyiplying 2 times 3 times 14 64 times 15 which gives 4,800 which can be reduced to 100 . times 81 8 3,888 81 15. A string which is 240 cm long vibrates at a frequency of 1,422 Hz when you place your finger at a certain distance from one end of the string. If the string has a fundamental frequency of 237 Hz, how far is your finger from the nearer end of the string? (a) (b) (c) (d) 40 cm. 50 cm. 60 cm. None of the above. Answer: (a) The fundamental frequency of the string is 237 Hz and since 1,422 Hz divided by 237 Hz is equal to 6, the string must be vibrating at its 6th harmonic. Your finger must therefore be one-sixth of the string’s length from the nearer end, which means the distance from the nearer end is given by 240 cm divided by 6 i.e. 40 cm. 5 16. A string which has 8 antinodes between its two ends is vibrating at a frequency of 1,760 Hz. A second string 110 cm long is vibrating at a frequency of 1,440 Hz. If the first string is 80 cm long, how many nodes are there between the two ends of the second string, not counting the nodes at both ends? (Assume that the two strings are identical in all respects except length.) (a) (b) (c) (d) 7 nodes. 8 nodes. 9 nodes. None of the above. Answer: (b) The first string has 8 antinodes and is thus at its 8th harmonic, and hence its fundamental frequency is given by 1,760 Hz divided by 8 i.e. 220 Hz. The fundamental 80 i.e. 160 Hz. Since the second frequency of the second string is thus equal to 220 Hz times 110 string is vibrating at 1,440 Hz, it must be at its 9th harmonic as 160 Hz times 9 is equal to 1,440 Hz. Hence the second string has 9 antinodes and 8 nodes between its two ends. 17. A string which is 75 cm long is vibrating with 5 nodes between its two ends (not counting the nodes at both ends) at a frequency of 780 Hz. A second string of length 65 cm is also vibrating with a number of nodes and antinodes between its two ends at a frequency of 750 Hz. What is the distance between the adjacent nodes on the second string? (Assume that the two strings are identical in all respects except length.) (a) (b) (c) (d) 14 cm. 15 cm. 16.25 cm. None of the above. Answer: (d) Since the first string has 5 nodes, it will have 6 antinodes and will thus be at its sixth harmonic. The fundamental frequency of the first string is equal to 780 Hz divided by 6 i.e. 130 Hz. The fundamental frequency of the second string is therefore given by 130 Hz times 75 i.e. 150 Hz. Since the second string is vibrating with a frequency of 750 Hz, and since 65 750 Hz divided by 150 Hz is equal to 5, it must be vibrating at its 5th harmonic. The distance between adjacent nodes on the second string is one-fifth of its length or 65 cm divided by 5 i.e. 13 cm. 18. A string which is labelled A has 3 nodes between its two ends (not counting the nodes at both ends) and is vibrating at a frequency of 1,280 Hz. A second string labelled B is 25% longer than the string A, and is sliced into 7 pieces of equal length. 4 of these 7 pieces are joined up to make a third string labelled C. When C is vibrating with 6 nodes between its two ends (not counting the nodes at both ends), what is its frequency of vibration? (Assume that the strings are identical in all respects except length.) (a) (b) (c) (d) 2,688 Hz. 3,136 Hz. 3,584 Hz. None of the above. Answer: (b) Since the string A has 3 nodes it has 4 antinodes and is at its 4th harmonic, which means that its fundamental frequency is given by 1,280 Hz divided by 4 i.e. 320 Hz. As the string B is 25% longer than A, its fundamental frequency must be given by 320 Hz 1 times 1.25 i.e. 256 Hz. The string C is four-sevenths the length of B, which means that its 6 fundamental frequency is given by 256 Hz times 47 i.e. 448 Hz. If C has 6 nodes, it will have 7 antinodes and will be at its 7th harmonic, and its frequency will be given by 448 Hz times 7 i.e. 3,136 Hz 19. An open pipe labelled A which has 5 antinodes between its two ends (not counting the antinodes at both ends) is vibrating with the same frequency as a closed pipe labelled B which is vibrating with 3 nodes between its two ends (not counting the node at one end). The closed pipe B is sliced to create 4 short open pipes and one short closed pipe, all of equal length. Two of the short open pipes and the short closed pipe are joined up to make a closed pipe labelled C. If the fundamental frequency of the open pipe A is 140 Hz, what is the frequency of the closed pipe C as it vibrates with 4 nodes between its two ends (not counting the node at one end)? (a) 800 Hz. (b) 1,400 Hz. (c) 2,200 Hz. (d) None of the above. Answer: (d) The open pipe A has 5 antinodes and hence has 6 nodes, and is thus at its 6th harmonic, and its frequency is given by 140 Hz times 6 i.e. 840 Hz. The closed pipe B has 3 nodes and is at its 7th harmonic, and its fundamental frequency is thus given by 840 Hz divided by 7 i.e. 120 Hz. The closed pipe C is three-fifths the length of the closed pipe B, and hence its fundamental frequency is equal to 120 Hz times 53 i.e. 200 Hz. When the closed pipe C vibrates with 4 nodes, it is at its 9th harmonic and its frequency is therefore given by 200 Hz times 9 i.e. 1,800 Hz. 20. A closed pipe is vibrating to produce a musical note which has a spectrum showing that all the harmonics of the musical note produced are present up to its 21st harmonic. A vibrating string which has a fundamental frequency of 112 Hz produces a note which also shows its fundamental frequency and all its harmonics, odd and even, up to its 21st harmonic. The frequency of the 4th line from the left in the spectrum of the closed pipe’s note is the same as that of the 8th line from the left in the spectrum of the note from the string. Calculate the frequency of the 11th line from the left in the spectrum of the closed pipe’s note. (Assume that the frequencies in each spectrum increase from left to right.) (a) 2,688 Hz. (b) 2,432 Hz. (c) 1,408 Hz. (d) None of the above. Answer: (a) Since the 8th line in the spectrum of the string’s note is its 8th harmonic, its frequency is given by 112 Hz times 8 i.e. 896 Hz. The 4th line from the left in the spectrum of the closed pipe’s note is its 7th harmonic which also has a frequency of 896 Hz, so the fundamental frequency of the closed pipe must be equal to 896 Hz divided by 7 i.e. 128 Hz. Since the 11th line in the spectrum of the closed pipe’s note is its 21st harmonic, its frequency must be equal to 128 Hz times 21 i.e. 2,688 Hz. 21. A recently discovered ethnic musical instrument plays a musical note which has a spectrum showing that all its harmonics (even and odd) are present up to its 13th harmonic. A square wave with a fundamental frequency of 270 Hz has a spectrum which also shows all its harmonics up to the 13th harmonic. If the 6th line from the left in the square wave note’s spectrum has the same frequency as the 9th line from the left in the spectrum of the note from the musical 7 instrument, what is the frequency of the 12th line from the left in the spectrum of the musical instrument’s note? (Assume that the frequencies in each spectrum increase from left to right.) (a) 2,160 Hz. (b) 3,960 Hz. (c) 4,290 Hz. (d) None of the above. Answer: (b) Since the square wave has only odd harmonics, the 6th line from the left in the square wave’s spectrum is its 11th harmonic, whose frequency must be given by 270 Hz times 11 i.e. 2,970 Hz. The 9th line from the left in the spectrum of the musical instrument’s note is its 9th harmonic as all its harmonics are present, and hence the fundamental frequency of the musical instrument’s note is equal to 2,970 Hz divided by 9 i.e. 330 Hz. The 12th line from the left in its spectrum must be its 12th harmonic whose frequency is equal to 330 Hz times 12 i.e. 3,960 Hz. 22. An open pipe labelled J is sliced into 7 pieces to make 7 short open pipes all of equal length. 5 of these short open pipes are joined up and one end is closed up to make a closed pipe labelled K. The other two short open pipes are joined to make an open pipe labelled L. Calculate the ratio of the interval between the frequency of the open pipe L when it vibrates with 3 nodes between its two ends, and the frequency of the closed pipe K when it vibrates with 6 nodes between its two ends (not counting the node at one end). (a) (b) 5 2 15 . 13 30 . 13 (c) (d) None of the above. Answer: (b) Let the frequency of the open pipe J be f Hz. The closed pipe labelled K is therefore five-sevenths the length of J and an open pipe of this length has a fundamental frequency equal to f Hz times 75 i.e. 7f5 Hz. The closed pipe K will have a fundamental frequency half of this i.e. 7f Hz. When K vibrates with 6 nodes it is at its 13th harmonic, and 10 Hz times 13 i.e. 91f Hz. The open pipe L is two-sevenths the its frequency will be equal to 7f 10 10 length of J, and hence its fundamental frequency is equal to f Hz times 27 i.e. 7f2 Hz. When L has 3 nodes it will be at its 3rd harmonic and its frequency will be equal to 7f2 Hz times 3 i.e. 21f Hz. The interval from 91f Hz to 21f Hz is given by 21f Hz divided by 91f Hz which is the 2 10 2 2 10 21f 10 210 15 same as 2 Hz times 91f Hz i.e. 182 . This ratio can be simplified to 13 . 23. Give the correct sequence of the following pipes arranged in order of increasing frequency. (i) The sixth harmonic frequency of an open pipe of length h cm. (ii) The eighth harmonic frequency of an open pipe of length 9h cm. 7 3h (iii) The fifth harmonic frequency of a closed pipe of length 7 cm. (iv) The ninth harmonic frequency of a closed pipe of length 5h cm. 7 (a) (i), (iii), (ii), (iv). (b) (iii), (i), (ii), (iv). (c) (iii), (i), (iv), (ii). (d) None of the above. 8 Answer: (b) Let f Hz be the fundamental frequency of an open pipe of length h cm. Its sixth harmonic frequency is therefore equal to 6f Hz. The fundamental frequency of an open pipe cm is given by f Hz times 7h i.e. 7f9 Hz, and its eighth harmonic has a frequency of length 9h 7 9h 7f 56f given by 9 Hz times 8 i.e. 9 Hz. An open pipe which has a length of 3h cm will have a 7 7f 7h fundamental frequency equal to f Hz times 3h i.e. 3 Hz. Therefore a closed pipe of the same length 3h cm will have a fundamental frequency half of 7f3 Hz i.e. 7f6 Hz. Its fifth harmonic 7 frequency is thus equal to 7f6 Hz times 5 i.e. 35f Hz. Finally a closed pipe which is 5h cm long 6 7 7f 21f 3h 5h has a fundamental frequency given by 6 Hz times 7 divided by 7 i.e. 30 Hz which can be simplified to 7f Hz. Its ninth harmonic frequency is equal to 7f Hz times 9 i.e. 63f Hz. The 10 10 10 pipes in order of increasing frequency are therefore (iii), (i), (ii) and (iv). 24. A ’cello has its strings correctly tuned to Just fifths with its A string at a frequency of 220 Hz. A ’cellist plays a musical note on the ’cello during a concert, producing a sound wave which travels from her ’cello towards the listeners at a speed of 330 metres per second. If the wavelength of the sound wave is 4 metres, which of the following is the most likely musical note being played? (Assume the notes played by the ’cello are part of the Just or Pythagorean scale.) (a) (b) (c) (d) D2. E2. A1. None of the above. Answer: (b) If the velocity of sound is 330 metres per second, the frequency of a note with a wavelength of 4 metres is equal to 330 metres per second divided by 4 metres i.e. 82.5 Hz. If we go down from this note by a Just fifth, we divide 82.5 Hz by 32 which is the same as multiplying it by 32 to obtain the frequency 55 Hz. Since 55 Hz multiplied by 4 is equal to 220 Hz which is the frequency of the A3 string on the ’cello, it is the note A1, and the note which is a Just fifth above A1 must be the note E2. 25. You are standing some distance from a long straight wall, and a policeman is standing between you and the wall such that the line from you to the policeman is perpendicular to the wall. The policeman fires a single shot from his pistol, and just 0.58 seconds after you see the flash of the pistol shot, you hear the echo of the pistol shot from the wall. If the distance between the policeman and the wall is 74.25 metres, how many seconds after you see the flash of the pistol shot would you have heard the direct sound of the shot? (Assume that sound travels at a speed of 330 metres per second, and that you see the pistol’s flash at the same time as when the pistol is fired.) (a) (b) (c) (d) 0.45 seconds. 0.225 seconds. 0.29 seconds. None of the above. Answer: (d) The echo from the pistol shot would have travelled from the policeman to the wall, then reflected from the wall and travelled back to the policeman and then travelled to you. Since the echo took 0.58 seconds to reach you after the flash, the total distance travelled by the echo is equal to 330 metres per second times 0.58 seconds i.e. 191.4 metres. If the policeman is 74.25 metres from the wall, the distance travelled by the echo from the pistol to the wall and back again to the policeman would be equal to 74.25 metres times 2 i.e. 148.5 9 metres. Therefore the distance between you and the policeman is given by 191.4 metres minus 148.5 metres i.e. 42.9 metres. This is the distance travelled by the direct sound of the shot, and the time taken for it to reach you would thus be equal to 42.9 metres divided by 330 metres per second i.e. 0.13 seconds. END OF TEST PAPER 10
© Copyright 2025