1. art and entertainment
2. visual art and design
3. design

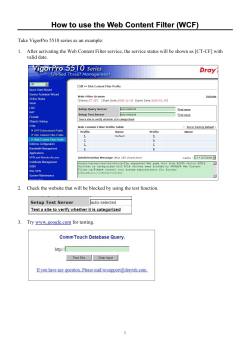
IEEE TRANSACTIONS
ON MICROWAVE
THEORY AND TECHNIQUES,
HairPin-Line
VOL. MTT-20,
and Hybrid
NO. 11, NOVEMBER
Abstract—A
new
class
hybrid
hairpin-line/half-wave
ported.
This
class
and TEM
printed-circuit
is generally
into
its input
filter
realizations
output
filters,
is particularly
not
two types.
and
microwave
first
to yield
at their
FRANKEL
is
have
I
re-
~[
been
INPUT
OUTPUT
by having
ends.
impedance
SIDNEY
of the filt er
filters
A) is characterized
practical
Filters
for microstrip
grounding
Half-Wave
and
filters,
Hairpin-line
(Type
AND
hairpin-line
suited
because
required.
The
well
limes open-circuited
has been found
CRISTAL
parallel-coupled-line
of filters
resonators
divided
of
G.
719
HairF)in-Line/
Parallel-Coupled-Line
EDWARD
1972
The
levels
Type
(a)
A
for narrow
+
to
approximately
25-percent
bandwidths.
The second (Type B) is char.
acterized
by having its input and output lines short-circuited
at their
ends.
However,
filter
are not
design
because
equations
mental
data
of space
presented
in this
for Type
for
several
widths
are given.
circuit
(MIC)
stripline
details
Theoretical
A bandpass
Experimental
filters
limitations,
paper.
filters
filters
results
of the
Type
background
are presented.
of 5- and
B
and
INPUT
Experi-
20-percent
band-
for two microwave-integrated-
Fig.
of
USE
stripline
grated-circuit
T
tems
methods
costs,
ticularly
and
systems
ground,
since
most
filter
filter
filters
and
unavailable.
present
The
an
filters
narrow
data
for
The
the
the above
image
reported
[1].
for
to
is awkward
A.
(b) Type
B.
of the
for
filters
present
theory
filter
for
3)
to
1) to
and
hybrid
circuits
have
for
at the
hairpin-line/
constant
been
several
for
Type
y
for
the
and
filters
These
circuits
negligible
(i.e.,
of
are
used
and
neighbors
based
difficult
a
2(a)
on the
the
sparse
assumption
tlhat
neighbors
is
assumption).
geometries
than
beyond
capacitance-matrix
using
be
the
the
differs
usual
one
nearest
assunnp-
Physically,
terms
of’ the basic
definitions
of the coupling
parameters.
case of the
admittance
(capacitance)
parameters,
the
can
the
respec-
assumption
to satisfy,
difference
by
(b),
tion).
In
the
sche-
Equivalent
derived
nearest
this
coupling
exact
reported.
shown
and
inductance-matrix
capacitive
(i.e.,
are
been
Fig.
arrays,
is more
negligible
1 have
microwave-filter
coupled-line
been
respectively.
in
beyond
a sparse
commonly
from,
of Fig.
were
How-
neither
have
filters
(b),
presented
coupling
parallel.
and
line,
[2].
filters,
equations
B hairpin-line
1 (a)
are
inductive
For
Manuscript
received
November
8, 1971; revised
June 8, 1972.
This work was conducted
at the Stanford
Research
Institute,
Menlo
Park, Calif. 94025, and supported
principally
by the U. S. Army
Electronics
Command
Laboratories
under Contract
DAAB07-70-C0044. The revised paper was financially
supported
by the National
Research Council of Canada under Grant A8242.
E. G. Cristal
was with the Stanford
Research
Institute,
Menlo
Park, Calif. 94025. He is now with the Department
of Electrical
Engineering
and the Communications
Research
Laboratory,
MclMaster University,
Hamilton,
Ont., Canada.
S. Frankel
is at P.O. Box 126, Menlo Park, Calif. 94025.
A and
circuits
A hairpin
6 Conference
hairpin-line
design
y in Fig.
authors
the Type
Region
finite-length
matically
tively.
previously
periodical
for
including
IEEE
approximate
The
experimental
propagation
line
lines,
discussed
nor
filters.
hairpin
terminated
ever,
of very
2) to present
present
and
are
.
been
hairpin-line
bandwidths
Equivalent
circuits
for hairpin-line
filters.
C; represents
the
2.
open-circuited
transmission
line of chara~ter@tic
impedance
C,.
Li represents
the short-circuited
transmission
hne of characteristic
imped mce L,. UE represents
the umt element
of character
stic
impedance
Z~,i+l. (a) Equivalent
circuit
for hairpin-line
filter of
Fig. 1 la). (b) Equivalent
circuit for hairpin-line
filter of Fig. 1(b)
to date,
have
paper
20 to 25 percent;
and
(b)
Fig.
Hairpin-line
although,
these
design
impedance
Equivalent
pre-
parallel-coupled-
hairpin-line
periodic
C would
connections
condition.
parallel-coupled-line
infinite
(a) Type
,N:T)l,fm...+jlliuTuT
is par-
transmission-line
condition,
purposes
equations;
several
or MI
size,
Circuits
half-wave
equations
to approximately
half-wave
lbl IC
circuits.
connections
above
is satisfactory
design
filters.
(a)
to
numerous
the
approximate
that
filter
the
the
design
respect
requiring
of such
available,
also satisfy
theory
not
sys-
manufacturing
with
in stripline
Among
microwave
other
integrated
realization
satisfies
to
reproducibility.
hybrid
geometries
cases.
designs
line
usually
in
in
advantages
constructed
utilize
Hairpin-line
‘N;I!!tdmT”’~
”’pu’
microwave-inte-
designs
preferred
of
and
useful
ferably
in
often
because
weight,
and/or
(N!I I C)
is
1.
are discussed.
1. INTRODUCTION
HE
OUTPUT
(b)
understood
in
IEEE TRANSACTIONS ON MICROWAVE
720
lr4PuT—
‘“’”’~
2
while
.
six
.
Three
.
.
.
3.
filter
of Fig.
half-wave
4(b)
consists
NOVEMBER
of one
1972
hairpin
and
resonators.
.
.
Fig.
the
THEORY AND TECHNIQUES,
.
of the
design
1701ding procedure
forobtainillg
ahairpiIl-lille
from a half-wave
parallel-coupled-line
filter.
filter
lows.
line
theory
1) In the limiting
case
filters),
the
go from
[5].
all
OUTPUT
INPUT ~
manner.
3)
stituting
hairpin
and
be individually
may
The
the
half-wave
between
resonators
paralleland
pairs
may
previ-
designer
hairpin-line/half-
all
a straightforward
coupling
as fol-
those
allows
hybrid
to
in
with
theory
to
are
parallel-coupled-
are identical
hairpin-line
approximate
herein
(half-wave
2) The
designs
of the
presented
parallel-coupled-line
coupled-line
(a)
equations
published
wave
[
—
advantages
and
ously
to
principal
equations
be
adjusted
of
chosen
at
simple
lines
con-
arbitrarily
any
point
in
the
design.
OUTPUT
In the
—
(b)
Fig.4.
Examples
of hybrid
parallel-coupled-line
hairpin-line/half-wave
filters.
nearest
tween
neighbors
a conductor
act
as partial
the
next-nearest
and
between
impedance-parameter
conductors
netic
(inductive)
only
field
electric
slightly
lines
to
case,
effect
spread
the
out
shields
neighbor.
over
the
be-
In the
“floating”
tendency
of
the
coupled
entire
mag-
line
reason
for
in
the
filters.
since
circuits
the
basis
mental
results.
sented
that
both
trix
compare
folding
pair
line
the
of
resonator
The
first
hairpin,
giving
hybrid
allel-coupled-line
filter
sired,
half-wave
second
second
hairpin
and
continued
Clearly,
possible.
tween
as
a large
It
has
turns
accounted
for
same
illustrates
consists
of
by
two
one
the
having
the
hybrid
and
all
into
The
filter
hairpin
a linear
cor-
half-wave
for
cp will
given
experimental
trial
and
Type
M I C.
in Section
are
a
may
wishes.
results
A filters
Design
con-
procedures
II 1. In
Section
IV
a
stated.
coupling
must
Fig.
be
filters
were
first
2(a)
very
graph
and
RESULTS
results
closer
VSIVll
w = 0.05
and
in
coupling
computed
picted
5 for
using
by the solid
used,
and
tions
of Cristal
4(a)
and
2(a)
and
to the
for
method
[3]
sponses
based
on
line
for
one
that
the
The
computed
responses
fractional
are
of
The
value
would
filters
Also,
the
by
more
for
response
2(a)
of cp. This
be obtained
the
for
design
halfequa-
of Fig.
transformation
cp = ~.
accurate
is de-
response
circuit
graph
are
resonator
of Fig.
using
of
1.355,
hairpin
equivalent
the
of
theoretical
circuit
any
hairpin-
bandwidth
ripple
values
identical
the
give
a four-resonator
equivalent
obtained
are
by
[3].
typical
passband
three
[5].
accurate
derived
below.
parallel-coupled-line
that
a more
should
in decibels).
the
these
circuit
on a sparse-capacitance-
A few
theoretical
Fig.
using
of
equivalent
using
a nominal
(cp value
cirhaving
designed
responses
circuit
therefore
responses
having
of filters
were
the
is based
discussed
of validity
equivalent
of Sat~Cristal
to experiment.
and
filter,
using
recalculated
and
range
and
I I 1. The
equivalent
circuit
assumption,
the
a number
method
equivalent
is identical
4(a)
then
transformation
The
[2],
Section
complex)
wave
resonators,
and
calculated
matrix
Iine
check
equations
5 to 20 percent
in
than
of Fig.
2(a)
of from
same
the
be
be-
EXPERIMENT.4L
design
procedures
shown
is
AND
theoretically
in Fig.
presented
if de-
greater
may
four
a
giving
process
cases
results.
pairs
correspond-
definition
and
conclusions
to
given
latter
the
par-
process
couplings
herein
filters.
the
line
the
in
(and
of cp = ~
words,
for
stripline
approximate
of Fig.
by
Next,
order
(but
realizations
folding
in
a
extent
designer
that
performance
half-wave
The
physical
However,
presented
electrical
as
the
half-wave
be folded,
filter.
determined
in designs
equations
basic
hybrid
times
24 dB.
from
be obtained
right.
between
into
discussed
THEORETICAL
bandwidths
ma-
judge
In
cuit
based
is folded
the
“coupling”
A value
In other
This
sense
Theory
of the
pre-
3 illustrates
may
of hybrid
introduced
approximately
design
been
on
line
a new
many
are
hairpin-line/half-wave
shown
number
on
experi-
to what
resonator
of
spacing)
theoretical
are
and
of
resonators.
III.
II,
equations
degree
the
in decibels
qualitative
mathematical
and
in both
II.
A.
approach,
parallel-coupled
Fig.
structed
a
degenerated
in Section
Section
and
unsatisfactory.
may
filters.
half-wave
the
and
has
A precise
presented
summary
responses
becomes
filters
resonators
process.
the
point
B
are
hairpin
in
resonators.
ing
to very
sparse-capacitance
method
hairpin-line
open-circuited
(b)
closeness
hairpin
to no coupling.
capaci-
and
may
relative
responds
In
re-
be judged
examples
reader
at what
design
Conceptually,
the
the
equations,
coupling
likelihood
either
filter
and
judge
constituting
Ultimately,
theoretical
computed
viewpoint
approximate
in
several
so that
any
must
between
sparse-inductance
theoretical
be
necessary
Nevertheless,
assumptions,
preclude
initially
design
the
constituting
used
consequently
lat-
A and
leads
procedures.
assumption
of comparisons
Type
of negligible
that
are
of either
the
that
neighbors
design
approximations
than
the
assumption
practical
practicality
for
the nearest
equivalent
the
the
The
rather
simplification
circuits
beyond
achieving
former
enormous
equivalent
coupling
complex
the
is the
hairpin-line
tive
on
making
assumption
sults
of
to
be given
The
ter
pairs
and
to denote
is
resonator.
array.
discussions
cp is used
parameter
order
the
following
parameter
Theoretical
equivalent
recircuit
CRISTAL AND FRANI03L : MICROWAVE
711
~lLTE1?S
40 -–—- -XT
I—
ep.m
\
dB
\
F‘.,
\\
C-Z;p::
:
\
\
\ \,\
\/l
;
,,,, +;‘r:
b’
,30}>’
---+
,\l’
“’&-
gzo,
.__:!,(>,
‘;
~ --*
;
‘$
10 -——-
—--
j
\\”
J&.—
-
~
‘,\
‘!
I
;:-
,\i
~-.
~
,
.-m
,,+...J.
.- ,_.
~.LL!L
.1.
1
J
--
89
88
o-
90
__–L~v
*-
–J
84
85
8s
O-DEGREES
Fig.
for
cp = 8 and
curate
12 dB
curves,
analysis
a function
predicts
remains
out
for
that
coupling
the
nearly
stripline
for
practice,
so
of practical
that
decibel
curve.
Fig.
the
effects
of the
diminished
cases.
Figs.
5 and
bandwidth
for
of
third
curve
curve
will
1.355,
of
ally
N,
possible
function
done
cp
But,
later.)
again
8-OEGREES
tighter
than
cp
value.
to plot
the
8, which
of
VSWR
12 and
the
Computed
VSWR
responses using approximate
exact equivalent
circuits
(N =4, w =0.15).
de-
passband
is also
VSWR
in the
the
the
co dB.
shown.
more
(A
This
accurate
from
is nearly
the
equi-
was
was
value
found
universal
computed
and
to
for
This
0.01-
a
the
and
it
contraction
curve.
was
be virtu-
bandwidth
Consequently,
bandwidth
the
equiva-
however,
design
resonators.
manner,
accurate
nominal
nominal
relative
above
more
Surprisingly,
contraction
of filter
narrow-
having
contraction
using
of the
for
7.
bandwidth.
less
batidwidth
Fig.
reflect
the
filters
Again,
examined
of cp in a single
in
for
calculated
A”
the
diminished
cases
the
number
values
bandwidth
was
independent
the
curves.
theoretical
as “Curve
calculated
circuit
percefitage
and
a
the
of the
bandwidth
function
w = 0.15,
be discussed
value.
lent
of
denoted
over
infinite-
merely
results
hairpin-line
nominal
all
typical
7 presents
reveals
In
be
approxi-
the
selectivity
Fig.
analysis
ripple
In
usually
lie
bandwidths
‘EI!III”H=E1
Thus
case.
would
curves
4 resonator
bandwidths
VSWR’S
is
8-dB
a worst
would
Computed
selectivity
responses using approximate
and exact equivalent
circuits
(N=4,
w = 0.05).
be pointed
cp = 12 and
in the
ac-
Fig.
filters.
responses
the
6 represent
89
VSWR
realization.
corresponding
differences
coupled
sign
the
the
the
geometries,
responses
between
6 presents
cases
10 to 20 dB
typical
in the range
k
~
88
and
more
that
It should
is essentially
of from
the
as cp decreases
planar
limit
mately
As expected,
all
cp = 8 dB
cp values
selected,
in
M IC
long-dash
that
larger
equiripple.
and
the
the
6.
Fig,
contraction
becomes
However,
is near
curve
by
Note
a bandwidth
which
coupling).
response
depicted
respectively.
of cp,
(tighter
are
“.
O–DEGREES
5.
Computed
VSWR
responses using approximate
and exact equivalent
circuits
(N= 4, w =0.05).
short-dash
-..
07
\“
is
as a
has been
and
O.1O-
Fig.
8.
Relative
bandwidth
contraction
versus coupling
parameter
cp.
and
722
IEEE TRANSACTIONS ON MICROWAVE
TABLE
SUMMARY OF EXPERIMENTAL
Filter
:
5
ripple
cases,
filters
analyze
lent
for
The
N=
from
the
may
a hairpin-line
circuit
bandwidth
to
by
fractional
a
l—the
substituting
in Section
A in Fig.
by
simplified
value
filter
w
first
the
result
into
the
of the
latter
technique
design
- to-
!
:
nominal
z
;
.
.
nominal
8, and
-30-
:
then
equations
-20-
2
8, and
the
Fig.
-40-
given
-50
13625
7. In
this
bandwidth
case,
filter
is illustrated
in order
with
0.15
used
in
the
design
VSWR
A)
a 0.15
a design
in
frac-
value
Fig.
to
III.
be
9.
Measured
for trial
of
0
Section
is seen
I 45
150
FREQuENcY
= 0.168
equations
(Curve
140
by Curve
to achieve
cp = 12 dB,
1 – 0.112
resulting
e,= 9.7
e,= 9.7
in
in
in
in 10 pin
in 10 pin
than
a specified
dividing
from
b =0.250
b =0.250
b =0.250
h= O.025
lz=O.025
0
1) to
Fig.
have
Tellite,
.,=2.32
Tellite,
c,= 2.32
Rexohte,
e,= 2.54
99.6-percent
alumina,
99.6-percent
alumina,
all
equiva-
the
from
to
value
WI=
was
the
less
ways:
multiplying
ordinate
ordinate
were
in two
In
Ground
Plane Spacing
or Substrate
Thickness
and Roughness
Dielectric
III.
A result
tional
curve
using
hairpin-line
bandwidth
by
11 resonators.
be utilized
by first
l—the
design
value
2(a)
0.1
0.1
7
plotted
filter
of Fig.
0.1
2
1972
SPECIFICATIONS
stripline
stripline
stripline
MIC
MIC
0.1
NOVEMBER
1
FILTER
Media
0.1
4
4
4 through
curve
Passband
Rlpple
(dB)
Resonators
0.05
0.05
0.20
0.05
0.20
deviations
0.5 percent.
2)
Type
Hairpin-line
Hybrid
Hairpin-line
Hairpin-line
Hairpin-line
;
dB
Fractional
Bandwidth
THEORY AND TECHNIQUES,
I
155
I
I
loss
I
I
1
1
s WAVE Mom
+
I
The
quite
I5 5
GHZ
attenuation
and return
stripline
filter 1.
1
I
—
$“.,..
satis-
factory.
B.
Experiment
In order
of
the
trial
with
cal
to experimentally
theory
and
stripline
and
widely
differing
and
material
mental
on
the
A filters
bandwidths.
in
results
Filters
of validity
a number
were
The
for
summarized
Filters:
range
equations,
Type
experimental
Str@ine
1)
M IC
are
the
design
specifications
filters
ments
check
filter
of
constructed
nominal
electri-
several
of the
Table
1. Some
expericom-
follow.
1,
2,
and
3
were
I
10
con-
I
I
1
I
,0
1
I
30
I
1
40
1
I
50
60
FREO” ENC, — G.,
structed
Filter
Fig.
in stripline,
1 was
1 (a).
quency
nominal
were
return
12 dB.
loss
This
Using
the
contraction
is
The
shown
points
approximately
0.044
designed
data
of
with
of Fig.
between
dB.
The
are
10-dB
and
could
0.035,
a cp value
8, the
0.05
x
(1 –
0.112)
determined
believed
results
from
that
the
=
of
antici-
0.044.
bandwidth
the
finite
additional
length
line
not
be
slightly
A
to be
bandwidth
ccmnections
pairs
wide-band
hairpin-line
frequency
response
filter 1.
for
between
in
the
Fig.
10,
responses,
typically
plane-wave
by
wave
mode.
experimental
This
resonators
dB
down
there
that
mode
hairpin-line
is
has been
was
the
of the
or
nearly
filters.
filter.
for
more,
by
always
However,
filter
1,
spurious
which
tuning
a noticeable
identified
this
coupling
low-level
excited
that
practice,
response
numerous
modes
additionally
In
increasing
frequency
shows
resonators
theory.
by
interior
40
at 2.8 GHz
hairpin
the
for
swept
caused
response
in
compensated
wide-band
shown
constituting
accounted
However,
is seen
Measured
of stripline
at-
9.
and
10.
freThe
Fig.
2,5
Fig.
in
center
measured
in
approximately
was
depicted
and
1.5 GHz.
contraction
experimentally
It
type
spacing.
is
w =
The
are
plane
of the
between
filter
bandwidth
smaller.
is
ground
bandwidth
10SS are
measured
respectively.
pated
and
return
attenuation
bandwidths
12-dB
filter
design
5 percent
and
midband
0.25-in
a hairpin-line
Its
tenuation
with
were
screws.
spurious
as a surface-
excited
its
in the
magni-
CRISTAL AND FRANKEL : MICROWAVE
0
-,0
.20
-3”
%
z
~
-40
-m
.60
I
I
0
I
I
[
I
I
,SUR,
I
I
‘-
N] wAVE1
I
I
ACE WAVE
S“.,ACE
MODE
MODE
\
-,0
--REDUCE.
,0
-m dB w,,.
ADDITION
OF
DIELECTRIC
.2,
F,LLER
\
&
1
1
1
-50
ti[
1
1
,
Fig,
Fig.
I
I
723
—
1
.70
1
I
FILTERS
12.
11.
1
I
3
1
1
!
4
F,,
CLIENT”
--l
I
1
1
5
,
G
2
FRE(XJENCY
hairpin-line/half-wave
response
Fig.
parallel-coupled-line
filter
13.
Measured
of stripline
could
using
line
be substantially
hybrid
inate
air
sult
of the
or 2) by
gaps
response
between
first
for
tions
as filter
filter
the
in Fig.
1, but
11. Fig.
was
of filter
method
at 2~0 is that
by
11 gives
identical
the
hybrid
the surface-wave
mode
does
mode
was
Results
couple
at
by
of the
second
given
filter
3, whose
bandwidth.
while
reduced
data
in Fig.
The
reduce
the
nating
air gaps
shows
that
was
second
coupling
Note
that
method
25 dB
are
attempts
surface-wave
dielectric
the
path
and
(but
spurious
10
down.
dB
Fig.
I
1,9
1.7
return
and
response
1.9
2
GHZ
loss for trial
for
stripline
filter
3.
of 12-dB
sults
or
elimiFig.
with
13
the
for
2)
the
JZIC
design
filters,
tested.
0.20
filters
was
the
a
about
a dielectric
reduced
filler
spurious
and
14-dB
cp value
(1 –
narrower
bandwidth
Filters:
In
theory
and
Photographs
is
order
again
these
this
filter
was
would
be
0.149.
smaller
with
the
than
the
previous
re-
filters.
to experimental
equations
5 of Table
of
loss are 0.1144
for
bandwidth
=
pws-
bandwidth
return
0.255)
is consistent
loss in the
fractional
The
bandwidth
4 and
return
fractional
X
which
the
of
2.5)
measured
the expected
measured
c,=
measured
respectively.
“theoretical,”
20-percent
by
The
the
points
w =
there
was only
— 26 dB.
3. The
so that
together),
= 2j0, which
insertion
jelly,
filter
0.135,
at
However,
to about
case.
the
clamped
passband
petroleum
spurious
by
tightly
14 presents
band
8 dB,
the TEM
to eliminate
mode
planes
narrow
between
of
hairpin-line
interfaces.
filler
reason
in this
illustrated
are for
the dielectric
a
the
Measured
ground
response
A
response
the
time
14.
(Vaseline
hairpin-
spurious
of 5 resonators
to the
between
without
data
specifica-
interrupts
approximately
method
re-
attenuation
12. The
at the same
2f0.
13. These
design
the
geometry
mode,
not
reduces
Fig.
1,s
1.5
FREQUENCY -
Fig,
measured
in a hybrid
in
freq[]ency
filter 3.
to elim-
configuration.
2 is shown
— (i”,
wide-band
hairpin-line
1) by
The
the
electrical
constructed
greatly
the
filler
interface.
parallel-coupled-line
photograph
this
dielectric
had
ways:
a dielectric
is illustrated
2, which
line/half-wave
that
in two
parallel-coupled-
using
technique
shown
data
reduced
hairpin-line/half-wave
geometries,
6
5
2.
304
!,4
tude
4
3
— . . .
Measured
wide-band
frequency
of hybrid
strip line filter 2.
Hybrid
~
with
I were
filters
y evaluate
regard
to
constructed
are
shown
MI
C
and
in
Fig.
lEEETRANSACTIONS
724
of the
ON MICROWAVE
filters.
face-wave
These
mode
cussion
always
strongly
for
when
the
In
stripline
(c, = 9.7),
only
only
The
well-known
tables
and
Fig.
15.
15(a)
MIChairpin-line
and
(b),
that
of differing
even-
plate
was
in
an
filters.
respectively.
difficulties
strates.
trial
In
might
have
and
odd-mode
placed
0,025
(a) Filter4.
order
been
in
the
effective
dielectric
constant
vicinity
measured
of the
erately
well
sured
VSW7R
for
G = 5.35
two
points
data.
to
be
defined
Tables
of the
of g~ values
parameter
in
the
pairs
and
cp
in
between
mea-
cp has
in
of
detailed
de-
the
essen-
below.
We
that
were
prototype
given
in
first
de-
filter
Table
filter,
that
give
gi,
Chebyshev,
[7].
The
between
resonator,
resonators.
a
values
[6],
coupling
II.
having
element
hairpin
hairpin
the
of responses
is the
a single
defined
set
be
[5].
normalized
II
may
hairpin-line
however,
in
the
design
for
prototype
types
to
existing
for
given
available
Table
hairpin-line
dual
equations
the
and
are
adjacent
been
badly
geometries.
procedures
equations
other
constituting
mod-
Conseis
mode
A
the
allow
low-pass
of al’
Butterworth,
Hence,
is
auxiliary
frequency
the
The
of match,
First
order
Type
summarized
those
for
4 corresponded
computed
only
sub-
are
modifying
cutoff
resulted
response
filter
theoretically
showed
alumina
and
of
attenuation
passband
with
the
procedures
by
is the
the
not
veloped
N
For
cut-
jo.
hybrid
equations;
design
because
cover
does
design
and
than
surface-wave
design
approximate
parameters
a metal
velocities
design
2j”0.
response
modifications
present
possible
encountered
above
corn pensated
The
avoid
velocities,
This
medial
to
5.
(b) Filter
Space
of the
than
surface-wave
is topologically
filter.
suitable
designs.
velopment
tial
for
2(a),
approximate
after
filter
Fig.
interdigital
utilized
of
PROCEDURES
circuit
in
mode
Equations
equivalent
shown
to
the
in
surface-wave
less
the
with
DESIGN
Hairpin-Line-Filter
filter,
the
fre-
frequency
the
greater
be accomplished
111.
(b)
of
cutoff
length
center
attenuation
Suppression
C can
A.
slightly
stopband
diswas
surface-wave
M I C media
considerably
is
degraded.
the
are
the
a wave
j ~ is the
on alumina
frequency
quently,
MI
the
The
the
mode
(approximately)
are
filters,
However,
filters
off
M I C media.
1972
to a sur-
in
surface-wave
resonators
propagates
MIC
mentioned
corresponds
at j = 2j ~, where
filter.
mode
(a)
in
mode
NOVEMBER
to be due
briefly
The
excited
hairpin
propagates
determined
was
filters.
this
dielectric.
the
were
that
of stripline
quency
THEORY AND TECHNIQUES,
line
and
not
Mathematically,
asz
indicatL~j
ing
that
the
the
peak
VSWR
less)
filter
so that
width
(0.1-dB
further
well
the
small
adjustment
brought
5 showed
4.
the
was
percent,
the
value
factor
which
from
resonator
the
the
same
The
of
1.35
The
vicinity
of
qualitative
practice,
would
a
have
attenuation
the
and
passband
behavior
for
as that
of
the
is chosen,
results
discrepancy
for
between
M I C filters
wide-band
1 An alternate
method
d:scriherl
by Podell [4].
spurious
would
the
was
theory
have
and
the appearance
responses
been
in
—
~L~iLjj
ing
nator,
A
the
of sig-
the
forj
=
i+
1
(1)
‘
are defined
;nit;al
resonators
method
and
different
in (2).
synthesized
all
a simple
arbitrary
having
the
is presented
values
Once
design
same
later
of
cp
for
the
will
cp
con-
Cp value.
for
obtain-
each
reso-
if desired.
hairpin-line
prototype
filter
The
defined
experi-
parameters
of hairpin
However,
lines.
A serious
Lij
where
factor
sist
be obtained
8. In
couplings
10 loglo
bandVSWR
corresponded
would
Fig.
cp=—
(1 .36 or
pursued.
that
specification.
in
However,
specifications
not
4.5
to
tuned.
frequencies
was
in the
up
filter
within
with
responses
filter
optimally
tuning
contraction
this
VSWR
not
between
ripple)
using
mental
was
measured
reasonably
nificant
was
assumed
array
in terms
coupling
filter
will
of
based
consist
2N+2
of its
on
of
coupled
inductance
conditions,
can
an
2N+
N-order
low-pass
2 parallel-coupled
lines
matrix
be written
is completely
which,
for
the
as
stopband
to use ‘(wiggly
lines”
as
1 The equation
for cp was originally
cp = — 20 loglo (Lij/v’L&y),
which is more logical than (1). However,
the computer
software
was
mistaklngly
written
using (1), and all of the experimental
and most
of the theoretical
data were compiled
before the error was noticed.
CRISTAL AND FRANKEL:
MICROWAVE
FILTERS
TABLE
AUXILIARY
EQUATIONS
iV
order of low-pass
low-pass
prototype
filter
w’
low-pass
prototype
radian
w
prototype
fractional
cutoff
band-edge
‘
1–;
EQUATIONS
and
i=~,2.
LP.~l,=+l = LPP
2
frequencies
p=z;–l
of the microwave
I i=l,2,
the bandcenter;
. . ..(N+l)
fi=zi
;
LP,P+L = kLPP
\
i=l,2,
. . ..N
.—
tan Ox;
1
—
ti@l’gi_lgi
G,=
N
LU = AU(l)
1
=–
...
1
LSI{bZ,SNqt = A1l(N+l)
the lower and upper
()
7
FILTERS
P=2;
+ A1l@+z)
2(1 – k)
tilter;
f~ is
111
FOR HAIRPIN-LINE
L=;, =
. . . . N + 1);
frequency;
f I and f~ are
filter
O,=;
(i = O, 1,2,
of the microwave
%
DESIGN
AzJiJ
values
where
–
DEFINITIONS
filter;
element
~=2f2–f1_f2–f,
f2+j,
TABLE
11
AND PARAMETER
g;
bandwidth
725
1
—,
G, =
fori=land
N+l;
fori=2,3,
. . ..N.
‘
the
convenient
form
c~j/c
by
the
equation
(4)
@l’V’gi-l&Ti
cp
hairpin
~ =
llyloP/101
k
arbitrary
which
coupling
where
in decibels;
e,
positive
controls
dimensionless
the immittance
Fori=land
parameter
level in the filter
N+l
G,
effective
1
. . ..N
E
@Er;
EO
pe rmittivity
From
A M(~) = hGi sin 01
sions
the
to ZAV-l
the
L
=
of propagation
L,,
l~j/(Z~~–l)
1,!
selfith
2A
In
source
terms
Lij
the
o
normalized
malized
medium;
inductance
per
unit
of the
conductors;
[
I
o
L,a
L33
LM
O
L34
JZ44
L45
by
inductance
capacitance
equations
Table
matrix
matrix
given
II 1. Having
from
may
Table
be
in Table
obtained
I I 1, the
computed
II,
the
nor-
by
the
will
=
[L]-’
(3)
at
C~j/ (8-’
self;th
YA
lsast
and
jth
capacitance
per
unit
length
of the
vari-
case
here,
the
C
. ..0
sparse
ordinarily
the
that
may
have
way
begin
to
same
cp
of signifi-
in compa
may
rin e-
also increase.
matrix
is approxi-
configurations
checking
of estimating
deviate
be
is increased,
will
filter
a
and
the
when
appreciably
the
from
behavior.
the
equations
of hairpin-line
transformation
and
frequencies,
is one
expected
consisting
the
capacitance
planar-array
previously,
are
bandwidth
C matrix
at microwave
theoretically
noted
filter
represent
diagonal
be small
fiIters,
of the
the
the
even
the
entries
generally
as the
terms
responses
may
only
narrow-band
for
used
ipal
(It
subdiagonal
will
terms
it is known
value.
However,
that
in Table
resonators,
allows
we
III
give
all of which
present
modification
next
of
a
the
l/zA.
~ These
The
for
Since
simple
conductors;
dimen-
from
-
However,
lower
However,
designs
Y~) ;
or mutual
physical
as is the
be dense.
entries
mately
As
C~j
and
t Other
the nonprincipal
where
c~j
generally
system.)
upper
cance.
filter
[c]
the
.
nonprinc
formula
med-
.
glected.
auxiliary
given
the
(,2)
sen,
impedance.
c~i/q
be determined
. ..0
Lga
nonphysical
length
0
Lg2
the
jth
of the
are
the
;
or mutual
and
in
for
may
is sparse,
L12
matrix
velocity
v
lines
matrix
1
where
of
charts.
LIIL]2
normalized
constant
space.
values
coupled
desig;n
If
matrix
dielectric
of free
numerical
of the
ous
= k[Gi2 + ~]
inductance
relative
ium;
= /JT
~ll(o
A,,@J = <z
less than
interior.
Fori=2,3,
‘4,,@) = 1
A,,(i)
usually
normalized
parameters
Cij
may
be converted
into
All
other
will be referred
to as principal
terms or principal
entries will be referred
to as nonm-inciBal.
–, -–_,.
entries.
726
IEEE TRANSACTIONS
ON MICROWAVE
THEORY AND TECHNIQUES,
NOVEMBER
.
..
1972
0
0
II
(a)
.
(b)
.
3
0
L2N*2
Fig.
16.
Matrix
transformation
filter responses
that leaves
invariant.
TABLE
NORMALIZED
L-VALUES
2..2
(c)
Fig.
hairpin-line
17.
Progressive
steps in transforming
a parallel-coupled-line
filter into an interdigital
filter.
(a) Parallel-coupled
line filter.
(b)
Intermediate
wicket
form
for interdigital
filter.
(c) Final
form
for interdigital
filter.
IV
FOR EXAMPLE
HYBRID
FILTER
OF FIG.
12
..0
0
Normalized
L-Matrix
;
:
5
6
7
8
1:
1.40641
1.40641
0.08874
L(I, I)
4
5
6
7
8
1.00000
1.31767
1.31767
1.35828
1.35828
1.35828
1.35828
1.31767
between
hybrid
filters
two
(a)
..,2
c1 ,
-C,2
222’%
o
L(I,
1+1)
0
0
Coupled-Line
The
under
discussion
forming
Fig.
ant,
provided
that
the
ROW 2N ,
a hairpin,
and
by
able
redundancy
numerical
parallel-coupled-
A
hairpin-line
that
Parallel-
filters
their
of the
responses
are
type
invari-
matrix
remains
constant.
In
%,,. .,
filter
Aii
to
fori=2,4,6,
addition,
physical
..0,
(2N)
realizability
(5)
The
that
and
visualized,
all
realizable
Aii
inductance
hairpin-line
(5),
whose
effect
is depicted
.Lij
values
suitable
satisfies
rows
appropriate
Thus
and wicket
(b) Wicket
resonators.
even-numbered
the
(5).
interdigital
matrix.
in determining
hairpin
a computer,
is easily
the
added
~atisfies
the
transformation
geometry
that
flexibility
for
on
physically
expression
i+l,
-%
z
and
values
to equivalent
Lii — 2L<,i+1+L~+1,
.331C:3
18.
Capacitance
matrices for equivalent
filters.
(a) Interdigital
filter
capacitance
filter capacitance
matrix,
Note
reveals
!T
(b)
0.00000
0.30031
Filters
for
0
“.
o—
Hairpin-Line/Half-Wave
theory
%3’33
0
1
be designed.
detailed
I
.
-C23
1“
lines
of Hybrid
‘22”22
%3
programmed
B. Design
1’
[:
hairpin-line/half-wave
may
CN+I,
N.,
0.30031
1.31767
1.00000
coupling
“..
‘1
L-Matrix
I
’33
1
0.30031
0.08874
0.08303
0.08570
0.06571
0.08570
0.08303
1
“23
10
—
1.00000
1;
Iine
1+1)
1.00000
1.40641
1.40641
1.35828
1.35828
1.35828
1.35828
Normalized
which
I -c,
L(I,
L (I, I)
I
have
values
not
the
in
is
the
in Fig.
16.
increment
a way
violating
matrices
easily
on
that
(6)
give
corresponding
filters.
transformation
re-
Aii
— Li,i+ly
=
i=2,4,
. . ..(2N)
(7’)
quires
Lii
Li,
The
mutual
pin
–
Li,i_l
parameters
inductance
resonators.
–
in
Liri~l
(5)
between
Thus
for all L
>0,
correspond
line
pairs
it is evident
that
to
the
constituting
there
self-
(6)
and
hair-
is consider-
is particularly
no
useful,
capacitive)
constituting
transforms
coupled-line
coupling
a
hairpin
the hairpin
resonator.
for
in
this
exists
case
no inductive
between
lines
resonator.
resonator
Repeated
hto
(and
originally
1% other words, (7)
a half-wave parallel-
application
of
(7)
per-
CRISTAL
AND
FRANKEL
: MICROWAVE
M
L11
*
I
LIZ
LIZ
L22
’23
’23
L33
0
o
*
the
design
of
serve
as an
filter
in Fig.
IV.
physical
The
would
By
L34
~’L12
2
N L22
‘2L23
o
‘2L23
2
N L33
●
o
.
.
.
.
NL34
Fig.
19.
Example
of inductance
hairpin-line/half-wave
The
example
L-matrix
for
a hairpin-resonator
is given
in the
upper
of the
filter
half
filter
like
transformation
par-
following
original
realization
given
This
in
leads
A,,
the
=
–
for
in
shown
ing
the
tween
to
any
the responses
identical.
matrix
at
this
(8)
lower
half
Fig.
At
of Table
IV
configuration
are
12.
suitable
of Fig.
The
use
Exact
of the
preceding
straightforward
tables
for
Tables
way
the
interdigital
to
below
some
digital
tional
may
the
needed
The
details
the
intermediate
cally
nected,
The
and
reader
is referred
as the
have
point
it
Fig.
at the
process
physical
filter
duals.
17(c)
can
and
It
form
that
known
can
proved
that
in Fig.
optimum
responses
[8].
that
in
Fig.
can
be
the
in Fig.
The
back
filter
to the
was
are
wicket
The
electrical
in
form
applied
the
capacitance
17(c).
(N+
18(a)
expanded
matrix
in
A
second
of
does
not
permit,
Fig.
1)X
(N+
1)
corresponding
into
the
capacitance
to
an
(2N+2)
matrix
(with
formally
filter
the
with
transformations
the
particufilter
inverted
it
transformation
very
sirlilar
to
obtain
cle-
the
C-
realized.
[10],
The
tion
of
level
changing
must
this
at
to
Fig.
be performed
transfer
&.@er;or
19.
Note
allowed
i and
is to
that
the
rows
and
scale
the
The
sections
of rows
the
transforma-
of
the
filter
is
transformation
Paired
and
columns,
trarls-
columns
fori=2,4,
1,
is
filter,
capacitance-matrix
pairs
i +
that
within
response.
the
on paired
The
is a special
transformations
points
to conventional
formations.
useful
transformation
specific
its
as applied
in
is
transformation
capacitance-matrix
effect
illustrated
that
congruence
to
The
only
the
input
exceptions
and
in
column
filter
of
constant-—say,
matrix
interdigital
X (2N+2)
1 (or
output)
flat
can
be
2N+2)
F’ig.
are
. . ..2N.
be intermixed.
larly
usej-ul
when
computer
are when
In
the
(9)
would
of the
filter
be carried
Both
modify
by
out
for
to
modify
terminations,
the
by
rowa suitable
the
factor
and
the
in
any
transformations
programmed
terminal.
transforming
order
be multiplied
This
transformation
16 may
may
share
would
ill.
ccmgruence
of
rule
impedances.
to change
impedance
The
tion
to this
output
or, equivalently,
given
maximally
but
proceed.
Lij
Transformations
inductance
row–columns
of Ctj are available
and
to
by
to obtain
physically
of
by C.
Fig.
to achieve
an interdigital
Tables
how
the
Next,
is then
Indwztance-Matrix
these
represent
that
performed
is then
in distinction
folding
filter
maybe
17(c).
[3].
interdigital
L-matrix
which
without
be con-
procedure
[9].
hairpin-line
17(b).
are physimay
given
folding
Chebyshev
Space
the
they
to be
hairpin-line/parallel-coupled-line
impedarme
design.
uniquely
shown
yield
Fig.
mathematically
of duality
filter
is well
18(a)
verified
if
principle
a hairpin-line
shown
the
be converted
the
into
considerations
been
filter
half-
in
other
L replaced
parallel-coupled-line
16 are
any
matrix
be replaced
so
be
but
were
capacitance
evident
to
resonators.
The
form
the
wickets
form
addi-
17(a)
shown
this
for
in Fig.
potential,
for
Consequently,
17(b),
Fig.
same
[9]
of folding
of the
the interdigital
has since
wicket
filter”
D.
itself
will
real,
of inter-
interdigital
shown
ends
justification
on
The
In
are
are
satisfy
filter
y (6) with
is perhaps
18(b)
in Fig.
matrix,
the
to satisf
a half-wave
hybrid
sired.
of
filters
18(b)
not
be-
Furthermore,
interdigital
need
normalization),
depicted
lar
summarize
design
[6],
a
design
filters.
to
result
filter
in
of hybrid
we
the
filt&-s.
grounded
yielding
original
based
filter
concerning
“wicket
the
close
exact
design
concisely
on interdigital
since
to the
facts
parallel-coupled-line
Then,
the
method
be conceptualized
wave
[8]
of
parallel-coupled-line
present
filters.
application
filters
hairpin-line/half-wave
order
allows
and
row
correspond-
coupling
a wicket.
if the wicket
each
latter
is no
a~, in Fig.
point
would
of
the
there
constituting
parameters
then
Cij
with
which
of the wicket
open-circuited
transformation
in
Of course,
this
ob-
18(b),
intermediate
18(b)
The
Fig.
pair
The
conditions.
1(a).
filters.
filter
line
of Table
Fig.
in
a wicket
coupling
this
that
matrix
will
0.08874
to the ph ysical
Using
.
for hairpin-line
represents
Designs
C.
“
0
realized,
results
●
●
o
The
A,8 =
NL34
J
be an all-hairpin-line
tained.
o
●
letting
the
NL12
.
0
O**.
Lll
“
0
12 with
(cp = 12 dB)
0
—
.
hybrid
illustration.
shown
0
L34
structures.
of 12 dB
o
●
o
allel-coupled-line
,.. .
●
.
mits
727
FILTERS
input
(or
il~z.
transformasequence
are
an interactive
or
particutime-
728
IEEE TRANSACTIONS
IV.
SUMMARY
Approximate
Iine
and
Iine
theory
hybrid
filters
have
are
based
pling
beyond
On
nearest
other
hood
the
hand,
rules
design
that
hybrid
that
practical
design
is
the
engineer
to
hairpin-line/haIf-wave
haIf-wave
and
of several
equivalent
yields
circuit
nearly
over
value.
The
showed
for
curve
satisfactory
that
for
the
designs
using
The
ap-
hairpin-line,
or
a systematic
using
(in
in
design
Chebyshev
from
the
paper.
of very
these
with
filters
can
be
con-
judged
narrow
this
A number
of trial
The
experimental
constructed
good
there
haps
due
was
the
coplanar
be
practicoupled
using
spurious
and j.
it was
be
2) bv
lengths
5- and
tested.
hybrid
filters
be
in
relatively
theory
after
accounted
for.
the
How-
contraction
connecting
for
stripline
per-
line
filters
of a surface-wave
at
center
mode
N$O, where
frequency
that
reduced
geometry
mode
of
and
pairs
resonators.
demonstrated
using
was
bandwidth
line
substantially
a hybrid
to
approximate
passbands
is the
and
judged
data
presence
surface-wave
and/or
finite
filters
constructed
factor
experimental
give
can
the
MIC
that
between
a dielectric
by
filler
N
two
and
How-
responses
methods:
the
re-
tended
is an even
filter.
spurious
interrupts
input
also
that
of the
these
the
path
output
to eliminate
data
quencies
just
above
it
had
a cutoff
frequency
pin
resonators
dielectric.
The
in M IC
filters.
the
a hybrid
To
effect
1)
by
of the
ports,
the
date,
fiIters
still
allowing
the
of the
the
and
that
only
was
prove
hairin
the
excited
way
mode
of over-
is to utilize
surface-wave
path
is interrupted.
be-
with
this
parallel-coupled-
satisfactory
a considerable
mode
the
always
practical
the
output
fre-
of the filters.
a wavelength
mode
presat
to when
hairpin-line/half-wave
may
of the
surface-wave
surface-wave
such
input
hybrid
Iine
that
for
per-
propagated
corresponding
surface-wave
allowing
passband
approximately
geometry
the
found
are
coming
design
was
principal
pass-
stopband
because
that
con-
in the
after
the
degraded
mode
the
Specifically,
tween
theory
severely
the
filters
well
However,
a surface-wave
reducing
hairpin-line
approximate
were
of
for
moderately
contraction.
formances
thereby
1972
mode.
C compared
in MIC
degree
media,
of flexibility
while
to
the
engineer.
The
fully
authors
and
wish
skillfully
described
experimental
air
to
thank
E.
fabricated
in this
paper
all
and
Fernandes,
of
the
recorded
who
care-
experimental
many
of the
data.
to approxi-
is near
hairpin-line
were
additional
hairpin
the
integer
ever,
with
to the
constituting
vealed
for
contraction
ever,
to
data
agreement
and
were
in strz+line
bandwidth
The
stripline
bandwidths
with
bandwidth
filters
to
lines.
20-percent
band
MI
NOVEMBER
ACKNOWLEDGMENT
Consequently,
are
;n
nominal
bandwidth
Interestingly,
realizing
cases),
the
however,
equations
bandwidths
theory
interface,
surface-wave
experimental
structed
ence
THEORY AND TECHNIQUES,
dielectric
to the
method,
an “exact”
approximate
a universal
presented
for
designs
contraction,
by
25 percent.
cal limit
likeli-
self-consistent
in
bandwidth
bandwidth
approximate
mately
to quite
any
design
filters
theoretical
equiripple
compensated
the
line
a diminished
traction
to rela-
procedures.
way.
Analysis
but
the
parallel-coupled-line,
parallel-coupled
simple
beyond
procedures.
of
Aldual
preclude
a set
equa-
and
leads
the
The
cou-
leads
design
assumption
procedure
permits
usual
coupling
and
circuits
and
negligible.
the
assumption
latter
hairpin-
inductive
is
than
circuits
the
of achieving
theory
that
neighbors
former
equivalent
proximate
The
capacitive
equivalent
for
at
coupling
parallel-coupled-
to satisfy
neighbors,
complicated
equations
assumption
of negligible
simple
the
design
presented.
the
difficult
assumption
nearest
been
on
the
more
tively
and
gaps
CONCLUSIONS
hairpin-line/half-wave
tions
though
AND
ON MICROWAVE
REFERENCES
[1] J. T. Bolljahn
and G. L. Matthaei,
“A study of the phase and
filter properties
of arrays of parallel
conductors
between
ground
planes, ” Proc. IRE, vol. 50: pp. 299-311,
Mar. 1962.
[2] S. Frankel,
“Equivalent
cu-cuits for TEM
structures
with periodic terminations,
“ in Proc. 1971 IEEE
Region 6 Conf. (Sacramento, Calif., May 11–13), Paper 5B-3, 1971.
[3] R. Sato and E. G. Cristal,
“Simplified
analysis of coupled transmission-line
networks,
” IEEE
Trans. Microwave
Theory Tech.,
vol. MTT-18,
pp. 122–131, Mar, 1970.
[4] A. Podell,
“A high directivity
microstrip
coupler
technique,”
in
~~7’E
G-MTT
1970 Int. Syw@. Dig. (May
11-14),
pp. 33-36,
. ..-.
“New design equations
for a class of microwave
[5] E. G. Cristal,
filters, ” IEEE
Trans. Microwave
Theory Tech. (Corresp.),
vol.
MTT-19,
pp. 486-490,
May 1971.
[6] G. L. Matthaei,
L. Young,
and E. M. T. Jones, Design of Microwave Filters, ImPedance-Matching
Networks,
and Coupling
Structures.
New York:
McGraw-Hil},
1964.
Network
Analyszs
and Synthesis.
New
York:
[7] L. Weinberg,
McGraw-Hill,
1962.
[8] R. J. Wenzel and M. C. Horton,
“Exact
design techniques
for
microwave filters, ” Bendix
Research
Lab.,
Southfield,
Mich.,
Final
Rep., Contract
DA28-043-AMC-00399
(E), Feb. l-Apr.
30, 1965.
[9] G. L. Matthaei,
“Interdigital
band-pass
filters, ” IEEE
Trans.
Microwave
Theory Tech. (1962 Symposium
Issue), vol. MTT-10,
pp. 479491,
Nov.
1962.
and practical
applications
of capaci[10] R. J. Wenzel, “Theoretical
tance matrix
transformations
to TEM
network
design, ” IEEE
Trans.
Microwave
Theory
Tech. (1966 Symposium
Issue),
vol.
MTT-14,
pp. 635-647,
Dec. 1966.
易迪拓培训
专注于微波、射频、天线设计人才的培养
网址：http://www.edatop.com
射 频 和 天 线 设 计 培 训 课 程 推 荐
易迪拓培训(www.edatop.com)由数名来自于研发第一线的资深工程师发起成立，致力并专注于微
波、射频、天线设计研发人才的培养；我们于 2006 年整合合并微波 EDA 网(www.mweda.com)，现
已发展成为国内最大的微波射频和天线设计人才培养基地，成功推出多套微波射频以及天线设计经典
培训课程和 ADS、HFSS 等专业软件使用培训课程，广受客户好评；并先后与人民邮电出版社、电子
工业出版社合作出版了多本专业图书，帮助数万名工程师提升了专业技术能力。客户遍布中兴通讯、
研通高频、埃威航电、国人通信等多家国内知名公司，以及台湾工业技术研究院、永业科技、全一电
子等多家台湾地区企业。
易迪拓培训课程列表：http://www.edatop.com/peixun/rfe/129.html
射频工程师养成培训课程套装
该套装精选了射频专业基础培训课程、射频仿真设计培训课程和射频电
路测量培训课程三个类别共 30 门视频培训课程和 3 本图书教材；旨在
引领学员全面学习一个射频工程师需要熟悉、理解和掌握的专业知识和
研发设计能力。通过套装的学习，能够让学员完全达到和胜任一个合格
的射频工程师的要求…
课程网址：http://www.edatop.com/peixun/rfe/110.html
ADS 学习培训课程套装
该套装是迄今国内最全面、最权威的 ADS 培训教程，共包含 10 门 ADS
学习培训课程。课程是由具有多年 ADS 使用经验的微波射频与通信系
统设计领域资深专家讲解，并多结合设计实例，由浅入深、详细而又
全面地讲解了 ADS 在微波射频电路设计、通信系统设计和电磁仿真设
计方面的内容。能让您在最短的时间内学会使用 ADS，迅速提升个人技
术能力，把 ADS 真正应用到实际研发工作中去，成为 ADS 设计专家...
课程网址： http://www.edatop.com/peixun/ads/13.html
HFSS 学习培训课程套装
该套课程套装包含了本站全部 HFSS 培训课程，是迄今国内最全面、最
专业的 HFSS 培训教程套装，可以帮助您从零开始，
全面深入学习 HFSS
的各项功能和在多个方面的工程应用。购买套装，更可超值赠送 3 个月
免费学习答疑，随时解答您学习过程中遇到的棘手问题，让您的 HFSS
学习更加轻松顺畅…
课程网址：http://www.edatop.com/peixun/hfss/11.html
`
易迪拓培训
专注于微波、射频、天线设计人才的培养
网址：http://www.edatop.com
CST 学习培训课程套装
该培训套装由易迪拓培训联合微波 EDA 网共同推出，是最全面、系统、
专业的 CST 微波工作室培训课程套装，所有课程都由经验丰富的专家授
课，视频教学，可以帮助您从零开始，全面系统地学习 CST 微波工作的
各项功能及其在微波射频、天线设计等领域的设计应用。且购买该套装，
还可超值赠送 3 个月免费学习答疑…
课程网址：http://www.edatop.com/peixun/cst/24.html
HFSS 天线设计培训课程套装
套装包含 6 门视频课程和 1 本图书，课程从基础讲起，内容由浅入深，
理论介绍和实际操作讲解相结合，全面系统的讲解了 HFSS 天线设计的
全过程。是国内最全面、最专业的 HFSS 天线设计课程，可以帮助您快
速学习掌握如何使用 HFSS 设计天线，让天线设计不再难…
课程网址：http://www.edatop.com/peixun/hfss/122.html
13.56MHz NFC/RFID 线圈天线设计培训课程套装
套装包含 4 门视频培训课程，培训将 13.56MHz 线圈天线设计原理和仿
真设计实践相结合，全面系统地讲解了 13.56MHz 线圈天线的工作原理、
设计方法、设计考量以及使用 HFSS 和 CST 仿真分析线圈天线的具体
操作，同时还介绍了 13.56MHz 线圈天线匹配电路的设计和调试。通过
该套课程的学习，可以帮助您快速学习掌握 13.56MHz 线圈天线及其匹
配电路的原理、设计和调试…
详情浏览：http://www.edatop.com/peixun/antenna/116.html
我们的课程优势：
※ 成立于 2004 年，10 多年丰富的行业经验，
※ 一直致力并专注于微波射频和天线设计工程师的培养，更了解该行业对人才的要求
※ 经验丰富的一线资深工程师讲授，结合实际工程案例，直观、实用、易学
联系我们：
※ 易迪拓培训官网：http://www.edatop.com
※ 微波 EDA 网：http://www.mweda.com
※ 官方淘宝店：http://shop36920890.taobao.com
专注于微波、射频、天线设计人才的培养
易迪拓培训
官方网址：http://www.edatop.com
淘宝网店：http://shop36920890.taobao.com