1. business and industrial
2. company
3. bankruptcy

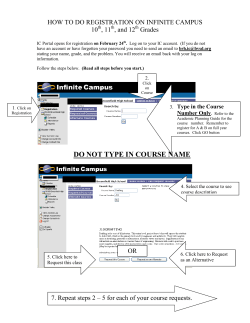
Instructor’s Resource Manual
E I G H T H
E
D
I
T
I
O N
GENERAL CHEMISTRY
Darrell D. Ebbing
Wayne State University
Steven D. Gammon
University of Idaho
HOUGHTON MIFFLIN COMPANY • BOSTON • NEW YORK
Vice President and Publisher: Charles Hartford
Executive Editor: Richard Stratton
Development Editor: Danielle Richardson
Editorial Associate: Rosemary Mack
Senior Project Editor: Nancy Blodget
Senior Manufacturing Coordinator: Priscilla Bailey
Executive Marketing Manager: Katherine Greig
Marketing Associate: Alexandra Shaw
Copyright © 2005 by Houghton Mifflin Company. All rights reserved.
Houghton Mifflin Company hereby grants you permission to reproduce the Houghton
Mifflin material contained in this work in classroom quantities, solely for use with the
accompanying Houghton Mifflin textbook. All reproductions must include the Houghton
Mifflin copyright notice, and no fee may be collected except to cover the cost of
duplication. If you wish to make any other use of this material, including reproducing or
transmitting the material or portions thereof in any form or by any electronic or
mechanical means including any information storage or retrieval system, you must obtain
prior written permission from Houghton Mifflin Company, unless such use is expressly
permitted by federal copyright law. If you wish to reproduce material acknowledging a
rights holder other than Houghton Mifflin Company, you must obtain permission from
the rights holder. Address inquiries to College Permissions, Houghton Mifflin Company,
222 Berkeley Street, Boston, MA 02116-3764.
Printed in the U.S.A.
Contents
Part I
Introduction
1
Part II
Chapter Essays
Part III
Alternate Sequence of Text Coverage
Part IV
Chapter Descriptions
Part V
Operational Skills Masterlist
Part VI
Correlation of Cumulative-Skills Problems with Text Sections
Part VII
Alternate Examples for Lecture
Part VIII
Brief Notes on Suggested Lecture Demonstrations
3
5
8
28
66
128
62
PART I
Introduction
General Chemistry, Eighth Edition, is designed to give the instructor the greatest flexibility in
creating a course for his or her students and to make the process of teaching with the text as
smooth as possible. The careful, logical, and clear development of material in each chapter,
with its appropriate division into parts, sections, and subsections, allows for flexible rearrangement to meet individual syllabus configurations.
To smooth the process of teaching with the text, we have worked diligently in several areas.
Each technical term is clearly defined at first mention, and each concept is carefully explained
and made as concrete as possible by using illustrations from everyday situations or by relating
the concept clearly to its use in chemistry. Descriptive and applied chemistry is emphasized
early on and throughout the book through the inclusion of interesting chemical facts in the
text, in problems, and in the boxed essays that occur within the chapters. We believe this
emphasis on descriptive chemistry is necessary to provide the motivation for learning chemical concepts. We have also added Concept Checks and Conceptual Problems to aid the student
in learning the concepts. In these, we ask students questions that require them to think and to
solve problems by first asking, What are the chemical concepts that apply here? These
questions are phrased to force a thoughtful answer rather than allowing the student to look
for a memorized algorithm. A Conceptual Guide is available that provides solutions to all of
these Concept Checks and Conceptual Problems. By paying attention to these areas, we have
removed a burden from the instructor, who can now concentrate his or her attention on the
main requirements of teaching—motivating the students, emphasizing important points,
discussing difficult concepts, drawing parallels, and so forth.
In the introduction to your course, it may be well to note for the students several features
of the text that are specifically designed to help them in their study of chemistry. Indexes of
textbooks tend to be underutilized, but the one we have prepared for General Chemistry, Eighth
Edition, is especially thorough. When students want to find a topic they covered earlier but
can’t remember where in the text it is covered, they should be encouraged to consult the index.
On the other hand, when they encounter a term whose definition escapes them, they should
turn to the extensive glossary placed just before the index.
In order to understand where a chapter is going and how the material is developed, students
should examine the contents given at the start of each chapter. You can make use of this
contents section as well. It will allow you to survey a chapter quickly to see how it corresponds
to your course plan and to see what deletions or changes of order you might wish to make.
You can refer the students to this contents section when you inform them of deletions or
changes in order or want to indicate parts of the chapter you intend to emphasize.
Note that important terms have been highlighted by black boldface type. To facilitate the
student’s review, these terms are gathered together at the end of the chapter in the order in
which they occur in the text. As the student goes through the list, he or she should recall the
Copyright © Houghton Mifflin Company. All rights reserved.
1
2
PART I
context in which the word occurred. All of these important terms are included in the glossary
at the end of the book.
Problem solving has received special attention in the text. Students should be aware that
key statements or equations used for problem solving are highlighted. Also, page numbers of
tables of data needed for problem solving are listed under Locations of Important Information
on the inside back cover of the book. The major problem-solving skills are explained in
Examples, most of which include a Problem Strategy that underscores the thinking process
involved in solving the problem. Some Examples include an Answer Check that employs a
“check of reasonableness” of the answer, based on general knowledge of the problem. The
Examples are followed by Exercises for the student to work out. The answers to these Exercises
are given at the back of the book. Corresponding end-of-chapter Problems are noted at the
ends of the Exercises. Problems have been divided into categories: Conceptual Problems,
Practice Problems, General Problems, and Cumulative-Skills problems (these have been
followed by Media Activities). Answers to odd-numbered Problems appear at the end of the
book. Complete solutions to Exercises, Review Questions, and Problems are available to
instructors in the Solutions Manual for General Chemistry. The Solutions Manual is also
available for sale to students if the instructor approves. Alternatively, you may prefer that
your students obtain the Student’s Solutions Manual, which contains solutions to only the
odd-numbered Problems (along with complete solutions to Exercises and Review Questions).
In Part II of this manual, we describe and list the chapter essays. In Part III we describe
several possible alternate sequences of text coverage, which can help you design your course.
Part IV can also help you with this; for example, the chapter descriptions given there point
out alternate placements of chapters. Part IV also discusses the development of the chapter
text, gives special notes on the chapter, and offers suggestions on how to abbreviate the
material if that seems appropriate. Part V gives an operational skills masterlist in which
operational skills are correlated with Examples, Exercises, and Problems. You may find this
of use in making reading and problem assignments. Part VI lists sections of the text that cover
material needed to solve each cumulative-skills problem. This list will help you avoid
assigning problems that require text sections you have omitted. Part VII gives a selection of
Alternate Examples you can use in your lectures. Part VIII describes lecture demonstrations
you may wish to try.
Copyright © Houghton Mifflin Company. All rights reserved.
PART II
Chapter Essays
The eighth edition of General Chemistry includes two series of boxed essays whose purpose is
to augment the main text. These essay series are titled A Chemist Looks At, and Instrumental
Methods.
The essays in the A Chemist Looks At series explore topics of general interest (such as human
vision) or subjects that are in the news (such as superconductors). Each essay applies the
principles of chemistry described in the text, perhaps expanding on them.
The Instrumental Methods essays describe some of the most important instrumental methods
used by research chemists today, such as mass spectrometry and x-ray diffractometry. These
descriptions are purposely brief and are intended only to make students aware that chemists
today routinely use sophisticated instruments in their work. Students are generally fascinated
to learn that modern chemistry relies so strongly on such instruments.
A Chemist Looks At
Essay Title
The Birth of the Post-it Note®
Thirty Seconds on the Island of Stability
Nitric Oxide Gas and Biological Signaling
Carbon Dioxide Gas and the Greenhouse Effect
Lucifers and Other Matches
Zapping Hamburger with Gamma Rays
Lasers and Compact Disc Players
Levitating Frogs and People
Ionic Liquids and Green Chemistry
Chemical Bonds in Nitroglycerin
Left-Handed and Right-Handed Molecules
Human Vision
Stratospheric Ozone (An Absorber of Ultraviolet Rays)
Removing Caffeine from Coffee
Liquid Crystal Displays
Water (A Special Substance for Planet Earth)
Hemoglobin Solubility and Sickle-Cell Anemia
The World’s Smallest Test Tubes
Superconductivty
Buckminsterfullerence—Third Form of Carbon
Silica Aerogels, the Lightest “Solids”
Copyright © Houghton Mifflin Company. All rights reserved.
Text Page
Chapter
Category
5
56
188
213
235
271
276
311
335
344
384
409
410
433
453
465
486
514
537
542
550
1
2
5
5
6
7
7
8
9
9
10
10
10
11
11
11
12
12
13
13
13
Everyday Life
Frontiers
Life Science
Environment
Everyday Life
Everyday Life
Materials
Frontiers
Frontiers
Everyday Life
Everyday Life
Life Science
Environment
Everyday Life
Everyday Life
Environment
Life Science
Frontiers
Materials
Materials
Materials
3
4
PART II
Essay Title
Text Page
Chapter
Category
602
629
667
682
700
748
784
883
892
973
992
1040
1058
14
15
16
16
17
18
19
21
21
23
23
25
25
Frontiers
Everyday Life
Life Science
Everyday Life
Environment
Environment
Life Science
Life Science
Environment
Everyday Life
Life Science
Materials
Life Science
Seeing Molecules React
Slime Molds and Leopards’ Spots
Taking Your Medicine
Unclogging the Sink and Other Chores
Acid Rain
Limestone Caves
Coupling of Reactions
Positron Emission Tomography (PET)
The Chernobyl Nuclear Accident
Salad Dressing and Chelate Stability
The Cooperative Release of Oxygen from Oxyhemoglobin
Discovery of Nylon
Tobacco Mosaic Virus and Atomic Force Microscopy
Instrumental Methods
Essay Title
Separation of Mixtures by Chromatography
Mass Spectrometry and Molecular Formula
Scanning Tunneling Microscopy
Nuclear Magnetic Resonance (NMR)
X Rays, Atomic Numbers, and Orbital Structure (Photoelectron
Spectroscopy)
Infrared Spectroscopy and Vibrations of Chemical Bonds
Automated X-Ray Diffractometry
Text Page
Chapter
14
98
282
298
1
3
7
8
305
363
464
8
9
11
Copyright © Houghton Mifflin Company. All rights reserved.
PART III
Alternate Sequence of Text Coverage
For a two-semester course, the first semester might cover a selection of material from Chapters
1 through 12, which treats basic chemistry, atomic and molecular structure, and states of matter
and solutions. The second semester would then cover a selection of material from the last half
of the text, which treats kinetics, equilibrium (including thermodynamics and electrochemistry), nuclear chemistry, and descriptive chemistry. You may want to look at Part IV of the
Instructor’s Resource Manual for suggestions on ways to select or abbreviate the material from
these chapters to fit your schedule.
A three-quarter course following the text sequence might begin with a selection from
Chapters 1 through 8. Thus, the first term would cover basic chemistry and atomic structure.
The second term would begin with chemical bonding (Chapters 9 and 10) and go through the
introductory chapters on chemical equilibrium (Chapters 15 and 16). The last term would
cover aqueous equilibrium, thermodynamics, electrochemistry, nuclear chemistry, and a
selection from the block of descriptive chemistry chapters (Chapters 13 through 25).
Alternate sequences of the text material can be easily designed. The figure given on the
following page may help you design your course by showing how the text chapters depend
on previous ones. An arrow pointing to a box indicates that the preceding chapter is a
prerequisite to the chapter given in the box. In addition, the chapter descriptions in Part IV
give suggestions on alternate placements of material and possible deletions of topics (see
under Placement of the Chapter and Abbreviation of the Material).
One possible alternate lecture schedule follows. In this schedule, coverage of gases just
precedes discussion of liquids and solids, and thermodynamics is covered before equilibrium.
Alternate Two-Semester Sequence
(Gases just before liquids; thermodynamics before equilibrium)
First Semester
Chapters 1 through 4
Chapters 7 through 10
Chapters 5, 11, 12
Chapter 13
Basic chemistry
Atomic and molecular structure
States of matter and solutions
Materials
Second Semester
Chapter 14
Chapter 6
Chapter 19
Chapter 15
Chemical kinetics
Thermochemistry
Thermodynamics (Sections 19.1 through 19.5)
Introduction to equilibrium (plus Sections 19.6 and 19.7)
Copyright © Houghton Mifflin Company. All rights reserved.
5
6
PART III
Ch. 1 Matter; Units
Ch. 2 Atomic Theory
Ch. 3 Stoichiometry
Ch. 4 Reactions; Intro.
Ch. 7 Quantum Theory
Ch. 5 Gases
Ch. 8 Electron Configurations
Ch. 6 Thermochemistry
Ch. 9 Ionic and Covalent Bonding
Ch. 10 Molecular Geometry
Ch. 11 Liquids and Solids
Ch. 13 Materials
Ch. 14 Rates of Reaction
Ch. 12 Solutions
Ch. 15 Equilibrium
Ch. 16 Acids and Bases
Ch. 17 Acid–Base Equilibria
Ch. 18 Solubility Equilibria
Ch. 19 Thermodynamics
Ch. 20 Electrochemistry
Ch. 21 Nuclear Chemistry
(Ch. 14 is useful but not required)
Ch. 22–25 Descriptive Chemistry
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Sequence of Text Coverage
Chapters 16 through 18
Chapter 20
Chapters 21 through 25
7
Aqueous equilibria
Electrochemistry
Nuclear and descriptive chemistry (select chapters)
The following would be a similar three-quarter sequence:
Alternate Three-Quarter Sequence
(Gases just before liquids; thermodynamics before equilibrium)
First Term
Chapters 1 through 4
Chapters 7 and 8
Chapter 9
Basic chemistry
Atomic structure
Chemical bonding
Second Term
Chapter 10
Chapters 5, 11, 12
Chapter 14
Chapter 6
Chapter 19
Molecular structure
States of matter and solutions
Chemical kinetics
Thermochemistry
Thermodynamics (Sections 19.1 through 19.5)
Third Term
Chapter 15
Chapters 16 through 18
Chapter 20
Chapters 13 and 21
through 25
Introduction to equilibrium (plus Sections 18.6 and 18.7)
Aqueous equilibria
Electrochemistry
Nuclear and descriptive chemistry (select chapters)
Copyright © Houghton Mifflin Company. All rights reserved.
PART IV
Chapter Descriptions
In this part of the Instructor’s Resource Manual, we look at each chapter of the text, describing
the logic of its present placement and possible alternate positions for it. We also describe the
development of the chapter material, note any special points related to each chapter, and
indicate possible ways to abbreviate the material if this is necessary and seems appropriate.
This part of the manual should be especially useful in designing a syllabus for your course.
As the lectures proceed, you may need to delete material to keep to your schedule; the section
on abbreviation of the material for each chapter gives suggestions for ways to do this.
CHAPTER 1 Chemistry and Measurement
The chapter opens with a brief introduction to chemistry, followed by a discussion of
measurement and significant figures.
Placement of the Chapter
After the introductory material is presented, it is appropriate to discuss measurement because
of its importance in problem solving.
Development of the Chapter
Chapter 1 is divided into two parts. The first part is a brief introduction to chemistry. Section
1.1 describes the central role of chemistry in modern science and technology. Section 1.2
describes the relationship between experiment and explanation, and Section 1.3 illustrates this
material with the law of conservation of mass. The last section of the first part (Section 1.4) is
an introduction to the way the chemist describes matter.
The second part of the chapter concerns measurement. Section 1.5 discusses significant
figures and the limitations on experimental measurement. Section 1.6 describes SI units,
including prefixes and base units. Section 1.7 discusses units such as volume and density that
are derived from the SI base units. Finally, Section 1.8 describes the conversion of units and
dimensional analysis.
Special Notes
Students should understand the main features of the International System, including prefixes,
base units, and derived units, but they also need to be familiar with traditional units, such as
the Angstrom and the liter. In any case, conversion of units is emphasized, so students can
8
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
9
easily move from, say, picometers to angstroms. Use of the conversion-factor method appears
again later in the text, particularly in Chapter 3 (stoichiometry).
Abbreviation of the Material
Most of the material in Chapter 1 is basic, and you will probably want to assign all of it as
reading. However, students may be familiar with much of this material from a high school
course; so after a brief introduction to chemistry, you might begin your lectures with significant figures and units, stressing unit conversions.
CHAPTER 2 Atoms, Molecules, and Ions
The chapter introduces basic concepts needed in the course: atomic theory, atomic structure,
atomic weight, periodic table, molecular and ionic substances, formulas, organic compounds,
naming of compounds, and chemical equations.
Placement of the Chapter
The early placement of this chapter is necessary because it introduces basic concepts needed
for subsequent work.
Development of the Chapter
Atomic theory forms the thread of the chapter. Section 2.1 begins with atomic theory, and
Sections 2.2 and 2.3 discuss atomic and nuclear structure. Section 2.4 describes atomic weights
and how they are obtained. The periodic table is introduced in Section 2.5. Section 2.6, which
begins the second part of the chapter, discusses molecular and ionic substances and how to
write chemical formulas. Section 2.7 gives a brief discussion of organic compounds. The
second part of the chapter ends with Section 2.8 on the naming of compounds. The final part
of the chapter consists of Sections 2.9 and 2.10 on the writing and balancing of chemical
equations, respectively.
Special Notes
The periodic table is introduced in Section 2.5 but will be discussed again in Chapter 8 in
connection with electron configurations and periodicity of some atomic properties.
Abbreviation of the Material
The chapter introduces basic concepts that students may have some familiarity with from a
previous course, so lecture time could be directed to the salient points. Sections 2.2 (on the
structure of the atom) and 2.3 (on nuclear structure and isotopes) and the portion of Section
2.4 on mass spectrometry and atomic weights can be discussed later (just before Chapter 7,
Quantum Theory of the Atom), except for a brief mention of atomic structure and isotopes.
Nomenclature could be delayed until later, perhaps after Chapter 9 on bonding.
Copyright © Houghton Mifflin Company. All rights reserved.
10
PART IV
CHAPTER 3 Calculations with Chemical Formulas and Equations
This chapter uses the concepts of formula weight and the mole to obtain chemical formulas
and to perform calculations with chemical equations.
Placement of the Chapter
This material follows Chapter 2 naturally, emphasizing the mole concept. However, you may
wish to postpone it until you have covered the block of chapters on atomic and molecular
structure (Chapters 7 through 10).
Development of the Chapter
This chapter consists of three parts. The first part, Sections 3.1 and 3.2, introduces the concepts
of formula weight and mole. The second part, Sections 3.3 through 3.5, describes how a
formula is obtained from analytical data. The third part, Sections 3.6 through 3.8, uses the
chemical equation to do mole–mass calculations.
Special Notes
The conversion-factor method (factor-label method) is used consistently to solve the problems
in this chapter. To illustrate how to obtain a formula, we begin with analytical data for acetic
acid, the compound featured in the chapter opening, and calculate the mass percentages of
elements (Example 3.9) and then the molecular formula (Example 3.12).
Abbreviation of the Material
If you are pressed for time, you might omit Section 3.4 on determining the percentage of carbon
and hydrogen by combustion. Theoretical and percentage yields (last half of Section 3.8) may
also be omitted.
CHAPTER 4 Chemical Reactions: An Introduction
This chapter introduces the basic concepts of reactions, particularly those concerned with ionic
reactions in aqueous solution.
Placement of the Chapter
Early treatment of this material makes it possible to refer to various chemical reactions to
illustrate the applications of principles. Moreover, this chapter can be useful in developing
laboratory work and as background for reading some of the essays. However, the subject can
be postponed, and even then some instructors may cover only parts of the chapter (say the
section on ionic equations).
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
11
Development of the Chapter
Because of the importance of ionic reactions in general chemistry, we begin by describing the
ionic theory of solutions (Section 4.1) and how ionic equations are used to represent ionic
reactions (Section 4.2). The second part of the chapter discusses the three main types of
chemical reaction: precipitation reactions (Section 4.3), acid–base reactions (Section 4.4), and
oxidation–reduction reactions (Sections 4.5 and 4.6). Section 4.6 treats only simple oxidation–
reduction reactions; more complicated cases are discussed in Section 20.1. The third part,
Sections 4.7 and 4.8, introduces the concept of molar concentration and then describes
calculations pertaining to diluting a solution. The final part, Sections 4.9 and 4.10, looks at
some calculations in quantitative analysis.
Special Notes
Acids and bases will be discussed in detail in Chapter 16. Electrochemistry, Chapter 20, uses
the concepts of oxidation–reduction reactions, and Section 20.1 discusses the balancing of
more complex oxidation–reduction reactions.
Abbreviation of the Material
Sections 4.1 and 4.2 complement the treatment of chemical equations in Section 2.9, at the end
of the previous chapter. The remainder of Chapter 4 can be treated to the extent appropriate
to your course. Since acids and bases are discussed in detail in Chapter 16, you may wish to
give only a brief treatment here. You can easily delay discussion of oxidation–reduction
reactions to the second term, if you prefer.
The part of the chapter on solutions and molarity flows naturally from mole considerations
and from stoichiometry, and its inclusion here is useful in the laboratory. However, it may be
postponed until Chapter 12 on solutions, where other concentration units are discussed. The
sections on quantitative analysis could be omitted.
CHAPTER 5 The Gaseous State
This chapter treats the gas laws and the kinetic-molecular theory.
Placement of the Chapter
Opinion is divided on where this material is best placed. Stoichiometry and the measurement
of gas volumes played key roles in the historical development of chemistry. Thus, there is
precedent for placing gases with stoichiometry. Moreover, the early discussion of gases allows
you to use the gas laws in a range of laboratory experiments. In addition, the study of gases
gives an excellent opportunity to illustrate the interplay of experiment and theory. On the
other hand, others prefer to present this chapter on gases immediately before Chapter 11 on
liquids and solids, giving a unit on the states of matter.
Copyright © Houghton Mifflin Company. All rights reserved.
12
PART IV
Development of the Chapter
The chapter stresses the role of experiment and theory. The first part begins with the measurement of pressure (Section 5.1) and moves to the empirical gas laws (Section 5.2), which can
be combined into the ideal gas law (Section 5.3). The ideal gas law is then applied to
stoichiometry problems (Section 5.4). Section 5.5 on the law of partial pressures concludes the
first part of the chapter. The second part deals with the kinetic theory of gases. Section 5.6
states the postulates of the theory, relating them to experiment, and then gives a heuristic
derivation of the ideal gas law. Section 5.7 on molecular speeds and diffusion and effusion
covers deductions from kinetic theory. Finally, Section 5.8 discusses deviations from ideality
in the context of kinetic theory and introduces the van der Waals equation.
Special Notes
Boyle’s and Charles’s laws may be stated as proportionalities (V∝1/P, V∝T, V∝n). Perhaps
because of the symmetry, Avogadro’s law is also sometimes stated this way. However, this is
incorrect because the volume of any substance is proportional to moles. The essential content
of the law is that the molar volume is the same for all gases, which is the statement given on
page 187.
The “derivation” of the ideal gas law, given in Section 5.6, is purely heuristic, which seems
appropriate for general chemistry.
Abbreviation of the Material
Most of the material in this chapter is basic. The text is sufficiently detailed and the concepts
are easily grasped, so a minimum of lecture time is needed. The last two sections of the chapter
(Sections 5.7 and 5.8) can be covered to the extent that time allows.
CHAPTER 6 Thermochemistry
Heats of reaction and the concept of enthalpy are discussed. Concepts of entropy and free
energy are deferred until Chapter 19 (the first law of thermodynamics is discussed explicitly
in that chapter).
Placement of the Chapter
In this position, the chapter follows soon after stoichiometry, so this aspect of thermochemistry
can be emphasized. The placement of this chapter also allows you to underscore the role of
energy in chemistry before embarking on a discussion of chemical bonding. However, it is
possible to delay this chapter (for example, to precede Chapter 19), and the intervening
chapters (Chapters 7–18) were written with this in mind. In these chapters, ∆H is briefly
defined as the heat of reaction. (The treatment of the Born–Haber cycle in Chapter 9 requires
a knowledge of Hess’s law.)
Development of the Chapter
The first part of the chapter begins with a discussion of energy (Section 6.1) and then covers
the basic properties of heats of reaction. After some terms are defined (Section 6.2), the concept
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
13
of enthalpy is discussed (Section 6.3), followed by a discussion of thermochemical equations
(Section 6.4), the stoichiometry of heats of reaction (Section 6.5), and measurement of heats of
reaction (Section 6.6). In the second part of the chapter, heats of reactions are related to one
another by Hess’s law (Section 6.7), and the concept of enthalpies of formation is discussed
(Section 6.8). Thermochemistry is applied to fuels in the last section (6.9).
Special Notes
Enthalpy is introduced here as the heat of reaction at constant pressure, which is sufficient for
discussing the elementary aspects of thermochemistry. However, a brief discussion at the end
of Section 6.3 relates enthalpy to internal energy. Enthalpy is also defined precisely in Chapter
19, where the first law of thermodynamics is discussed and where the distinction between
internal energy and enthalpy is stressed.
Abbreviation of the Material
Sections 6.1 to 6.5 are basic; after covering those sections, you can abbreviate the material in
various ways. For example, Sections 6.6 and 6.9 might be omitted.
CHAPTER 7 Quantum Theory of the Atom
This chapter begins by presenting the properties of light as a prelude to describing Bohr’s
theory of the hydrogen atom. The chapter ends with a discussion of quantum numbers and
atomic orbitals. Electron configurations of atoms are dealt with in the next chapter.
Placement of the Chapter
This chapter could follow Chapter 2, which introduces atomic structure. However, the
intervening chapters on chemical reactions and stoichiometry make possible a wealth of
laboratory experiments, whereas it is more difficult to come up with experiments for Chapters
7 through 10.
Development of the Chapter
The chapter consists of two parts. The first part introduces the concepts of light waves (Section
7.1) and photons (Section 7.2), from which much of our information on atomic structure comes.
The first part concludes with a look at the Bohr theory of the hydrogen atom (Section 7.3). The
second part of the chapter introduces quantum mechanics (Section 7.4) and quantum numbers
and atomic orbitals (Section 7.5).
Special Notes
The section on the Bohr theory focuses on those aspects of the theory that carry over into
modern quantum theory: energy levels and transitions between levels. It does not emphasize
classical orbits.
Copyright © Houghton Mifflin Company. All rights reserved.
14
PART IV
Abbreviation of the Material
The most important section of the chapter is the concluding one on quantum numbers and
atomic orbitals. You could simply discuss the basic concepts of light waves, photons, the Bohr
theory, and quantum mechanics without emphasizing calculations. Then you could go to
Section 7.5, where you would concentrate on the quantum numbers.
CHAPTER 8 Electron Configurations and Periodicity
This chapter is a continuation of Chapter 7, which introduced the concepts of atomic orbitals
and quantum numbers. Here we look at the electron configurations of atoms and describe the
relationship between these configurations and the periodic behavior of the elements.
Placement of the Chapter
The chapter is a continuation of Chapter 7 and should follow it.
Development of the Chapter
In the first part of the chapter, we look at the electronic structure of atoms. Section 8.1 discusses
electron spin and the Pauli exclusion principle, Sections 8.2 and 8.3 describe the building-up
principle for obtaining the ground-state electron configurations of atoms, and Section 8.4
introduces Hund’s rule and orbital diagrams of atoms. In the second part of the chapter,
Sections 8.5 and 8.6 describe the periodic table and its relationship to the electron configurations of atoms. Section 8.7 gives brief descriptions of the main-group elements.
Special Notes
Theoretical calculations show that the 3d subshell is just below the 4s subshell in energy
throughout the transition elements, even though the 4s fills before the 3d. The explanation is
that the total energy of atoms, which is what determines the ground-state electron configurations, depends not only on the energy of the individual orbitals but also on the repulsions of
electrons. You can still keep the discussion elementary by referring to the order of filling or
building-up order of the atomic orbitals, noting that this order is the same as the order of
energy of the orbitals with some exceptions that occur when the sublevels are close in energy.
The building-up order reproduces most of the ground-state configurations correctly but
otherwise has no fundamental significance. By ordering the subshells of a ground-state
configuration by the principal quantum number, you obtain the valence-shell configuration
at the far right. Also, you place the most easily ionized electrons at the far right. For example,
if you write the electron configuration for iron as 1s22s22p63s23p63d64s2, the configuration of
Fe2+ is written by taking away the 4s electrons.
The chapter emphasizes how to obtain the electron configurations of atoms from the
position of the element in the periodic table. This helps students learn the relationship between
the configurations and the periodic table.
Abbreviation of the Material
Most of the material given in this chapter is important for subsequent work. In particular,
students should be able to obtain the electron configuration and orbital diagram for an atom
and understand the relationship of these to the periodic table. Moreover, students need to
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
15
understand the concepts of ionization energy and electron affinity to understand the discussion of the ionic bond in the next chapter. One could omit the last section, which briefly
describes the main-group elements. Otherwise, lecture time can be saved by concentrating on
the salient points: electron configurations and orbital diagrams of the atoms, a brief description of the periodic table, and a brief discussion of ionization energy and electron affinity.
CHAPTER 9 Ionic and Covalent Bonding
The chapter discusses the elementary aspects of chemical bonding, concluding with sections
on bond properties.
Placement of the Chapter
The chapter builds on the concepts of atomic structure introduced in Chapters 7 and 8. Thus,
the concepts of ionization energy and electron affinity described toward the end of the
previous chapter flow smoothly into the subject of ionic bonding.
Development of the Chapter
The chapter begins with ionic bonding, describing ionic bonds (Section 9.1) and the electron
configurations of ions (Section 9.2). Section 9.3 discusses the concept of ionic radii. Then the
chapter looks at covalent bonding in Sections 9.4 and 9.5. A general method of writing Lewis
electron-dot formulas is given in Section 9.6. The writing of Lewis formulas is elaborated on
in Sections 9.7 through 9.9. Section 9.7 describes resonance and Section 9.8 discusses exceptions
to the octet rule. The final section on writing Lewis formulas (Section 9.9) introduces the
concept of formal charge and applies it to choosing the most appropriate Lewis formula. The
last sections cover bond properties. Section 9.10 discusses bond length and bond order, and
Section 9.11 discusses bond energy.
Special Notes
The electron-dot formula, as rough a description as it is, provides a lot of information in a
clear and simple fashion. It is introduced first for atoms and is used to describe ions. Then it
is used again when covalent bonding is described. The method given in Section 9.6 for writing
electron-dot formulas will work for most of the molecules encountered in general chemistry,
including exceptions to the octet rule. Note that you first distribute electrons to the atoms
surrounding the central atom (usually the most electropositive atom) to satisfy the octet rule
for them. However, the electrons on the central atom may or may not satisfy the octet rule.
Abbreviation of the Material
The most important material is in Sections 9.1, 9.2, and 9.4 through 9.7. The remainder of the
chapter can be covered to the extent that time permits.
Copyright © Houghton Mifflin Company. All rights reserved.
16
PART IV
CHAPTER 10 Molecular Geometry and Chemical Bonding Theory
This chapter covers more advanced bonding concepts than those presented in the previous
chapter, including molecular geometry, hybrid orbitals, and molecular orbital theory.
Placement of the Chapter
The chapter follows Chapter 9 logically, but it can be postponed. For example, you can discuss
it just before Chapter 22 on the descriptive chemistry of the main-group elements.
Development of the Chapter
The chapter begins with the VSEPR model (Section 10.1) because of its simplicity and its
reliance on electron-dot formulas, which were covered in the previous chapter. Section 10.2
relates dipole moment and molecular geometry. The next two sections (10.3 and 10.4) discuss
the valence bond description of the electronic structure of molecules. The last part of the
chapter, Sections 10.5 through 10.7, describes molecular orbital theory.
Special Notes
In describing the order of filling of molecular orbitals, we give the order at the bottom of page
404, but in the marginal note there we state that the order by energy of the σ2p orbital is below
that of the π2p orbital in the case of O2 and of F2. However, this change in order has no essential
effect on the electron configuration or on deductions of bond order or magnetic character.
Abbreviation of the Material
Discussion of the VSEPR model can be abbreviated to cover up to four electron pairs, omitting
five and six pairs. In that case, you simply omit the last portion of Section 10.1. The remainder
of the chapter can be condensed or omitted to suit your needs.
CHAPTER 11 States of Matter; Liquids and Solids
This chapter looks at the states of matter (particularly liquids and solids) and their transformations from one state to another.
Placement of the Chapter
Chapters 11 and 12 deal with matter in bulk and require some knowledge of chemical bonding.
Development of the Chapter
The chapter begins with a comparison of gases, liquids, and solids (Section 11.1). Then follow
two sections on changes of state: Section 11.2 on phase transitions and Section 11.3 on phase
diagrams. Changes of state are discussed near the beginning of the chapter because they are
some of the most important properties of liquids and solids. The next section describes some
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
17
additional properties of liquids (Section 11.4); these properties are then explained in terms of
intermolecular forces (Section 11.5). By describing the properties before intermolecular forces,
we stress that experiment precedes explanation. The last part of the chapter describes the solid
state: types of solids (Section 11.6), crystalline solids (Sections 11.7 through 11.9), and determining crystal structure by x-ray diffraction (Section 11.10).
Abbreviation of the Material
You might choose to omit discussion of the Clausius–Clapeyron equation in Section 11.2 and
phase diagrams in Section 11.3. In the part on the solid state, you might omit Section 11.8 and
perhaps Sections 11.9 and 11.10.
CHAPTER 12 Solutions
This chapter looks at solution formation, colligative properties, concentration units, and
colloids.
Placement of the Chapter
The chapter continues the discussion of matter in bulk begun in Chapter 11.
Development of the Chapter
The chapter begins by looking at the types of solutions (Section 12.1), then describes the
solution process (Section 12.2) and the effects of temperature and pressure on solubility
(Section 12.3). In preparation for a discussion of colligative properties, Section 12.4 describes
various units of concentration. Then Sections 12.5 through 12.8 look at colligative properties.
The final section of the chapter describes colloids.
Special Notes
Some textbooks apply Le Chatelier’s principle and heats of solution to predict the temperature
dependence of the solubility of salts. The difficulty in doing this has been discussed in the
literature (G. M. Bodner, J. Chem. Educ. 1980, 57, 117; see also R. Treptow, J. Chem. Educ. 1984,
61, 499). Essentially, the problem is that what is required is the differential heat of solution at
saturation, whereas what we usually have in mind is an integral heat of solution. Note, for
example, that NaOH is more soluble with increasing temperature even though the (integral)
heat of solution is negative. It seems best simply to state that most ionic substances are more
soluble at higher temperature, noting a few exceptions, and leave it at that.
Abbreviation of the Material
You could concentrate your attention on Sections 12.4 through 12.8, omitting or condensing
the material in the rest of the chapter.
Copyright © Houghton Mifflin Company. All rights reserved.
18
PART IV
CHAPTER 13 Materials of Technology
This chapter looks at some of the metallic and nonmetallic materials of modern technology.
For example, the chapter briefly discusses nanotechnology, in which one studies materials
with a view toward developing useful applications.
Placement of the Chapter
The chapter follows the basic chapters (1 to 12) describing the structure of matter. So, it
provides an opportunity to apply the concepts just learned. Alternately, the chapter could be
treated with the descriptive chapters at the end of the course.
Development of the Chapter
The chapter is divided into two parts, a part on metals (Sections 13.1 to 13.3) and a part on
nonmetallic materials (Sections 13.4 to 13.8). Section 13.1 describes the natural sources of the
metallic elements, Section 21.2 discusses metallurgy, and Section 21.3 explains the bonding in
metals in terms of molecular orbital theory. Section 13.4 describes the different forms of carbon,
diamond, graphite, and the fullerenes, materials that have important uses in modern technology. Section 13.5 discusses the theory of semiconductors, which are the basis of solid-state
electronics devices. Section 13.6 describes some chemistry of silicon (the basic material in
semiconductor devices), silica, and the silicates. The final two sections of the chapter, Sections
13.7 and 13.8, cover ceramics and composites (a material constructed of two or more different
kinds of materials).
Abbreviation of the Material
Various selections of material are possible. Some of the frontiers work has been in the area of
nonmetallic materials, so you might restrict yourself to a selection from Sections 13.4 to 13.8.
CHAPTER 14 Rates of Reaction
This chapter looks at some important questions concerning chemical reactions: how fast do
chemical reactions occur, what factors affect this rate, and how do reactions occur at the
molecular level?
Placement of the Chapter
Although not essential for the equilibrium chapters that follow, chemical kinetics can give
some insight into chemical equilibrium. An alternate position for this discussion would be
after Chapter 20 and before Chapter 21. Early drafts of the book placed the chapter here, so
there is no difficulty with this order.
Development of the Chapter
The chapter has been written to stress the experimental basis of the subject. It begins with the
definition of reaction rate (Section 14.1) and its experimental measurement (Section 14.2). The
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
19
next sections describe the dependence of rate on concentrations of substances (Section 14.3)
and how the concentrations vary with time (Section 14.4). The following two sections (14.5
and 14.6) discuss how rates of reaction vary with temperature. The second part of the chapter
looks at reaction mechanisms, first discussing elementary reactions (Section 14.7) and then
showing how the reaction mechanism is related to the rate law (Section 14.8). The last section
(14.9) describes catalysis, explaining it in terms of the reaction mechanism.
Special Notes
Chemical kinetics can be a rather abstract subject. The treatment given here emphasizes
experimental results in order to reduce this abstract character. Reaction mechanisms are
carefully described as explanations of experimental observations, and the provisional status
of these explanations is emphasized.
Abbreviation of the Material
If time is short, you can pick and choose topics. Sections 14.1 and 14.3 through 14.5 are
important ones to cover. Then, after a brief introduction to mechanisms, Section 14.9 on
catalysis would complete the discussion of the factors affecting reaction rates.
CHAPTER 15 Chemical Equilibrium
This chapter begins a block of chapters dealing with chemical equilibrium. The method used
to solve equilibrium problems is described here, and that method is used uniformly throughout the chapters on equilibrium calculations. Gaseous reactions are used to illustrate the
principles.
Placement of the Chapter
Although this placement of equilibrium is a typical one, an alternate sequence in which
thermodynamics precedes chemical equilibrium is described in Part III.
Development of the Chapter
The chapter begins with three sections (15.1 through 15.3) describing chemical equilibrium
and defining the equilibrium constant. The second part of the chapter (Sections 15.4 through
15.6) discusses the use of the equilibrium constant. Thus, Section 15.5 introduces the concept
of reaction quotient, and Section 15.6 describes how to use the equilibrium constant to
calculate equilibrium concentrations. The last three sections of the chapter (Sections 15.7
through 15.9) discuss how changing the conditions in a reaction affects the yield of product.
Special Notes
Section 15.1 introduces chemical equilibrium as a dynamic equilibrium. This discussion is
self-contained and does not rely on Chapter 14. A brief subsection in Section 15.2 (Equilibrium—A Kinetics Argument) does use the concepts of reaction rate from Chapter 14 to obtain
the equilibrium constant, and you may want to omit it if you have not covered Chapter 14.
Copyright © Houghton Mifflin Company. All rights reserved.
20
PART IV
Example 15.1 asks for the equilibrium amounts of substances given the starting amounts
and the amount of one substance in the equilibrium mixture. The problem is essentially one
in stoichiometry, but it is set up in the way equilibrium problems will be set up throughout
(with a table of starting, change, and equilibrium amounts). Thus, the example forms a bridge
from stoichiometry, which students are familiar with, to equilibrium problems. The first
example of a calculation of equilibrium concentrations from the equilibrium constant is given
in Example 15.7. It is described as a three-step problem: set up a table with starting, change,
and equilibrium concentrations; substitute the expressions for equilibrium concentrations into
the equilibrium equation; solve the equation. Note that the table is set up to parallel the
chemical equation, with coefficients of x corresponding to coefficients in the chemical equation.
Note that the common statement of Le Chatelier’s principle in terms of “stresses” is too
vague and may give the wrong result unless the stresses are restricted to intensive variables.
See R. S. Treptow, J. Chem. Educ. 1980, 57, 417–420; see also I. N. Levine, Physical Chemistry, 3rd
ed.; Wiley: New York, 1988; p. 186.
Abbreviation of the Material
Because of the importance of the topic, most of the chapter should be covered if possible. Each
section gives added insight into the concept of equilibrium. However, a basic treatment would
include the introduction to equilibrium and the equilibrium constant (Sections 15.1 through
15.3), plus calculations with Kc (Section 15.6). Le Chatelier’s principle applied to adding or
removing substances (Section 15.7) gives students a qualitative way of looking at such things
as the common-ion effect (treated later in Sections 17.5 and 18.2). The reaction quotient,
introduced in Section 15.5, is used to discuss precipitation in Chapter 18, but the discussion
there (Section 18.3) will stand on its own.
CHAPTER 16 Acids and Bases
This chapter discusses the three main acid–base concepts and introduces the pH concept
needed for the next chapter.
Placement of the Chapter
The chapter consists of three parts: acid–base concepts, acid and base strengths, and self-ionization of water and pH. Section 16.1 reviews the Arrhenius concept, which was discussed in
Chapter 4. Section 16.2 describes the Brønsted–Lowry concept in more detail than was done
in Chapter 4. Section 16.3 describes the Lewis concept of acids and bases. After describing
these acid–base concepts, Sections 16.4 and 16.5 discuss the relative strengths of acids and
bases and the relationship of acid strength to molecular structure. The last sections (16.6
through 16.8) discuss the concepts of self-ionization of water and of pH, which are central to
understanding the following chapter.
Special Notes
Acids and bases were discussed in Chapter 4. Chapter 16 is a more complete discussion,
including the pH concept.
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
21
Abbreviation of the Material
If you spent a fair bit of time on acids and bases in lecturing on Chapter 4, you could treat the
first part of Chapter 16 briefly. Sections 16.3 through 16.5 are optional. The sections on
self-ionization and pH are important for the next chapter.
CHAPTER 17 Acid–Base Equilibria
This chapter looks quantitatively at acid–base equilibria, including hydrolysis, common-ion
effect, and buffers.
Placement of the Chapter
The chapter could follow rather than precede Chapter 18 on solubility. In that case, Sections
18.4 and 18.7 should be postponed until Ka is introduced.
Development of the Chapter
The chapter is organized in two parts. The first part deals with solutions containing a weak
acid or base, and the second part with solutions of a weak acid or base to which a common
ion is added. Sections 17.1 through 17.4 treat acid–base equilibria in a unified way, progressing
from monoprotic to polyprotic acids, then to bases and to salts. Once the method described
in Examples 17.2 and 17.3 for weak acids is mastered, the treatment of base and salt solutions
is seen to be essentially the same. Section 17.5 looks specifically at the common-ion effect;
then Section 17.6 discusses buffers. The final section looks at pH changes during acid–base
titrations.
Special Notes
The unity of the subject of equilibrium calculations has been stressed; the general method was
described in Example 15.7.
To obtain the 5% rule stated just before Example 17.3, start with the equilibrium equation
(C is the acid concentration).
Ka =
x2
C−x
Then,
x2 = Ka(C − x)
KaC [1 − x/(2C)]
KaC (1 − x/C)1/2 艑 √

x=√

The expression on the right was obtained by retaining only the first two terms of a power
series expansion of [1 – x/(2C)]1/2. If you drop the term in x in this expression (equal to
KaC[x/(2C)], the solution of the equation is equivalent to dropping x in the denominator of
Copyright © Houghton Mifflin Company. All rights reserved.
22
PART IV
the equilibrium equation, in which case x = (KaC)1/2. The fractional error in this approximation
is
Ka/C

KaC √
x √
=
=
2C
2
2C
If the error is to be less than or equal to 5%,
√

Ka/C
× 100 ≤ 5
2
which is equivalent to the rule in the text.
Abbreviation of the Material
The most important material in the chapter concerns acid–base ionization equilibria (Sections
17.1 and 17.3). The remaining sections can be emphasized or not depending on the time
available.
CHAPTER 18 Solubility and Complex-Ion Equilibria
The chapter deals with solubility and precipitation, as well as complex-ion formation and its
effect on solubility.
Placement of the Chapter
This chapter can be treated before acid–base equilibria, except for Sections 18.4 and 18.7, which
should be postponed until Ka is discussed.
Development of the Chapter
Solubility equilibria are described in the first part of the chapter, starting with a discussion of
Ksp (Section 18.1) and following with the common-ion effect (Section 18.2), precipitation
calculations (Section 18.3), and the effect of pH on solubility (Section 18.4). The next part looks
at complex-ion equilibria, discussing the formation of complex ions (Section 18.5) and the
effect of complex-ion formation on solubility (Section 18.6). The final section of the chapter
briefly describes the qualitative analysis scheme for metal ions.
Special Notes
Both Chapters 17 and 18 are important to understanding the qualitative analysis of metal ions,
but Chapter 18 is especially pertinent. Note the inclusion of fractional precipitation in Section
18.3 and the separation of metal ions by sulfide precipitation in Section 18.4, both of which
are useful in discussing qualitative analysis.
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
23
Abbreviation of the Material
The most important topics involving Ksp are covered in Sections 18.1 through 18.3 (to the end
of Example 18.7). The coverage of the rest of the chapter can be tailored to individual
circumstances. Thus, if the course is accompanied by extensive laboratory work in qualitative
analysis, Section 18.7 could be omitted.
CHAPTER 19 Thermodynamics and Equilibrium
This chapter looks at the spontaneity of a chemical reaction in terms of the thermodynamic
concepts of entropy and free energy. The equilibrium constant is related to free energy in the
last two sections.
Placement of the Chapter
The chapter has been placed just before the chapter on electrochemistry and after the block of
chapters on chemical equilibrium, but it could be covered earlier. For example, it could easily
follow Chapter 14, or it could even be covered before Chapter 14 if Sections 19.6 and 19.7
(which refer to the equilibrium constant) are postponed until the equilibrium constant is
defined. Chapter 19 has been divided into three parts, with the last part treating free energy
and equilibrium constants, to facilitate this rearrangement.
Development of the Chapter
The chapter begins with a section that reviews thermochemistry and introduces the first law
of thermodynamics. It also gives the calculation of ∆H for the preparation of urea from NH3
and CO2. Later, we calculate ∆S and ∆G for this reaction, which was introduced in the chapter
opening, as an illustration of thermodynamic concepts. Entropy is discussed in Sections 19.2
and 19.3, and free energy in the remainder of the chapter. Spontaneity of a reaction and free
energy are related in Section 19.4, and further interpretation of free energy is given in Section
19.5. Free energy is related to the equilibrium constant in Section 19.6, and then the change of
free energy with temperature is discussed in Section 19.7.
Special Notes
The emphasis of the chapter is on using thermodynamics to obtain a criterion of spontaneity
for a chemical reaction. This provides the unifying thread of the chapter, giving a clean
presentation without getting bogged down in detail. The criterion that the quantity ∆H – ∆S
is negative for a spontaneous change is developed immediately from the second law of
thermodynamics (at the end of Section 19.2). This sets the stage for the next two sections: first,
how to find ∆S; then, defining free energy as a convenient way to express this criterion of
spontaneity in terms of a single quantity.
Copyright © Houghton Mifflin Company. All rights reserved.
24
PART IV
Abbreviation of the Material
The basic sections of the chapter are 19.2 through 19.4. The remaining sections may be covered
to the extent desired. Section 19.5 simply amplifies the meaning of free energy, and Section
19.6 links free energy to the equilibrium constant.
CHAPTER 20 Electrochemistry
This chapter looks at electrochemistry and what it has to say about the spontaneity of reaction
and about the electrolytic decomposition of a substance.
Placement of the Chapter
The chapter has been placed after Chapter 19 to make use of the concepts of spontaneous
reaction and free energy introduced there.
Development of the Chapter
The chapter is divided into three parts. The first part (Section 20.1) treats the balancing of
oxidation–reduction reactions. The second part of the chapter deals with voltaic cells. Section
20.2 describes the construction of a voltaic cell, and Section 20.3 introduces notation used to
represent such a cell. Sections 20.4 and 20.5 discuss the concept of cell emf, Section 20.6 relates
the cell emf to the reaction equilibrium, and Section 20.7 looks at the dependence of cell emf
on reactant concentrations. The final section (20.8) of this part describes some commercial
voltaic (galvanic) cells.
The third part of the chapter deals with electrolytic cells. Section 20.9 discusses the
electrolysis of molten salts; Section 20.10 describes the electrolysis of aqueous solutions. The
last section (20.11) treats the stoichiometry of electrolysis.
Special Notes
Electrolytic cells have been treated at the end of the chapter to use the concept of electrode
potential in discussing the ease of electrolytic decomposition in Section 20.10. However,
Section 20.11 on the stoichiometry of electrolysis could be moved to the beginning of the
chapter.
Abbreviation of the Material
The basic material is covered in Sections 20.2 through 20.5 and 20.11. Other sections may be
omitted if you are pressed for time.
CHAPTER 21 Nuclear Chemistry
This chapter looks at nuclear reactions, the rate of radioactive decay, and the energy change
that results from nuclear fission and nuclear fusion.
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
25
Placement of the Chapter
This chapter returns to rates of reaction (introduced in Chapter 14) after the block of equilibrium chapters (Chapters 15–20). Chapter 14 provides background for discussing rate of
radioactive decay. However, the pertinent equations for rate of radioactive decay are given in
the text, so the chapter could be covered before chemical kinetics if desired. You could easily
treat the material on nuclear reactions in Sections 21.1 and 21.2 just after the discussion of
nuclear structure in Section 2.3 if that seems desirable.
Development of the Chapter
The chapter is divided into two parts, the first one looking at various nuclear reactions and
characteristics of radioactivity and the second looking at the energy of nuclear reactions. The
first part begins by describing the types of nuclear reactions: radioactivity (Section 21.1) and
nuclear bombardment reactions (Section 21.2). The chapter then discusses ways to detect the
radiations from radioactive decay (Section 21.3). The next section (21.4) looks at the rate of
radioactive decay and the half-life of a radioactive isotope. Section 21.5 discusses chemical
and medical applications of radioactive isotopes. The second part of the chapter begins by
explaining the concepts of mass–energy equivalence and nuclear binding energy (Section
21.6). The chapter ends with a discussion of nuclear fission and fusion (Section 21.7).
Special Notes
We have avoided using the expression “conversion of mass to energy,” which is misleading.
Consider a system consisting of an electron and a positron. The rest mass of this system
changes from 2me to zero if the two particles collide and annihilate one another. But the
surroundings increase in mass by 2me by absorbing the two gamma-ray photons that are
emitted. Therefore, the total mass remains constant. Similarly, the total energy remains
constant. The equation E = mc2 simply states that if a system has a given quantity of mass, it
has a quantity of energy equal to mc2. See R. P. Bauman, J. Chem. Educ. 1966, 43, 366, and R. S.
Treptow, J. Chem. Educ. 1986, 63, 103.
Abbreviation of the Material
The basic material is in Sections 21.1, 21.2, 21.4, and 21.6. Other sections may be omitted.
CHAPTER 22 Chemistry of the Main-Group Elements
This chapter looks at the descriptive chemistry of the main-group or representative elements.
Placement of the Chapter
The principles introduced in the previous chapters are applied to the chemistry of the elements
in Chapters 22 through 25. Much of this material could be covered earlier, however.
Copyright © Houghton Mifflin Company. All rights reserved.
26
PART IV
Development of the Chapter
The first section of the chapter (22.1) deals with the main-group elements in general before
going on to the sections describing the families of elements. The first part of the chapter
discusses the main-group metals (Sections 22.2 through 22.4). The second part of the chapter
discusses the main-group nonmetals (Sections 22.5 through 22.10).
Special Notes
The periodic table is used to correlate information about the main-group elements. Section
22.1 provides the overview for this discussion. Within each group, we concentrate on one or
two elements to focus the discussion. By discussing only one element in a section, you can
pick and choose sections to meet your needs. You can include only a few elements or all of
those described.
Abbreviation of the Material
You can pick as many or as few elements as you wish from this chapter.
CHAPTER 23 The Transition Elements
This chapter looks at some descriptive chemistry of the transition elements and at the structure
of coordination compounds.
Placement of the Chapter
This chapter continues the discussion of the descriptive chemistry of the elements, which
began in Chapter 22.
Development of the Chapter
The chapter consists of two parts, the first looking at the properties of the transition elements
and the second looking at complexes and coordination compounds. Section 23.1 describes the
periodic trends seen in the properties of the transition elements, and Section 23.2 looks at some
chemistry of chromium and copper. Section 23.3 begins the second part by discussing the
formation and structure of complexes; basic terms are defined here. Section 23.4 introduces
the nomenclature of coordination compounds, and Section 23.5 discusses isomerism. The last
two sections look at the electronic structure of transition-metal complexes in terms of valence
bond theory (Section 23.6) and crystal field theory (Section 23.7).
Abbreviation of the Material
You can tailor the material to the needs of your particular course. If you wish to concentrate
on coordination compounds, you can begin the chapter with Section 23.3. In looking at the
electronic structure of complexes, you could limit the treatment to octahedral complexes,
omitting any discussion of tetrahedral and square planar complexes.
Copyright © Houghton Mifflin Company. All rights reserved.
Chapter Descriptions
27
CHAPTER 24 Organic Chemistry
This chapter gives a brief introduction to organic chemistry.
Placement of the Chapter
The chapter is part of the block of chapters covering the descriptive chemistry of the elements.
Properties of various organic compounds were treated in earlier chapters, but this is the first
organized treatment of the subject other than what was done in Section 2.7. It is required
background for the next chapter on polymer molecules. Although it occurs near the end of
the book, this discussion of organic chemistry is easily moved to an earlier position in the
general chemistry course.
Development of the Chapter
The first section (24.1) discusses the bonding of carbon. After that the chapter is divided
logically into the study of hydrocarbons and derivatives of hydrocarbons. In the first part,
Sections 24.2 through 24.4 explores the structure of different series of hydrocarbons. Section
24.5 then describes the naming of these hydrocarbons. In the second part of the chapter,
Sections 24.6 and 24.7 discusses oxygen and nitrogen derivatives of the hydrocarbons.
Abbreviation of the Material
One possibility for an abridged treatment would cover Sections 24.1, 24.2, 24.3, and 24.6.
CHAPTER 25 Polymer Materials: Synthetic and Biological
This chapter is a brief introduction into polymers.
Placement of the Chapter
The chapter relies on the discussion of organic chemistry in Chapter 24.
Development of the Chapter
The chapter is divided into two parts, one on synthetic polymers and the other on biological
polymers, with an emphasis on proteins and their biosynthesis starting with the genetic code.
In the first part of the chapter, Section 25.2 describes the synthesis of organic polymers. Section
25.3 describes electrical conducting polymers. In the second part of the chapter, 25.3 describes
proteins. Section 25.4 describes nucleic acids.
Abbreviation of the Material
You could choose to cover only one part of the chapter, either the one on synthetic polymers
or the one on biological polymers.
Copyright © Houghton Mifflin Company. All rights reserved.
PART V
Operational Skills Masterlist
Chapter 1 Chemistry and Measurement
Examples
Exercises
Problems
1. Using the law of conservation of
mass Given the masses of all substances
in a chemical reaction except one, calculate the mass of this one substance.
1.1
1.1
1.31, 1.32,
1.33, 1.34
2. Using significant figures in calculations Given an arithmetic setup, report
the answer to the correct number of significant figures and round it properly.
1.2
1.3
1.55, 1.56
3. Converting from one temperature
scale to another Given a temperature
reading on one scale, convert it to another scale—Celsius, Kelvin, or Fahrenheit.
1.3
1.5
1.63, 1.69,
1.65, 1.66
4. Calculating the density of a substance Given the mass and volume of a
substance, calculate the density.
1.4
1.6
1.67, 1.68,
1.69, 1.70
5. Using the density to relate mass and
volume Given the mass and density of a
substance, calculate the volume; or given
the volume and density, calculate the
mass.
1.5
1.7
1.71, 1.72,
1.73, 1.74
6. Converting units Given an equation
relating one unit to another (or a series of
such equations), convert a measurement
expressed in one unit to a new unit.
1.6, 1.7, 1.8
1.8, 1.9,
1.10
1.75, 1.76,
1.77, 1.78,
1.79, 1.80,
1.81, 1.82,
1.83, 1.84
28
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Chapter 2 Atoms, Molecules, and Ions
Examples
Exercises
Problems
1. Writing nuclide symbols Given the
number of protons and neutrons in a nucleus, write its nuclide symbol.
2.1
2.1
2.41, 2.42
2. Determining atomic weight from isotopic masses and fractional abundances Given the isotopic masses (in
atomic mass units) and fractional isotopic
abundances for a naturally occurring element, calculate its atomic weight.
2.2
2.2
2.45, 2.46,
2.47, 2.48
3. Writing an ionic formula, given the
ions Given the formulas of a cation and
an anion, write the formula of the ionic
compound of these ions.
2.3
2.4
2.69, 2.70
4. Writing a name of a compound from
its formula, or vice versa Given the
name of a simple compound (ionic, binary molecular, acid, or hydrate), write
the name, or vice versa.
2.4, 2.5,
2.6, 2.7,
2.10, 2.11
2.5, 2.6, 2.7,
2.8, 2.11,
2.12
2.71, 2.72,
2.73, 2.74,
2.77, 2.78,
2.79, 2.80,
2.85, 2.86,
2.87, 2.88
5. Writing the name of a binary molecular compound from its molecular
model Given the molecular model of a
binary compound, write the name.
2.8
2.9
2.81, 2.82
6. Writing the name and formula of an
anion from the acid Given the name
and formula of an oxoacid, write the
name and formula of the oxoanion; or
from the name and formula of the oxoanion, write the formula and name of the
oxoacid.
2.9
2.10
2.83, 2.84
7. Balancing simple equations Given
the formulas of the reactants and products in a chemical reaction, obtain the
coefficients of the balanced equation.
2.12
2.13
2.91, 2.92
Copyright © Houghton Mifflin Company. All rights reserved.
29
30
PART V
Chapter 3 Calculations with Chemical
Formulas and Equations
Examples
Exercises
Problems
1. Calculating the formula weight from
a formula or molecular model Given
the formula of a compound and a table of
atomic weights, calculate the formula
weight.
3.1, 3.2
3.1, 3.2
3.21, 3.22,
3.23, 3.24
2. Calculating the mass of an atom or
molecule Using the molar mass and
Avogadro’s number, calculate the mass of
an atom or molecule in grams.
3.3
3.3
3.27, 3.28,
3.29, 3.30
3. Converting moles of substance to
grams, and vice versa Given the moles
of a compound with a known formula,
calculate the mass. Or, given the mass of
a compound with a known formula, calculate the moles.
3.4, 3.5
3.4, 3.5
3.31, 3.32,
3.33, 3.34,
3.35, 3.36
4. Calculating the number of molecules
in a given mass Given the mass of a
sample of a molecular substance and its
formula, calculate the number of molecules in the sample.
3.6
3.6
3.39, 3.40,
3.41, 3.42
5. Calculating the percentage composition from the formula Given the formula of a compound, calculate the mass
percentages of the elements in it.
3.7
3.7
3.51, 3.52,
3.53, 3.54
6. Calculating the mass of an element
in a given mass of compound Given
the mass percentages of elements in a
given mass of a compound, calculate the
mass of any element.
3.8
3.8
3.55, 3.56
7. Calculating the percentages of C and
H by combustion Given the masses of
CO2 and H2O obtained from the combustion of a known mass of a compound of
C, H, and O, compute the mass percentages of each element.
3.9
3.9
3.57, 3.58
Note: A table of atomic weights is necessary for
most of these skills.
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Examples
Exercises
Problems
8. Determining the empirical formula
from percentage composition Given the
masses of elements in a known mass of
compound, or given its percentage composition, obtain the empirical formula.
3.10, 3.11
3.10, 3.11
3.59, 3.60,
3.61, 3.62,
3.63, 3.64
9. Determining the molecular formula
from percentage composition and molecular weight Given the empirical formula and molecular weight of a
substance, obtain its molecular formula.
3.12
3.12
3.67, 3.68,
3.69, 3.70
10. Relating quantities in a chemical
equation Given a chemical equation
and the amount of one substance, calculate the amount of another substance involved in the reaction.
3.13, 3.14
3.14, 3.15,
3.16
3.77, 3.78,
3.79, 3.80,
3.81, 3.82,
3.83, 3.84
11. Calculating with a limiting reactant Given the amounts of reactants and
the chemical equation, find the limiting
reactant; then calculate the amount of a
product.
3.15, 3.16
3.17, 3.18
3.85, 3.86,
3.87, 3.88
Copyright © Houghton Mifflin Company. All rights reserved.
31
32
PART V
Chapter 4 Chemical Reactions: An Introduction
Examples
Exercises
Problems
1. Using the solubility rules Cover the
formula of an ionic compound, predict its
solubility in water.
4.1
4.1
4.23, 4.24
2. Writing net ionic equations Given a
molecular equation, write the corresponding net ionic equation.
4.2
4.2
4.27, 4.28
3. Deciding whether precipitation will
occur Using solubility rules, decide
whether two soluble ionic compounds
will react to form a precipitate. If they
will, write the net ionic equation.
4.3
4.3
4.31, 4.32,
4.33, 4.34
4. Classifying acids and bases as strong
or weak Given the formula of an acid or
base, classify it as strong or weak.
4.4
4.4
4.35, 4.36
5. Writing an equation for a neutralization Given an acid and a base, write the
molecular equation and then the net ionic
equation for the neutralization reaction.
4.5
4.5
4.37, 4.38,
4.39, 4.40
6. Writing an equation for a reaction
with gas formation Given the reaction
between a carbonate, sulfide, or sulfite
and an acid, write the molecular and net
ionic equations.
4.6
4.7
4.45, 4.46,
4.47, 4.48
7. Assigning oxidation numbers Given
the formula of a simple compound or
ion, obtain the oxidation numbers of the
atoms, using the rules for assigning oxidation numbers.
4.7
4.8
4.49, 4.50,
4.51, 4.52
8. Balancing equations by the half-reaction method Given the skeleton equation for an oxidation–reduction equation,
complete and balance it.
4.8
4.9
4.59, 4.60
9. Calculating molarity from mass and
volume Given the mass of the solute
and the volume of the solution, calculate
the molarity.
4.9
4.10
4.61, 4.62,
4.63, 4.64
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Examples
Exercises
Problems
10. Using molarity as a conversion factor Given the volume and molarity of a
solution, calculate the amount of solute.
Or, given the amount of solute and the
molarity of a solution, calculate the
volume.
4.10
4.12
4.65, 4.66,
4.67, 4.68,
4.69, 4.70,
4.71, 4.72
11. Diluting a solution Calculate the
volume of solution of known molarity required to make a specified volume of solution with different molarity.
4.11
4.13
4.73, 4.74
12. Determining the amount of species
by gravimetric analysis Given the
amount of a precipitate in a gravimetric
analysis, calculate the amount of a related species.
4.12
4.14
4.77, 4.78
13. Calculating the volume of reactant
solution needed Given the chemical
equation, calculate the volume of solution of known molarity of one substance
that just reacts with a given volume of solution of another substance.
4.13
4.15
4.83, 4.84
14. Calculating the quantity of substance in a titrated solution Calculate
the mass of one substance that reacts
with a given volume of known molarity
of solution of another substance.
4.14
4.16
4.85, 4.86
Copyright © Houghton Mifflin Company. All rights reserved.
33
34
PART V
Chapter 5 The Gaseous State
Examples
Exercises
Problems
1. Relating liquid height and pressure
Given the density of a liquid used in a barometer or manometer and the height of
the column of liquid, obtain the pressure
reading in mmHg.
5.1
5.1
5.31, 5.32
2. Using the empirical gas laws Given
an initial volume occupied by a gas, calculate the final volume when the pressure changes at fixed temperature; when
the temperature changes at fixed pressure; and when both pressure and temperature change.
5.2, 5.3, 5.4
5.2, 5.3, 5.4
5.33, 5.34,
5.35, 5.36,
5.39, 5.40,
5.41, 5.42,
5.45, 5.46
3. Deriving empirical gas laws from the
ideal gas law Starting from the ideal gas
law, derive the relationship between any
two variables.
5.5
5.5
5.49, 5.50
4. Using the ideal gas law Given any
three of the variables P, V, T, and n for a
gas, calculate the fourth one from the
ideal gas law.
5.6
5.6
5.51, 5.52,
5.53, 5.54,
5.55, 5.56
5. Relating gas density and molecular
weight Given the molecular weight, calculate the density of a gas for a particular
temperature and pressure; or, given the
gas density, calculate the molecular
weight.
5.7, 5.8
5.7, 5.8
5.57, 5.58,
5.59, 5.60,
5.61, 5.62,
5.63, 5.64
6. Solving stoichiometry problems involving gas volumes Given the volume
(or mass) of one substance in a reaction,
calculate the mass (or volume) of another
produced or used up.
5.9
5.9
5.67, 5.68,
5.69, 5.70,
5.71, 5.72
7. Calculating partial pressures and
mole fractions of a gas in a mixture
Given the masses of gases in a mixture,
calculate the partial pressures and mole
fractions.
5.10
5.10
5.75, 5.76,
5.77, 5.78
8. Calculating the amount of gas collected over water Given the volume, total pressure, and temperature of gas
collected over water, calculate the mass
of the dry gas.
5.11
5.11
5.81, 5.82
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Examples
Exercises
Problems
9. Calculating the rms speed of gas
molecules Given the molecular weight
and temperature of a gas, calculate the
rms molecular speed.
5.12
5.12, 5.13
5.83, 5.84,
5.85, 5.86,
5.87, 5.88
10. Calculating the ratio of effusion
rates of gases Given the molecular
weights of two gases, calculate the ratio
of rates of effusion; or, given the relative
effusion rates of a known and an unknown gas, obtain the molecular weight
of the unknown gas (as in Exercise 5.15).
5.13
5.14, 5.15
5.89, 5.90,
5.91, 5.92,
5.93, 5.94
11. Using the van der Waals equation
Given n, T, V, and the van der Waals constants a and b for a gas, calculate the pressure from the van der Waals equation.
5.14
5.16
5.95, 5.96
Copyright © Houghton Mifflin Company. All rights reserved.
35
36
PART V
Chapter 6 Thermochemistry
Examples
Exercises
Problems
1. Calculating kinetic energy Given
the mass and speed of an object, calculate
the kinetic energy.
6.1
6.1
6.37, 6.38,
6.39, 6.40
2. Writing thermochemical equations
Given a chemical equation, states of substances, and the quantity of heat absorbed or evolved for molar amounts,
write the thermochemical equation.
6.2
6.3
6.45, 6.46
3. Manipulating thermochemical equations Given a thermochemical equation,
write the thermochemical equation for
different multiples of the coefficients or
for the reverse reaction.
6.3
6.4
6.47, 6.48,
6.49, 6.50
4. Calculating the heat of reaction from
the stoichiometry Given the value of
∆H for a chemical equation, calculate the
heat of reaction for a given mass of reactant or product.
6.4
6.5
6.51, 6.52,
6.53, 6.54
5. Relating heat and specific heat
Given any three of the quantities q, s, m,
and ∆t, calculate the fourth one.
6.5
6.6
6.57, 6.58
6. Calculating ∆H from calorimetric
data Given the amounts of reactants and
the temperature change of a calorimeter
of specified heat capacity, calculate the
heat of reaction.
6.6
6.7
6.61, 6.62,
6.63, 6.64
7. Applying Hess’s law Given a set of
reactions with enthalpy changes, calculate ∆H for a reaction obtained from these
other reactions by using Hess’s law.
6.7
6.8
6.65, 6.66,
6.67, 6.68
8. Calculating the heat of phase transition from standard enthalpies of
formation Given a table of standard
enthalpies of formation, calculate the
heat of phase transition.
6.8
6.9
6.71, 6.72
9. Calculating the enthalpy of reaction
from standard enthalpies of formation
Given a table of standard enthalpies of
formation, calculate the enthalpy of reaction.
6.9
6.10, 6.11
6.73, 6.74,
6.75, 6.76,
6.77, 6.78
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Chapter 7 Quantum Theory of the Atom
Examples
Exercises
Problems
1. Relating wavelength and frequency
of light Given the frequency of light, calculate the wavelength, or vice versa.
7.1, 7.2
7.1, 7.2
7.29, 7.30,
7.31, 7.32
2. Calculating the energy of a photon
Given the frequency or wavelength of
light, calculate the energy associated with
one photon.
7.3
7.3
7.37, 7.38,
7.39, 7.40
3. Determining the wavelength or frequency of a hydrogen atom transition
Given the initial and final principal quantum numbers for an electron transition in
the hydrogen atom, calculate the frequency or wavelength of light emitted.
You need the value of RH.
7.4
7.4
7.43, 7.44,
7.45, 7.46
4. Applying the de Broglie relation
Given the mass and speed of a particle,
calculate the wavelength of the associated wave.
7.5
7.6
7.51, 7.52
5. Using the rules for quantum numbers Given a set of quantum numbers n,
l, ml, and ms, state whether that set is permissible for an electron.
7.6
7.7
7.63, 7.64
Copyright © Houghton Mifflin Company. All rights reserved.
37
38
PART V
Chapter 8 Electron Configurations
and Periodicity
Examples
Exercises
Problems
1. Applying the Pauli exclusion principle Given an orbital diagram or electron
configuration, decide whether it is possible or not, according to the Pauli exclusion principle.
8.1
8.1
8.35, 8.36,
8.37, 8.38
2. Determining the configuration of an
atom using the building-up principle
Given the atomic number of an atom,
write the complete electron configuration
for the ground state, according to the
building-up principle.
8.2
8.2
8.41, 8.42,
8.43, 8.44
3. Determining the configuration of an
atom using the period and group numbers Given the period and group for an
element, write the configuration of the
outer electrons.
8.3
8.3, 8.4
8.45, 8.46,
8.47, 8.48,
8.49, 8.50
4. Applying Hund’s rule Given the
electron configuration for the ground
state of an atom, write the orbital diagram.
8.4
8.5
8.51, 8.52
5. Applying periodic trends Using the
known trends and referring to a periodic
table, arrange a series of elements in order by atomic radius or ionization energy.
8.5, 8.6
8.6, 8.7
8.55, 8.56,
8.57, 8.58
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Chapter 9 Ionic and Covalent Bonding
Examples
Exercises
Problems
1. Using Lewis symbols to represent
ionic bond formation Given a metallic
and a nonmetallic main-group element,
use Lewis symbols to represent the transfer of electrons to form ions of noble-gas
configurations.
9.1
9.1
9.31, 9.32
2. Writing electron configurations of
ions Given an ion, write the electron
configuration. For an ion of a main-group
element, give the Lewis symbol.
9.2, 9.3
9.2, 9.3, 9.4
9.33, 9.34,
9.35, 9.36,
9.37, 9.38
3. Using periodic trends to obtain relative ionic radii Given a series of ions, arrange them in order of increasing ionic
radius.
9.4
9.7
9.43, 9.44
4. Using electronegativities to obtain
relative bond polarities Given the electronegativities of the atoms, arrange a series of bonds in order by polarity.
9.5
9.8
9.51, 9.52
5. Writing Lewis formulas Given the
molecular formula of a simple compound
or ion, write the Lewis electron-dot formula.
9.6, 9.7,
9.8, 9.10
9.9, 9.10,
9.11, 9.13
9.55, 9.56,
9.57, 9.58,
9.59, 9.60,
9.65, 9.66
6. Writing resonance formulas Given a
simple molecule with delocalized bonding, write the resonance description.
9.9
9.12
9.61, 9.62,
9.63, 9.64
7. Using formal charges to determine
the best Lewis formula Given two or
more Lewis formulas, use formal charges
to determine which formula best describes the electron distribution or gives
the most plausible molecular structure.
9.11
9.15
9.71, 9.72
8. Relating bond order and bond
length Know the relationship between
bond order and bond length.
9.12
9.17
9.77, 9.78
9. Estimating ∆H from bond energies
Given a table of bond energies, estimate
the heat of reaction.
9.13
9.18
9.79, 9.80
Copyright © Houghton Mifflin Company. All rights reserved.
39
40
PART V
Chapter 10 Molecular Geometry
and Chemical Bonding Theory
Examples
Exercises
Problems
1. Predicting molecular geometries
Given the formula of a simple molecule,
predict its geometry, using the VSEPR
model.
10.1, 10.2
10.1, 10.2
10.27, 10.28,
10.29, 10.30,
10.33, 10.34,
10.35, 10.36
2. Relating dipole moment and molecular geometry State what geometries of a
molecule AXn are consistent with the information that the molecule has a
nonzero dipole moment.
10.3
10.3, 10.4
10.37, 10.38,
10.39, 10.40
3. Applying valence bond theory
Given the formula of a simple molecule,
describe its bonding, using valence bond
theory.
10.4, 10.5,
10.6
10.5, 10.6,
10.7
10.41, 10.42,
10.43, 10.44,
10.47, 10.48,
10.49, 10.50,
10.51, 10.52
4. Describing molecular orbital configurations Given the formula of a diatomic
molecule obtained from first- or secondperiod elements, deduce the molecular
orbital configuration, the bond order, and
whether the molecular substance is diamagnetic or paramagnetic.
10.7, 10.8
10.9, 10.10
10.55, 10.56,
10.57, 10.58
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
41
Chapter 11 States of Matter; Liquids and Solids
Examples
Exercises
Problems
1. Calculating the heat required for a
phase change of a given mass of substance Given the heat of fusion (or vaporization) of a substance, calculate the
amount of heat required to melt (or vaporize) a given quantity of that substance.
11.1
11.1
11.37, 11.38,
11.39, 11.40
2. Calculating vapor pressures and
heats of vaporization Given the vapor
pressure of a liquid at one temperature
and its heat of vaporization, calculate the
vapor pressure at another temperature.
Given the vapor pressures of a liquid at
two temperatures, calculate the heat of
vaporization.
11.2, 11.3
11.2, 11.3
11.43, 11.44,
11.45, 11.46
3. Relating the conditions for the liquefaction of gases to the critical temperature Given the critical temperature and
pressure of a substance, describe the conditions necessary for liquefying the gaseous substance.
11.4
11.4
11.51, 11.52
4. Identifying intermolecular forces
Given the molecular structure, state the
kinds of intermolecular forces expected
for a substance.
11.5
11.5
11.57, 11.58
5. Determining relative vapor pressure
on the basis of intermolecular attraction Given two liquids, decide on the basis of the intermolecular forces which has
the higher vapor pressure at a given temperature or which has the lower boiling
point.
11.6
11.6, 11.7
11.61, 11.62,
11.63, 11.64
6. Identifying types of solids From
what you know about the bonding in a
solid, classify it as molecular, metallic,
ionic, or covalent network.
11.7
11.8
11.67, 11.68,
11.69, 11.70
7. Determining the relative melting
points based on types of solids Given a
list of substances, arrange them in order
of increasing melting point from what
you know of their structures.
11.8
11.9
11.71, 11.72
Copyright © Houghton Mifflin Company. All rights reserved.
42
PART V
Examples
Exercises
Problems
8. Determining the number of atoms
per unit cell Given the description of a
unit cell, find the number of atoms per
cell.
11.9
11.10
11.77, 11.78
9. Calculating atomic mass from unitcell dimension and density Given the
edge length of the unit cell, the crystal
structure, and the density of a metal, calculate the mass of a metal ion.
11.10
11.11
11.79, 11.80
10. Calculating unit-cell dimension
from unit-cell type and density Given
the unit-cell structure, the density, and
the atomic weight for an element, calculate the edge length of the unit cell.
11.11
11.12
11.81, 11.82
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
43
Chapter 12 Solutions
Examples
Exercises
Problems
1. Applying Henry’s law Given the
solubility of a gas at one pressure, find its
solubility at another pressure.
12.1
12.4
12.41, 12.42
2. Calculating solution concentration
Given the mass percent of solute, state
how to prepare a given mass of solution.
Given the masses of solute and solvent,
find the molality and mole fractions.
12.2, 12.3,
12.4
12.5, 12.6,
12.7
12.43, 12.44,
12.45, 12.46,
12.47, 12.48,
12.49, 12.50,
12.51, 12.52
3. Converting concentration units
Given the molality of a solution, calculate
the mole fractions of solute and solvent;
and given the mole fractions, calculate
the molality. Given the density, calculate
the molarity from the molality, and vice
versa.
12.5, 12.6,
12.7, 12.8
12.8, 12.9,
12.10, 12.11
12.53, 12.54,
12.55, 12.56,
12.57, 12.58,
12.59, 12.60
4. Calculating vapor-pressure lowering Given the mole fraction of solute in
a solution of nonvolatile, undissociated
solute and the vapor pressure of pure solvent, calculate the vapor-pressure lowering and vapor pressure of the solution.
12.9
12.12
12.61, 12.62
5. Calculating boiling-point elevation
and freezing-point depression Given
the molality of a solution of nonvolatile,
undissociated solute, calculate the boiling-point elevation and freezing-point depression.
12.10
12.13
12.63, 12.64
6. Calculating molecular weights
Given the masses of solvent and solute
and the molality of the solution, find the
molecular weight of the solute. Given the
masses of solvent and solute, the freezingpoint depression, and Kf, find the molecular weight of the solute.
12.11, 12.12
12.14, 12.15
12.67, 12.68,
12.69, 12.70
7. Calculating osmotic pressure Given
the molarity and the temperature of a solution, calculate its osmotic pressure.
12.13
12.16
12.71, 12.72
Copyright © Houghton Mifflin Company. All rights reserved.
44
PART V
8. Determining colligative properties
of ionic solutions Given the concentration of ionic compound in a solution, calculate the magnitude of a colligative
property; if i is not given, assume the
value based on the formula of the ionic
compound.
Examples
Exercises
Problems
12.14
12.17
12.73, 12.74
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Chapter 13 Materials of Technology
Examples
Exercises
Problems
Note: The problem-solving skills used in this chapter are discussed in previous chapters.
Copyright © Houghton Mifflin Company. All rights reserved.
45
46
PART V
Chapter 14 Rates of Reaction
Examples
Exercises
Problems
1. Relating the different ways of expressing reaction rates Given the balanced equation for a reaction, relate the
different possible ways of defining the
rate of the reaction.
14.1
14.1
14.33, 14.34
2. Calculating the average reaction
rate Given the concentration of reactant
or product at two different times, calculate the average rate of reaction over that
time interval.
14.2
14.2
14.37, 14.38
3. Determining the order of reaction
from the rate law Given an empirical
rate law, obtain the orders with respect to
each reactant (and catalyst, if any) and
the overall order.
14.3
14.3
14.41, 14.42,
14.43, 14.44
4. Determining the rate law from initial
rates Given initial concentrations and
initial-rate data (in which the concentrations of all species are changed one at a
time, holding the others constant), find
the rate law for the reaction.
14.4
14.4
14.45, 14.46,
14.47, 14.48,
14.49, 14.50
5. Using an integrated rate law Given
the rate constant and initial reactant concentration for a first-order, second-order,
or zero-order reactions, calculate the reactant concentration after a definite time, or
calculate the time it takes for the concentration to decrease to a prescribed value.
14.5
14.5
14.51, 14.52,
14.53, 14.54,
14.55, 14.56
6. Relating the half-life of a reaction to
the rate constant Given the rate constant for a reaction, calculate the half-life.
14.6
14.6
14.57, 14.58
7. Using the Arrhenius equation
Given the values of the rate constant for
two temperatures, find the activation energy and calculate the rate constant at a
third temperature.
14.7
14.7
14.71, 14.62,
14.73, 14.74
8. Writing the overall chemical equation from a mechanism Given a mechanism for a reaction, obtain the overall
chemical equation.
14.8
14.8
14.77, 14.78
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
47
Examples
Exercises
Problems
9. Determining the molecularity of an
elementary reaction Given an elementary reaction, state the molecularity.
14.9
14.9
14.79, 14.80
10. Writing the rate equation for an elementary reaction Given an elementary
reaction, write the rate equation.
14.10
14.10
14.81, 14.82
11. Determining the rate law from a
mechanism Given a mechanism with an
initial slow step, obtain the rate law.
Given a mechanism with an initial fast,
equilibrium step, obtain the rate law.
14.11, 14.12
14.11, 14.12
14.83, 14.84,
14.85, 14.86
Copyright © Houghton Mifflin Company. All rights reserved.
48
PART V
Chapter 15 Chemical Equilibrium
Examples
Exercises
Problems
1. Applying stoichiometry to an equilibrium mixture Given the starting
amounts of reactants and the amount of
one substance at equilibrium, find the
equilibrium composition.
15.1
15.1
15.23, 15.24,
15.25, 15.26,
15.27, 15.28
2. Writing equilibrium-constant expressions Given the chemical equation,
write the equilibrium-constant expression.
15.2, 15.4
15.2, 15.6
15.29, 15.30,
15.49, 15.50
3. Obtaining an equilibrium constant
from reaction composition Given the
equilibrium composition, find Kc.
15.3
15.4
15.39, 15.40,
15.41, 15.42
4. Using the reaction quotient Given
the concentrations of substances in a reaction mixture, predict the direction of reaction.
15.5
15.8
15.55, 15.56
5. Obtaining one equilibrium concentration given the others Given Kc and
all concentrations of substances but one
in an equilibrium mixture, calculate the
concentration of this one substance.
15.6
15.9
15.59, 15.60
6. Solving equilibrium problems
Given the starting composition and Kc of
a reaction mixture, calculate the equilibrium composition.
15.7, 15.8
15.10, 15.11
15.61, 15.62,
15.63, 15.64
7. Applying Le Chatelier’s principle
Given a reaction, use Le Chatelier’s principle to decide the effect of adding or removing a substance, changing the
pressure, or changing the temperature.
15.9, 15.10,
15.11
15.12, 15.13,
15.14
15.67, 15.68,
15.69, 15.70,
15.71, 15.72
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
49
Chapter 16 Acids and Bases
Examples
Exercises
Problems
1. Identifying acid and base species
Given a proton-transfer reaction, label
the acids and bases, and name the conjugate acid-base pairs.
16.1
16.1
16.29, 16.30
2. Identifying Lewis acid and base species Given a reaction involving the donation of an electron pair, identify the Lewis
acid and the Lewis base.
16.2
16.2
16.33, 16.34,
16.35, 16.36
3. Deciding whether reactants or products are favored in an acid–base reaction Given an acid–base reaction and
the relative strengths of acids (or bases),
decide whether reactants or products are
favored.
16.3
16.3
16.39, 16.40,
16.41, 16.42
4. Calculating concentrations of H3O+
and OH– in solutions of a strong acid or
base Given the concentration of a strong
acid or base, calculate the hydronium-ion
and hydroxide-ion concentrations.
16.4
16.5
16.47, 16.48
5. Calculating the pH from the
hydronium-ion concentration, or vice
versa Given the hydronium-ion concentration, calculate the pH; or given the pH,
calculate the hydronium-ion concentration.
16.5, 16.6
16.7, 16.8,
16.9, 16.10
16.61, 16.62,
16.67, 16.68,
16.69, 16.70,
16.71, 16.72
Copyright © Houghton Mifflin Company. All rights reserved.
50
PART V
Examples
Exercises
Problems
Chapter 17 Acid–Base Equilibria
Examples
Exercises
Problems
1. Determining Ka (or Kb) from the solution pH Given the molarity and pH of a
solution of a weak acid, calculate Ka for
the acid. The Kb for a base can be determined in a similar way (see Exercise 16.5).
17.1
17.1
17.29, 17.30
2. Calculating concentrations of species
in a weak acid solution using Ka Given
Ka, calculate the hydrogen-ion concentration and pH of a solution of a weak acid
of known molarity. Given Ka1, Ka2, and
the molarity of a diprotic acid solution,
calculate the pH and the concentrations
of H+, HA–, and A2–.
17.2, 17.3,
17.4
17.2, 17.3,
17.4
17.31, 17.32,
17.37, 17.38,
17.41, 17.42
3. Calculating concentrations of species
in a weak base solution using Kb Given
Kb, calculate the hydrogen-ion concentration and pH of a solution of a weak base
of known molarity.
17.5
17.6
17.47, 17.48
4. Predicting whether a salt solution is
acidic, basic, or neutral Decide whether
an aqueous solution of a given salt is
acidic, basic, or neutral.
17.6
17.7
17.53, 17.54
5. Obtaining Ka from Kb or Kb from Ka
Calculate Ka for a cation or Kb for an anion from the ionization constant of the
conjugate base or acid.
17.7
17.8
17.57, 17.58
6. Calculating concentrations of species
in a salt solution Given the concentration of a solution of a salt in which one
ion hydrolyzes, and given the ionization
constant of the conjugate acid or base of
this ion, calculate the H+ concentration.
17.8
17.9
17.59, 17.60,
17.61, 17.62
7. Calculating the common-ion effect
on acid ionization Given Ka and the concentrations of weak acid and strong acid
in a solution, calculate the degree of ionization of the weak acid. Given Ka and the
concentrations of weak acid and its salt
in a solution, calculate the pH.
17.9, 17.10
17.10, 17.11
17.63, 17.64,
17.65, 17.66
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
51
8. Calculating the pH of a buffer from
given volumes of solution Given concentrations and volumes of acid and conjugate base from which a buffer is
prepared, calculate the buffer pH.
17.11
17.12
17.69, 17.70
9. Calculating the pH of a buffer when
a strong acid or strong base is added
Calculate the pH of a given volume of
buffer solution (given the concentrations
of conjugate acid and base in the buffer)
to which a specified amount of strong
acid or strong base is added.
17.12
17.13
17.71, 17.72
10. Calculating the pH of a solution of
a strong acid and a strong base Calculate the pH during the titration of a
strong acid by a strong base, given the
volumes and concentrations of the acid
and base.
17.13
17.14
17.79, 17.80
11. Calculating the pH at the equivalence point in the titration of a weak
acid by a strong base Calculate the pH
at the equivalence point for the titration
of a weak acid by a strong base. Be able
to do the same type of calculation for the
titration of a weak base by a strong acid.
17.14
17.15
17.81, 17.82
Copyright © Houghton Mifflin Company. All rights reserved.
52
PART V
Chapter 18 Solubility and
Complex-Ion Equilibria
Examples
Exercises
Problems
1. Writing solubility product expressions Write the solubility product expression for a given ionic compound.
18.1
18.1
18.21, 18.22
2. Calculating Ksp from the solubility,
or vice versa Given the solubility of a
slightly soluble ionic compound, calculate Ksp. Given Ksp, calculate the solubility
of an ionic compound.
18.2, 18.3,
18.4
18.2, 18.3,
18.4
18.23, 18.24,
18.25, 18.26,
18.29, 18.30,
18.31, 18.32
3. Calculating the solubility of a
slightly soluble salt in a solution of a
common ion Given the solubility product constant, calculate the molar solubility of a slightly soluble ionic compound
in a solution that contains a common ion.
18.5
18.5
18.33, 18.34,
18.35, 18.36
4. Predicting whether precipitation will
occur Given the concentrations of ions
originally in solution, determine whether
a precipitate is expected to form. Determine whether a precipitate is expected to
form when two solutions of known volume and molarity are mixed. For both
problems, you will need the solubility
product constant.
18.6, 18.7
18.6, 18.7
18.41, 18.42,
18.43, 18.44
5. Determining the qualitative effect of
pH on solubility Decide whether the
solubility of a salt will be greatly increased by decreasing the pH.
18.8
18.8
18.53, 18.54
6. Calculating the concentration of a
metal ion in equilibrium with a complex ion Calculate the concentration of
an aqueous metal ion in equilibrium with
the complex ion, given the original metalion and ligand concentrations. The formation constant Kf of the complex ion is
required.
18.9
18.9
18.57, 18.58
7. Predicting whether a precipitate will
form in the presence of the complex
ion Predict whether an ionic compound
will precipitate from a solution of known
concentrations of cation, anion, and ligand that complexes with the cation. Kf
and Ksp are required.
18.10
18.10
18.59, 18.60
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
8. Calculating the solubility of a
slightly soluble ionic compound in a solution of the complex ion Calculate the
molar solubility of a slightly soluble ionic
compound in a solution of known concentration of a ligand that complexes
with the cation. Ksp and Kf are required.
Copyright © Houghton Mifflin Company. All rights reserved.
53
Examples
Exercises
Problems
18.11
18.11
18.61, 18.62
54
PART V
Chapter 19 Thermodynamics and Equilibrium
Examples
Exercises
Problems
1. Calculating the entropy change for a
phase transition Given the heat of
phase transition and the temperature of
the transition, calculate the entropy
change of the system, ∆S.
19.1
19.3
19.29, 19.30
2. Predicting the sign of the entropy
change of a reaction Predict the sign of
∆S° for a reaction to which the rules
listed in the text can be clearly applied.
19.2
19.4
19.33, 19.34
3. Calculating ∆S° for a reaction Given
the standard entropies of reactants and
products, calculate the change of entropy,
∆S°, for the reaction.
19.3
19.5
19.35, 19.36
4. Calculating ∆G° from ∆H° and ∆S°
Given enthalpies of formation and standard entropies of reactants and products,
calculate the standard free-energy
change, ∆G°, for a reaction.
19.4
19.6
19.39, 19.40
5. Calculating ∆G° from standard free
energies of formation Given the free energies of formation of reactants and products, calculate the standard free-energy
change, ∆G°, for a reaction.
19.5
19.7
19.43, 19.44
6. Interpreting the sign of ∆G° Use the
standard free-energy change to determine the spontaneity of a reaction.
19.6
19.8
19.47, 19.48
7. Writing the expression for a thermodynamic equilibrium constant For any
balanced chemical equation, write the expression for the thermodynamic equilibrium constant.
19.7
19.9
19.53, 19.54
8. Calculating K from the standard freeenergy change Given the standard freeenergy change for a reaction, calculate
the thermodynamic equilibrium constant.
19.8, 19.9
19.10, 19.11
19.55, 19.56,
19.57, 19.58,
19.59, 19.60
9. Calculating ∆G° and K at various
temperatures Given ∆H° and ∆S° at
25°C, calculate ∆G° and K for a reaction
at a temperature other than 25°C.
19.10
19.12
19.61, 19.62
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
55
Chapter 20 Electrochemistry
Examples
Exercises
Problems
1. Balancing equations in acid and basic solutions by the half-reaction
method Given the skeleton equation for
an oxidation–reduction equation, complete and balance it.
20.1, 20.2
20.1, 20.2
20.29, 20.30,
20.31, 20.32
2. Sketching and labeling a voltaic cell
Given a verbal description of a voltaic
cell, sketch the cell, labeling the anode
and cathode, and give the directions of
electron flow and ion migration.
20.3
20.3
20.37, 20.38
3. Writing the cell reaction from the cell
notation Given the notation for a voltaic
cell, write the overall cell reaction. Alternatively, given the cell reaction, write the
cell notation.
20.4
20.5
20.47, 20.48
4. Calculating the quantity of work
from a given amount of cell reactant
Given the emf and overall reaction for a
voltaic cell, calculate the maximum work
that can be obtained from a given
amount of reactant.
20.5
20.6
20.51, 20.52,
20.53, 20.54
5. Determining the relative strengths of
oxidizing and reducing agents Given a
table of standard electrode potentials, list
oxidizing or reducing agents by increasing strength.
20.6
20.7
20.55, 20.56,
20.57, 20.58
6. Determining the direction of spontaneity from electrode potentials Given
standard electrode potentials, decide the
direction of spontaneity for an oxidation–
reduction reaction under standard conditions.
20.7
20.8
20.59, 20.60
7. Calculating the emf from standard
potentials Given standard electrode potentials, calculate the standard emf of a
voltaic cell.
20.8
20.9
20.63, 20.64
8. Calculating the free-energy change
from electrode potentials Given standard electrode potentials, calculate the
standard free-energy change for an oxidation–reduction reaction.
20.9
20.10
20.67, 20.68
Copyright © Houghton Mifflin Company. All rights reserved.
56
PART V
Examples
Exercises
Problems
9. Calculating the cell emf from freeenergy change Given a table of standard free energies of formation, calculate
the standard emf of a voltaic cell.
20.10
20.11
20.71, 20.72
10. Calculating the equilibrium constant from cell emf Given standard potentials (or standard emf), calculate the
equilibrium constant of an oxidation–reduction reaction.
20.11
20.12
20.75, 20.76
11. Calculating the cell emf for nonstandard conditions Given standard electrode potentials and the concentrations of
substances in a voltaic cell, calculate the
cell emf.
20.12
20.13
20.79, 20.80
12. Predicting the half-reactions in an
aqueous electrolysis Using values of
electrode potentials, decide which electrode reactions actually occur in the electrolysis of an aqueous solution.
20.13
20.16
20.87, 20.88
13. Relating the amounts of charge and
product in an electrolysis Given the
amount of product obtained by electrolysis, calculate the amount of charge that
flowed. Given the amount of charge that
flowed, calculate the amount of product
obtained by electrolysis.
20.14, 20.15
20.17, 20.18
20.89, 20.90,
20.91, 20.92
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
57
Chapter 21 Nuclear Chemistry
Examples
Exercises
Problems
1. Writing a nuclear equation Given a
word description of a radioactive decay
process, write the nuclear equation.
21.1
21.1
21.29, 21.30,
21.31, 21.32
2. Deducing a product or reactant in a
nuclear equation Given all but one of
the reactants and products in a nuclear reaction, find that one nuclide.
21.2, 21.6
21.2, 21.6
21.33, 21.34,
21.35, 21.36,
21.49, 21.50,
21.51, 21.52
3. Predicting the relative stabilities of
nuclides Given a number of nuclides,
determine which are most likely to be radioactive and which are most likely to be
stable.
21.3
21.3
21.37, 21.38
4. Predicting the type of radioactive decay Predict the type of radioactive decay
that is most likely for given nuclides.
21.4
21.4
21.39, 21.40
5. Using the notation for a bombardment reaction Given an equation for a
nuclear bombardment reaction, write the
abbreviated notation, or vice versa.
21.5
21.5
21.43, 21.44,
21.45, 21.46
6. Calculating the decay constant from
the activity Given the activity (disintegrations per second) of a radioactive isotope, obtain the decay constant.
21.7
21.7
21.53, 21.54,
21.55, 21.56
7. Relating the decay constant, half-life,
and activity Given the decay constant of
a radioactive isotope, obtain the half-life,
or vice versa. Given the decay constant
and mass of a radioactive isotope, calculate the activity of the sample.
21.8, 21.9
21.8, 21.9
21.57, 21.58,
21.59, 21.60,
21.61, 21.62
8. Determining the fraction of nuclei remaining after a specified time Given
the half-life of a radioactive isotope, calculate the fraction remaining after a specified time.
21.10
21.10
21.65, 21.66
9. Applying the carbon-14 dating
method Given the disintegrations of
carbon-14 nuclei per gram of carbon in a
dead organic object, calculate the age of
the object—that is, the time since its
death.
21.11
21.11
21.71, 21.72
Copyright © Houghton Mifflin Company. All rights reserved.
58
PART V
10. Calculating the energy change for a
nuclear reaction Given nuclear masses,
calculate the energy change for a nuclear
reaction. Obtain the answer in joules per
mole or MeV per particle.
Examples
Exercises
Problems
21.12
21.12
21.77, 21.78
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
Chapter 22 Chemistry of the Main-Group Elements
Note: The problem-solving skills used in this chapter are discussed in previous chapters.
Copyright © Houghton Mifflin Company. All rights reserved.
59
60
PART V
Chapter 23 The Transition Elements
Examples
Exercises
Problems
1. Writing the IUPAC name given the
structural formula of a coordination
compound, and vice versa Given the
structural formulas of coordination compounds, write the IUPAC names; given
the IUPAC names of complexes, write the
structural formulas.
23.1, 23.2
23.1, 23.2
23.43, 23.44,
23.45, 23.46,
23.47, 23.48
2. Deciding whether isomers are possible Given the formula of a complex, decide whether geometric isomers are
possible and, if so, draw them. Given the
structural formula of a complex, decide
whether enantiomers (optical isomers)
are possible and, if so, draw them.
23.3, 23.4
23.3, 23.4
23.49, 23.50,
23.51, 23.52
3. Describing the bonding in a complex
ion Given a transition-metal complex
ion, describe the bonding types (highspin and low-spin, if both exist), using valence bond theory for octahedral and
four-coordinate complexes. Give the
number of unpaired electrons in the complex. Do the same using crystal field
theory.
23.5, 23.6
23.5, 23.6
23.53, 23.54,
23.55, 23.56
4. Predicting the relative wavelengths
of absorption of complex ions Given
two complexes that differ only in the ligands, predict, on the basis of the spectrochemical series, which complex absorbs
at higher wavelength. Given the absorption maxima, predict the colors of the
complexes.
23.7
23.7
23.57, 23.58,
23.59, 23.60
Copyright © Houghton Mifflin Company. All rights reserved.
Operational Skills Masterlist
61
Chapter 24 Organic Chemistry
Examples
Exercises
Problems
1. Writing a condensed structural
formula Given the structural formula of
a hydrocarbon, write the condensed structure formula.
24.1
24.1
24.23, 24.24
2. Predicting cis–trans isomers Given a
condensed structural formula of an
alkene, decide whether cis and trans isomers are possible, and, if so, draw the
structural formulas.
24.2
24.2
24.25, 24.26
3. Predicting the major product in an
addition reaction Predict the major
product in the addition of an unsymmetrical reagent to an unsymmetrical alkene.
24.3
24.3
24.31, 24.32
4. Writing the IUPAC name of a hydrocarbon given the structural formula, and
vice versa Given the structure of a hydrocarbon, state the IUPAC name. Given
the IUPAC name of a hydrocarbon, write
the structural formula.
24.4, 24.5,
24.6
24.4, 24.5,
24.6, 24.7,
24.8, 24.9
24.33, 24.34,
24.35, 24.36,
24.37, 24.38,
24.39, 24.40,
24.41, 24.42,
24.43, 24.44
Examples
Exercises
Problems
Chapter 25 Polymer Materials: Synthetic
and Biological
Note: The problem-solving skills used in this chapter are discussed in previous chapters.
Copyright © Houghton Mifflin Company. All rights reserved.
PART VI
Correlation of Cumulative-Skills Problems
with Text Sections
Listed below are the cumulative-skills problems found at the end of the chapters and the
sections of the text needed to solve each problem. You can use this list to be sure that a problem
you wish to assign does not require any sections you may have omitted from students’
required reading.
Chapter 1
1.137 and 1.138: 1.3, 1.5, 1.7
1.139 and 1.140: 1.5, 1.7
1.141 and 1.142: 1.5, 1.7, 1.8
1.143 and 1.144: 1.3, 1.5, 1.7
1.145 and 1.146: 1.5, 1.7, 1.8
1.147 and 1.148: 1.5, 1.7, 1.8
Chapter 2
2.125 and 2.126: 1.7, 1.8
2.127 and 2.128: 1.2, 2.6
2.129 and 2.130: 1.7, 2.4
Chapter 3
3.109 and 3.110: 1.3, 1.8, 3.2
3.111 and 3.112: 1.8, 3.1, 3.2
3.113 and 3.114: 1.3, 3.2, 3.5
3.115 and 3.116: 1.8, 3.2, 3.3
Chapter 4
4.119 and 4.120: 2.6, 2.8, 4.2
4.121 and 4.122: 1.3, 1.8, 4.2
4.123 and 4.124: 1.3, 4.2, 4.3, 4.4
4.125 and 4.126: 2.6, 4.5
4.127 and 4.128: 1.7, 3.1, 3.2, 3.3
4.129 and 4.130: 3.7, 4.7, 4.10
62
Copyright © Houghton Mifflin Company. All rights reserved.
Correlation of Cumulative-Skills Problems with Text Sections
63
4.131 and 4.132: 3.2, 3.7
4.133 and 4.134: 1.7, 2.10, 4.3, 4.9
4.135 and 4.136: 3.1, 3.3, 3.7
4.137 and 4.138: 1.7, 3.1, 4.7, 4.10
4.139 and 4.140: 3.3, 3.7, 4.4, 4.10
Chapter 5
5.125 and 5.126: 3.2, 3.3, 5.3
5.127 and 5.128: 3.2, 5.1, 5.3, 5.4, 5.5
5.129 and 5.130: 3.7, 3.8, 5.3, 5.4
5.131 and 5.132: 1.7, 3.2, 5.3
5.133 and 5.134: 1.7, 5.3, 5.7
Chapter 6
6.115 and 6.116: 6.1, 6.6
6.117 and 6.118: 2.10, 3.2, 3.3, 6.5
6.119 and 6.120: 3.2, 6.5
6.121 and 6.122: 3.2, 3.8, 6.5
6.123 and 6.124: 5.3, 6.6
6.125 and 6.126: 2.10, 3.2, 3.7, 6.8
Chapter 7
7.85 and 7.86: 1.8, 3.2, 7.2
7.87 and 7.88: 1.8, 6.6, 7.2
7.89 and 7.90: 1.8, 6.1, 7.2
7.91 and 7.92: 1.7, 6.1, 7.2, 7.4
Chapter 8
8.79 and 8.80: 2.9, 2.10, 5.3, 8.7
8.81 and 8.82: 2.4, 3.3, 8.7
8.83 and 8.84: 3.2, 8.6
8.85 and 8.86: 3.2, 7.3, 8.6
8.87 and 8.88: 6.7, 8.6
Chapter 9
9.105 and 9.106: 2.10, 4.4, 9.5
9.107 and 9.108: 2.8, 3.5, 9.4, 9.6
9.109 and 9.110: 3.5, 9.6
9.111 and 9.112: 5.3, 9.1, 9.4, 9.6, 9.8
9.113 and 9.114: 6.8, 9.11
9.115 and 9.116: 9.5, 9.11
9.117 and 9.118: 8.6, 9.5, 9.11
Copyright © Houghton Mifflin Company. All rights reserved.
64
PART VI
Chapter 10
10.77 and 10.78: 2.6, 3.3, 3.5, 9.6, 9.8, 10.1, 10.3
10.79 and 10.80: 2.10, 5.3, 10.3
10.81 and 10.82: 9.10, 10.1, 10.3, 10.4
10.83 and 10.84: 9.6, 9.7, 9.8, 9.11, 10.1, 10.3
Chapter 11
11.117 and 11.118: 3.2, 5.3, 5.5, 11.2
11.119 and 11.120: 1.7, 3.2, 6.2, 16.2
11.121 and 11.122: 3.2, 6.6, 11.2
11.123 and 11.124: 1.7, 3.2, 5.3, 11.2
Chapter 12
12.105 and 12.106: 3.2, 4.1, 4.7, 12.4
12.107 and 12.108: 6.7, 8.6, 12.2
12.109 and 12.110: 3.2, 12.4
12.111 and 12.112: 1.7, 3.2, 4.7
12.113 and 12.114: 12.4, 12.6
12.115 and 12.116: 3.2, 3.5, 12.4, 12.6
Chapter 14
14.123 and 14.124: 2.10, 5.3, 14.3
14.125 and 14.126: 2.10, 6.2, 6.8, 14.4
14.127 and 14.128: 5.3, 14.4
Chapter 15
15.107 and 15.108: 3.2, 4.7, 15.2, 15.6
15.109 and 15.110: 3.2, 5.3, 15.6
Chapter 16
16.103 and 16.104: 3.2, 3.7, 9.6, 16.4
16.105 and 16.106: 3.2, 5.3, 16.3
Chapter 17
17.125 and 17.126: 1.7, 3.3, 16.8, 17.1
17.127 and 17.128: 1.7, 12.4, 12.6, 17.1
17.129 and 17.130: 1.7, 4.4, 4.7, 16.8, 17.6
Copyright © Houghton Mifflin Company. All rights reserved.
Correlation of Cumulative-Skills Problems with Text Sections
65
Chapter 18
18.101 and 18.102: 17.6, 18.1, 18.3, 18.4
18.103 and 18.104: 15.2, 15.6, 17.1, 17.6, 18.1, 18.3
18.105 and 18.106: 3.2, 4.2, 4.3, 4.7, 18.1, 18.3
Chapter 19
19.99 and 19.100: 6.2, 15.2, 19.3, 19.4, 19.6
19.101 and 19.102: 6.2, 15.2, 19.3, 19.4, 19.6
19.103 and 19.104: 17.1, 19.4, 19.6, 19.7
Chapter 20
20.123 and 20.124: 6.8, 19.2, 19.4, 20.5
20.125 and 20.126: 16.8, 20.4, 20.6
20.127 and 20.128: 15.8, 17.6, 20.4, 20.6
20.129 and 20.130: 18.1, 20.4, 20.5, 20.6
Chapter 21
21.99 and 21.100: 2.6, 3.2, 14.4, 21.4
21.101 and 21.102: 5.3, 21.1, 21.6
21.103 and 21.104: 5.3, 6.2, 6.8, 21.1, 21.2, 21.6
Copyright © Houghton Mifflin Company. All rights reserved.
PART VII
Alternate Examples for Lecture
CHAPTER 1 Chemistry and Measurement
Alternate Example 1.1 Using the Law of Conservation of Mass
Aluminum powder burns in oxygen to produce a substance called aluminum oxide. A sample
of 2.00 grams of aluminum is burned in oxygen and produces 3.78 grams of aluminum oxide.
How many grams of oxygen were used in this reaction?
Answer: 1.78 grams
Alternate Example 1.2 Using Significant Figures in Calculations
Perform the following calculations, rounding the answers to the correct number of significant
figures.
(a)
5.8914
(b) 0.453 – 1.59 (c) 0.456 – 0.421 (d) 92.34 × (0.456 – 0.421)
1.289 × 7.28
Answers: (a) 0.628 (b) –1.14 (c) 0.035 (d) 3.2
Alternate Example 1.3 Converting from One Temperature Scale to Another
In winter, the average low temperature of interior Alaska is –30°F (two significant figures).
What is this temperature in degrees Celsius? in kelvins?
Answer: –34°C; 239 K
Alternate Example 1.4 Calculating the Density of a Substance
Oil of wintergreen is a colorless liquid used as a flavoring. A 28.1-g sample of oil of wintergreen
has a volume of 23.7 mL. What is the density of oil of wintergreen?
Answer: 1.19 g/mL
66
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
67
Alternate Example 1.5 Using the Density to Relate Mass and Volume
A sample of gasoline has a density of 0.718 g/mL. What is the volume of 454 g of gasoline?
Answer: 632 mL
Alternate Example 1.6 Converting Units: Metric Unit to Metric Unit
A sample of sodium metal is burned in chlorine gas, producing 573 mg of sodium chloride.
How many grams is this? How many kilograms?
Answer: 0.573 g; 5.73 × 10–4 kg
Alternate Example 1.7 Converting Units: Metric Volume to Metric Volume
An experiment calls for 54.3 mL of ethanol. What is this volume in cubic meters?
Answer: 5.43 × 10–5 m3
First Alternate Example 1.8 Converting Units: Any Unit to Another Unit
The Star of Asia sapphire in the Smithsonian Institution weighs 330 carats (three significant
figures). What is this weight in grams? One carat equals 200 mg (exact).
Answer: 66.0 g
Second Alternate Example 1.8 Converting Units: Any Unit to Another Unit
The dimensions of Noah’s ark were reported as 3.0 × 102 cubits by 5.0 × 101 cubits. Express the
size in units of feet and meters (1 cubit = 1.5 ft).
Answer: 4.5 × 102 ft by 75 ft; 1.4 × 102 m by 23 m
CHAPTER 2 Atoms, Molecules, and Ions
Alternate Example 2.1 Writing Nuclide Symbols
Write the nuclide symbol for the nucleus that has 19 protons and 20 neutrons.
Answer:
39
K
19
Copyright © Houghton Mifflin Company. All rights reserved.
68
PART VII
Alternate Example 2.2 Determining Atomic Weight from Isotopic Masses and
Fractional Abundances
An element has four naturally occurring isotopes. The mass and percentage abundance of
each isotope are as follows:
Percentage
Abundance
Mass (amu)
1.48
23.6
22.6
52.3
203.973
205.9745
206.9759
207.9766
What is the atomic weight and the name of the element?
Answer: 207 amu; lead
Alternate Example 2.3 Writing an Ionic Formula, Given the Ions
(a) What is the formula of magnesium nitride, which is composed of the ions Mg2+ and N3–?
(b) What is the formula of calcium phosphate, which is composed of the ions Ca2+ and PO43–?
Answers: (a) Mg3N2 (b) Ca3(PO4)2
Alternate Example 2.4 Naming an Ionic Compound from Its Formula
Name the following: (a) BaO, (b) Cr2(SO4)3.
Answers: (a) barium oxide (b) chromium(III) sulfate
Alternate Example 2.5 Writing the Formula from the Name of an Ionic Compound
Write the formulas for the following: (a) potassium carbonate, (b) manganese(II) sulfate,
(c) selenium tetrafluoride.
Answers: (a) K2CO3 (b) MnSO4 (c) SeF4
Alternate Example 2.6 Naming a Binary Compound from Its Formula
Name the following compounds: (a) OF2, (b) S4N4, (c) BCl3.
Answers: (a) oxygen difluoride (b) tetrasulfur tetranitride (c) boron trichloride
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
69
Alternate Example 2.7 Writing the Formula from the Name of a Binary Compound
Give the formula for each of the following: (a) carbon disulfide, (b) nitrogen tribromide,
(c) dinitrogen tetrafluoride.
Answers: (a) CS2 (b) NBr3 (c) N2F4
Alternate Example 2.9 Writing the Name and Formula of an Anion from the Acid
Bromine has an oxoacid HBrO2, whose name is bromous acid (compare chlorous acid, HClO2).
What is the name and formula of the corresponding anion?
Answer: bromite ion, BrO2–
Alternate Example 2.10 Naming a Hydrate from Its Formula
A compound whose common name is green vitriol has the chemical formula FeSO4⋅7H2O.
What is the chemical name of this compound?
Answer: iron(II) sulfate heptahydrate
Alternate Example 2.11 Writing the Formula from the Name of a Hydrate
Calcium chloride hexahydrate is used to melt snow from roads. What is the formula of this
compound?
Answer: CaCl2⋅6H2O
Alternate Example 2.12 Balancing Simple Equations
Balance the following equations.
(a) CS2 + O2 → CO2 + SO2
(b) NH3 + O2 → NO + H2O
(c) C2H5OH + O2 → CO2 + H2O
Answers:
(a) CS2 + 3O2 → CO2 + 2SO2
(b) 4NH3 + 5O2 → 4NO + 6H2O
(c) C2H5OH + 3O2 → 2CO2 + 3H2O
Copyright © Houghton Mifflin Company. All rights reserved.
70
PART VII
CHAPTER 3 Calculations with Chemical Formulas and Equations
Alternate Example 3.1 Calculating the Formula Weight from a Formula
Calculate the formula weight of the following compounds from their formulas (obtain the
answers to three significant figures): (a) calcium hydroxide, Ca(OH)2; (b) methylamine,
CH3NH2.
Answers: (a) 74.1 amu (b) 31.1 amu
Alternate Example 3.3 Calculating the Mass of an Atom or Molecule
What is the mass of the nitric acid molecule, HNO3?
Answer: 1.05 × 10–22 g
Alternate Example 3.4 Converting Moles of Substance to Grams
A sample of nitric acid contains 0.253 mol HNO3. How many grams is this?
Answer: 15.9 g
First Alternate Example 3.5 Converting Grams of Substance to Moles
Calcite is a mineral composed of calcium carbonate, CaCO3. A sample of calcite composed of
pure calcium carbonate weighs 23.6 g. How many moles of calcium carbonate is this?
Answer: 0.236 mol CaCO3
Second Alternate Example 3.5 Converting Grams of Substance to Moles
The average daily requirement of the essential amino acid leucine, C6H14O2N, is 2.2 g for an
adult. How many moles of leucine are required daily?
Answer: 0.017 mol
Alternate Example 3.6 Calculating the Number of Atoms in a Given Mass
The daily requirement of chromium in the human diet is 1.0 × 10–6 g. How many atoms of
chromium does this represent?
Answer: 1.2 × 1016 atoms
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
71
Alternate Example 3.7 Calculating the Percentage Composition from the Formula
Lead(II) chromate, PbCrO4, is used as a paint pigment (chrome yellow). What is the percentage
composition of lead(II) chromate?
Answer: 64.1% Pb, 16.1% Cr, and 19.8% O
Alternate Example 3.8 Calculating the Mass of an Element in a Given Mass of
Compound
The chemical name of table sugar is sucrose, C12H22O11. How many grams of carbon (C) are
in 61.8 g sucrose?
Answer: 26.0 g
Alternate Example 3.9 Calculating the Percentages of C and H by Combustion
Benzene is a liquid compound composed of carbon and hydrogen; it is used in the preparation
of polystyrene plastic. A sample of benzene weighing 342 mg is burned in oxygen and forms
1156 mg of carbon dioxide. What is the percentage composition of benzene?
Answer: 92.3% C and 7.7% H
Alternate Example 3.10 Determining the Empirical Formula from Percentage
Composition (Binary Compound)
In Alternate Example 3.8, we determined the percentage composition of benzene: 92.3% C and
7.7% H. What is the empirical formula of benzene?
Answer: CH
Alternate Example 3.11 Determining the Empirical Formula from Percentage
Composition (General)
Sodium pyrophosphate is used in detergent preparations. The mass percentages of the
elements in this compound are 34.6% Na, 23.3% P, and 42.1% O. What is the empirical formula
of sodium pyrophosphate?
Answer: Na4P2O7
First Alternate Example 3.12 Determining the Molecular Formula from Percentage
Composition and Molecular Weight
The percentage composition of benzene is 92.3% C and 7.7% H. In Alternate Example 3.9, we
found the empirical formula of benzene from these data to be CH. In a separate experiment,
the molecular weight of benzene was determined to be 78.1 amu. What is the molecular
formula of benzene?
Answer: C6H6
Copyright © Houghton Mifflin Company. All rights reserved.
72
PART VII
Second Alternate Example 3.12 Determining the Molecular Formula from
Percentage Composition and Molecular Weight
Hexamethylene is one of the materials used to produce a type of nylon. Elemental analysis of
the substance gives 62.1% C, 13.8% H, and 24.1% N. Its molecular weight is 116 amu. What is
its molecular formula?
Answer: C6H16N2
Alternate Example 3.13 Relating the Quantity of Reactant to Quantity of Product
Propane, C3H8, is normally a gas, but it is sold as a fuel compressed as a liquid in steel
cylinders. The gas burns according to the equation
C3H8(g) + 5O2(g) → 3CO2(g) + 4H2O(g)
How many grams of CO2 are produced when 20.0 g of propane is burned?
Answer: 59.9 g CO2
Alternate Example 3.14 Relating the Quantities of Two Reactants (or Two Products)
How many grams of O2 are required to burn 20.0 g C3H8?
Answer: 72.6 g O2
Alternate Example 3.15 Calculating with a Limiting Reactant (Involving Moles)
Magnesium metal is used to prepare zirconium metal, which is used to make the container
for nuclear fuel (the nuclear fuel rods).
ZrCl4(g) + 2Mg(l) → 2MgCl2(s) + Zr(s)
How many moles of zirconium metal can be produced from a reaction mixture containing
0.20 mol ZrCl4 and 0.50 mol Mg?
Answer: 0.20 mol
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
73
Alternate Example 3.16 Calculating with a Limiting Reactant (Involving Masses)
Urea, CH4N2O, is used as a nitrogen fertilizer. It is manufactured from ammonia and carbon
dioxide at high pressure and high temperature.
2NH3 + CO2 → CH4N2O + H2O
In a laboratory experiment, 10.0 g NH3 and 10.0 g CO2 were added to a reaction vessel. What
is the maximum quantity (in grams) of urea that can be obtained? How many grams of the
excess reactant are left at the end of the reaction?
Answer: 13.6 g CH4N2O; 2.3 g NH3
CHAPTER 4 Chemical Reactions: An Introduction
Alternate Example 4.2 Writing Net Ionic Equations
Write a net ionic equation for the following molecular equations.
(a) H2SO4(aq) + Mg(OH)2(s) → MgSO4(aq) + 2H2O(l)
(b) KCl(aq) + AgNO3(aq) → KNO3(aq) + AgCl(s)
Answers: (a) 2H+(aq) + Mg(OH)2(s) → Mg2+(aq) + 2H2O(l) (b) Cl–(aq) + Ag+(aq) →
AgCl(s)
First Alternate Example 4.3 Deciding Whether Precipitation Occurs
For each of the following, decide whether precipitation will occur. If it does, write the
molecular equation and the net ionic equation.
(a) KBr + MgSO4 →
(b) NaOH + MgCl2 →
Answers: (a) no reaction (b) 2NaOH(aq) + MgCl2(aq) → 2NaCl(aq) + Mg(OH)2(s);
2OH–(aq) + Mg2+(aq) → Mg(OH)2(s)
Second Alternate Example 4.3 Deciding Whether Precipitation Occurs
Decide whether a precipitation reaction will occur for the following. If precipitation does
occur, write the molecular equation and the net ionic equation for the reaction.
K3PO4 + CaCl2 →
Answer: 2K3PO4(aq) + 3CaCl2(aq) → 6KCl(aq) + Ca3(PO4)2(s); 2PO43–(aq) + 3Ca2+(aq) →
Ca3(PO4)2(s)
Copyright © Houghton Mifflin Company. All rights reserved.
74
PART VII
Alternate Example 4.4 Classifying Acids and Bases as Strong or Weak
Classify each of the following as a strong or weak acid or base: (a) KOH, (b) H2S, (c) CH3NH2,
(d) HClO4.
Answers: (a) strong base (b) weak acid (c) weak base (d) strong acid
Alternate Example 4.5 Writing an Equation for a Neutralization
Write the molecular equation and the net ionic equation for the neutralization of sulfurous
acid, H2SO3, by potassium hydroxide, KOH.
Answer: H2SO3(aq) + 2KOH(aq) → K2SO3(aq) + 2H2O(l); H2SO3(aq) + 2OH–(aq) →
SO32–(aq) + 2H2O(l)
Alternate Example 4.6 Writing an Equation for a Reaction with Gas Formation
Write the molecular equation and the net ionic equation for the reaction of copper(II) carbonate
with hydrochloric acid.
Answer: CuCO3(s) + 2HCl(aq) → CuCl2(aq) + H2O(l) + CO2(g); CuCO3(s) + 2H+(aq) →
Cu2+(aq) + H2O(l) + CO2(g)
First Alternate Example 4.7 Assigning Oxidation Numbers
Potassium permanganate, KMnO4, is a purple-colored compound; potassium manganate,
K2MnO4, is a green-colored compound. Obtain the oxidation numbers of the manganese atom
in these compounds.
Answer: +7, +6
Second Alternate Example 4.7 Assigning Oxidation Numbers
What is the oxidation number of Cr in the dichromate ion, Cr2O72–?
Answer: +6
Alternate Example 4.9 Calculating Molarity from Mass and Volume
You place a 1.53-g sample of potassium dichromate, K2Cr2O7, into a 50.0-mL volumetric flask
and add water to bring the solution up to the mark on the neck of the flask. What is the molarity
of K2Cr2O7 in the solution?
Answer: 0.104 M
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
75
Alternate Example 4.10 Using Molarity as a Conversion Factor
A solution of sodium chloride used for intravenous transfusion (physiological saline solution)
has a concentration of 0.154 M NaCl. How many moles of NaCl are contained in a 500-mL
bottle of physiological saline solution? How many grams of NaCl are in the 500 mL of solution?
Answer: 0.0770 mol; 4.50 g
Alternate Example 4.11 Diluting a Solution
A saturated stock solution of NaCl is 6.00 M. How much of this stock solution is needed to
prepare 1.00 L of physiological saline solution, which is 0.154 M NaCl?
Answer: 25.7 mL
Alternate Example 4.12 Determining the Amount of a Species by
Gravimetric Analysis
A soluble silver compound was analyzed for the percentage of silver by adding sodium
chloride solution to precipitate the silver ion as silver chloride. If 1.583 g of silver compound
gave 1.788 g of silver chloride, what is the mass percentage of silver in the compound?
Answer: 85.01%
Alternate Example 4.13 Calculating the Volume of Reactant Solution Needed
Zinc sulfide reacts with hydrochloric acid to produce hydrogen sulfide gas:
ZnS(s) + 2HCl(aq) → ZnCl2(aq) + H2S(g)
How many milliliters of 0.0512 M HCl are required to react with 0.392 g ZnS?
Answer: 157 mL
Alternate Example 4.14 Calculating the Quantity of Substance in a Titrated Solution
A dilute solution of hydrogen peroxide is sold in drugstores as a mild antiseptic. A typical
solution was analyzed for the percentage of hydrogen peroxide by titrating it with potassium
permanganate:
5H2O2(aq) + 2KMnO4(aq) + 6H+(aq) → 8H2O(l) + 5O2(g) + 2K+(aq) + 2Mn2+(aq)
What is the mass percentage of H2O2 in a solution if 57.5 g of solution required 38.9 mL of
0.534 M KMnO4?
Answer: 3.07%
Copyright © Houghton Mifflin Company. All rights reserved.
76
PART VII
CHAPTER 5 The Gaseous State
Alternate Example 5.2 Using Boyle’s Law
A volume of oxygen gas occupies 38.7 mL at 751 mmHg and 21°C. What is the volume if the
pressure changes to 359 mmHg while the temperature remains constant?
Answer: 81.0 mL
Alternate Example 5.3 Using Charles’s Law
You prepared carbon dioxide by adding HCl(aq) to marble chips (CaCO3). According to your
calculations, you should obtain 79.4 mL of CO2 at 0°C and 760 mmHg. How many milliliters
of gas would you obtain at 27°C?
Answer: 87.2 mL
Alternate Example 5.4 Using the Combined Gas Law
Divers working from a North Sea drilling platform experience pressures of 5.0 × 101 atm at a
depth of 5.0 × 102 m. If a balloon is inflated to a volume of 5.0 L (the volume of a lung) at that
depth at a water temperature of 4.0°C, what would the volume of the balloon be on the surface
(1.0 atm pressure) at a temperature of 11°C?
Answer: 2.6 × 102 L
Alternate Example 5.5 Deriving Empirical Gas Laws from the Ideal Gas Law
You put varying amounts of gas into a given container at a given temperature. Use the ideal
gas law to show that the amount (moles) of gas is proportional to pressure at constant
temperature and volume.
Answer: n ∝ P
Alternate Example 5.6 Using the Ideal Gas Law
A 50.0-L cylinder of nitrogen, N2, has a pressure of 17.1 atm at 23°C. What is the mass of
nitrogen in the cylinder?
Answer: 985 g N2
Alternate Example 5.7 Calculating Gas Density
What is the density of methane gas (natural gas), CH4, at 125°C and 3.50 atm?
Answer: 1.72 g/L
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
77
Alternate Example 5.8 Determining the Molecular Weight of a Vapor
A 500.0-mL flask containing a sample of octane, a component of gasoline, is placed in a boiling
water bath in Denver, where the atmospheric pressure is 634 mmHg and water boils at 95.0°C.
The mass of the vapor required to fill the flask is 1.57 g. What is the molecular weight of octane?
The empirical formula of octane is C4H9. What is the molecular formula of octane?
Answer: 114 amu; C8H18
Alternate Example 5.9 Solving Stoichiometry Problems Involving Gas Volumes
When a 2.0-L bottle of concentrated HCl was spilled, 1.2 kg of CaCO3 was required to
neutralize the spill. What volume of CO2 was released by the neutralization at 735 mmHg and
20°C?
CaCO3(s) + 2HCl(aq) → CaCl2(aq) + H2O(l) + CO2(g)
Answer: 3.0 × 102 L CO2
First Alternate Example 5.10 Calculating Partial Pressures of a Gas in a Mixture
A 100.0-mL sample of air exhaled from the lungs is analyzed and found to contain 0.0830 g
N2, 0.0194 g O2, 0.00640 g CO2, and 0.00441 g water vapor at 35°C. What is the partial pressure
of each component and the total pressure of the sample?
Answer: P(N2) = 0.749 atm; P(O2) = 0.153 atm; P(CO2) = 0.0368 atm; P(H2O) = 0.0619 atm;
Ptotal = 1.00 atm
Second Alternate Example 5.10 Calculating Mole Fractions of a Gas in a Mixture
The partial pressure of air in the alveoli, the air sacs in the lungs, is as follows: nitrogen, 570.0
mmHg; oxygen, 103.0 mmHg; carbon dioxide, 40.0 mmHg; and water vapor, 47.0 mmHg.
What is the mole fraction of each component of alveolar air?
Answer: mol N2 = 0.7500; mol O2 = 0.1355; mol CO2 = 0.0526; mol H2O = 0.0618
Alternate Example 5.11 Calculating the Amount of Gas Collected over Water
You prepare nitrogen gas by heating ammonium nitrite:
NH4NO2(s) → N2(g) + 2H2O(l)
If you collected the nitrogen over water at 22°C and 727 mmHg, how many liters of gas would
you obtain from 5.68 g NH4NO2?
Answer: 2.31 L
Copyright © Houghton Mifflin Company. All rights reserved.
78
PART VII
Alternate Example 5.12 Calculating the rms Speed of Gas Molecules
What is the rms speed of carbon dioxide molecules in a container of gas at 23°C?
Answer: 4.10 × 102 m/s
Alternate Example 5.13 Calculating the Ratio of Effusion Rates of Gases
Both hydrogen and helium have been used as the buoyant gas in blimps. If a small leak were
to occur, which gas would effuse more rapidly and by what factor?
Answer: H2; 1.4 times as fast
Alternate Example 5.14 Using the van der Waals Equation
Use the van der Waals equation to calculate the pressure exerted by 2.00 mol CO2 that has a
volume of 10.0 L at 25°C. Compare with the pressure obtained from the ideal gas law.
Answer: 4.79 atm (van der Waals equation); 4.89 atm (ideal gas law)
CHAPTER 6 Thermochemistry
Alternate Example 6.1 Calculating Kinetic Energy
A person weighing 75.0 kg (165 lb) runs a course in 1.78 m/s (4.00 mph). What is this person’s
kinetic energy?
Answer: 119 J
Alternate Example 6.2 Writing Thermochemical Equations
Sulfur, S8, burns in air to produce sulfur dioxide. The reaction evolves 9.31 kJ of heat per gram
of sulfur at constant pressure. Write the thermochemical equation for this reaction.
Answer: S8(s) + 8O2(g) → 8SO2(g); ∆H = –2.39 × 103 kJ
Alternate Example 6.3 Manipulating Thermochemical Equations
When sulfur burns in air, the following reaction occurs (see Alternate Example 6.2):
S8(s) + 8O2(g) → 8SO2(g); ∆H = –2.39 × 103 kJ
Write the thermochemical equation for the dissociation of one mole of sulfur dioxide into its
elements.
Answer: SO2(g) → 18 S8(s) + O2(g); ∆H = +296 kJ
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
79
First Alternate Example 6.4 Calculating the Heat of Reaction from the Stoichiometry
You burn 15.0 g of sulfur in air. How much heat evolves from this amount of sulfur? The
thermochemical equation is
S8(s) + 8O2(g) → 8SO2(g); ∆H = –2.39 × 103 kJ
(This was obtained in Alternate Example 6.2.)
Answer: 1.40 × 102 kJ (q = –1.40 × 102 kJ)
Second Alternate Example 6.4 Calculating the Heat of Reaction
from the Stoichiometry
The daily energy requirement for a 20-year-old male weighing 67 kg is 1.3 × 104 kJ. For a
20-year-old female weighing 58 kg, the daily requirement is 8.8 × 103 kJ. If all this energy were
to be provided by the combustion of glucose, C6H12O6, how many grams of glucose would
have to be consumed by the male and the female each day?
C6H12O6(s) + 6O2(g) → 6CO2(g) + 6H2O(l); ∆H = –2.82 × 103 kJ
Answer: male, 830 g/day; female, 560 g/day
Alternate Example 6.5 Relating Heat and Specific Heat
A piece of zinc weighing 35.8 g was heated from 20.00°C to 28.00°C. How much heat was
required? The specific heat of zinc is 0.388 J/(g⋅°C).
Answer: 111 J
Alternate Example 6.6 Calculating ∆H from Calorimetric Data
Nitromethane, CH3NO2, an organic solvent, burns in oxygen to give the following reaction:
CH3NO2(l) + 34 O2(g) → CO2(g) + 32 H2O(l) + 12 N2(g)
You place a 1.724-g sample of nitromethane in a calorimeter with oxygen. The nitromethane
is ignited and burns in oxygen. The temperature of the calorimeter increases from 22.23°C to
28.81°C. In a separate experiment, you determine that the heat capacity of the calorimeter and
its contents is 3.044 kJ/°C. What is the ∆H of reaction (expressed as a thermochemical
equation)?
Answer: CH3NO2(l) + 34 O2(g) → CO2(g) + 32 H2O(l) + 12 N2(g); ∆H = –709 kJ
Copyright © Houghton Mifflin Company. All rights reserved.
80
PART VII
Alternate Example 6.7 Applying Hess’s Law
What is the enthalpy of reaction, ∆H, for the reaction of calcium metal with water?
Ca(s) + 2H2O(l) → Ca2+(aq) + 2OH–(aq) + H2(g)
This reaction occurs very slowly, so it is impractical to measure ∆H directly. However, ∆H of
the following reactions can be measured:
H+(aq) + OH–(aq) → H2O(l); ∆H = –55.9 kJ
Ca(s) + 2H+(aq) → Ca2+(aq) + H2(g); ∆H = –543.0 kJ
Answer: –431.2 kJ
Alternate Example 6.8 Calculating the Heat of Phase Transition from Standard
Enthalpies of Formation
What is the heat of vaporization of methanol, CH3OH, at 25°C and 1 atm? Use standard
enthalpies of formation (Appendix C).
Answer: 37.4 kJ/mol
Alternate Example 6.9 Calculating the Enthalpy of Reaction from Standard
Enthalpies of Formation
Methyl alcohol, CH3OH, is toxic because liver enzymes oxidize it to formaldehyde, HCHO,
which can coagulate protein. Calculate ∆H° for the following reaction; standard enthalpies of
formation are (in kJ/mol): CH3OH(aq), –245.9; HCHO(aq), –150.2; H2O(l), –285.8.
2CH3OH(aq) + O2(g) → 2HCHO(aq) + 2H2O(l)
Answer: –380.2 kJ
CHAPTER 7 Quantum Theory of the Atom
Alternate Example 7.1 Obtaining the Wavelength of Light from Its Frequency
What is the wavelength of blue light with a frequency of 6.4 × 1014/s?
Answer: 470 nm
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
81
Alternate Example 7.2 Obtaining the Frequency of Light from Its Wavelength
What is the frequency of red light having a wavelength of 681 nm?
Answer: 4.41 × 1014/s
Alternate Example 7.3 Calculating the Energy of a Photon
The blue–green line of the hydrogen atom spectrum has a wavelength of 486 nm. What is the
energy of a photon of this light?
Answer: 4.09 × 10–19 J
Alternate Example 7.4 Determining the Wavelength or Frequency of a Hydrogen
Atom Transition
What is the wavelength of the light emitted when the electron in a hydrogen atom undergoes
a transition from energy level n = 6 to level n = 3?
Answer: 1.09 × 103 nm (near infrared)
Alternate Example 7.5 Applying the de Broglie Relation
Compare the wavelengths of (a) an electron traveling at a speed of one-hundredth the speed
of light with (b) that of a baseball of mass 0.145 kg having a speed of 26.8 m/s (60.0 mi/hr).
Answers: (a) 243 pm (b) 1.71 × 10–34 m (too small to measure)
Alternate Example 7.6 Using the Rules for Quantum Numbers
Which of the following are permissible as sets of quantum numbers for an atomic orbital?
1
(a) n = 4, l = 4, ml = 0, ms = 2
1
(b) n = 3, l = 2, ml = 1, ms = – 2
3
(c) n = 2, l = 0, ml = 0, ms = 2
1
(d) n = 5, l = 3, ml = –3, ms = 2
Answers: (a) impermissible (l equals n) (b) permissible (c) impermissible ( 32 is not allowed for ms) (d) permissible
Copyright © Houghton Mifflin Company. All rights reserved.
82
PART VII
CHAPTER 8 Electron Configurations and Periodicity
Alternate Example 8.1 Applying the Pauli Exclusion Principle
Which of the following electron configurations or orbital diagrams are allowed and which are
not allowed by the Pauli exclusion principle? If they are not allowed, explain why.
(a)
(b)
(c)
(d)
1s22s12p3
1s22s12p8
1s22s22p63s23p63d8
1s22s22p63s23p63d11
(e)
Answers: (a) allowed (b) not allowed; only six electrons can be put into a p subshell
(c) allowed (d) not allowed; only ten electrons can be put into a d subshell (e) not allowed;
two electrons in an orbital must have opposite spin
Alternate Example 8.2 Determining the Configuration of an Atom Using the
Building-Up Principle
Write the complete electron configuration of the arsenic atom, As, using the building-up
principle.
Answer: 1s22s22p63s23p6 3d104s24p3
Alternate Example 8.3 Determining the Configuration of an Atom Using the Period
and Group Numbers
What are the electron configurations for the valence electrons of arsenic and cadmium?
Answer: arsenic—4s24p3; cadmium—4d105s2
Alternate Example 8.4 Applying Hund’s Rule
Write an orbital diagram for the ground state of the nickel atom.
Answer:
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
83
Alternate Example 8.5 Determining Relative Atomic Sizes from Periodic Trends
Refer to a periodic table and arrange the following in order of increasing atomic radius: Br,
Se, Te.
Answer: Te, Se, Br
Alternate Example 8.6 Determining Relative Ionization Energies from
Periodic Trends
Refer to the periodic table and arrange the following in order of increasing ionization energy:
As, Br, Sb.
Answer: Sb, As, Br
CHAPTER 9 Ionic and Covalent Bonding
Alternate Example 9.1 Using Lewis Symbols to Represent Ionic Bond Formation
Represent the transfer of electrons in forming calcium oxide, CaO, from atoms.
Answer:
Alternate Example 9.2 Writing the Electron Configuration and Lewis Symbol for a
Main-Group Ion
Obtain the electron configuration and the Lewis symbol for the chloride ion, Cl–.
Answer:
Alternate Example 9.3 Writing Electron Configurations of Transition-Metal Ions
Obtain the electron configurations of Mn and Mn2+.
Answer: Mn—1s22s22p63s23p63d54s2; Mn2+—1s22s22p63s23p63d5
Alternate Example 9.4 Using Periodic Trends to Obtain Relative Ionic Radii
Using a periodic table only, arrange the following ions in order of increasing ionic radius: Br–,
Se2–, Sr2+.
Answer: Sr2+, Br–, Se2–
Copyright © Houghton Mifflin Company. All rights reserved.
84
PART VII
Alternate Example 9.5 Using Electronegativities to Obtain Relative Bond Polarities
Using electronegativities, arrange the following bonds in order by increasing polarity: C—N,
Na—F, O—H.
Answer: C—N, O—H, Na—F
Alternate Example 9.6 Writing Lewis Formulas (Single Bonds Only)
Write electron-dot formulas for the following: (a) OF2; (b) NF3; (c) hydroxylamine, NH2OH.
Answers:
Alternate Example 9.7 Writing Lewis Formulas (Including Multiple Bonds)
Write electron-dot formulas for the following: (a) CO2, (b) HCN.
Answers:
Alternate Example 9.8 Writing Lewis Formulas (Ionic Species)
Phosphorus pentachloride exists in the solid state as the ionic compound [PCl4+][PCl6–]; it
exists in the gas phase as the PCl5 molecule. Write the Lewis formula of the PCl4+ ion.
Answer:
Alternate Example 9.9 Writing Resonance Formulas
Draw resonance formulas of the acetate ion, CH3COO–.
Answer:
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
85
Alternate Example 9.10 Writing Lewis Formulas (Exceptions to the Octet Rule)
Obtain the Lewis formula of the IF5 molecule.
Answer:
Alternate Example 9.11 Using Formal Charges to Determine the Best Lewis Formula
Compare the formal charges for the following electron-dot formulas of CO2:
Which is the preferred electron-dot formula?
Answer:
Alternate Example 9.12 Relating Bond Order and Bond Length
Consider the propylene molecule:
One of the carbon–carbon bonds has a length of 150 pm; the other has a length of 134 pm.
Identify each bond with a bond length.
—C bond length is 134 pm.
Answer: The C—C bond length is 150 pm; the C—
Copyright © Houghton Mifflin Company. All rights reserved.
86
PART VII
Alternate Example 9.13 Estimating ∆H from Bond Energies
Estimate the enthalpy change for the following reaction, using bond energies:
Answer: ∆H = –158 kJ
CHAPTER 10 Molecular Geometry and Chemical Bonding Theory
Alternate Example 10.1 Predicting Molecular Geometries (Two, Three, or Four
Electron Pairs)
Use the VSEPR model to predict the geometries of the following molecules: (a) AsF3, (b) PH4+,
(c) BCl3.
Answers: (a) trigonal pyramidal (b) tetrahedral (c) trigonal planar
Alternate Example 10.2 Predicting Molecular Geometries (Five or Six Electron Pairs)
Using the VSEPR model, predict the geometry of the following species: (a) ICl3, (b) ICl4–.
Answers: (a) T-shaped (b) square planar
Alternate Example 10.3 Relating Dipole Moment and Molecular Geometry
Which of the following molecules would be expected to have a zero dipole moment on the
basis of their geometry?
(a) GeF4 (b) SF2 (c) XeF2 (d) AsF3
Answers: GeF4, XeF2
Alternate Example 10.4 Applying Valence Bond Theory (Two, Three, or Four
Electron Pairs)
Use valence bond theory to describe the bonding about an N atom in N2F4.
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
87
Answer: The orbital diagram of the ground-state N atom is
The hybridized atom is
Each nitrogen atom forms two N—F bonds, one N—N bond, and one lone pair. An N—F bond
is formed by the overlap of an sp3 orbital on N with the singly occupied 2p orbital on the F
atom. One sp3 orbital on N is used for the lone pair.
Alternate Example 10.5 Applying Valence Bond Theory (Five or Six Electron Pairs)
Use valence bond theory to describe the bonding in the ClF2– ion.
Answer: The orbital diagram of the ground state of the Cl– ion is
The sp3d hybridized ion is
The equatorial sp3d hybrid orbitals are used for lone pairs; the axial hybrid orbitals are used
in forming Cl—F bonds. Each Cl—F bond is formed by overlapping an sp3d hybrid orbital on
Cl– with a singly occupied 2p orbital on F.
Alternate Example 10.6 Applying Valence Bond Theory (Multiple Bonding)
Describe the bonding about the C atom in formaldehyde, CH2O, using valence bond theory.
Answer: The C and O atoms are sp2 hybridized; each atom has an unhybridized 2p orbital
perpendicular to the plane of the hybrid orbitals on that atom. Each C—H bond is formed by
the overlap of the 1s orbital on the H atom with an sp2 hybrid orbital on C. The C—
—O bond
consists of a σ and a π orbital, each doubly occupied. The σ bond is formed by the overlap of
an sp2 hybrid orbital on the C atom with an sp2 hybrid orbital on the O atom. The π bond is
formed by the overlap of the 2p orbital on C with the 2p orbital on O.
Copyright © Houghton Mifflin Company. All rights reserved.
88
PART VII
Alternate Example 10.7 Describing Molecular Orbital Configurations
(Homonuclear Diatomic Molecules)
Give the orbital diagram and electron configuration of the F2 molecule. Is the molecular
substance diamagnetic or paramagnetic? What is the order of the bond in F2?
Answer: The orbital diagram is
σ2s σ∗2s
π2p
σ2p
π∗2p
σ∗2p
The configuration is
KK(σ2s)2(σ∗2s)2(π2p)4(σ2p)2(π∗2p)4
The molecular substance is diamagnetic; the bond order is 1.
Alternate Example 10.8 Describing Molecular Orbital Configurations
(Heteronuclear Diatomic Molecules)
A number of compounds of the nitrosonium ion, NO+, are known, including nitrosonium
hydrogen sulfate, (NO+)(HSO42–). Use the molecular orbitals similar to those of a homonuclear
diatomic molecule and obtain the orbital diagram, electron configuration, bond order, and
magnetic characteristics of the NO+ ion. (Note that the stability of the positive ion results from
the loss of an antibonding electron from NO.)
Answer: The orbital diagram is
σ2s σ∗2s
π2p
σ2p
π∗2p
σ∗2p
The electron configuration is
KK(σ2s)2(σ∗2s)2(π2p)4(σ2p)2
The bond order is 3; a substance containing the ion is diamagnetic (provided the anion is
also diamagnetic).
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
89
CHAPTER 11 States of Matter; Liquids and Solids
First Alternate Example 11.1 Calculating the Heat Required for a Phase Change of
a Given Mass of Substance
The fuel requirements of some homes are supplied by propane gas, C3H8, contained as the
liquid in steel cylinders. If a home uses 2.40 kg of propane in an average day, how much heat
must be absorbed by the propane cylinder each day to evaporate the liquid propane, forming
the gas that is subsequently burned? The heat of vaporization of propane is 16.9 kJ/mol.
Answer: 920 kJ
Second Alternate Example 11.1 Calculating the Heat Required for a Phase
Change of a Given Mass of Substance
A 25.0-g ice cube at 0°C is placed in a glass with 2.50 × 102 g of tea at 25.0°C. To what
temperature will the tea cool? Assume that no heat is lost to the surroundings and that the
specific heat of tea is 4.184 J/(g⋅°C). The heat of fusion of ice is 6.01 kJ/mol.
Answer: 15.5°C
Alternate Example 11.2 Calculating the Vapor Pressure at a Given Temperature
The vapor pressure of diethyl ether (commonly known simply as ether) is 439.8 mmHg at
20.0°C. The heat of vaporization of ether is 28.2 kJ/mol. What is the vapor pressure at 34°C?
Answer: 746 mmHg (The normal b.p. is 34.0°C.)
Alternate Example 11.3 Calculating the Heat of Vaporization from Vapor Pressures
The vapor pressures of ethanol (“alcohol”) are 100 mmHg and 760 mmHg (three significant
figures for each) at 34.9°C and 78.4°C, respectively. What is the heat of vaporization of alcohol?
Answer: 42.0 kJ/mol
Alternate Example 11.4 Relating the Conditions for the Liquefaction of Gases to the
Critical Temperature
Carbon dioxide is available in steel cylinders as the liquid at room temperature. Oxygen,
however, is available in steel cylinders (at room temperature) as the compressed gas, not the
liquid. Explain the difference. The critical temperatures of CO2 and O2 are 31°C and –119°C,
respectively.
Answer: The carbon dioxide is below its critical temperature, so under sufficient pressure
it liquefies. Oxygen, on the other hand, is above its critical temperature, so it cannot be
liquefied no matter how great the pressure.
Copyright © Houghton Mifflin Company. All rights reserved.
90
PART VII
Alternate Example 11.5 Identifying Intermolecular Forces
Identify the intermolecular forces that you expect for each of the following substances: (a) O2,
(b) H2O2, (c) CHBr3.
Answers: (a) London forces (b) dipole–dipole, hydrogen bonding, London forces
(c) dipole–dipole, London forces
Alternate Example 11.6 Determining Relative Vapor Pressure on the Basis of
Intermolecular Attraction
Which substance in each of the following pairs has the higher vapor pressure? (a) BCl3 or PCl3,
(b) H2O2 or H2S.
Answers: (a) BCl3 (b) H2S
Alternate Example 11.7 Identifying Types of Solids
Identify the type of solid that you would expect for each of the following substances: (a) NF3,
(b) CaBr2, (c) Na, (d) Ge.
Answers: (a) molecular (b) ionic (c) metallic (d) covalent network
Alternate Example 11.8 Determining Relative Melting Points Based on
Types of Solids
For each of the following, identify the type of solid. Then arrange the substances in order by
increasing melting point.
CaO, CH3CH2OH, NaCl, CH3Cl
Answer: CH3Cl (molecular), CH3CH2OH (molecular), NaCl (ionic), CaO (ionic)
Alternate Example 11.9 Determining the Number of Atoms per Unit Cell
How many atoms are there in a body-centered cubic lattice of a potassium crystal, in which
there are potassium atoms at each lattice point?
Answer: Two atoms
Alternate Example 11.10 Calculating Atomic Mass from Unit-Cell Dimension
and Density
Polonium crystallizes in a simple cubic lattice (one Po atom at each lattice point) with a
unit-cell length of 336 pm. The density of polonium metal is 9.20 g/cm3. Calculate the atomic
weight of polonium from these data. Avogadro’s number is 6.022 × 1023/mol.
Answer: 210 amu
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
91
Alternate Example 11.11 Calculating Unit-Cell Dimension from
Unit-Cell Type and Density
Sodium metal has a body-centered cubic lattice with one sodium atom at each lattice point.
The density of sodium metal is 0.968 g/cm3. Calculate the length of an edge of the unit cell.
The atomic weight of sodium is 22.99 amu.
Answer: 429 pm
CHAPTER 12 Solutions
Alternate Example 12.1 Applying Henry’s Law
Helium–oxygen mixtures are sometimes used as the breathing gas in deep-sea diving. At sea
level (where the pressure is 1.0 atm), the solubility of pure helium in blood is 0.94 g/100 mL.
What is the solubility of pure helium at a depth of 1500 ft? Pressure increases by 1.0 atm for
each 33 ft of depth, so at 1500 ft the pressure is 46 atm. (For a helium–oxygen mixture, the
solubility of helium will depend on the initial partial pressure of helium in the mixture, which
will be less than 1.0 atm.)
Answer: 43 g/100 mL
Alternate Example 12.2 Calculating Mass Percentage of Solute
An experiment calls for 36.0 g of a 5.00% aqueous solution of potassium bromide. Describe
how you would make up such a solution.
Answer: Dissolve 1.8 g KBr in 34.2 g H2O.
Alternate Example 12.3 Calculating the Molality of Solute
Iodine dissolves in various organic solvents, such as methylene chloride, in which it forms an
orange solution. What is the molality of I2 in a solution of 5.00 g of iodine, I2, in 30.0 g of
methylene chloride, CH2Cl2?
Answer: 0.657 m
First Alternate Example 12.4 Calculating the Mole Fractions of Components
A solution of iodine in methylene chloride, CH2Cl2, contains 1.50 g I2 and 56.00 g CH2Cl2.
What are the mole fractions of each component in the solution?
Answer: 8.89 × 10–3 mole fraction I2; 0.9911 mole fraction CH2Cl2
Copyright © Houghton Mifflin Company. All rights reserved.
92
PART VII
Second Alternate Example 12.4 Calculating the Mole Fractions of Components
A bottle of bourbon is labeled 94 proof, or 47% by volume alcohol in water. What is the mole
fraction of ethyl alcohol, C2H5OH, in the bourbon? The density of ethyl alcohol is 0.80 g/mL.
Answer: 0.22 mole fraction C2H5OH
Alternate Example 12.5 Converting Molality to Mole Fractions
A 3.6 m solution of calcium chloride is used in tractor tires to give them weight; the addition
of CaCl2 prevents the water from freezing at temperatures above about –20°C. What are the
mole fractions of CaCl2 and water in such a solution?
Answer: 0.061 mole fraction CaCl2, 0.939 mole fraction H2O
Alternate Example 12.6 Converting Mole Fractions to Molality
A solution contains 8.89 × 10–3 mole fraction I2 dissolved in 0.9911 mole fraction CH2Cl2
(methylene chloride). What is the molality of I2 in the solution?
Answer: 0.106 m
Alternate Example 12.7 Converting Molality to Molarity
Citric acid, HC6H7O7, is often used in fruit beverages to add tartness. An aqueous solution of
citric acid is 2.331 m HC6H7O7. What is the molarity of citric acid in the solution? The density
of the solution is 1.1346 g/mL.
Answer: 1.772 M
Alternate Example 12.8 Converting Molarity to Molality
An aqueous solution of ethanol, C2H5OH, is 14.1 M C2H5OH. The density of the solution is
0.853 g/cm3. What is the molality of ethanol in the solution?
Answer: 69.3 m
Alternate Example 12.9 Calculating Vapor-Pressure Lowering
Eugenol, C10H12O2, is the chief constituent of oil of clove. It is a pale yellow liquid that
dissolves in ethanol, C2H5OH; it has a boiling point of 255°C (thus, it has a relatively low vapor
pressure at room temperature). What is the vapor-pressure lowering at 20.0°C of ethanol
containing 8.56 g of eugenol in 50.0 g of ethanol? The vapor pressure of ethanol at 20.0°C is
44.6 mmHg.
Answer: 2.04 mmHg
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
93
Alternate Example 12.10 Calculating Boiling-Point Elevation and
Freezing-Point Depression
A solution was made up of eugenol, C10H12O2, in diethyl ether (“ether”). If the solution was
0.575 m eugenol in ether, what was the freezing point and the boiling point of the solution?
The freezing point and the boiling point of pure ether are –116.3°C and 34.6°C, respectively;
the freezing-point depression and boiling-point-elevation constants are 1.79°C/m and
2.02°C/m, respectively.
Answer: –117.3°C; 35.8°C
Alternate Example 12.11 Calculating the Molecular Weight of a Solute from
Molality
Anethole is the chief constituent of oil of anise, a flavoring agent having a licorice-like flavor.
A solution of 58.1 mg of anethole in 5.00 g of benzene is determined by freezing-point
depression to have a molality of 0.0784 m. What is the molecular weight of anethole?
Answer: 148 amu
First Alternate Example 12.12 Calculating the Molecular Weight from
Freezing-Point Depression
In a freezing-point experiment, the molality of a solution of 58.1 mg of anethole in 5.00 g of
benzene was determined to be 0.0784 m. What is the molecular weight of anethole?
Answer: 148 amu
Second Alternate Example 12.12 Calculating the Molecular Weight from
Boiling-Point Elevation
An 11.2-g sample of sulfur was dissolved in 40.0 g of carbon disulfide. The boiling-point
elevation of carbon disulfide was found to be 2.63°C. What is the molecular weight of the
sulfur in solution? What is the formula of molecular sulfur?
Answer: 256 amu; S8
Alternative Example 12.13 Calculating Osmotic Pressure
Dextran, a polymer of glucose units, is produced by bacteria growing in sucrose solutions.
Solutions of dextran in water have been used as a blood plasma substitute. What is the osmotic
pressure (in mmHg) at 21°C of a solution containing 1.50 g of dextran dissolved in 100.0 mL
of aqueous solution, if the average molecular weight of the dextran is 4.0 × 104 amu?
According to Example 5.1 on text page 182, 760.0 mmHg is equivalent to the pressure
exerted by a column of water 10.334 m high. Thus, each 1.00 mmHg of pressure is equivalent
to the pressure of a 1.36-cm column of water. If the density of this dextran solution is equal to
that of water, what height of solution would exert a pressure equal to its osmotic pressure?
Answer: 6.9 mmHg; 9.4 cm
Copyright © Houghton Mifflin Company. All rights reserved.
94
PART VII
Alternate Example 12.14 Determining Colligative Properties of Ionic Solutions
What is the osmotic pressure at 25.0°C of an isotonic saline solution (a solution having an
osmotic pressure equal to that of blood) that contains 0.900 g NaCl in 100.0 mL of aqueous
solution? Assume that i has the ideal value (based on the formula).
Answer: 7.53 atm
CHAPTER 14 Rates of Reaction
Alternate Example 14.1 Relating the Different Ways of Expressing Reaction Rates
Peroxydisulfate ion oxidizes iodide ion to the triiodide ion, I3–. (The triiodide ion has a brown
color and is formed by the reaction of iodine with iodide ion.) The reaction is
S2O82–(aq) + 3I–(aq) → 2SO42–(aq) + I3–(aq)
How is the rate of reaction that is expressed as the rate of formation of I3– related to the rate
of reaction of I–?
Answer:
∆[I3 −] 1 [I −]
=
3 ∆t
∆t
Alternate Example 14.2 Calculating the Average Reaction Rate
Calculate the average rate of formation of O2 in the following reaction during the time interval
from 1200 s to 1800 s using the data given in Figure 13.5 on text page 542.
2N2O5(g) → 4NO2(g) + O2(g)
The data are
Time
1200
1800
Answer:
[O2]
0.0036
0.0048
∆[O2]
= 2.0 × 10−6 M/s
∆t
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
95
Alternate Example 14.3 Determining the Order of Reaction from the Rate Law
Hydrogen peroxide oxidizes iodide ion in acidic solution:
H2O2(aq) + 3I–(aq) + 2H+(aq) → I3–(aq) + 2H2O(l)
The rate law for this reaction is
Rate = k[H2O2][I–]
What is the order of reaction with respect to each reactant species? What is the overall order?
Answer: First order with respect to H2O2, first order with respect to I–, second order overall.
Alternate Example 14.4 Determining the Rate Law from Initial Rates
Iron(II) is oxidized to iron(III) by chlorine in an acidic solution:
+
H
2Fe2+(aq) + Cl2(aq) → 2Fe3+(aq) + 2Cl–(aq)
The following data were collected (the rates given are relative, not actual, rates):
Initial Concentrations (mol/L)
Exp. 1
Exp. 2
Exp. 3
Exp. 4
Exp. 5
Exp. 6
[Fe2+]
0.0020
0.0040
0.0020
0.0040
0.0020
0.0020
[Cl2]
0.0020
0.0020
0.0040
0.0040
0.0020
0.0020
[H+]
1.0
1.0
1.0
1.0
0.5
0.1
Rate
1.0 × 10–5
2.0 × 10–5
2.0 × 10–5
4.0 × 10–5
2.0 × 10–5
1.0 × 10–4
What is the reaction order with respect to Fe2+, Cl2, and H+? What is the rate law and the
relative rate constant?
Answer: First order with respect to both Fe2+ and Cl2; –1 order with respect to H+.
k[Fe2+][Cl2]
Rate =
; k = 2.5.
[H+]
Alternate Example 14.5 Using an Integrated Rate Law
Cyclopropane is used as an anesthetic. The isomerization of cyclopropane to propene is a
first-order reaction with a rate constant of 9.2/s at 1000°C. If an initial sample of cyclopropane
has a concentration of 6.00 M, what will the cyclopropane concentration be after 1.00 s?
Answer: 6.1 × 10–4 M
Copyright © Houghton Mifflin Company. All rights reserved.
96
PART VII
Alternate Example 14.6 Relating the Half-Life of a Reaction to the Rate Constant
Ammonium nitrite is unstable because ammonium ion reacts with nitrite ion to produce
nitrogen:
NH4+(aq) + NO2–(aq) → N2(g) + 2H2O(l)
In a solution that is 10.0 M in NH4+, the reaction is first order in nitrite ion (for low
concentrations), and the rate constant at 25°C is 3.0 × 10–3/s. What is the half-life of the
reaction?
Answer: 2.3 × 102 s
Alternate Example 14.7 Using the Arrhenius Equation
A convenient rule of thumb is that the rate of a reaction doubles for a 10°C change in
temperature. What is the activation energy for a reaction whose rate doubles from 10.0°C to
20.0°C? By what factor would the reaction rate increase if the temperature were increased from
10.0°C to 25.0°C?
Answer: 47.8 kJ/mol; 2.78
Alternate Example 14.8 Writing the Overall Chemical Equation from a Mechanism
Chlorofluorocarbons, such as CCl2F2, decompose in the stratosphere from the irradiation with
short-wavelength ultraviolet light present at those altitudes. The decomposition yields chlorine atoms. These chlorine atoms catalyze the decomposition of ozone in the presence of
oxygen atoms (available in the stratosphere from the ultraviolet irradiation of O2) to give
oxygen molecules. The mechanism of the decomposition is
Cl(g) + O3(g) → ClO(g) + O2(g)
ClO(g) + O(g) → Cl(g) + O2(g)
What is the overall chemical equation for the decomposition of ozone?
Answer: Cl(g) + O3(g) → ClO(g) + O2(g)
ClO(g) + O(g) → Cl(g) + O2(g)
O3(g) + O(g) → 2O2(g)
Note that Cl atoms are used up in the first step but regenerated in the second step, so Cl atoms
do not appear in the overall equation. In other words, Cl functions as a catalyst (see Section
13.9).
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
97
Alternate Example 14.9 Determining the Molecularity of an Elementary Reaction
What is the molecularity of each of the steps in the mechanism of ozone decomposition
described in Alternate Example 13.8?
Answer: Each step is bimolecular.
Alternate Example 14.10 Writing the Rate Equation for an Elementary Reaction
(a) Write the rate equation for the first step in the ozone decomposition mechanism described
in Alternate Example 13.8. (b) Write the rate equation for the following elementary reaction:
NO(g) + NO(g) → N2O2(g).
Answers: (a) Rate = k[Cl][O3] (b) Rate = k[NO]2
Alternate Example 14.11 Determining the Rate Law from a Mechanism with an
Initial Slow Step
The decomposition of hydrogen peroxide is catalyzed by iodide ion. The mechanism is
thought to be
H2O2(aq) + I–(aq) → H2O(l) + IO–(aq)
IO–(aq) + H2O2(aq) → H2O(l) + O2(g) + I–(aq)
At 25°C, the first step is slow relative to the second step. What is the rate law predicted by this
mechanism?
Answer: Rate = k[H2O2][I–]
Alternate Example 14.12 Determining the Rate Law from a Mechanism with an
Initial Fast, Equilibrium Step
The mechanism for the decomposition of hydrogen peroxide in the presence of iodide ion is
described in Alternate Example 13.11. At 100°C, the first step is fast relative to the second step.
What is the rate law predicted by this mechanism? Note that [H2O] can be taken as constant.
Answer: Rate = k[H2O2]2[I–]
Copyright © Houghton Mifflin Company. All rights reserved.
98
PART VII
CHAPTER 15 Chemical Equilibrium
Alternate Example 15.1 Applying Stoichiometry to an Equilibrium Mixture
When heated, phosphorus pentachloride, PCl5, forms PCl3 and Cl2 as follows:
PCl5(g)
PCl3(g) + Cl2(g)
When 1.00 mol PCl5 in a 1.00-L container is allowed to come to equilibrium at a certain
temperature, the mixture is found to contain 0.135 mol PCl3. What is the molar composition
of the mixture; that is, how many moles of each substance are present?
Answer: 0.135 mol PCl3, 0.135 mol Cl2, and 0.865 mol PCl5
Alternate Example 15.2 Writing Equilibrium-Constant Expressions
Methanol, wood alcohol, is made commercially by hydrogenation of carbon monoxide at
elevated temperature and pressure in the presence of a catalyst:
2H2(g) + CO(g)
CH3OH(g)
What is the Kc expression for this reaction?
Answer: Kc =
[CH3OH]
[H2]2[CO]
Alternate Example 15.3 Obtaining an Equilibrium Constant from Reaction
Composition
Carbon dioxide decomposes at elevated temperatures to carbon monoxide and oxygen:
2CO2(g)
2CO(g) + O2(g)
At 3000 K, 2.00 mol CO2 is placed into a 1.00-L container and allowed to come to equilibrium.
At equilibrium, 0.90 mol CO2 remains. What is the value for Kc at this temperature?
Answer: Kc = 0.82
Alternate Example 15.4 Writing Kc for a Reaction with Pure Solids or Liquids
When water, in the form of steam, is passed over hot coke (carbon), a mixture of hydrogen
and carbon monoxide, called water gas, is formed. This mixture can be used as a fuel. Write
the equilibrium-constant (Kc) expression for this process.
H2O(g) + C(s)
Answer: Kc =
CO(g) + H2(g)
[CO][H2]
[H2O]
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
99
Alternate Example 15.5 Using the Reaction Quotient
Nickel(II) oxide can be reduced to the metal by treatment with carbon monoxide.
CO(g) + NiO(s)
CO2(g) + Ni(s)
If the partial pressure of CO is 100. mmHg and the total pressure of CO and CO2 does not
exceed 1.0 atm, will reaction occur at 1500 K at equilibrium? (Kp = 700. at 1500 K.)
Answer: Reaction will occur; Q = 6.6, and more Ni(s) will form.
Alternate Example 15.6 Obtaining One Equilibrium Concentration Given the Others
Nitrogen and oxygen form nitric oxide.
N2(g) + O2(g)
2NO(g)
If an equilibrium mixture at 25°C contains 0.040 mol/L of N2 and 0.010 mol/L of O2, what is
the concentration of NO in this mixture? The equilibrium constant at 25°C is 1 × 10–30.
Answer: 2 × 10–17 mol/L
Alternate Example 15.7 Solving an Equilibrium Problem (Involving a Linear
Equation in x)
Hydrogen iodide decomposes to hydrogen gas and iodine gas.
2HI(g)
H2(g) + I2(g)
At 800 K, the equilibrium constant, Kc, for this reaction is 0.016. If 0.50 mol HI is placed in a
5.0-L flask, what will be the composition of the equilibrium mixture?
Answer: [HI] = 0.080 M; [H2] = [I2] = 0.010 M
Alternate Example 15.8 Solving an Equilibrium Problem (Involving a Quadratic
Equation in x)
N2O4 decomposes to NO2; the equilibrium equation in the gaseous phase is
N2O4(g)
2NO2(g)
At 100°C, Kc = 0.36. If a 1.00-L flask initially contains 0.100 mol N2O4/L, what will be the
concentration of NO2 at equilibrium?
Answer: [NO2] = 0.12 mol/L
Copyright © Houghton Mifflin Company. All rights reserved.
100
PART VII
Alternate Example 15.9 Applying Le Chatelier’s Principle When a
Concentration Is Altered
The Fischer–Tropsch process for the synthesis of gasoline consists of passing a mixture of
carbon monoxide and hydrogen over an iron–cobalt catalyst. A typical reaction that occurs in
the process is as follows:
8CO(g) + 17H2(g)
C8H18(g) + 8H2O(g)
Suppose the reaction mixture comes to equilibrium at 200°C, then is suddenly cooled to room
temperature where octane (C8H18) liquefies. The remaining gases are then reheated to 200°C.
What is the direction of the reaction as equilibrium is attained?
Answer: Left to right
Alternate Example 15.10 Applying Le Chatelier’s Principle When the
Pressure Is Altered
A typical reaction that occurs in the Fischer–Tropsch process is
8CO(g) + 17H2(g)
C8H18(g) + 8H2O(g)
Would you expect more or less of the product octane, C8H18, at equilibrium as the pressure
increases?
Answer: More product at high pressure
Altered Example 15.11 Applying Le Chatelier’s Principle When the
Temperature Is Altered
Calculate ∆H° for the chemical equation given in the previous alternate example, using
standard heats of formation, ∆H°f . From the result, predict whether more or less octane, C8H18,
would be produced at 200°C than at 20°C. Values of ∆H°f (in kJ/mol) are as follows: CO(g),
–110; C8H18(g), –209; H2O(g), –242.
Answer: ∆H° = –1265 kJ. Higher temperature favors less product at equilibrium. (However,
equilibrium is attained more quickly at higher temperature.)
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
101
CHAPTER 16 Acids and Bases
Alternate Example 16.1 Identifying Acid and Base Species
Identify the acid and base species in the following equations:
(a) CO32–(aq) + H2O(l)
HCO3–(aq) + OH–(aq)
–
(b) C2H3O2 (aq) + HNO2(aq)
HC2H3O2(aq) + NO2–(aq)
Answers: (a) acid species—H2O, HCO3–; base species—CO32–, OH– (b) acid species—
HNO2, HC2H3O2; base species—C2H3O2–, NO2–
Alternate Example 16.2 Identifying Lewis Acid and Base Species
Calcium oxide reacts with sulfur dioxide to produce calcium sulfite. The reaction is useful in
removing sulfur dioxide from the gases produced in the combustion of sulfur-containing
materials. We can represent this reaction as the reaction of the oxide ion with sulfur dioxide.
Label each species on the left as either Lewis acid or Lewis base.
Answer: O2–, Lewis base; SO2, Lewis acid
Alternate Example 16.3 Deciding Whether Reactants or Products Are Favored in an
Acid–Base Reaction
Decide which species are favored at the completion of the following reaction:
HCN(aq) + HSO3–(aq)
CN–(aq) + H2SO3(aq)
Answer: The reactants are favored.
Alternate Example 16.4 Calculating Concentrations of H3O+and OH– in Solutions of
a Strong Acid or Base
Calculate the concentrations of hydronium ion and hydroxide ion at 25°C in: (a) 0.10 M HCl,
(b) 1.4 × 10–4 M Mg(OH)2, a strong base.
Answers: (a) [H3O+] = 0.10 M; [OH–] = 1.0 × 10–13 M
(b) [H3O+] = 3.6 × 10–11 M; [OH–] = 2.8 × 10–4 M
Copyright © Houghton Mifflin Company. All rights reserved.
102
PART VII
Alternate Example 16.5 Calculating the pH from the Hydronium-Ion Concentration
Calculate the pH of typical adult blood, which has a hydronium-ion concentration of
4.0 × 10–8 M.
Answer: pH = 7.40
Alternate Example 16.6 Calculating the Hydronium-Ion Concentration from the pH
The pH of natural rain is 5.60. Calculate its hydronium-ion concentration.
Answer: [H3O+] = 2.5 × 10–6 M
CHAPTER 17 Acid–Base Equilibria
Alternate Example 17.1 Determining Ka from the Solution pH
Sore-throat medications sometimes contain the weak acid phenol, HC6H5O. A 0.10 M solution
of phenol has a pH of 5.43 at 25°C. What is the acid-ionization constant, Ka, for this acid at
25°C? What is its degree of ionization?
Answer: Ka = 1.4 × 10–10; degree of ionization = 3.7 × 10–5
Alternate Example 17.2 Calculating Concentrations of Species in a Weak Acid
Solution Using Ka (Approximation Method)
Para-hydroxybenzoic acid is used to make certain dyes. What are the concentrations of this
acid, of hydrogen ion, and of para-hydroxybenzoate anion in a 0.200 M aqueous solution at
25°C? What is the pH of the solution and the degree of ionization of this acid? The Ka of this
acid is 2.6 × 10–5.
Answer: [p-hydroxybenzoic acid] = 0.198 M; [H+] = [anion] = 2.3 × 10–3 M; pH = 2.64; degree
of ionization = 0.012
Alternate Example 17.3 Calculating Concentrations of Species in a Weak Acid
Solution Using Ka (Quadratic Formula)
What is the pH at 25°C of 400 mL of aqueous solution containing 0.400 mol of chloroacetic
acid, a monoprotic acid? The Ka = 1.35 × 10–3.
Answer: [H+] = 3.6 × 10–2 M (using quadratic formula); pH = 1.44
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
103
Alternate Example 17.4 Calculating Concentrations of Species in a Solution of a
Diprotic Acid
Tartaric acid, H2C4H4O6, is a diprotic acid used in food products. What is the pH of a 0.10 M
solution and the concentration of the C4H4O62– ion? Ka1 = 9.2 × 10–4 and Ka2 = 4.3 × 10–5.
Answer: pH = 2.02; [C4H4O62–] = 4.3 × 10–5 (= Ka2)
Alternate Example 17.5 Calculating Concentrations of Species in a Weak Base
Solution Using Kb
Aniline, C6H5NH2, is used in the manufacturing of some perfumes. What is the pH of a 0.035
M solution of aniline at 25°C ? The Kb = 4.2 × 10–10 at 25°C.
Answer: pH = 8.56
Alternate Example 17.6 Predicting Whether a Salt Solution Is Acidic, Basic,
or Neutral
Ammonium nitrate, NH4NO3, is administered as an intravenous solution to patients whose
blood pH has deviated from the normal value of 7.40. Would this substance be used for
acidosis (blood pH < 7.40) or alkalosis (blood pH > 7.40)?
Answer: NH4NO3 is a salt of a weak base and strong acid, so its solution would be acidic;
it would be used for alkalosis.
Alternate Example 17.7 Obtaining Ka from Kb or Kb from Ka
Obtain the Kb for the F– ion, the ion added to public water supplies to protect teeth. For HF,
Ka = 6.8 × 10–4.
Answer: Kb = 1.5 × 10–11
Alternate Example 17.8 Calculating Concentrations of Species in a Salt Solution
Household bleach is a 5% solution of sodium hypochlorite, NaClO. This corresponds to a
molar concentration of about 0.70 M NaClO (Kb = 2.86 × 10–7). What are the OH– concentration
and the pH of such a solution?
Answer: [OH–] = 4.5 × 10–4; pH = 10.65
Copyright © Houghton Mifflin Company. All rights reserved.
104
PART VII
Alternate Example 17.9 Calculating the Common-Ion Effect on Acid Ionization
(Effect of a Strong Acid)
Calculate the degree of ionization of benzoic acid, HC7H5O2, in a 0.15 M solution to which
sufficient HCl is added to make it also 0.010 M HCl. Compare the degree of ionization to that
of 0.15 M benzoic acid (no HCl). Ka = 6.3 × 10–5.
Answer: Degree of ionization = 0.0063; this is much smaller than the degree of ionization
of 0.15 M benzoic acid without HCl (0.020).
Alternate Example 17.10 Calculating the Common-Ion Effect on Acid Ionization
(Effect of a Conjugate Base)
Calculate the pH of a 0.10 M solution of HF to which sufficient sodium fluoride is added to
make the concentration 0.20 M NaF. The Ka of HF = 6.8 × 10–4.
Answer: pH = 3.47
Alternate Example 17.11 Calculating the pH of a Buffer from Given Volumes
of Solution
What is the pH of a buffer made by mixing 1.00 L of 0.020 M benzoic acid, HC7H5O2, with 3.00
L of 0.060 M sodium benzoate, NaC7H5O2? The Ka for benzoic acid is 6.3 × 10–5.
Answer: pH = 5.15
Alternate Example 17.12 Calculating the pH of a Buffer When a Strong Acid or
Strong Base Is Added
Calculate the pH change that will result from the addition of 5.0 mL of 0.10 M HCl to 50.0 mL
of a buffer containing 0.10 M NH3 and 0.10 M NH4+. How much would the pH of 50.0 mL of
water change if the same amount of acid were added?
Answer: The pH of the buffer decreases by 0.09 pH units from 9.26 to 9.17. The pH of the
water decreases by 4.96 pH units from 7.00 to 2.04.
Alternate Example 17.13 Calculating the pH of a Solution of a Strong Acid and a
Strong Base
Calculate the pOH and the pH of a solution in which 10.0 mL of 0.100 M HCl is added to 25.0
mL of 0.100 M NaOH.
Answer: pOH = 1.368; pH = 12.632
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
105
Alternate Example 17.14 Calculating the pH at the Equivalence Point in the
Titration of a Weak Acid by a Strong Base
Calculate the [OH–] and the pH at the equivalence point for the titration of 500. mL of 0.10 M
propionic acid with 0.050 M calcium hydroxide. (This can be used to prepare a preservative
for bread.) Ka = 1.3 × 10–5.
Answer: [OH–] = 6.2 × 10–6 M; pH = 8.79
CHAPTER 18 Solubility and Complex-Ion Equilibria
Alternate Example 18.1
Writing Solubility Product Expressions
Write the solubility product expression for the following salts: (a) Hg2Cl2; (b) HgCl2.
Answers: (a) Hg2Cl2: Ksp = [Hg22+][Cl–]2
(b) HgCl2: Ksp = [Hg2+][Cl–]2
Alternate Example 18.2 Calculating Ksp from the Solubility (Simple Example)
Exactly 0.133 mg of AgBr will dissolve in 1.00 L of water. What is the value of Ksp for AgBr?
Answer: Ksp = 5.02 × 10–13
Alternate Example 18.3 Calculating Ksp from the Solubility
(More Complicated Example)
An experimenter finds that the solubility of barium fluoride is 1.1 g in 1.00 L of water at 25°C.
What is the value of Ksp for barium fluoride, BaF2, at this temperature?
Answer: Ksp = 1.0 × 10–6
Alternate Example 18.4 Calculating the Solubility from Ksp
Calomel, whose chemical name is mercury(I) chloride, Hg2Cl2, was once used in medicine (as
a laxative and diuretic). It has a Ksp equal to 1.3 × 10–18. What is the solubility of Hg2Cl2 in
grams per liter?
Answer: 6.9 × 10–7 mol/L = 3.2 × 10–4 g/L
Copyright © Houghton Mifflin Company. All rights reserved.
106
PART VII
Alternate Example 18.5 Calculating the Solubility of a Slightly Soluble Salt in a
Solution of a Common Ion
What is the molar solubility of silver chloride in 1.0 L of solution that contains 2.0 × 10–2 mol
of HCl?
Answer: 9.0 × 10–9 M
Alternate Example 18.6 Predicting Whether Precipitation Will Occur
(Given the Ion Concentrations)
One form of kidney stones is calcium phosphate, Ca3(PO4)2, which has a Ksp of 1 × 10–26. If a
sample of urine contains 1.0 × 10–3 M Ca2+ and 1.0 × 10–8 M PO43– ion, calculate Qc and predict
whether Ca3(PO4)2 will precipitate.
Answer: Qc = 1.0 × 10–25. Precipitation will occur.
Alternate Example 18.7 Predicting Whether Precipitation Will Occur
(Given Solution Volumes and Concentrations)
Exactly 0.400 L of 0.50 M Pb2+ and 1.60 L of 2.50 × 10–2 M Cl– are mixed together to form
2.00 L of solution. Calculate Qc and predict whether PbCl2 will precipitate. The Ksp of PbCl2 is
1.6 × 10–5.
Answer: Qc = 4.0 × 10–5. Precipitation will occur.
Alternate Example 18.8 Determining the Qualitative Effect of pH on Solubility
Consider the two slightly soluble salts barium fluoride and silver bromide. Which of these
would have its solubility more affected by the addition of strong acid? Would the solubility
of that salt increase or decrease?
Answer: The barium fluoride is much more soluble in acidic solution, whereas the solubility of the silver bromide is not affected.
Alternate Example 18.9 Calculating the Concentration of a Metal Ion in
Equilibrium with a Complex Ion
What is the concentration of Ag+(aq) ion in 0.00010 M AgNO3 that is also 1.0 M CN–? The Kf
for Ag(CN)2– is 5.6 × 1018.
Answer: [Ag+] = 1.8 × 10–23 M
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
107
Alternate Example 18.10 Predicting Whether a Precipitate Will Form in the
Presence of the Complex Ion
Silver chloride usually does not precipitate in solutions of 1.00 M NH3 (see Example 17.10 on
text page 745). However, silver bromide has a smaller Ksp. Will silver bromide precipitate from
a solution containing 0.010 M AgNO3, 0.010 M NaBr, and 1.00 M NH3? Calculate the Qc value
and compare it with silver bromide’s Ksp of 5.0 × 10–13.
Answer: Qc = 6.1 × 10–12; thus, AgBr will precipitate.
Alternate Example 18.11 Calculating the Solubility of a Slightly Soluble Ionic
Compound in a Solution of the Complex Ion
Calculate the molar solubility of AgBr in 1.0 M NH3 at 25°C.
Answer: [Ag(NH3)2+] = 2.9 × 10–3 M = molar solubility
CHAPTER 19 Thermodynamics and Equilibrium
Alternate Example 19.1 Calculating the Entropy Change for a Phase Transition
Acetone, CH3COCH3, is a volatile liquid solvent (it is used in nail polish, for example). The
standard enthalpy of formation of the liquid at 25°C is –247.6 kJ/mol; the same quantity for
the vapor is –216.6 kJ/mol. What is the entropy change when 1.00 mol liquid acetone vaporizes
at 25°C?
Answer: 104.0 J/(K⋅mol)
Alternate Example 19.2 Predicting the Sign of the Entropy Change of a Reaction
The opening to Chapter 6, on thermochemistry, describes the endothermic reaction of solid
barium hydroxide octahydrate and solid ammonium nitrate:
Ba(OH)2⋅8H2O(s) + 2NH4NO3(s) → 2NH3(g) + 10H2O(l) + Ba(NO3)2(aq)
Predict the sign of ∆S° for the reaction.
Answer: positive
Copyright © Houghton Mifflin Company. All rights reserved.
108
PART VII
Alternate Example 19.3 Calculating ∆S° for a Reaction
When wine is exposed to air in the presence of certain bacteria, the ethyl alcohol is oxidized
to acetic acid, giving vinegar. Calculate the standard entropy change at 25°C for the following
similar reaction:
CH3CH2OH(l) + O2(g) → CH3COOH(l) + H2O(l)
The standard entropies of the substances in J/(K⋅mol) at 25°C are CH3CH2OH(l), 161; O2(g),
205; CH3COOH(l), 160; H2O(l), 69.9.
Answer: ∆S° = –136 J/K
Alternate Example 19.4 Calculating ∆G° from ∆H ° and ∆S°
Using standard enthalpies of formation and the value of ∆S° obtained in Alternate Example
18.3, calculate ∆G° at 25°C for the oxidation of ethyl alcohol to acetic acid. (See Alternate
Example 18.3 for the equation.) The standard enthalpies of formation of the substances in
kJ/mol at 25°C are CH3CH2OH(l), –277.6; CH3COOH(l), –487.0; H2O(l), –285.8.
Answer: ∆H° = –495.2 kJ, ∆G° = –454.7 kJ
Alternate Example 19.5 Calculating ∆G° from Standard Free Energies of Formation
Calculate the free-energy change, ∆G°, at 25°C for the oxidation of ethyl alcohol to acetic acid
using standard free energies of formation. (See Alternate Example 18.3 for the equation.) The
standard free energies of formation of the substances in kJ/mol at 25°C are CH3CH2OH(l),
–174.8; CH3COOH(l), –392.5; H2O(l), –237.2.
Answer: ∆G° = –454.9 kJ
Alternate Example 19.6 Interpreting the Sign of ∆G°
Consider the reaction discussed in Alternate Example 18.2:
Ba(OH)2⋅8H2O(s) + 2NH4NO3(s) → 2NH3(g) + 10H2O(l) + Ba(NO3)2(aq)
The standard enthalpy change at 25°C is 170.4 kJ; the standard entropy change at 25°C is 657
J/K. Calculate ∆G° at 25°C for the reaction. Interpret the values of ∆H°, ∆S°, and ∆G°.
Answer: ∆G° = –25 kJ. The positive value of ∆H° indicates an endothermic reaction; the
large positive ∆S° indicates the formation of considerable disorder (formation of gas, liquid,
and solution from two crystalline solids); the negative ∆G° indicates a spontaneous reaction.
Note that the negative ∆G° results from the fact that although ∆H° is positive, ∆S° is a large
positive number.
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
109
Alternate Example 19.7 Writing the Expression for a Thermodynamic Equilibrium
Constant
Write the expressions for the thermodynamic equilibrium constants for each of the following
reactions.
2NO2(g)
(a) N2O4(g)
Zn2+(aq) + H2(g)
(b) Zn(s) + 2H+(aq)
p(NO2)2
[Zn2+]p(H2)
(b) K =
Answers: (a) K =
p(N2O4)
[H+]2
Alternate Example 19.8 Calculating K from the Standard Free-Energy Change
(Molecular Equation)
Calculate the value of the thermodynamic equilibrium constant at 25°C for the reaction given
in Alternate Example 18.7(a):
N2O4(g)
2NO2(g)
The values of the standard free energy of formation of the substances in kJ/mol at 25°C are
NO2, 51.30; N2O4, 97.82.
Answer: K = 0.145
Alternate Example 19.9 Calculating K from the Standard Free-Energy Change
(Net Ionic Equation)
Calculate the value of the thermodynamic equilibrium constant at 25°C for the reaction given
in Alternate Example 18.7(b):
Zn(s) + 2H+(aq)
Zn2+(aq) + H2(g)
The values of the standard free energy of formation of the substances in kJ/mol at 25°C are
H+(aq), 0; Zn2+(aq), –147.2.
Answer: K = 6.1 × 1025
Copyright © Houghton Mifflin Company. All rights reserved.
110
PART VII
Alternate Example 19.10 Calculating ∆G° and K at Various Temperatures
Obtain the standard free-energy change and Kp at 35°C for the reaction whose free-energy
change and equilibrium constant were obtained at 25°C in Alternate Example 18.8:
N2O4(g)
2NO2(g)
The standard enthalpies of formation of the substances in kJ/mol at 25°C are N2O4, 9.16; NO2,
33.2. The standard entropies at 25°C, in J/(K⋅mol) are N2O4, 304.3; NO2, 239.9.
Answer: ∆G° at 35°C is 3.2 kJ; Ksp is 0.29.
CHAPTER 20 Electrochemistry
Alternate Example 20.1 Balancing Equations by the Half-Reaction Method (Acidic
Solution)
Nitrate ion in acid solution (nitric acid) is an oxidizing agent. When it reacts with zinc, the
metal is oxidized to the zinc ion, Zn2+, and nitrate is reduced. Assume that nitrate is reduced
to the ammonium ion, NH4+. Write the balanced ionic equation for this reaction; use the
half-reaction method.
Answer: 4Zn + NO3– + 10H+ → 4Zn2+ + NH4+ + 3H2O
Alternate Example 20.2 Balancing Equations by the Half-Reaction Method (Basic
Solution)
Lead(II) ion, Pb2+, yields the plumbite ion, Pb(OH)3–, in basic solution. In turn, this ion is
oxidized in basic hypochlorite solution, ClO–, to lead(IV) oxide, PbO2. Balance the equation
for this reaction, using the half-reaction method. The skeleton equation is
Pb(OH)3– + ClO– → PbO2 + Cl–
Answer: ClO– + Pb(OH)3– → Cl– + PbO2 + H2O + OH–
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
111
Alternate Example 20.3 Sketching and Labeling a Voltaic Cell
You construct one half-cell of a voltaic cell by inserting a copper metal strip into a solution of
copper(II) sulfate. You construct another half-cell by inserting an aluminum metal strip into
a solution of aluminum nitrate. You now connect the half-cells by a salt bridge. When
connected to an external circuit, the aluminum is oxidized. Sketch the resulting voltaic cell.
Label the anode and cathode, showing the corresponding half-reactions. Indicate the direction
of electron flow in the external circuit.
Answer: Aluminum is the anode; copper is the cathode. The electrons flow from the anode
to the cathode. The half-reactions are
Al(s) → Al3+(aq) + 3e–
Cu2+(aq) + 2e– → Cu(s)
Alternate Example 20.4 Writing the Cell Reaction from the Cell Notation
The cell notation for the voltaic cell in Alternate Example 19.3 is
Al(s)Al3+(aq)Cu2+(aq)Cu(s)
Write the cell reaction.
Answer: 2Al(s) + 3Cu2+(aq) → 2Al3+(aq) + 3Cu(s)
Alternate Example 20.5 Calculating the Quantity of Work from a Given Amount of
Cell Reactant
The emf of a particular cell constructed as described in Alternate Example 19.3 is 0.500 V. The
cell reaction, given in Alternate Example 19.2, is
2Al(s) + 3Cu2+(aq) → 2Al3+(aq) + 3Cu(s)
Calculate the maximum electrical work of this cell obtained from 1.00 g of aluminum.
Answer: –5.36 kJ
Alternate Example 20.6 Determining the Relative Strengths of Oxidizing and
Reducing Agents
(a) Which is the stronger reducing agent under standard conditions, Sn2+ (to Sn4+) or Fe (to
Fe2+)? (b) Which is the stronger oxidizing agent under standard conditions, Cl2 or MnO4–?
Answers: (a) Fe (b) MnO4–
Copyright © Houghton Mifflin Company. All rights reserved.
112
PART VII
Alternate Example 20.7 Determining the Direction of Spontaneity from
Electrode Potentials
Will dichromate ion oxidize manganese(II) ion to permanganate ion in acid solution under
standard conditions?
Answer: no
Alternate Example 20.8 Calculating the emf from Standard Potentials
A fuel cell is simply a voltaic cell that uses a continuous supply of electrode materials to
provide a continuous supply of electrical energy. A fuel cell employed by NASA on spacecraft
uses hydrogen and oxygen under basic conditions to produce electricity; the water also
produced can be used for drinking. The net reaction is
2H2(g) + O2(g) → 2H2O(l)
Calculate the standard emf of the oxygen–hydrogen fuel cell.
2H2O(l) + 2e–
H2(g) + 2OH–(aq)
O2(g) + 2H2O(l) + 4e–
4OH–(aq)
E° = –0.83 V
E° = 0.40 V
Answer: 1.23 V
Alternate Example 20.9 Calculating the Free-Energy Change from
Electrode Potentials
Calculate the standard free-energy change for the net reaction used in the hydrogen–oxygen
fuel cell described in Alternate Example 19.8.
2H2(g) + O2(g) → 2H2O(l)
The emf of the cell was calculated in that example. How does this compare with ∆G°f of H2O(l)?
Answer: –475 kJ (for the formation of 2 mol H2O); this is 2∆G°f
Alternate Example 20.10 Calculating the Cell emf from Free-Energy Change
A voltaic cell consists of one half-cell with Fe dipping into an aqueous solution of 1.0 M FeCl2
and the other half-cell with Ag dipping into an aqueous solution of 1.0 M AgNO3. Obtain the
standard free-energy change for the cell reaction using standard free energies of formation.
The standard free energies of formation of the ions in kJ/mol are Ag+(aq), 77; Fe2+(aq), –85.
What is the emf of this cell?
Answer: –239 kJ, 1.24 V
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
113
Alternate Example 20.11 Calculating the Equilibrium Constant from Cell emf
Calculate the equilibrium constant K at 25°C for the following reaction from the standard emf.
Pb2+(aq) + Fe(s)
Pb(s) + Fe2+(aq)
Answer: E°cell = 0.28 V, K = 2.9 × 109
Alternate Example 20.12 Calculating the Cell emf for Nonstandard Conditions
A pH meter is constructed using hydrogen gas bubbling over an inert platinum electrode (the
hydrogen electrode) at a pressure of 1.2 atm. The other electrode is aluminum metal immersed
in a 0.20 M Al3+ solution. What is the cell emf when the hydrogen electrode is immersed in a
sample of acid rain with a pH of 4.0 at 25°C? If the electrode is placed in a sample of shampoo
solution and the emf is 1.17 V, what is the pH of the shampoo solution? The cell reaction is
2Al(s) + 6H+(aq)
2Al3+(aq) + 3H2(g)
Answer: emf = 1.43 V; pH = 8.4
Alternate Example 20.13 Predicting the Half-Reactions in an Aqueous Electrolysis
Describe what you expect to happen at the electrodes when an aqueous solution of sodium
iodide is electrolyzed.
Answer: H2O is reduced to H2 (in preference to the reduction of Na+ to Na); I– is oxidized
(in preference to the oxidation of H2O to O2).
Alternate Example 20.14 Calculating the Amount of Charge from the Amount of
Product in an Electrolysis
What electric charge is required to plate a piece of automobile molding with 1.00 g of
chromium metal using a chromium(III) ion solution? If the electrolysis current is 2.00 A, how
long does the plating take?
Answer: 5.57 × 103 C; 46.4 min
Alternate Example 20.15 Calculating the Amount of Product from the Amount of
Charge in an Electrolysis
A solution of nickel salt is electrolyzed to nickel metal by a current of 2.43 A. If this current
flows for 10.0 min, how many coulombs is this? How much nickel metal is deposited in the
electrolysis?
Answer: 1.46 × 103 C; 0.443 g
Copyright © Houghton Mifflin Company. All rights reserved.
114
PART VII
CHAPTER 21 Nuclear Chemistry
Alternate Example 21.1 Writing a Nuclear Equation
Radon-222 is a radioactive noble gas that is sometimes present as an air pollutant in homes
built over soil with high uranium content (uranium-238 decays to radium-226, which in turn
decays to radon-222). A radon-222 nucleus decays to polonium-218 by emitting an alpha
particle. Write the nuclear equation for this decay process.
Answer:
222
Rn
86
Po + 42He
→ 218
84
Alternate Example 21.2 Deducing a Product or Reactant in a Nuclear Equation
Iodine-131 is used in the diagnosis and treatment of thyroid cancer. This isotope decays by
beta emission. What is the product nucleus?
Answer:
131
Xe
54
Alternate Example 21.3 Predicting the Relative Stabilities of Nuclides
Predict which nucleus in each pair should be more stable and explain why: (a) astatine-210,
lead-207; (b) molybdenum-91, molybdenum-92; (c) calcium-37, calcium-42.
Answers: (a) Pb-207. It has an atomic number less than 83, whereas At has an atomic
number greater than 83. (b) Mo-92. It has a magic number of neutrons; Mo-91 does not. (c)
Ca-42. It lies within the band of stability; Ca-37 lies below the band of stability.
Alternate Example 21.4 Predicting the Type of Radioactive Decay
Thallium-201 is a radioactive isotope used in the diagnosis of circulatory impairment and
heart disease. How do you expect it to decay?
Answer: Positron emission or electron capture. (Electron capture is more likely because
thallium is a heavy element.)
Alternate Example 21.5 Using the Notation for a Bombardment Reaction
Sodium-22 is made by the bombardment of magnesium-24 (the most abundant isotope of
magnesium) by deuterons. An alpha particle is the other product. Write the abbreviated
notation for the nuclear reaction.
Answer:
24
Mg(d,
12
α)22
11
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
115
Alternate Example 21.6 Determining the Product Nucleus in a Nuclear
Bombardment Reaction
A neutron is produced when lithium-7 is bombarded with a proton. What product nucleus is
obtained in this reaction?
Answer: Be-7
Alternate Example 21.7 Calculating the Decay Constant from the Activity
The thorium-234 isotope decays by emitting a beta particle. A 50.0-µg sample of thorium-234
has an activity of 1.16 Ci. What is the decay constant for thorium-234?
Answer: 3.34 × 10–7/s
Alternate Example 21.8 Calculating the Half-Life from the Decay Constant
Thallium-201 is used in the diagnosis of heart disease. The isotope decays by electron capture;
the decay constant is 2.63 × 10–6/s. What is the half-life of this isotope in days?
Answer: 3.05 days
Alternate Example 21.9 Calculating the Decay Constant and Activity
from the Half-Life
Iodine-131 is used in the diagnosis and treatment of thyroid disorders. The half-life for the
beta decay of iodine-131 is 8.07 days. What is the decay constant (in /s)? What is the activity
in curies of a 1.0-µg sample of iodine-131?
Answer: 9.94 × 10–7/s; 0.12 Ci
Alternate Example 21.10 Determining the Fraction of Nuclei Remaining After a
Specified Time
A 0.500-g sample of iodine-131 is obtained by a hospital. How much will remain after a period
of one week? The half-life is 8.07 days.
Answer: 54.8%, or 0.274 g
Alternate Example 21.11 Applying the Carbon-14 Dating Method
A sample of wheat recovered from a cave was analyzed and gave 12.8 disintegrations of
carbon-14 per minute per gram of carbon. What is the age of the grain? Carbon from living
materials decays at a rate of 15.3 disintegrations per minute per gram of carbon. The half-life
of carbon-14 is 5730 y.
Answer: 1.48 × 103 y
Copyright © Houghton Mifflin Company. All rights reserved.
116
PART VII
Alternate Example 21.12 Calculating the Energy Change for a Nuclear Reaction
Consider the following nuclear reaction in which a lithium-7 nucleus is bombarded with a
hydrogen nucleus to produce two alpha particles:
7
Li
3
+ 11H → 242He
What is the energy change of this reaction per gram of lithium? The nuclear masses are
7.01436 amu; 11H, 1.00728 amu; 42He, 4.00150 amu.
7
Li,
3
Answer: –2.387 × 1011 J/g
CHAPTER 23 The Transition Elements
Alternate Example 23.1 Writing the IUPAC Name Given the Structural Formula of a
Coordination Compound
Give the IUPAC name of the coordination compound [Cu(CN)4(H2O)2]Cl2.
Answer: tetracyanodiaquacopper(II) chloride
Alternate Example 23.2 Writing the Structural Formula Given the IUPAC Name of a
Coordination Compound
What is the structural formula of hexaamminecobalt(II) tetrachloroaurate(III)?
Answer: [Co(NH3)6][AuCl4]2
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
117
Alternate Example 23.3 Deciding Whether Geometric Isomers Are Possible
Sketch the geometric isomers of dichlorodiammineplatinum(II) and dichlorotetraamminecobalt(III) ion.
Answer:
Alternate Example 23.4 Deciding Whether Optical Isomers Are Possible
Do any of the following have optical isomers? If so, describe the isomers. (a) transCo(NH3)2(en)23+ (b) cis-Co(NH3)2(en)23+
Answer: (b) only; the optical isomers are similar to those in Figure 23.16B in the text. (Note:
Models are very useful to illustrate the optical isomers.)
Alternate Example 23.5 Describing the Bonding in an Octahedral Complex Ion
(Crystal Field Theory)
Both Fe2+ and Co3+ have 3d6 configurations and form hexaammine complexes. However, the
iron(II) complex is paramagnetic, and the cobalt(III) complex is diamagnetic. Using crystal
field theory, obtain the number of unpaired electrons in each complex; also note whether each
complex is high-spin or low-spin.
Answer: Fe(NH3)42+, four unpaired electrons, high-spin; Co(NH3)43+, no unpaired electrons, low-spin
Copyright © Houghton Mifflin Company. All rights reserved.
118
PART VII
Alternate Example 23.6 Describing the Bonding in a Four-Coordinate Complex Ion
(Crystal Field Theory)
The qualitative analysis of nickel(II) ion is based on the reaction of the ion with the organic
compound dimethylglyoxime to form the chelate bis)dimethylglyoximato)nickel(II), a redcolored insoluble compound.
The lone pair on each nitrogen atom bonds to the nickel atom. The complex is diamagnetic.
Describe the distribution of d electrons in the nickel(II) complex bis(dimethylglyoximato)nickel(II). The complex has a square planar geometry.
Answer: The distribution of electrons is
Alternate Example 23.7 Predicting the Relative Wavelengths of Absorption of
Complex Ions
Earlier we described the square planar complex of nickel and dimethylglyoxime (Alternate
Example 23.6), noting that it has a red color. The text describes the bonding in the square planar
complex Ni(CN)42–; this complex ion has a yellow color. Imagine that the ligands in Ni(CN)42–
are exchanged for the dimethylglyoxime ligands. Is the color change from yellow to red in the
direction that you would expect? Note that the bonding of dimethylglyoxime to nickel is
through lone pairs on nitrogen atoms (similar to bonding in ammonia).
Answer: The bonding changes from strong (with CN– ligands) to weak (with lone pairs on
N atoms, as in NH3). The crystal field splitting becomes smaller, so the wavelength of the
transition (which gives rise to the color) becomes longer, which is in the direction expected.
Copyright © Houghton Mifflin Company. All rights reserved.
Alternate Examples for Lecture
119
CHAPTER 24 Organic Chemistry
Alternate Example 24.2 Predicting cis–trans Isomers
Consider each of the alkenes given in Alternate Example 24.3. Can either of them exist as
cis–trans isomers? If so, draw the structural formulas and label each as cis or trans.
Answers: (a) 3,4-dimethyl-3-hexene
(b) 3-ethyl-3-hexene; no geometric isomers
Alternate Example 24.3 Predicting the Major Product in an Addition Reaction
Water, HOH, can add across a double bond (in the presence of an acid). What would you
expect to be the major organic product when 1-pentene reacts with water in an addition
reaction?
Answer:
Alternate Example 24.4 Writing the IUPAC Name of an Alkane Given the Structural
Formula
Give the IUPAC name for each of the following:
Answers: (a) 2,3-dimethyl-4-t-butylheptane (b) 2-methylhexane
Copyright © Houghton Mifflin Company. All rights reserved.
120
PART VII
Alternate Example 24.5 Writing the Structural Formula of an Alkane Given the
IUPAC Name
Write the condensed structural formula of 2,3,5-trimethylhexane.
Answer:
Alternate Example 24.6 Writing the IUPAC Name of an Alkene Given the Structural
Formula
Name the alkenes whose structural formulas are given below.
Answers: (a) 3,4-dimethyl-3-hexene (b) 3-ethyl-3-hexene
Copyright © Houghton Mifflin Company. All rights reserved.
PART VIII
Brief Notes on Suggested Lecture Demonstrations
This part of the Instructor’s Resource Manual gives suggestions for lecture demonstrations
correlated with appropriate sections of the text. These include demonstrations that are
described in the text, perhaps in a figure. Although the brief descriptions given here may be
sufficient (see “Caution” note below), the following references also give descriptions of lecture
demonstrations, and you may find them helpful:
“Test Demonstrations in Chemistry,” J. Chem. Educ., Easton, Pa., 1962.
Hubert N. Alyea, “TOPS in General Chemistry,” J. Chem. Educ., Easton, Pa., 1967.
Bassam Z. Shakhashiri, Chemical Demonstrations, Vol. 1 (1983), Vol. 2 (1985), Vol. 3 (1989), and
Vol. 4 (1992), University of Wisconsin Press, Madison.
Lee R. Summerlin and James L. Ealy, Jr., Chemical Demonstrations, Vol. 1, American Chemical
Society, Washington, D.C., 1985.
Lee R. Summerlin, Christie L. Borgford, and Julie B. Ealy, Chemical Demonstrations, Vol. 2,
American Chemical Society, Washington, D.C., 1987.
CAUTION: These brief notes for suggested lecture demonstrations are intended for use
by professional chemists who understand the reactions and procedures involved. Because
these are brief notes, other references should be consulted for details in handling chemicals
and observing precautions to ensure the safety of students and demonstrator.
CHAPTER 1 Chemistry and Measurement
Section 1.3 Demonstration of the Conservation of Mass Using a
Magnesium Flash Bulb
Weigh a flash bulb, flash it, and reweigh after cooling. Note constancy of mass. Use digital
balance weighing to milligram accuracy. Have a student read the scale, putting the result on
the overhead or blackboard.
Section 1.4 Separation of a Mixture
Mix sodium chloride and silicon carbide or other water-insoluble material. Add water to
dissolve the NaCl and filter to remove silicon carbide.
Copyright © Houghton Mifflin Company. All rights reserved.
121
122
PART VIII
Section 1.4 Separation of Ink by Paper Chromatography
Spot ink about 1–2 cm from the bottom of a strip of filter paper. Then dip the end in 50%
ethanol to separate the dyes. When the dyes have separated, pass the sheet around for
inspection.
Sections 1.6 and 1.8 Comparison of Metric and Common U.S. Units
Compare the following side by side: 1 qt water with 1 L water, 1 lb salt with 0.5 kg salt, 1 oz
water with 30 mL water, yardstick with meterstick.
CHAPTER 2 Atoms, Molecules, and Ions
Opening: Reaction of Sodium and Chlorine
Melt or ignite a small piece of sodium (about 1 g) in a deflagration spoon and lower into a
bottle of chlorine gas. Contrast the characteristics of the reactants and products.
Section 2.2 Cathode Rays
Show a demonstration-model cathode-ray tube (similar to the one shown in Figure 2.4 on text
page 46); show how the rays are bent by a magnetic field. (A similar demonstration of an
electric field requires that the electric plates be inside the tube; they cannot be outside, because
of polarization of the glass.) Note that a paddle wheel is sometimes used to demonstrate the
momentum of electrons, but the motion of the wheel is actually the result of convection from
residual gas.
Section 2.6 Models of Molecules and NaCl Crystal
Compare various molecular models; discuss the structure of NaCl using a model of the crystal.
Show a reaction in terms of molecular models.
CHAPTER 3 Calculations with Chemical Formulas and Equations
Section 3.2 Mole of Substance
Show a mole of each of various substances (see Figure 3.2). Note the range of volumes. Later,
when discussing gases, comment on the difference between gases, which have the same molar
volume, and other substances.
Copyright © Houghton Mifflin Company. All rights reserved.
Brief Notes on Suggested Lecture Demonstrations
123
CHAPTER 4 Chemical Reactions: An Introduction
Section 4.1 Conductivity of Solutions
Test the electrical conductivity of various solutions using an apparatus like that described in
the text. The following are possibilities to test: distilled water, 0.1 M HCl, 0.1 M acetic acid,
6 M acetic acid, glacial acetic acid, HCl in benzene, 0.1 M KClO3, molten KClO3, tap water.
A device that can be used to illustrate conductivity consists of two bulbs, one a neon glow
bulb, the other a 7-watt night-light tungsten bulb wired through electrodes to the 110-volt line.
Only the glow bulb will light for weak electrolytes, but both will light for strong electrolytes.
Section 4.3 Precipitation
Add a solution of NaI to a solution of Pb(NO3)2 to show the formation of a precipitate. You
can use large volumes of solutions that will show up in a lecture room, or use small volumes
with a TOPS projector.
Section 4.5 Silver or Lead Tree (Displacement Reaction)
Bend copper wire in the shape of a tree with limbs and place in a solution of silver nitrate. The
wire grows crystals, giving the appearance of a tree with branches and leaves. Alternatively,
use zinc wire placed in lead acetate solution.
Section 4.5 Oxidation of Sugar by Potassium Chlorate
Carefully mix equal volumes of potassium chlorate and sugar (do not grind!). Place in a pile
on a square of asbestos and add a few drops of concentrated sulfuric acid. The mixture bursts
into violent flame.
Section 4.5 Oxidation of Glycerol by Potassium Permanganate
Place a pile of potassium permanganate crystals on a square of asbestos. Pour a small quantity
of glycerol over the crystals. Within a few seconds, the mixture ignites.
Section 4.5 Hydrogen Peroxide—Oxidizing and Reducing Properties
Add 3% H2O2 to dilute KI to which some sulfuric acid has been added. Iodide ion is oxidized
in acid solution by hydrogen peroxide to iodine. The iodine forms a blue complex with starch.
Add 3% H2O2 to dilute KMnO4 to which some sulfuric acid has been added. The purple
color of permanganate ion fades as the ion is reduced by hydrogen peroxide in acid solution.
Section 4.7 Ammonia Fountain
Fill a dry flask from a cylinder of ammonia, holding the flask upside-down when filling so
that the ammonia will displace air. Press the dropper to push some water into the flask
containing the ammonia to start the fountain. The demonstration is interesting and can be
used to introduce some properties of an important reagent.
Copyright © Houghton Mifflin Company. All rights reserved.
124
PART VIII
CHAPTER 5 The Gaseous State
Section 5.1 Effect of Reducing the Pressure on a Column of Mercury
Insert a closed-end barometer tube into one hole of a two-holed stopper and a glass tube
connected to rubber tubing into the other hole. Fill the barometer tube with mercury and place
the stopper and tube ends into a wide-mouth bottle containing mercury. Attach the rubber
tube to an aspirator or vacuum pump. Comment on the change of mercury column height as
pressure is reduced.
Section 5.2 Cartesian Diver
Fill a large bottle with water and place an inverted vial containing air into the water in the
neck of the bottle. Fit a stopper into the neck. When you press the stopper, the air in the vial
is compressed (water is not) and the vial sinks. Contrast compressibility of gases with the
relative compressibility of liquids. See TOPS manual, page 74, for a description of a TOPS
demonstration.
Section 5.2 Effect of Temperature Change on Gas Volume
Fill a balloon with helium. Submerge the balloon in liquid nitrogen until it contracts to a small
volume. When the balloon is thrown toward the audience, it will expand, hover for a period
of time, then rise. Pour the excess liquid nitrogen on the floor for a dramatic ending.
Section 5.2 Charles’s Law
Fit a flask with a one-hole stopper through which a short length of glass tubing passes. Heat
the flask over a water bath (note the bath temperature and the air temperature). Remove the
flask from the water bath and quickly immerse upside-down in a large beaker of water at
room temperature (add food coloring to the water for visibility). As the air in the flask cools,
water enters the flask. Adjust the pressure in the flask to atmospheric pressure by making the
levels of water inside and outside the flask equal. Remove the flask from the beaker without
allowing more water to enter. Measure the volume of water in the flask, then measure the
volume of the flask. Confirm Charles’s law from the change in volume of air in the flask.
Section 5.2 Molar Volume of a Gas
Display a cube that has a volume of 22.4 L.
CHAPTER 6 Thermochemistry
Section 6.2 An Endothermic Reaction
Place about 0.1 mol of barium hydroxide octahydrate crystals in a 250-mL Erlenmeyer flask.
Also have ready 0.2 mol of ammonium nitrate (or ammonium thiocyanate) powder in a test
Copyright © Houghton Mifflin Company. All rights reserved.
Brief Notes on Suggested Lecture Demonstrations
125
tube. Add water to a small, smooth board to form a puddle. Then add the ammonium salt to
the flask containing the barium hydroxide and stopper tightly to contain the ammonia fumes.
A slurry will form soon after the solids are mixed. Place the flask in the puddle of water on
the board. Talk for about two minutes, giving the flask time to freeze to the board. Now move
the board from side to side to show that a solution has been formed; then carefully turn the
board upside-down to show that the flask has frozen to it. See Figure 6.1.
Section 6.2 An Exothermic Reaction (Rusting of Iron)
Wash steel wool in dilute hydrochloric acid or acetic acid. Rinse well in water. Wrap the steel
wool around the bulb of a thermometer or around a projection thermocouple. The temperature
rises as the iron rusts.
CHAPTER 7 Quantum Theory of the Atom
Section 7.3 Atomic Line Spectra
Provide students with diffraction gratings in slide mounts (available from Edmund Scientific
Company). Have the students observe various atomic line spectra from discharge tubes (lines
are to the right, as you look at the grating), as well as from a continuous source, such as a
tungsten bulb.
CHAPTER 8 Electron Configurations and Periodicity
Section 8.7 Reactivity of Some Metallic Elements
Clean a strip of magnesium ribbon and a piece of aluminum wire with steel wool. Place each
in some water containing several drops of phenolphthalein. Also place small pieces of calcium,
then sodium, and finally potassium into water containing phenolphthalein. Note the relative
rates of reaction.
CHAPTER 9 Ionic and Covalent Bonding
Section 9.2 Color of Ions in Aqueous Solution
Place aqueous solutions of ions in flat dishes, as in Figure 9.6. Project with overhead.
Copyright © Houghton Mifflin Company. All rights reserved.
126
PART VIII
CHAPTER 10 Molecular Geometry and Chemical Bonding Theory
Section 10.1 Arrangements of Electron Pairs
Tie two to six balloons of about the same size to show the different arrangements. See Figure
10.3.
Section 10.1 Molecular Shapes
Show lecture-size models of molecules with various geometries.
Section 10.6 Paramagnetism of Oxygen
To prepare liquid oxygen, place a tall test tube in a Dewar flask containing liquid nitrogen.
Pass oxygen gas through a glass tube into the test tube. Blue liquid oxygen will form in the
test tube.
Pour liquid nitrogen over the poles of a strong magnet to cool it. Note that nitrogen does
not stick to the magnet poles. Now pour liquid oxygen over the poles; note that the oxygen
does stick to the magnet poles. See Figure 10.29.
CHAPTER 11 States of Matter; Liquids and Solids
Section 11.2 Sublimation of Iodine
Place iodine crystals in a beaker and cover with watch glass or evaporating dish containing
ice. Heat with a low flame. Iodine will sublime and collect on the underside of the watch glass
or dish. See Figure 11.3.
CHAPTER 12 Solutions
Section 12.2 Supersaturated Solutions
Fill a flask three-fourths full with sodium thiosulfate pentahydrate or sodium acetate trihydrate crystals. Add just enough water to dampen the crystals. Cover and heat slowly until a
solution forms without any solid (you may need to add some water). Allow it to cool slowly
to give the supersaturated solution. If you have difficulty keeping the solution from crystallizing prematurely, add a small quantity of water and reheat to give a solution. When ready,
seed with a crystal of the solute. Crystallization is dramatic (see Figure 12.4). Have students
note how warm the resulting solution is. The solid mixture can be reheated to repeat the
demonstration.
Copyright © Houghton Mifflin Company. All rights reserved.
Brief Notes on Suggested Lecture Demonstrations
127
Section 12.3 Effect of Temperature Change on Solubility
Potassium nitrate, ammonium nitrate, or boric acid may be used to demonstrate an increase
in solubility with increasing temperature. Add more than the required amount of salt to
saturate the solution at room temperature. Then show that heating will dissolve more salt.
Ceric sulfate, calcium hydroxide, or calcium acetate may be used to demonstrate a decrease
in solubility with temperature. Add more than the required amount to saturate a hot solution.
Then show that more dissolves on cooling.
Section 12.7 Osmosis
Cover the wide end of a thistle-tube funnel with a semipermeable membrane. Add a sugar
syrup (to which a food dye has been added for visibility) to the tube end of the funnel. Immerse
the wide end in distilled water. Note rise in height of liquid after a half-hour. See Figure 12.24.
Section 12.9 Colloids
The following are some colloids that can be used to demonstrate filterability and the Tyndall
effect:
1.
2.
3.
Gelatin: Prepare a 2% solution by dissolving gelatin in boiling water.
Colloidal sulfur: Saturate a half liter of cold water with SO2, then pass H2S through the
solution for several minutes. Colloidal sulfur forms.
Colloidal arsenic sulfide: Add about 1 g As2O3 to a liter of water and bring to a boil.
Pass H2S into the hot solution for several minutes. Colloidal As2S3 forms.
Compare a true solution with a colloidal solution. Show that both pass through a filter, but
only the colloid gives the Tyndall effect. A laser pointer is a convenient light source for the
Tyndall effect.
CHAPTER 14 Rates of Reaction
Section 14.3 Iodine Clock Reaction
The essential reaction involves the oxidation of iodide ion by peroxydisulfate ion:
2I− + S2O82− → I2 + 2SO42−
This reaction is slow. The I2 reacts quickly with thiosulfate ion in the solution. When thiosulfate
ion is used up, the concentration of I2 increases and gives a blue color with starch indicator.
Prepare a solution containing 200 mL 0.2 M KI, 100 mL 0.005 M Na2S2O3, and 1 mL 1% starch
indicator. Add to 100 mL (NH4)2S2O8. The concentrations may be varied to change the time
before the blue color appears.
Copyright © Houghton Mifflin Company. All rights reserved.
128
PART VIII
CHAPTER 15 Chemical Equilibrium
Section 15.7 Le Chatelier’s Principle: Iron Thiocyanate Complex
Mix small quantities of iron(III) nitrate and potassium thiocyanate in about a liter of water to
give a dilute, orange-yellow solution of Fe(CNS)2+. Apportion the solution among three
beakers. Add Fe3+ to one beaker and CNS– to another to shift the equilibrium. Note the deep
red color obtained in both cases, which results from the formation of more Fe(CNS)2+, as
predicted by Le Chatelier’s principle. See also Figure 15.8 for another demonstration.
Section 15.8 Effect of Changing the Temperature: NO2–N2O4 Equilibrium
Use three sealed tubes containing a small amount of nitrogen dioxide gas (tubes are commercially available). Place one in an ice bath and another in boiling water, leaving the third one
at room temperature. Colorless N2O4 is stabler at lower temperatures and reddish-brown NO2
at higher temperatures. Note that conversion of the tetroxide to the dioxide is an endothermic
process.
Section 15.9 Ostwald Process for Preparing Nitric Acid (Platinum Catalysis)
A small coil of platinum wire or platinum foil is affixed to a glass rod that passes through a
three-hole rubber stopper. A glass tube also passes through the stopper and opens at its lower
end near the platinum. The other end is connected to a source of oxygen. When the rubberstopper assembly is placed in a flask containing concentrated ammonia, the platinum wire
should be about a centimeter above the solution. Before putting the rubber stopper in the flask,
heat the platinum. When the stopper assembly is placed in the flask, the platinum will continue
to glow for several minutes from the exothermic reaction of NH3 and O2 to produce NO. Note
that the use of a copper wire instead of platinum yields N2 instead of NO.
CHAPTER 16 Acids and Bases
Section 16.4 Relative Acid and Base Strengths
See Bassam Z. Shakhashiri, Chemical Demonstrations, Vol. 3 (Madison: University of Wisconsin Press, 1989), no. 8.25, pp. 158–161.
Section 16.8 Acid–Base Indicators
Add different indicators to solutions previously made up with various pH values. Color
changes can be shown as acid and base are added. See Figures 16.10 and 16.11 in the text.
Copyright © Houghton Mifflin Company. All rights reserved.
Brief Notes on Suggested Lecture Demonstrations
129
CHAPTER 17 Acid–Base Equilibria
Section 17.4 Hydrolysis of Salts
Measure the pH of a number of salt solutions, using either a pH meter or acid–base indicators.
Have a student read the pH meter.
Section 17.6 Effect of Adding Acid or Base to a Buffer
Prepare a buffer solution from equal volumes of 1 M acetic acid and 1 M sodium acetate (pH
of buffer is 4.7). Add strong base (or strong acid) from a buret to a certain volume of water
and watch the change of pH. Repeat, but with the same volume of buffer. Compare the results.
CHAPTER 18 Solubility and Complex-Ion Equilibria
Section 18.5 Amphoteric Hydroxides
Place a solution of zinc chloride in one beaker and a solution of sodium hydroxide in another.
Pour some of the sodium hydroxide solution into the zinc chloride solution and note the
formation of a white precipitate of zinc hydroxide. Then add more sodium hydroxide until
the precipitate dissolves. See Figure 18.8.
Section 18.6 Solubility of Silver Salts and Complex-Ion Formation
Prepare 0.1 M solutions of the following compounds: AgNO3, Na2CO3, NaOH, NaCl, NaBr,
Na2S2O3, NaI, NaCN, and Na2S. Also have available 6 M NH3. To the AgNO3 solution, add
the following solutions in order: Na2CO3 (gives pale yellow precipitate of Ag2CO3), NaOH
(gives brown precipitate of Ag2O), NaCl (gives white precipitate of AgCl), NH3 (precipitate
dissolves to give silver ammine complex ion), NaBr (gives pale yellow precipitate of AgBr),
Na2S2O3 (dissolves precipitate to form thiosulfate complex ion), NaI (forms yellow precipitate
of AgI), NaCN (precipitate dissolves to give dicyanoargentate ion), and Na2S (forms black
precipitate of Ag2S).
CHAPTER 20 Electrochemistry
Section 20.2 Voltaic Cells
Place a metal electrode in a beaker containing a solution of the metal ion. Couple this half-cell
with a similar half-cell of another metal using a salt bridge consisting of filter paper soaked
in saturated KCl solution. Measure the emf of the voltaic cell with a voltmeter.
Copyright © Houghton Mifflin Company. All rights reserved.
130
PART VIII
Section 20.8 Lead Storage Cell
Hang two strips of lead foil over the edge of a beaker filled with 0.1 M sulfuric acid. Attach
clips to the lead electrodes and attach the wire leads to a 7.5-V battery or power supply. Note
the evolution of gas during the charging of the cell. Now attach the lead cell to a bell or 1.5-V
bulb to demonstrate that the cell has been charged. (Lead sulfate first forms on the lead strips;
during charging at the positive plate, PbSO4 + 2H2O forms PbO2 + 4H+ + SO42−; at the negative
plate, PbSO4 gives Pb + SO42−.)
Section 20.10 Electrolysis of Water
Fill a Hoffman apparatus with 0.1 M sulfuric acid and operate at 22 V. Oxygen dissolves more
readily than hydrogen, so the volume ratio will not be exactly 2 to 1 unless that apparatus has
been operated previously to saturate the solution with the gases.
Section 20.11 Electrolysis of Copper Sulfate
Rinse several square centimeters of copper gauze (to be used as the cathode) in distilled water,
dry, and weigh to nearest 0.01 g. Use a strip of copper as the anode. Immerse electrodes in a
solution made from 1 L water, 200 g CuSO4, and 80 g concentrated H2SO4. Connect in series
to resistance and ammeter; electrolyze for 30 min at 0.25 A. Rinse cathode and weigh. Compare
with calculated value.
CHAPTER 21 Nuclear Chemistry
Section 21.3 Cloud Chamber
Construct a cloud chamber from a screw-top jar. Glue pieces of felt on the bottom of the jar
and the top of the lid. Saturate both pieces of felt with methanol and screw on the lid. Invert
the jar with the lid on a block of dry ice. Wait about 15 min. Shine a spotlight through the side
of the jar. Cloud tracks from cosmic rays or ambient radioactivity will be seen. A gamma-ray
source will produce many tracks.
Section 21.3 Detection and Absorption of Beta Rays
Use uranyl nitrate as a beta-ray source and detect with a Geiger counter. Vary the position of
the source and note decrease in counts with distance (inverse square law). Sheets of paper and
metal may be used to test for absorption of beta rays.
Copyright © Houghton Mifflin Company. All rights reserved.
Brief Notes on Suggested Lecture Demonstrations
131
CHAPTER 22 Chemistry of the Main-Group Elements
Section 22.2 Properties of Sodium Metal
Slice off a piece of sodium metal to demonstrate the softness of the metal and its silvery
appearance (see Figure 22.2). Show malleability by flattening the piece with the side of the
knife. Put a piece of the metal in water to demonstrate its chemical reactivity. (It is advisable
to use a plastic shield to protect the audience.)
Section 22.2 Solvay Process
Saturate concentrated ammonia solution with sodium chloride contained in a beaker. Add
pieces of dry ice. Sodium hydrogen carbonate will precipitate. See Figure 22.8.
Section 22.3 Burning of Magnesium in Air, H2O, and CO2
Magnesium metal burns in air to give a mixture of the oxide and the nitride. In water vapor
(steam), magnesium burns to give MgO and H2. In CO2, the metal gives MgO and C.
Demonstrate the burning in air; then show that the metal continues to burn when placed in
the vapor over boiling water. Show that a match flame is extinguished when inserted in a
beaker containing dry ice (or pour carbon dioxide gas over the flame), but magnesium will
continue to burn.
Section 22.4 Reaction of Aluminum with Acid and Base
Demonstrate the reactions of aluminum metal with hydrochloric acid and with sodium
hydroxide solution. Note the evolution of gas (hydrogen) in both cases. You can place beaker
on overhead to project.
Section 22.7 Burning of Phosphorus
Prepare a solution containing 1 g of white phosphorus in about 10 mL of carbon disulfide.
(Use the solution carefully and do not store!) Place several drops of the solution on some paper
on a square of asbestos. The paper will ignite as soon as the solvent evaporates.
Section 22.8 Preparation of Oxygen
Add water to sodium peroxide in a test tube or flask. Test the evolution of oxygen with a
smoldering wood splint. As an alternative preparation, heat a mixture of potassium chlorate
with a small amount of manganese dioxide. (CAUTION: Avoid organic contaminants!)
Section 22.8 Dehydrating Action of Concentrated Sulfuric Acid
Place a pile of sugar in a beaker, moisten slightly with water, and pour concentrated sulfuric
acid on to the pile. A column of porous carbon forms. (A hood is advisable for this demonstration. Otherwise, you might consider showing a video; see Video series A and C.)
Copyright © Houghton Mifflin Company. All rights reserved.
132
PART VIII
CHAPTER 23 The Transition Elements
Section 23.1 Oxidation States of Vanadium
Prepare a solution that is 1 M sodium vanadate (or vanadyl sulfate) in 1 M sulfuric acid. Pour
through a Jones reductor to give vanadium(II) sulfate solution. Fill lower half of a cylinder
with this solution. Then carefully pour in 0.01 M potassium permanganate and let stand.
Layers illustrating four oxidation states of vanadium will form: violet vanadous (+2), emerald
green vanadic (+3), deep blue vanadyl (+4), and yellow vanadate (+5). See Figure 23.2.
CHAPTER 24 Organic Chemistry
Section 24.3 Preparation of Acetylene
Drop several pieces of calcium carbide in a beaker containing water. Ignite with a match on
the end of a long stick. (Use a clear explosion shield!) The acetylene ignites with a pop. See
Figure 24.10 for another demonstration.
Section 24.6 Silver-Mirror Test for Aldehydes
Dissolve about 10 g of silver nitrate in 0.5 L of water. Add several drops of sodium hydroxide
solution. Then add concentrated ammonia until the precipitate that first forms is just dissolved. Do not add excess ammonia. Pour the solution into a very clean flask and add some
formaldehyde solution or acetaldehyde. Note the formation of a silver mirror. Dilute the
solutions and dispose of them promptly after use.
CHAPTER 25 Polymer Materials: Synthetic and Biological
Section 25.1 Preparation of Nylon
Carefully pour a solution containing 60 g of hexamethylene diamine per liter of water over a
solution containing 60 mL of sebacoyl chloride (or adipoyl chloride) per liter of hexane to form
two layers. A film of nylon forms at the interface of the layers. Grab this film with forceps and
pull upward to form a filament. Add a dye to one solution to increase visibility. Wash filament
well with water to remove hydrochloric acid if it is to be passed around for inspection. See
Figure 25.4.
Copyright © Houghton Mifflin Company. All rights reserved.