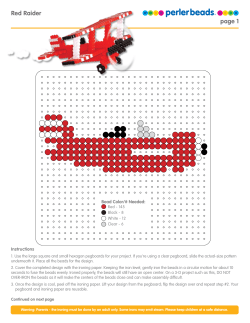
—A review Supersonic biplane Kazuhiro Kusunose , Kisa Matsushima
Progress in Aerospace Sciences 47 (2011) 53–87 Contents lists available at ScienceDirect Progress in Aerospace Sciences journal homepage: www.elsevier.com/locate/paerosci Supersonic biplane—A review Kazuhiro Kusunose a, Kisa Matsushima b,n, Daigo Maruyama c a b c JAXA, Japan University of Toyama, Japan ONERA, France a r t i c l e i n f o abstract Available online 19 November 2010 One of the fundamental problems preventing commercial transport aircraft from supersonic flight is the generation of strong sonic booms. Sonic booms are the ground-level manifestation of shock waves created by airplanes flying at supersonic speeds. The strength of the shock waves generated by an aircraft flying at supersonic speed is a direct function of both the aircraft’s weight and its occupying volume; it has been very difficult to sufficiently reduce the shock waves generated by the heavier and larger conventional supersonic transport (SST) configuration to meet acceptable at-ground sonic-boom levels. It is our dream to develop a quiet SST aircraft that can carry more than 100 passengers while meeting acceptable at-ground sonic-boom levels. We have started a supersonic-biplane project at Tohoku University since 2004. We meet the challenge of quiet SST flight by extending the classic two-dimensional (2-D) Busemann biplane concept to a 3-D supersonic-biplane wing that effectively reduces the shock waves generated by the aircraft. A lifted airfoil at supersonic speeds, in general, generates shock waves (therefore, wave drag) through two fundamentally different mechanisms. One is due to the airfoil’s lift, and the other is due to its thickness. Multi-airfoil configurations can reduce wave drag by redistributing the system’s total lift among the individual airfoil elements, knowing that wave drag of an airfoil element is proportional to the square of its lift. Likewise, the wave drag due to airfoil thickness can also be nearly eliminated using the Busemann biplane concept, which promotes favorable wave interactions between two neighboring airfoil elements. One of the main objectives of our supersonic-biplane study is, with the help of modern computational fluid dynamics (CFD) tools, to find biplane configurations that simultaneously exhibit both traits. We first re-analyzed using CFD tools, the classic Busemann biplane configurations to understand its basic wave-cancellation concept. We then designed a 2-D supersonic biplane that exhibits both wave-reduction and cancellation effects simultaneously, utilizing an inverse-design method. The designed supersonic biplane not only showed the desired aerodynamic characteristics at its design condition but also outperformed a zero-thickness flat-plate airfoil. (Zero-thickness flat-plate airfoils are known as the most efficient monoplane airfoil at supersonic speeds.) Also discussed in this paper is how to design 2-D biplanes, not only at their design Mach numbers but also at off-design conditions. Supersonic biplanes have unacceptable characteristics at their off-design conditions such as flow choking and its related hysteresis problems. Flow choking causes rapid increase of wave drag and it continues to be kept up to the Mach numbers greater the cruise (design) Mach numbers due to its hysteresis. Some wing devices such as slats and flaps, which could be used at take-off and landing conditions as high-lift devices, were utilized to overcome these off-design problems. Then supersonic-biplane airfoils were extended to 3-D wings. Because that rectangular-shaped 3-D biplane wings showed undesirable aerodynamic characteristics at their wingtips, a tapered-wing planform was chosen for the study. A 3-D biplane wing having a taper ratio and aspect ratio of 0.25 and 5.12, respectively, was designed utilizing the inverse-design method. Aerodynamic characteristics of the designed biplane wing were further improved by using winglets at its wingtips. Flow choking and its hysteresis problems, however, occurred at their off-design conditions. It was shown that these off-design problems could also be resolved by utilizing slats and flaps. Finally, a study on the aerodynamic characteristics of wing–body configurations was conducted using the tapered biplane wing. In this study a body was chosen in order to generate strong shock waves at its nose region. Preliminary parametric studies on the interference effects between the body and the tapered biplane wing were performed by choosing several different wing locations on the body. From this study, it can be concluded that the aerodynamic characteristics of the tapered biplane wing are minimally affected by the disturbances generated from the body, and that the biplane wing shows promise for quiet commercial supersonic transport. & 2010 Elsevier Ltd. All rights reserved. n Corresponding author. Tel./fax: + 81 76 445 6796. E-mail addresses: [email protected], [email protected] (K. Matsushima). 0376-0421/$ - see front matter & 2010 Elsevier Ltd. All rights reserved. doi:10.1016/j.paerosci.2010.09.003 54 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Contents 1. 2. 3. 4. 5. 6. 7. 8. Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 54 History . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 56 2.1. Origin of the Busemann biplane . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 56 2.2. Continuing research on the Busemann biplane . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 56 2.3. Descendants and derivatives of the biplane concept . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 57 2-D theory and construction of biplane airfoils . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 57 3.1. Basic theory . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 57 3.1.1. Wave-reduction effect . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 57 3.1.2. Wave-cancellation effect (Busemann biplane) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 58 3.1.3. An ideal biplane configuration, the Licher biplane . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 58 3.2. CFD analysis . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 59 3.2.1. Analyses using unstructured grid . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 59 3.2.2. Hysteresis analysis . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 60 Design for biplane airfoil of better performance . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 63 4.1. Inverse design . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 63 4.1.1. Pressure and geometry relation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 63 4.1.2. Basic equation and procedure for the inverse-problem design . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 64 4.2. Inverse design for biplane airfoil at a Mach number of 1.7 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 64 4.2.1. Design process . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 64 4.2.2. General features of the designed airfoil . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 65 4.3. Drag polar diagrams . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 67 3-D extension of biplane airfoils . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 67 5.1. Busemann biplane wing with rectangular planform . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 67 5.2. Planform parametric study . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 68 5.2.1. Effect of changing sweep angles . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 69 5.2.2. Effect by changing taper ratios . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 69 5.3. Introduction of winglet . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 69 5.4. Design for better-performance biplane wing . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 70 5.4.1. Application of the 2-D designed airfoil . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 70 5.4.2. 3-D inverse design . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 70 Wing–fuselage interference. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 73 6.1. Wing–fuselage configuration. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 73 6.2. Aerodynamic performance at cruise condition. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 74 6.3. Application of designed wing to wing–fuselage combination . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 77 Experiment. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 80 Conclusions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 82 Acknowledgments . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 83 A.1. Aerodynamic center . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 83 A.1.1. Biplane and diamond airfoils. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 83 A.1.2. Relation between an A.C. location and load distributions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 84 References . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 86 1. Introduction Beginning with the first flight achieved by the Wright brothers in 1903, the past 100 years of aviation history have been full of remarkable milestones. In 1947, the Bell X-1 experimental aircraft broke the sound barrier, ushering in the era of supersonic flight. In military aviation, airplane speed records have been continuously broken and topped; one of NASA’s experimental aircraft recently flew at Mach-10 speeds. Commercial aviation, however, has not enjoyed as many advances in supersonic flight; Concorde (1969–2003) was the first but also the last supersonic transport aircraft ever built. Concorde’s supersonic flights were, unfortunately, terminated, due to its poor fuel efficiency and unacceptable at-ground noise level. A fundamental problem preventing commercial transport aircraft from supersonic flight is the creation of strong shock waves, whose effects are felt on the ground in the form of sonic booms. Because the strength of the shock waves generated by an aircraft flying at supersonic speed is a direct function of both the aircraft’s weight and its occupying volume, it has been deemed nearly impossible to sufficiently reduce the shock waves generated by the heavier and larger conventional commercial aircraft to meet acceptable at-ground sonic-boom levels. In this paper, we focus on supersonic biplanes proposed by a group at Tohoku University [1–6]. They tried to extend the classic Busemann biplane concept [7–10] to develop a practical supersonic-biplane wing that will generate sufficient lift at supersonic flights without increasing a severe wave-drag penalty. In general, a lifted airfoil generates shock waves through two fundamentally different mechanisms: one is due to its lift and the other is due to its thickness. The wave drag due to lift cannot be eliminated completely; it can only be reduced through multi-airfoil configurations. Based on the 2-D supersonic thin-airfoil theory [8] wave drag of an airfoil is proportional to the square of its lift. Multiairfoil configurations redistribute the system’s total lift among the individual airfoil elements, reducing the lift of each of the individual elements and, therefore, the total wave drag of the system. This will be referred to as the ‘‘wave-reduction effect’’ in the rest of this paper [6]. Likewise, wave drag due to airfoil thickness can also be nearly eliminated using the Busemann biplane concept, which promotes favorable wave interactions between the two neighboring airfoil elements. By choosing their geometries and their relative locations strategically, the waves generated by the two elements cancel each other (the ‘‘wave-cancellation effect’’ [6]). However, it is important to remember that skin friction of the biplane system will increase because of the increased surface area. One of the main objectives of our supersonic-biplane study is, with the help of modern CFD tools, K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Nomenclature Symbols A An A1, Ai A2, At Ain AR b c, C cmid cref croot ctip c1, c2 Cd Cdp Cdf Cdfric Cdtotal CD Cl CL Cp D f, g f(x) F FU, FL G, h h l L l/d L/D cross sectional area cross sectional area at the throat of nozzle section area of inlet section area of throat cross sectional area at the inlet of nozzle aspect ratio semi-span length chord length position of the mid-chord at the wingtip of the threedimensional wing reference chord length chord at the wing root of the three-dimensional wing chord at the wingtip of the three-dimensional wing Busemann coefficients wave-drag coefficient of airfoil (wing section) pressure drag coefficient of the two-dimensional airfoil in Navier–Stokes simulations friction drag coefficient of the two-dimensional airfoil in Navier–Stokes simulations friction drag coefficient total drag coefficient in Navier–Stokes simulations drag coefficient lift coefficient (wing section) lift coefficient pressure coefficient wave drag function airfoil geometry pressure force acting on airfoil pressure forces acting on upper and lower elements of biplane gap between biplane elements gap between two elements of the biplane surface length defined along the airfoil surface lift lift-to-drag ratio of the two-dimensional airfoil (wing section) lift-to-drag ratio to obtain 2-D and 3-D biplane configurations that simultaneously exhibit both traits. Kusunose and his group at Tohoku University in 2004 began to study supersonic biplanes for the next generation supersonic transport by utilizing CFD tools (Navier–Stokes codes, but mainly in their inviscid Euler modes) [11–13]. In this paper we introduce a brief history of the classic Busemann biplane and its related researches, followed by a discussion on the fundamental theory of supersonic biplane. In general, supersonic biplanes show superior aerodynamic characteristics at their design Mach numbers. However, they have poor performances at their off-design conditions. Flow choking occurs at high subsonic speeds [9,14], and continues to Mach numbers greater than the design Mach number in the acceleration stage due to flow hysteresis [14,15]. Since the internal flow of a biplane is identical to that of an intake diffuser, the characteristics of choking and hysteresis of supersonic biplanes can be analyzed based on such characteristics of a supersonic diffuser under start and un-start conditions [16]. M Msw Mt MN MN P P0, PN DP q r, y, xm R Re Ds S t UN w wlt x, y, z x y z X, Y, Z xi xw z a b, b0 g d e y y m r 55 Mach number swallowing Mach number Mach number behind oblique shock wave free-stream Mach number inlet Mach number static pressure total and free-stream pressures pressure jump due to local flow inclination dynamic pressure parameters of skewed cylindrical coordinate system gas constant Reynolds number entropy production wing reference area airfoil thickness free-stream velocity span width of biplane winglet (wingtip panel) parameters of the Cartesian coordinate system streamwise coordinate span-wise coordinate vertical coordinate body coordinate system incident point from the leading edge the location of wing for wing–fuselage configurations gap between biplane elements angle of attack shock-wave angle ratio of specific heats margin wedge angle df/dx-a reflection angle Mach angle fluid density Subscripts i t N inlet throat free-stream We then proceed with an overview of important progress made by Kusunose’s group on 2-D and 3-D supersonic biplanes. It will be discussed how to design supersonic biplanes, not only at their design Mach numbers but also at their off-design conditions. At their design Mach numbers, 2-D and 3-D supersonic biplanes that exhibit both wave-reduction and wave-cancellation effects simultaneously are designed using an inverse-design method [3,6,17,18]. At offdesign conditions hinged slats and flaps are applied to avoid flow choking and accompanying hysteresis problems [6,17–22]. These slats and flaps can be used as high-lift devices at take-off and landing conditions. Several studies on sonic-boom propagation mechanisms through the atmosphere are given in Ref. [23,24]. Aerodynamic studies on wing planforms and wing–body configurations are also conducted using a tapered biplane wing [25–30]. Preliminary parametric studies on the interference effect between the body and biplane wing are performed by choosing several different wing locations on the body [31,32]. We finally make a brief review on recent experimental investigations closely related to current biplane developments [33–39]. 56 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 2. History HIGH-SPEED AERONAUTICS 2.1. Origin of the Busemann biplane Today, one can learn about the Busemann biplane only in a traditional textbook. For example, in Liepmann and Roshko [8], it is introduced as one of the wave-drag reduction methods utilizing interference between its two element wings. The interference is simply explained in Fig. 1, which is extracted from Ref. [8]. The first proposal of the supersonic-biplane concept was made by Dr. Adolf Busemann at the Fifth Volta Congress in Rome in 1935. Fig. 2 shows a picture of the congress where many famous aerodynamicists, including A. Busemann himself, are identified [40]. This 1935 Volta Congress is regarded as the threshold of modern high-speed aerodynamics. The main topic was ‘‘High Velocity in Aviation,’’ with the President being G.A. Crocco. Here, Busemann presented a paper titled ‘‘Aerodynamic lift at supersonic speed’’ [7] in which he mostly discussed the concept of the swept wing. In the supplement of the conference proceedings, Busemann indicated how to cancel wave drag to make a wing equal to a flat plane by means of biplane configuration. The article was Wave-cancellation Pressure distribution on inside surface Fig. 3. Busemann biplane proposed in (Ref. [41]). written in German. Its title is ‘‘Auftribe des Doppeldeckers bei Uebershallgeschwindigkeit’’, which means ‘‘Investigation of Biplanes in Supersonic flows’’. Later, in 1955 in Ref. [41], he presented the figure of the 1935 biplane again, which is shown in Fig. 3. He introduced it as the biplane of zero wave drag and noise that he had demonstrated at the Volta Congress in 1935. 2.2. Continuing research on the Busemann biplane Wave reflection Busemann biplane Figure 1 of Ref. [41] titled “Biplane of zero drag and noise”. Busemann biplane Off-design Fig. 1. Simple description for mutual cancellation of waves on a biplane (Ref. [8]). C.Wieselsberger A. Busemann G.A.Crocco L.Prandtl Fig. 2. The Fifth Volta Congress (Ref. [40]). By the courtesy of http://www.dglr.de/ literatur/publikationen/pfeilfluegel/Kapitel1.pdf After the Fifth Volta Congress, several researchers were dedicated to the study of a supersonic biplane. Until 1958, studies on Busemann-type biplanes appeared in many papers. Almost all of the studies were two dimensional because of the lack of computational power and refined experimental strategy when compared with the technology of the present day. V.O. Walcher carried out a parametric study regarding the leading and trailing edge (L.E. and T.E.) angles of each upper and lower element of a biplane [42]. In 1944, M.J. Lighthill discussed the advantages and disadvantages of the Busemann biplane. As a disadvantage, he pointed out that the biplane had a greater wing area than a monoplane, which increased skin-friction drag, and that the passage between the two elements might ‘‘choke’’ [43]. In the same year, wind-tunnel experiments were conducted by A. Ferri in Italy [14]. These were done for both non-lifting and lifting cases. Ferri measured aerodynamic forces and took Schlieren photographs to observe the viscous effect of the boundary layer and shock movement phenomena. He observed undesirable ‘‘choke’’ and ‘‘hysteresis’’ occurrences, both of which will be discussed later in this paper. In spite of the disadvantages, Ferri concluded that the biplane was promising to obtain better efficiency than that of an isolated wing. In a 1947 NACA paper published in the United States, W.E. Moeckel performed a detailed parametric study on the spacing of the gap and the L.E. and T.E. angles of the two elements of the biplane for lifting cases. One of his results was to find that unsymmetrical biplanes whose lower elements were thicker than upper elements would have higher L/D than symmetrical biplanes [44]. Based on Moeckel’s conclusion, R.M. Licher made an analysis to design the optimal L/D unsymmetrical biplane with the given Cl as a constraint by using the supersonic linear theory in 1955 [10]. This is an interesting paper, which would lead us to a practical supersonic-biplane transport. This will also be discussed in the next chapter. After the publication of Ref. [10], however, no further publication could be found in the literature except for Ref. [45]. It appears that something had discouraged researchers from further studying the Busemann biplane. K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 given by 2.3. Descendants and derivatives of the biplane concept Inspired by the Busemann biplane concept, a lot of research was inspired to consider the configuration wherein shock waves interact with other multiple elements to produce favorable wave interaction and reduce wave drag. Recently, in 2004, such research was summarized in Ref. [46]. The author of Ref. [46], Dr. D.M. Bushnell of NASA Langley Research Center, called the concept the ‘‘favorable shock-wave-interference approach’’ for drag reduction. He concluded that the issues of the concept can be increasingly addressed through the progress of ‘‘smart material’’ and ‘‘flow control’’ technologies. Some of the issues such as the derivatives of the biplane concept can be found in Refs. [47–49]. 3. 2-D theory and construction of biplane airfoils 3.1. Basic theory 3.1.1. Wave-reduction effect In this section we demonstrate the wave-reduction effect of 2-D biplanes. We compare the wave drag of two different airfoils, a single flat-plate airfoil and a parallel flat-plate biplane, at a supersonic flow condition. The conditions are such that both airfoils generate the same amount of lift (the ‘‘constant-lift condition’’). Using the 2-D (inviscid) supersonic thin-airfoil theory [8], the local pressure jump can be expressed as a function of the (small) local flow inclination angle a, measured from the oncoming flow direction, as sketched in Fig. 4 DP P1 ¼ gM12 pp1 ffia ¼ qffiffiffiffiffiffiffiffiffiffiffiffiffi P1 M12 1 57 ð1Þ where g and M1 represent the ratio of specific heats and the Mach number of the oncoming flow, respectively. Symbols P and P1 are the local and oncoming flow pressures. It can be shown that the lift and wave drag of a single flat-plate airfoil with a small angle of attack aS as sketched in Fig. 5 are 4aS LS ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C 2 1 M1 ð2Þ 4a2S q1 C DS ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi 2 1 M1 ð3Þ where the symbols qN and C represent the free-stream dynamic pressure defined by q1 2 1 gM1 r U2 ¼ P1 2 1 1 2 and the chord length of the flat-plate airfoil, respectively [6,8]. Next, we calculate lift and drag of a biplane airfoil. The biplane, as shown in Fig. 6, is constructed of two parallel flat plates having the same chord length as that of the single flat plate C at an angle of attack ab. Assuming that the compression wave (or expansion waves) generated from the leading edge (L.E.) of one element does not interact with the neighboring element, lift and drag of the biplane can be calculated in the same way as they were calculated for the single flat-plate airfoil [6]. The lift and drag of the biplane airfoil can be calculated as follows: 8ab Lb ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C 2 1 M1 ð4Þ 8a2b q1 C Db ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi 2 1 M1 ð5Þ We then adjust the biplane’s angle of attack ab such that the constant-lift condition is met. Equating the lift of the biplane with the lift produced by the single flat-plate airfoil (Eqs. (2) and (4)), the two incidence angles ab and aS have the following relationship: ab ¼ aS ð6Þ 2 Under the constant-lift condition, the wave drag of the biplane reduces to ! a 2 4a2S 8 1 S pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi Db ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C q1 C ¼ ð7Þ 2 1 2 2 1 2 M1 M1 Compared with Eq. (3), it is clear that the wave drag of the biplane reduces to 1/2 of the original single-plate airfoil under the constant-lift condition [6]. Fig. 4. Pressure jump through a compression or an expansion wave. Fig. 5. Single flat-plate airfoil with angle of attack aS. Fig. 6. Biplane airfoil with angle of attack ab. 58 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Similarly, it can be easily shown that the wave drag of an n-plate system reduces to 1/n of the original single-plate airfoil under a specified lift condition, as long as the compression wave (or expansion waves) generated from the L.E. of each element of the n-plate biplane system does not interact with the neighboring elements. We should remember, however, that skin-friction drag of the n-plate airfoil will increase to n-times that of the single flatplate airfoil because of the increased surface area. The increase in skin-friction drag is an inevitable byproduct of our wave-drag reduction process using multi-element airfoil systems. The skin friction of a single flat-plate airfoil can be estimated using the following incompressible turbulent boundary-layer formula [50]: Cdfric ¼ 2Cf Fig. 8. Pressure distribution on Busemann biplane. ð8Þ where Cf ¼ 0:027 ðReÞ1=7 ð9Þ In this paper lift and drag coefficients of both single-plate and biplane airfoils are defined by Cl L/qNC, Cd D/qNC based on the chord length of single-plate airfoil C. The symbol Re denotes the Reynolds number based on the flat-plate chord length, C. For the conversion from incompressible to compressible flows, a relationship between the skin-friction coefficient (for incompressible flows) and Mach number plotted in Fig. 13.10 of Ref. [8] can be used. 3.1.2. Wave-cancellation effect (Busemann biplane) The biplane configuration can also significantly reduce wave drag due to airfoil thickness. Within a supersonic thin-airfoil approximation, Busemann [7] showed that the wave drag of a zero-lifted diamond airfoil can be completely eliminated by simply splitting the diamond airfoil into two elements and positioning them in a way such that the waves generated by those elements cancel each other [8,9], as sketched in Fig. 7. The wedge angles of the diamond airfoil and the Busemann biplane are 2e and e, respectively. Remember that pressures acting on the front half and rear half of its inner surfaces, P1 and P2, respectively (see Fig. 8), are identical. Total lift and wave drag acting on the Busemann biplane are, therefore, both zero under the zero-lift condition. Within a supersonic thin-airfoil approximation, lift and drag acting on the Busemann biplane are identical to those acting on a flat-plate airfoil at a small angle of attack [6,9]. However, in the actual case wave drag is always larger than that of the flat-plate airfoil, because of the entropy produced due to the shock waves existing between the biplane elements. Generally, in supersonic speeds, wave drag due to airfoil thickness is large relative to that due to its lift. Supersonic aircraft are therefore severely limited in their wing thickness. If the Fig. 7. Wave-cancellation effect of Busemann biplane. Fig. 9. Diamond airfoil at zero angle of attack. Fig. 10. Wave-drag components of a diamond airfoil. wave-cancellation effect is used effectively, the strong restriction currently imposed on the wing thickness of supersonic aircraft may be relaxed considerably. Remember that lift and wave drag of a diamond airfoil (see Fig. 9) with a small incidence angle a can be expressed, using the same supersonic thin-airfoil approximation, as ([8]) 4a LD ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C þ 2 1 M1 ð10Þ " 2 # 4 t 2 DD ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi a þ q1 C þ UUU 2 1 C M1 ð11Þ Symbol t/C represents the thickness–chord ratio of the diamond airfoil. In Fig. 10 wave-drag components due to lift and due to thickness are calculated for a lifted diamond airfoil for two different airfoil thicknesses t/C¼0.5 and 0.10 at constant-lift condition Cl ¼ 0.10 and flow condition MN ¼1.7. Skin-friction coefficients are calculated using Eq. (8), which is based on a flatplate airfoil with chord length C, with the help of the Mach number correction factor given in Ref. 8. 3.1.3. An ideal biplane configuration, the Licher biplane The previously discussed wave-reduction model (flat-plate biplane model shown in Fig. 6), however, cannot be combined to Busemann’s wave-cancellation concept directly, because wave interactions that are required for the Busemann biplane do not occur. We, therefore, need to seek a biplane configuration that has those two desirable characteristics simultaneously in order to attain a significant wave-drag reduction. An unsymmetrical K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 biplane configuration discussed by R. Licher in 1955 [10] (sketched in Fig. 11) exhibits both of the desirable characteristics: the wave-reduction effect and the wavecancellation effect. By promoting favorable wave interactions between the upper and lower elements, the wave drag due to lift can be reduced to 2/3 of that of a single flat plate under the identical lift condition. Additionally, the Busemann wavecancellation concept can be applied to the system to eliminate wave drag due to airfoil thickness. Wave-reduction effect of the Licher biplane is discussed next. Utilizing the supersonic thin-airfoil theory [8], the Licher biplane can be split into its lift component and thickness components, as shown in Fig. 11. Analysis of wave drag due to thickness for the Licher biplane is identical to that of the Busemann biplane, and has therefore been discussed in the previous section. We, therefore, focus on lift and wave drag due to its lift component. The symbol a denotes the flow inclination angle of the upper (flat-plate) element; it also denotes the flow inclination angle of the lower surface of the lower element (see Fig. 12). The wedge angle of the lower element (having a halfdiamond shape) has been also chosen to be a. This particular shape and location of the lower element cause the compression wave generated from the leading edge of the upper element and the expansion waves generated from the throat of the lower element to cancel each other. This can be shown through arguments similar to the wave-interaction analysis of the Busemann biplane. Finally, we can show that the pressure along the entire upper surface of the lower element is uniform, and that its value is identical to that of the free-stream. It is, therefore, clear that the upper element of the system acts like single flatplate airfoil with the incidence angle of a, and pressure acting on the lower surface of the lower element contributes to the system as an additional aerodynamic force. Detailed discussions are given in [6]. 59 Lift acting on the Licher biplane system shown in Fig. 12 can be calculated as ! 4a 2a 3 4a pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiq1 C L ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C þ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C ¼ ð12Þ 2 1 2 1 2 1 2 M1 M1 M1 Comparing Eq. (12) with Eq. (2), it is clear that the Licher biplane generates 1.5 times the lift of a single flat-plate airfoil with the same angle of attack. We now adjust the angle of attack a of the Licher biplane so that it generates the same amount of lift as a single flat-plate airfoil with an angle of attack aS (constant-lift condition). Since both the single and Licher airfoils have the same reference chord length C, a and aS have the following relationship: 2 3 a ¼ aS ð13Þ Because of the constant-lift condition ! 3 4a 4aS pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiq1 C ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C ¼ LSingle L¼ 2 1 2 1 2 M1 M1 ð14Þ Similar to the lift analysis, wave drag of the Licher biplane system can be expressed as ([6]) ! 4a2 2a2 3 4a2 pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiq1 C D ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C þ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi q1 C ¼ ð15Þ 2 1 2 1 2 1 2 M1 M1 M1 When the constant-lift condition (L¼LSingle) is considered, the drag Eq. (15) reduces (with the help of Eq. (13)) to ! ! 2 4a2S 3 4 2 2 2 pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiq1 C ¼ DSingle aS q1 C ¼ D¼ 2 1 3 2 1 2 3 3 M1 M1 ð16Þ Eq. (16) shows that the wave drag due to lift of the Licher biplane reduces to 2/3 that of a single flat-plate airfoil under constant-lift condition [6,10]. 3.2. CFD analysis Fig. 11. Decomposition of Licher Biplane into its lift and thickness components. 3.2.1. Analyses using unstructured grid In this section, analysis results from CFD simulations using an unstructured grid approach are mentioned [18–20]. For the purpose of examining the characteristics of Busemann biplanes at off-design conditions with zero incidence angle, the thickness– chord ratio (t/c) of the Busemann biplane and a diamond airfoil were selected as 0.10 (its equivalent wedge angle, e, being 5.711) (shown in Fig. 13). The gap of the biplane has been adjusted (z/c¼0.5) to obtain minimum drag at free-stream Mach number, MN ¼1.7, which will be referred as the design Mach number hereinafter in this paper. Inviscid flow analyses were performed using the TAS code (Ref. [51,52]). The grids around the diamond airfoil and the Busemann biplane are shown in Fig. 14. Wave-drag coefficient (Cd) calculated from CFD analyses and theory based on the supersonic thin-airfoil approximation are shown in Table 1. They are in good agreement. The reliability of the CFD code was Busemann biplane Diamond airfoil t1 z t2 c (z/c=0.5, t/c=0.10) Fig. 12. Geometry of lift component of Licher Biplane. t c t1=t2 (t/c=0.10) Fig. 13. Baseline models at zero-lift conditions (MN ¼ 1.7). 60 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Zero-Lift conditions 0.16 Busemann Biplane Busemann Biplane Diamond Airfoil Diamond Airfoil 0.14 t z 0.12 c Cd 0.10 (z/c=0.5, t/c=0.05) t 0.08 c (t/c=0. 10) 0.06 0.04 Fig. 14. Mesh visualizations of a Busemann biplane baseline model (twodimensional unstructured grid, grid numbers are 0.20 million). 0.02 0.00 0.0 0.5 1.0 1.5 2.0 2.5 3.0 M Diamond airfoil Busemann biplane CFD Theory Error (%) 0.0291 0.00189 0.0292 – 0.34 – discussed and validated in Ref. (6). It is clear that wave drag of the Busemann biplane is almost completely eliminated being less than 1/10 of that of the diamond airfoil. Because the biplane configuration is similar to the supersonic converging and diverging nozzle, supersonic biplanes may have the disadvantage of having choked flow at a wide range of transonic flow regions. The choked-flow phenomenon generates significant wave drag at the biplane’s off-design Mach numbers. Fig. 15 shows the detailed wave-drag characteristics of the Busemann biplane over a range of flight Mach numbers (0.3o MN o3.0), including its design Mach number MN ¼1.7. Flow-hysteresis problem that caused by the continuous change in the free-stream Mach number will not be considered. CFD analyses, including the flow-hysteresis problem, will be discussed in the next section. Flow choking and its concomitant hysteresis problem were also observed in experiments [14,34,35]. Let us now return to Fig. 15 again. We can observe a range of Mach numbers (1.64oMN o2.0) where wave drag remains nearly at its minimum value. The existence of this low wavedrag range is critical for the development of actual airplanes in the future. From Fig. 15, however, we also observe a high wave-drag range of Mach numbers in the transonic flow region where wave drag is even greater than that of the baseline diamond airfoil. Some of the strategies to counter against choking (high drag) are airfoil morphing and the adaptation of Fowler motion. Figs. 16 and 17 show simple diagrams of morphing and Fowler motion used in this study. Morphing alters the area ratio of the inlet area to the throat. With the morphing strategy, reduction in wave drag is expected because of the change in airfoil thickness. The thickness–chord ratio (t/c) is changed from 0.10 to 0.06 on each element as shown in Fig. 16. Fig. 18 shows wave-drag characteristics at various Mach numbers of the Busemann biplane with morphing and with Fowler motion. It can be observed that much lower wave drag than that of the diamond airfoil is achieved over a wide range of free-stream Mach numbers [6,18]. However, it is obvious that the biplane with Fowler motion has higher friction drag than other biplanes because of the increase in surface area. 3.2.2. Hysteresis analysis In real flight, airplanes accelerate from take-off to cruise Mach number continuously. During the acceleration stage, choking will Fig. 15. Wave-drag characteristics of the diamond airfoil and the Busemann biplane (zero lift). Fig. 16. A simple diagram of the Busemann biplane with morphing. Fig. 17. A simple diagram of the Busemann biplane with Fowler motion. 0.16 Busemann Biplane Diamond Airfoil Morphing Fowler 0.14 0.12 0.10 Cd Table 1 Wave-drag coefficients (Cd) of a diamond airfoil and a Busemann biplane (zero lift). 0.08 0.06 0.04 0.02 0.00 0.0 0.5 1.0 1.5 M 2.0 2.5 3.0 Fig. 18. Wave-drag characteristics of the biplane with morphing (zero lift). occur and it may cause flow-hysteresis problems. Therefore it is necessary to simulate the actual processes by changing the airplane speed continuously. 3.2.2.1. One-dimensional theory on intake diffuser. It is necessary to discover methods that are applicable to Busemann-type biplanes in order to avoid the choked-flow and flow-hysteresis problems at off-design conditions. Before we examine how these problems can be overcome, it may be useful to discuss the start/un-start characteristics of a supersonic inlets diffuser (see Fig. 19), for they K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 61 share many similar characteristics with the Busemann biplane. In Fig. 19, the line in red shows the Kantrowitz limit [16,53] (given by Eq. (17)), which is the Mach number that the flow speed of inlet diffusers must exceed before the inlets can go from the unstart to start condition, once a bow shock is generated in front of its inlet. 1=2 1=ðg1Þ 2 2 At ðg1ÞM1 þ2 2gM1 ðg1Þ ¼ ð17Þ 2 2 Ai ðg þ1ÞM1 ðg þ1ÞM1 It is reasonable to assume that this rule can be applied to the Busemann biplane in order to avoid the choked-flow and flowhysteresis of the Busemann biplane. In fact, the results from CFD analyses are in good agreement with the values that are calculated using Eqs. (17) and (18). Here the At/Ai of the Busemann biplane is 0.8, as shown by the solid line in Fig. 19. The predicted Mach number of the starting and unstarting are 2.154 and 1.600, respectively. where Ai is the area of inlet and At is the area of throat. Also, the line in blue refers to the isentropic contraction limit [53], where the Mach number is MN ¼1.0 at the throat of supersonic inlets. The isentropic contraction limit is calculated by Eq. (18). ðg þ 1Þ=2ðg1Þ 2 At ðg1ÞM1 þ2 ¼ M1 ð18Þ Ai gþ1 3.2.2.2. Quasi-unsteady CFD simulations. Inviscid CFD analyses (Euler simulation) of the Busemann biplane were conducted to trace the hysteresis. For the simulation we use the following strategy: a quasi-unsteady simulation. We divide the acceleration process from 0.6 to 2.18 of free-stream Mach numbers into small intervals of 0.1 or so. We conducted a series of simulations as MN was raised discretely along the intervals using the previous simulation result imposed as the initial condition. Figs. 20 and 21 show Cp color maps at each Mach number in acceleration and deceleration stages. Fig. 22 shows a Cd–MN graph. We observe that a detached shock wave is generated at an upstream location of the airfoils and it gets closer to the leading edge with each increment of a free-stream Mach number. Once a certain Mach number is reached, the detached shock wave attaches to the leading edge and is swallowed to a downstream location of the throat. As a consequence, choking disappears (in this case at MN ¼2.18). This phenomenon is similar to that of the starting process of the intake diffuser. The value of the starting Mach number given from CFD agrees with that predicted from the 1-D flow equation (Eq. (17)). Kantrowitz limit Isentropic Contraction limit Ai At Fig. 19. Start/un-start characteristics of supersonic inlet diffuser. 3.2.2.3. How to minimize hysteresis problem. From Fig. 19 it is evident that as the area ratio At/Ai increases, the biplane becomes more and more startable at lower Mach numbers. The biplane equipped with hinged slats and flaps, shown in Fig. 23, was used for the study. With the hinged slat the sectional area ratio of the inlet to the throat (At/Ai) increases to 0.909. According to the Fig. 20. Cp color maps around the Busemann biplane on acceleration (from 0.6 to 2.18 of free-stream Mach number per about 0.1) (0.6 r MN r1.1); (1.2r MN r1.7) and (1.8r MN r 2.18). (For interpretation of the references to color in this figure legend, the reader is referred to the web version of this article). 62 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Fig. 23. A simple diagram of the Busemann biplane equipped with hinged slats and flaps. Fig. 24. Cp color maps of the biplane equipped with hinged slats and flaps on acceleration (zero lift). (For interpretation of the references to color in this figure legend, the reader is referred to the web version of this article). 0.16 0.14 0.16 Impalsive start Analyses 0.14 Acceleration Cd 0.06 0.00 0.0 0.08 M =1.64 1.0 1.5 M 2.0 2.5 M =2.18 M =1.56 1.0 1.5 M 2.0 2.5 Design Mach number M =1.7 M =2.18 0.5 Hysteresis 0.02 0.08 0.02 Slat and Flap Acceleration 0.10 0.00 0.5 0.04 Acceleration 0.04 Hysteresis 0.10 Busemann 0.06 Deceleration 0.12 Deceleration Slat and Flap Deceleration 0.12 Cd Fig. 21. Cp color maps around the Busemann biplane on deceleration (from 2.18 to 1.7 of free-stream Mach number per about 0.1) (2.18 Z MN Z1.7). (For interpretation of the references to color in this figure legend, the reader is referred to the web version of this article). Busemann 3.0 Fig. 25. Wave-drag characteristics of the Busemann biplane and the one equipped with hinged slats and flaps on quasi-unsteady flow (zero lift). Design Mach number M =1.7 Fig. 22. Wave-drag characteristics of Busemann biplane on quasi-unsteady flow (zero lift). equations of the intake diffuser, the biplane equipped with hinged slats and flaps can start at MN ¼1.55. Choking will no longer occur at the cruise Mach number (MN ¼1.7). CFD analyses were conducted on the Busemann biplane equipped with slats and flaps using a quasi-unsteady simulation [6,18,21]. Fig. 24 shows Cp color maps near the starting Mach number. Fig. 25 shows a Cd–MN graph. We can see the starting occurs at MN ¼1.56 and that choking disappears. Note that based on the one-dimensional theory from Eq. (17), the predicted starting Mach number is 1.55. For the Busemann biplane with morphing discussed in the previous section (see Fig. 16), the starting condition is MN ¼ 1.41. The ratio of the section area of the inlet to that of the throat (At/Ai) is 0.936 (With the theoretical starting Mach number being 1.40). K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 63 Cp Eddy viscosity Fig. 26. A simple diagram of the Busemann biplane equipped with hinged slats and flaps utilized as a high-lift-device. Busemann biplane 2.5 Cp 2 2 3 4 5 6 7 Eddy viscosity 8 1 0 1.5 Cl -1 1 every 1degree plotted 5 0.5 3 2 Busemann Busemann biplane biplane with HLD with 1 0 0 0 Busemann Busemann biplane biplane 0.05 0.1 0.15 0.2 HLD Busemann biplane equipped with slats (10deg) and flaps (15deg) 0.25 Cdtotal Fig. 27. Drag polar diagram of the Busemann biplane and the one equipped with hinged slats and flaps utilized as a high-lift-device angle of attack 41. 3.2.2.4. High-lift condition. In this section, take-off and landing conditions (MN ¼0.2) are discussed [6,18]. The Navier–Stokes equation was used for the analyses, with a Reynolds number of 30 million. A one-equation turbulence model by Spalart–Allmaras [54] is adopted to treat turbulent boundary layers for viscous flow computations. The same hinged slats and flaps mentioned before are used as a high-lift-device. The geometry used for the analysis is shown in Fig. 26. The positions of the hinges are the same as those of the biplane shown in Fig. 23 (30% chord length). Fig. 27 shows a drag polar diagram (where, Cdtotal refers to the total drag coefficient: wave drag plus friction drag) and Fig. 28 shows Cp and eddy viscosity color maps at an angle of attack of 41. The calculated lift and drag are given in Table 2. It can be observed that sufficient lift (Cl 42.0) is generated by utilizing hinged slats and flaps. Viscous effects at off-design conditions are also discussed briefly. Fig. 29 shows Cdtotal characteristics near its start and unstart Mach numbers. Here, results from both Navier–Stokes and Euler simulations are compared. It shows that there are no drastic changes in flow characteristics caused by viscous effects, with the exception of the starting Mach (reduced from 2.18 to 2.14). Fig. 28. Cp and eddy viscosity color maps of the Busemann biplane and the one equipped with slats and flaps as a high-lift-device (A.o.A¼ 41). (For interpretation of the references to color in this figure legend, the reader is referred to the web version of this article). Table 2 CFD analysis results of both two biplanes at an angle of attack 41. Busemann biplane HLD 4.1. Inverse design 4.1.1. Pressure and geometry relation As discussed in the previous section, biplane airfoils can be good candidates for the wings of next generation SST. We, therefore employed inverse designing to optimize the biplane airfoil at its lifted condition. The inverse-design process calculates Cdtotal 0.612 2.025 0.0485 0.0955 Euler Acceleration Euler Deceleration NS Acceleration NS Deceleration 0.10 0.08 0.06 M =2.14 0.04 M =2.18 0.02 0.00 1.2 4. Design for biplane airfoil of better performance Cl 0.12 Cdtotal 4 M =1.64 2.2 1.7 Design Mach number M =1.7 M Fig. 29. Cd characteristics of the Busemann biplane on quasi-unsteady simulation over a range of free-stream Mach numbers (Euler and Navier–Stokes analyses). the airfoil geometry, necessary for the specified target pressure distribution to occur along its surface [6,17–19]. In order to determine the desired geometry, the relationship between the 64 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 surface pressure distribution and geometry is required. Here, we use an algebraic relationship derived from the oblique shock relations [8]. An airfoil’s geometry f(x) is related to its pressure distribution, as follows: Cp ¼ c1 y þ c2 y 2 ð19Þ z c2 ¼ ðM1 2 2Þ2 þ gM1 4 2ðM1 2 1Þ2 x Fig. 31. Airfoil geometries based on the current and target pressure distributions. Initial Airfoil Target Cp Cp Grid Generation ð20Þ a is the angle of attack of the airfoil. Also, x represents the airfoilchord direction (see Fig. 30) and g is the ratio of specific heats. MN ( 41.0) is the free-stream Mach number. Eq. (19) is the second-order equation with respect to change in geometry. Splitting the airfoil geometry into the upper and lower surfaces, Eq. (19) yields 2 df þ ðxÞ df þ ðxÞ Cp þ ¼ c 1 a þc2 a dx dx 2 df ðxÞ df ðxÞ Cp ¼ c1 ð21Þ a þ c2 a dx dx f(x)+ Δ f(x) Flow A.O.A = α where y represents the local flow deflection angle (df/dx a) along the airfoil surface. The symbols c1, c2, are the Busemann coefficients given as 2 c1 ¼ qffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi , M1 2 1 f (x) Cp Target Cp Flow Solver (Euler) Current Cp Cp Current Cp Grid Generation Cp< YES Inverse Design f f+ f NO f : Geometry Correction Designed Airfoil Fig. 32. The iterative design method of the inverse-problem design. where subscripts + and denote the upper and lower surfaces, respectively. 4.1.2. Basic equation and procedure for the inverse-problem design Eq. (21) can be used for design problems. In Ref. [55], Ogoshi and Shima performed the wing-section design of an SST using Eq. (21). However, the interaction effects between the two biplane elements must be considered. Then, we must adopt the perturbation form (i.e. D-form) of Eq. (21) as the basic equation for our inverse-design procedures [56]. Taking the small perturbation form (Cp-Cp + DCp and f-f+ Df) of Eq. (21), we obtain D-form equations [57]: 2 dDf þ ðxÞ df þ ðxÞ dDf þ ðxÞ dDf þ ðxÞ DCp þ ¼ c1 þ 2c2 a þc2 dx dx dx dx ð22Þ DCp ¼ c1 2 dDf ðxÞ df ðxÞ dDf ðxÞ dDf þ ðxÞ þ2c2 a þ c2 dx dx dx dx ð23Þ where + and indicate upper and lower surfaces, respectively (see Fig. 31). With the above D-form equations, an iterative design method is constructed. Fig. 32 illustrates the iterating process. First, the flow field around the initial configuration is analyzed to obtain the ‘‘initial’’ pressure distribution of the airfoil. At this time, the target pressure distribution should be specified. Next, an inverse-problem solver is employed to calculate the x-derivative of the correction value for the airfoil geometry, dDf 7 /dx; this Fig. 30. Airfoil and flow direction. x-derivative is related to the difference between the target and the current pressure distributions, denoted as DCp (Cp-residual). In particularly, the geometry correction term Df (see Fig. 31 again) is determined from DCp. Solving Eqs. (22) and (23) for dDf + /dx and dDf /dx, the airfoil geometry is updated: Z x dDf 7 update ðxÞ ¼ f 7 ðxÞ þ ðxÞ dx ð24Þ f7 dx 0 where the symbol 0 indicates the x coordinate of the airfoil leading edge. In this approach, however, it is evident that there is no guarantee in obtaining an airfoil that has a closed trailing edge. We, therefore, may need to make further (but minor) modifications to the obtained geometry with the closed trailing edge [6]. We, then, calculate the flow field around the updated airfoil geometry for the next cycle. An optimal airfoil design can be obtained through repeating this process until DCp (Cp-residual) becomes negligible. 4.2. Inverse design for biplane airfoil at a Mach number of 1.7 4.2.1. Design process For the inverse design, a Licher-type biplane was selected as the initial biplane configuration (a ¼1.01, Cl ¼0.0812, Cd ¼0.00449) and from this the geometries of upper and lower elements would be designed [18,19]. The design procedure is shown in Fig. 33. As a design condition, free-stream Mach number MN ¼ 1.7 and angle of attack a ¼11 were selected (here, a represents the angle of the lower surface of the lower element against the freestream direction). A flow solver called TAS code, using unstructured grids, was used to calculate the flow fields around the biplane. Both the target and initial pressure distributions for both the upper and lower elements used for the biplane design are shown in Fig. 34. Target Cp distributions are constructed in such ways to generate more lift on the upper surface of the upper element and also to create additional lift but generating lower drag on the lower surface of the upper element (especially near the K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 TargetCp Cpof Target of UpperWing Wing Upper Designed DesignedAirfoil Airfoil 65 Initial Airfoil Current Cp of Upper Wing YES Cp Cp< Cp< Target Cp Flow Solver (Euler) Current Cp Grid Generation NO Grid Generation Inverse InverseDesign Design f f f+ f Grid GridGeneration Generation f+ f Inverse Design NO FlowSolver Solver Flow (Euler) (Euler) Cp Target Cp Cp< Current Cp Current Cp of Lower Wing YES f : Geometry Correction Target of TargetCp Cpof Lower Wing Wing Lower Designed Airfoil Fig. 33. Design cycle. Upper Element Upper Element INITIAL INITIAL -0.1 -0.10 TARGET TARGET 0.0 -0.05 0.1 0.00 Cp Cp 0.3 0.5 0.6 -0.1 Designed 0.05 0.2 0.4 TARGET An additional lift but lower drag Remove Lift on the upper surface of the upper element 0.0 0.1 0.2 0.15 0.20 0.4 0.5 0.6 (x/c) 0.3 0.10 0.7 0.8 0.9 1.0 0.25 -0.1 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 (x/c) 1.1 Lower Element Lower Element INITIAL INITIAL -0.1 -0.10 TARGET TARGET 0.0 Designed 0.00 0.1 Cp Cp 0.2 0.3 A cause of reflection shock wave 0.5 0.1 0.2 0.3 0.05 0.10 0.15 Remove 0.4 0.6 -0.1 0.0 TARGET -0.05 0.4 0.5 0.6 (x/c) 0.7 0.8 0.9 1.0 0.20 1.1 0.25 -0.1 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 (x/c) Fig. 34. Target Cp distributions. Fig. 35. Cp distributions of the designed biplane configuration. trailing edge). The obtained Cp distributions (after 14 times iterations) of the upper and lower elements, along with the target values, are plotted as shown in Fig. 35. The initial and designed geometries are compared in Fig. 36. The gain of the angle of attack (of the lower surface on the lower element) is approximately 0.191 at its design point, a ¼1.01 (compared with the initial Lichertype biplane). The total maximum thickness–chord ratio (t/c) of the designed biplane is 0.102. Its lift and wave drag are Cl ¼0.115, Cd ¼0.00531 (L/D ¼21.72). It is clear that a biplane having better aerodynamic performances was designed compared with that of the Licher biplane. Detailed aerodynamic performances of those biplanes are given in Table 3. A Cp contour map at this design point is shown in Fig. 37. It was confirmed that this inverse-design method would work well for the 3-D biplane configurations to determine its wing-section geometries [30,58]. 4.2.2. General features of the designed airfoil Observing the geometry of the designed biplane, the trailing edge of the upper element of the designed biplane configuration was modified so that its curvature was aligned to the free-stream direction, creating additional lift. It should also be noted that the 66 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 compression waves from the leading edge of the biplane elements and the expansion waves generated from its throats nearly cancelled each other out, eliminating the initially observed pressure peaks at the throats. Navier–Stokes analyses were also performed on the designed biplane. The Reynolds number is 32 million. Flow conditions are identical to those discussed in the previous section. Fig. 38 shows the pressure distributions of the designed biplane obtained from Navier–Stokes simulations. We observed that pressure peaks arise a short distance in front of the throats due to the boundary layer effect. Cp distributions obtained from Euler simulations are very Upper Element 0.30 (z/c) 0.25 0.20 Initial Designed Biplane 0.15 -0.1 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 Fig. 37. Cp contour map of the designed biplane at MN ¼ 1.7 (a ffi 11). (x/c) LicherAB1.5 NS Cp distributions Euler Lower Euler Upper Lower Element -0.15 -0.1 Initial NS NS Upper Lower 0.0 Designed Biplane -0.20 Cp (z/c) 0.1 0.2 -0.25 0.3 0.4 -0.1 -0.30 -0.1 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 0.1 0.3 0.5 0.7 0.9 1.1 (x/c) (x/c) Fig. 38. Cp distributions of the designed biplane configuration in Navier–Stokes simulations. Fig. 36. Section airfoil geometries of the designed biplane (t/c¼ 0.102). Table 3 The aerodynamic performance in Euler simulations. a (deg.) 0.0 0.5 1.0 1.5 2.0 2.5 3.0 3.5 Busemann Cl Cd L/D 0.0000 0.00218 0.00 0.0284 0.00245 11.61 0.0571 0.00325 17.59 0.0858 0.00458 18.72 0.1146 0.00647 17.72 0.1435 0.00891 16.11 0.1727 0.01192 14.49 0.2021 0.01551 13.03 Diamond Cl Cd L/D 0.0000 0.02891 0.00 0.0257 0.02914 0.88 0.0515 0.02983 1.73 0.0773 0.03100 2.49 0.1031 0.03264 3.16 0.1290 0.03475 3.71 0.1550 0.03734 4.15 0.1810 0.04041 4.48 Licher Cl Cd L/D 0.0231 0.00345 6.71 0.0521 0.00370 14.10 0.0812 0.00449 18.06 0.1102 0.00586 18.80 0.1394 0.00780 17.88 0.1687 0.01031 16.36 0.1982 0.01346 14.73 0.2279 0.01725 13.21 0.0580 0.00336 16.38 0.0867 0.00414 20.93 0.1154 0.00531 21.72 0.1442 0.00701 20.55 0.1730 0.00925 18.70 0.2018 0.01202 16.79 0.2307 0.01534 15.04 0.2598 0.0192 13.51 Flat plate (theory) Cl 0.0000 0.00000 Cd L/D – 0.0254 0.00022 114.59 0.0508 0.00089 57.30 0.0762 0.00199 38.20 0.1016 0.00355 28.65 0.1270 0.00554 22.92 0.1523 0.00798 19.10 0.1777 0.01086 16.37 Designed Cl Cd L/D K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 0.04 0.2 Cd wave 0.03 0.18 (Cd) 0.16 Cd friction (Cdf) 0.14 0.12 Cl Cdtotal 67 0.02 0.1 Flat Plate Busemann Licher Designed 0.08 0.06 0.01 0.04 0.02 0 Diamond Busemann Designed Fig. 39. Evaluation of Cdtotal at the same lift conditions (Cl ffi0.11) (diamond airfoil, Busemann biplane, designed biplane). Table 4 The aerodynamic performance in Navier–Stokes simulations. a (deg.) Cl Cd Cdfric Cdtotal Cl (in Euler) Cd (in Euler) Diamond 0 0 2 0.104 0.0292 0.0329 0.00394 0.00414 0.0332 0.0370 0 0.103 0.0289 0.0326 Busemann 0 0 2 0.116 0.00185 0.00639 0.00777 0.00780 0.00962 0.0142 0 0.115 0.00218 0.00647 Designed 1.19 0.116 0.00479 0.00794 0.0127 0.115 0.00531 0 -1 0 2 1 3 4 [deg] Fig. 41. Cl-a characteristics of various airfoils. are obtained by the supersonic thin-airfoil theory whose equation is given from Eq. (3), as, pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi 2 1 4a2 M1 Cd ¼ pffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi ¼ ð25Þ Cl2 2 1 4 M1 Note that the single flat-plate airfoil of zero thickness has only wave drag due to its lift. Therefore, during supersonic flights the single flat-plate airfoil has the lowest wave drag among monoplanes. The designed biplane has lower wave drag than the Lichertype biplane over a wide range of Cl. Particularly when Cl 40.14, the total wave drag of the designed biplane becomes less than that of the zero-thickness single flat-plate airfoil. It is surprising to find a biplane configuration that can have a lower wave drag than that of a flat-plate airfoil. From Table 3, one can see that at a range of sufficient lift of 0.1 rCl r0.2, the designed biplane exhibits a 12 to 35 count reduction in Cd, compared to that of the Busemann biplane. Fig. 41 shows a Cl-a graph on the above-mentioned airfoils. The lower the a of an airfoil is, the weaker the shock waves generated from the lower surface are. It can be observed from Fig. 41 that the designed biplane has the highest Cl among those with the same a. In other words, the designed biplane emits the weakest shock waves toward the ground at a given Cl condition. Tabulated geometry of this 2-D designed biplane is given in Ref. [6,28]. Fig. 40. Wave-drag polar diagrams of the designed biplane. 5. 3-D extension of biplane airfoils similar to those of Navier–Stokes simulations. In Fig. 39 the total Cd (shown as Cdtotal: wave drag plus friction drag) at the identical lift conditions (Cl ffi 0.11) are compared among the diamond airfoil, the Busemann biplane and the designed biplane. Drag components are tabulated in Table 4. It was observed that the designed biplane had nearly the same friction drag as the original Busemann biplane but with lower wave drag reducing the total drag coefficient from 142 counts to 127 counts (here, 1count means 10 4). 4.3. Drag polar diagrams Fig. 40 shows the drag polar curve (from the inviscid analysis) of the designed biplane at the cruise Mach number (MN ¼1.7) compared against the curves of other airfoils. The figure includes a zero-thickness single flat-plate airfoil, a Busemann biplane, a Licher-type biplane and the designed biplane. Numerical data are given in Table 3. The characteristics of the single flat-plate airfoil 5.1. Busemann biplane wing with rectangular planform The 2-D Busemann biplane was then extended to a 3-D rectangular wing. The wing-section geometry of this rectangular wing was identical to that of the 2-D Busemann biplane. The reference wing area (based on one element of the wing) of this wing was chosen to be 1.0 and the semi-span length and aspect ratio of the wing were 1 and 2, respectively. Inviscid flow analysis around the biplane wing was conducted at the free-stream Mach number of 1.7. The number of nodes used in the CFD analysis was approximately 1.10 million. Typical meshes are shown in Fig. 42. It also shows Cp contours of the rectangular biplane wing. It is clear that adequate interference between shock waves and expansion waves does not occur at wingtip regions. Figs. 43 and 44 show Cp distributions at wing-span stations and span-wise Cd distributions of the rectangular biplane wing. Pressure leaks are observed inside of the Mach cone generated at the leading edge of the wingtip. As a result of those pressure leaks, the two-dimensionality of the flow is 68 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 flow y Cp visualizations Top view croot mid-chord line Free stream x z Front view z x y Side view Effects of Mach cone z Span direction y x Fig. 42. Surface Cp and mesh visualizations of a rectangular Busemann biplane wing (reference area 1). Fig. 45. Orthographic drawing, and mesh and surface Cp visualization of a tapered Busemann biplane wing (reference area 1). Fig. 43. Cp distributions at each 10% span stations of a rectangular Busemann biplane wing (reference area 1). Fig. 46. Span-wise Cd distributions of a rectangular and a tapered Busemann biplane wings (reference area 1, zero-lift conditions). 2D Fig. 44. Span-wise Cd distributions of a rectangular Busemann biplane wing (reference area 1). lost at the wingtip area. The wave-drag coefficient of the biplane wing increased to 0.00685, knowing that Cd of the 2-D Busemann biplane is 0.00218 [30–32]. 5.2. Planform parametric study In order to reduce the undesirable pressure leaks occurring at the wingtips, tapered wings were considered. Fig. 45 shows the surface Cp contours of a tapered Busemann biplane wing. The wing reference (based on one element of the wing) area is also set to 1 and its taper ratio is 0.25. Therefore, the semi-span length and the aspect ratio will be 1.6 and 5.12, respectively. It is clear that the area affected by the Mach cones generated from the wingtips is significantly reduced. The CD of this tapered wing is 0.00300, which is significantly lower than that of the above-mentioned K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 69 rectangular wing. Fig. 46 shows span-wise wave-drag distributions along the rectangular and the tapered biplane wings. It also shows Cd of the 2-D Busemann biplane is included as a reference condition. This tapered wing also indicates another favorable effect. The Cd distributions in the mid-wing areas are lower than that of the 2-D case (see Fig. 46). In several of the following sections, the reasons for this favorable effect will be investigated. 5.2.1. Effect of changing sweep angles First of all, we checked the sweep angle effect on a tapered wing, with fixed taper ratio of 0.25. Here, the sweep angle is defined as the angle of the wing’s leading edge to the free-stream. Fig. 47 shows the CD characteristics relative to sweep angle. In this study the sweep angle effect was evaluated by choosing a parameter defined by the mid-chord apex at the wingtip (Cmid/ Croot) (see Fig. 47 for the definition of Cmid/Croot). It can be seen that the tapered wing with Cmid/Croot of around 0.5 achieved the lowest wave drag. Fig. 48 illustrates simple diagrams of two different wings termed Case 1 and Case 2, and their span-wise Cd distributions. Case 1 has no sweep (Cmid/Croot ¼0.125). Case 2 has a sweep angle and its mid-chord line is normal to the free-stream direction (Cmid/Croot ¼0.5). As shown in Fig. 47, Case 2 has a lower CD than that of Case 1. Fig. 49 shows Cp contours of the inner surfaces of Case 1 and Case 2 with the help of Cp distributions of those two wings at 50% semi-span stations. It also shows the Cp distributions of the 2-D case are included as a reference. It can be observed that Cp distributions of Case 1 and Case 2 wings differ from that of the two-dimensional result due to the sweep effect of the wing. It is clear that the Case 2 wing performs better than the Case 1 wing. 5.2.2. Effect by changing taper ratios The characteristics of CD were examined by changing the taper ratio of wing. The mid-chord of the wing was fixed to be normal to the free-stream direction (Case 2, Cmid/Croot ¼ 0.5 in Fig. 47). flow y cmid Fig. 48. Simple diagram of interaction of the shock waves and the expansion waves and span-wise Cd distributions of Case 1 and Case 2 (zero-lift conditions). ctip croot x croot/ctip= 0.25 Reference area = 1 2D Fig. 50 shows CD characteristics due to the change of aspect ratio. The taper ratio of the wing and aspect ratio are uniquely related because that the reference wing areas of the wings are fixed to 1. The smaller the taper ratio is, the lower the CD is. The aspect ratio also increases with decreasing taper ratio. However, CD does not decrease significantly when the taper ratio is less than 0.25. Therefore, a taper ratio of 0.25 was selected. The aspect ratio of this wing becomes 5.12. In the next section, the design of a threedimensional biplane wing, with this selected planform (the parameters of the planform are shown in Table 5), will be discussed using the inverse-design approach. Remember that the wave-drag coefficient of the Busemann biplane wing (whose wing section has Busemann biplane geometry) in this planform has values of CD ¼0.00300 at zero-lift conditions [30]. In Ref. [26], various biplane-wing planforms were systematically investigated in terms of drag reduction. 5.3. Introduction of winglet Fig. 47. CD characteristics with changes of the sweep angle (reference area 1, zero-lift conditions). During the extension of a supersonic-biplane airfoil to a threedimensional wing unfavorable pressure leaks, that would destroy 70 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 the desirable wave interactions were observed at the wingtip regions (see Figs. 42 and 43). In order to eliminate those pressure leaks at the wingtips, a winglet was introduced to the supersonic biplanes. Fig. 51 shows Cp and mesh contours of Busemann biplane wings with and without winglets. Table 6 shows drag coefficients of these biplane wings. Note that the wing planforms are identical to those discussed in the previous sections (see Table 5). It is clear that the unfavorable wingtip effects can be nearly eliminated and the aerodynamic performance improved by using the winglets. Furthermore, the winglets may be a necessity from a structural point of view. As can be seen in Fig. 51, the pressures of the inner surfaces of the biplane wing are higher than those of the outer surfaces. Therefore, winglets may play a critical role in minimizing flutter and bending problems. 5.4. Design for better-performance biplane wing Fig. 49. Cp Visualizations of the inner surfaces and Cp distributions at 50% semispan stations (not affected by Mach cones) of Case 1 and Case 2. flow croot ctip Reference area = 1 Fig. 50. CD and aspect ratio characteristics with changes of taper ratio (zero-lift conditions). Table 5 Geometric parameters of the selected wing planform as a baseline model. Parameters Conditions Taper ratio Aspect ratio Semi-span length Reference area Mid-chord line 0.25 5.12 1.6 1 Normal to the free-stream 5.4.1. Application of the 2-D designed airfoil The practical design of a 3-D biplane wing for high L/D at sufficient lift conditions (CL 40.1) is discussed in this section [30,32]. The inverse-problem method used for 2-D biplane designs in the previous section was also used. In this section, we discuss how to design both the upper and lower elements of the wing utilizing the inverse method at each span-station. The previously discussed 2-D designed airfoil is introduced in this case as the initial geometry of the wing section. The finally designed wing, therefore, will have different wing-section geometries at each span-station. Note that the planform of the biplane wing is fixed in this study. 5.4.2. 3-D inverse design 5.4.2.1. Design condition. For the inverse-design approach, an initial wing model is required. For the planform of the initial wing, the tapered wing shown in Table 5 was chosen. The reference wing area and its taper ratio are 1 and 0.25, respectively. The semi-span length and the aspect ratio of the wing are 1.6 and 5.12, respectively. For the geometries of wing sections of the initial wing, the 2-D designed biplane geometry (defined as ‘‘Designed Airfoil’’ in Section 4.2) was used. The number of nodes used for the simulations is approximately 1.10 million. Note that this wing, which we call the ‘‘Initial Wing’’, has values of CL ¼0.111, CD ¼0.00621 and L/D ¼17.9. As a reference, the aerodynamic performances of the ‘‘Designed Airfoil’’ and ‘‘Initial Wing’’ are tabulated in Table 7. 5.4.2.2. Design process. The inverse-design method discussed in Section 4 had been confirmed to work well for the 3-D design [58]. We then applied the method at ten span stations (every 10% of wing span from 0 to 90% of the wing). The design procedure shown in Fig. 52 was carried out using the specified (target) pressure distributions along both the upper and lower elements. First, design iterations were performed on the upper element until the obtained Cp distributions converged to the target (upper) Cp distributions, while the lower element wing configuration was kept fixed. Then, the lower element was designed, while the configuration of the newly designed upper element remained fixed. If the aerodynamic performance of the newly designed wing section exhibits no improvement over that of the initial wing section, geometry of the initial wing section will be used instead. 5.4.2.3. Analysis of the initial model. The ‘‘Initial Wing’’ was used as the initial model of the inverse-design process. Target pressure distributions required for this wing design will be discussed in the K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Busemann biplane without winglet 71 Busemann biplane with winglet Flow Flow Spanwise Cd distributions Fig. 51. Cp and mesh visualizations and Cd distributions of the tapered Busemann biplane wing with and without a winglet. Table 6 Drag coefficients of Busemann biplanes without winglet and with winglet at zerolift conditions. CD Without winglet With winglet 0.00300 0.00258 Table 7 Aerodynamic performance of 2-D ‘‘Designed Airfoil’’ and 3-D ‘‘Initial Wing’’. Designed airfoil Initial wing Lift coefficient Drag coefficient Lift-to-drag ratio 0.115 0.111 0.00531 0.00621 21.7 17.9 next section. Fig. 53 shows Cp visualizations of the inner surfaces and Cp distributions of the ‘‘Initial Wing’’. Let us focus on the Cp distributions along the inner surfaces (Cp distributions along the lower surface of the upper element and along the upper surface of the lower element). Compared to the Cp distributions of the ‘‘Designed Airfoil’’ (see Fig. 35 shown in Section 4), large pressure peaks near throats were recognized on both the upper and lower elements. Additionally, the values of the pressure coefficients in the areas not affected by the wing root (y/b of 50% to 80%) were greater than those in the areas that were affected (y/b of 0 to 40%). Note that the region affected by the wing root is confined inside the Mach cones generated from the wing root. Fig. 54 shows span-wise Cl, Cd and l/d distributions of the ‘‘Initial Wing’’. It can be observed that the areas affected by the Mach cones from the wing root and the wingtip are characterized by poor aerodynamic performance. However, those regions which are not affected by the Mach cones have a higher lift-to-drag coefficient than those of 2-D designed airfoil. These are typical characteristics of the 3-D tapered biplane wings as mentioned before. The goal of the design here is to generate a biplane wing geometry, which has better aerodynamic performance than the initial wing by specifying desirable Cp distributions (as target pressures) at each wing-span station. 5.4.2.4. Guideline for setting target pressure distributions. In order to prescribe target pressure distributions for our three-dimensional wing design, we employed the following two strategies. First we tried to remove the unnecessary pressure peaks occurred around the mid-chord (near the throat) areas in the baseline ‘‘Initial Wing’’. Second, we extend the ‘‘good’’ Cp distributions that are obtained from the mid-wing region of the ‘‘Initial Wing’’, into the regions near the wingtip and wing root. Target pressure distributions for the upper and lower elements used for the threedimensional wing design are shown in Figs. 55 and 56, respectively. 5.4.2.5. Design results. Let us now focus on the target Cp distributions and the obtained Cp distributions from the designed wings. As mentioned earlier, the upper element was first designed. 72 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 DesignDesign cyclecycle of each of eachelement element Inverse Design f f + f GridGeneration Generation Grid Target Target Cp Cpofof One OneElement Element NO Cp < Cp YES Target Cp Target Cp Current Current Cp Cp Flow Solver (Euler) Grid Generation Grid Generation at each span station InitialWing Wing Initial DesignedWing Wing Designed start goal Fig. 52. Design flow chart of one element of a three-dimensional biplane wing. Upper element 0 10% 20% 30% 40% 50% 60% 70% 80% 90% M Lower element 0 10% 20% 30% 40% 50% 60% 70% 80% 90% M Fig. 53. Cp visualizations of the inner surfaces and Cp distributions of the ‘‘Initial Wing’’. Details of the target Cp distributions of the upper element are shown in Fig. 55. Fig. 57 shows the obtained Cp distributions of the designed wing (after 14 iterations of the inverse-design cycle) and the Cp contours of the inner surfaces of the upper wing. This designed wing was termed the ‘‘Upper Designed Wing’’. The obtained Cp distributions successfully converged with the target distributions. CL, CD and L/D are 0.120, 0.00662 and 18.1, respectively. L/D was improved with the increase of CL. Fig. 58 shows the designed section geometries of the ‘‘Upper Designed Wing’’. Fig. 59 shows its span-wise Cl, Cd and l/d distributions. The geometries around the wing symmetry section were altered to have greater angles of attack. Span-wise Cl in the areas affected by Mach cones from the wing root increased without reductions of the span-wise l/d. Next, the lower element was designed. The Cp distributions of the lower element obtained after 14 iterations of the previously discussed upper-element design cycle, were used as the initial distributions on the lower-element wing. The initial and target Cp distributions of the lower element are shown in Fig. 56. As mentioned in the previous section, the purpose of the discrepancy between the initial and the target Cp distributions is to remove the pressure peaks at the mid-chords. Fig. 60 shows the obtained Cp distributions after 14 iterations and the Cp visualization of the inner surfaces of the designed biplane wing. The newly designed K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 73 wing was termed ‘‘Upper and Lower Designed Wing’’. It is clear that the pressure peaks were successfully removed. Unfortunately, the Cp distribution at the wing root did not converge to its target distributions. The designed wing has CL, CD and L/D of 0.122, 0.00664 and 18.3, respectively. Fig. 61 shows designed section geometries of the ‘‘Upper and Lower Designed Wing’’. Fig. 62 shows its span-wise Cl, Cd and l/d distributions. Although improvements were seen throughout most of the areas, the span-wise Cl and l/d at the wing root section showed no improvement over those of the ‘‘Initial Wing’’. We, therefore, conducted a further modification. The geometry at the wing root section of the lower element of the ‘‘Upper and Lowe Designed Wing’’ was replaced with that of the ‘‘Initial Wing’’. This wing was termed the ‘‘Final Wing’’. Fig. 63 shows span-wise Cl, Cd and l/d distributions, and Fig. 64 shows Cp visualizations of the inner surfaces of the ‘‘Final Wing’’. This wing maintained higher l/d than the ‘‘Initial Wing’’ at all span stations, and more lift was created in the areas affected by Mach cones originating from the wing root. The ‘‘Final Wing’’ has values of CL ¼0.125, CD ¼0.00678 and L/D ¼18.4. The 3-D designing was terminated at this point. 5.4.2.6. Comparison with other three-dimensional supersonic-biplane wings. Table 8 shows the aerodynamic performances of various three-dimensional tapered biplane wings. The designed biplane wings show the highest aerodynamic performance among the Busemann and Licher biplane wings. Fig. 65 shows a drag polar diagram of the biplane wings. Fig. 65 also includes the drag polar diagram of the 2-D zero-thickness single flat-plate airfoil. Note that the 2-D flat-plate airfoil is identical to the 3-D flat-plate wing with an infinite span that has only wave drag due to its lift. It is notable that the ‘‘Final Wing’’ having a sufficient thickness achieves lower wave drag than the 3-D zero-thickness flat-plate wing with an infinite span at CL 40.17 at its design Mach number 1.7. Fig. 54. Span-wise Cl, Cd and l/d distributions of the ‘‘Initial Wing’’. 0 10% 20% 30% 40% 50% 60% 70% 80% 90% 5.4.2.7. Final wing with winglet. The winglet discussed in the previous sections was applied to the ‘‘Final Wing’’. The lift-to-drag ratio was significantly improved from 18.4 to 19.6 at the identical lift coefficients of 0.125. Fig. 66 shows Cp contours of the inner surfaces of the ‘‘Final Wing with Winglet’’. Fig. 67 shows a drag polar curve of the ‘‘Final Wing with Winglet’’. The wing achieved lower drag than the single flat-plate airfoil at CL 40.16. 6. Wing–fuselage interference Fig. 55. Target Cp distributions of the upper element. 0 10% 20% 30% 40% 50% 60% 70% 80% 90% 0 Target 10% Target 20% Target 30% Target 40% Target 50% Target 60% Target 70% Target 80% Target 90% Target Fig. 56. Target Cp distributions of the lower element, and Cp distributions of the lower element of the ‘‘Upper Designed Wing’’ as initial Cp distributions. As a first step in working towards the designing a practical SST with biplane wings, the interference effects between the biplane wing and body (fuselage) were investigated. In this chapter, aerodynamic characteristics of wing–body configurations are simulated utilizing CFD and the aerodynamic performance of the biplane wings affected by those wing–body configurations is discussed [27–32]. 6.1. Wing–fuselage configuration A wing–body configuration shown in Fig. 68 (Refs. [27,28,31]) was adopted to investigate wave-interference effects from the body. The body has a conical configuration at the nose and a rectangular parallelepiped configuration in the rear. This body shape was chosen in order to generate strong shock waves and expansion waves. A biplane wing is attached to the body in a way that the disturbances from the body can influence the entire wing. The baseline wing configuration used in the study is a Busemann 74 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Fig. 57. Cp visualization of the inner surface, and target and obtained Cp distributions of the upper element of the ‘‘Upper Designed Wing’’. Fig. 59. Span-wise Cl, Cd and l/d distributions of the ‘‘Upper Designed Wing’’. Fig. 58. Designed section geometries of the ‘‘Upper Designed Wing’’. biplane wing with a tapered planform. Details of the geometries will later be presented in Fig. 70. Fig. 69 shows typical body effects on the biplane wing. Two types of supersonic-biplane wings are used for the wing– body configurations in this study. The configurations of these two biplane wings are identical except for the existence of winglets. The wing has the section shape of a Busemann biplane airfoil whose total thickness–chord ratio and gap-to-chord ratio between its two elements are 0.10 and 0.505, respectively. The wing has the tapered planform (discussed in the previous section) with a taper ratio of 0.25. The wing reference area is 1, and the aspect ratio is 5.12 with the mid-chord line being normal to the free-stream direction. Details of the wing parameters are shown in Fig. 70 and given in Table 9. In the study, four different wing locations relative to the body were investigated. Totally, there are eight types of wing–body configurations (without winglet (w/o wlt) and with winglet (w/wlt)). The overview of those four different wing locations is shown in Fig. 71. 6.2. Aerodynamic performance at cruise condition Fig. 72 shows a mesh visualization used for the inviscid flow analysis at the cruise Mach number of 1.7. The number of nodes of each wing–body configuration is about 2.0 million. Fig. 73 shows Cp contours of the body symmetry plane z ¼0 for each wing–body configuration. The cases of ‘‘w/wlt’’ are described in order to show shock and expansion waves generated from body. When the leading edge of the wing root is positioned at xw ¼3 or 3.5, the wings are strongly affected by both compression and expansion waves from the body. On the other hand, when xw ¼4 or 4.5, the wings are exposed only to the expansion waves. The wings w/o wlt are equally affected by those waves from the body. Fig. 74 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 M 75 0 10% 20% 30% 40% 50% 60% 70% 80% 90% 0 Target 10% Target 20% Target 30% Target 40% Target 50% Target 60% Target 70% Target 80% Target 90% Target Fig. 60. Cp visualization of the inner surface, and target and obtained Cp distributions of the lower element of the ‘‘Upper and Lower Designed Wing’’. Fig. 61. Designed section geometries of the ‘‘Upper and Lower Designed Wing’’. Fig. 62. Span-wise Cl, Cd and l/d distributions of the ‘‘Upper and Lower Designed Wing’’. shows drag polars calculated from the wings themselves of the wing–body configurations (w/o wlt and w/wlt) and of the wingalone (simple wing) cases. The details of the aerodynamic performance are tabulated in Tables 10 and 11. All the wing– body configuration cases with the exception of the case where xw ¼ 3 have better aerodynamic performance than the wingalone cases. Fig. 75 shows the surface Cp distributions at zero lift conditions (zero angle of attack) of the wing–body configurations w/o wlt and w/wlt. The Cp contours of those are along the inner surfaces of the biplane wings. For the instances where xw ¼4 and 4.5, in which the wings are affected only by the expansion waves, there is very little difference in aerodynamic characteristics between the w/o wlt and w/wlt cases. However, there are noticeable differences when xw ¼3 and 3.5, in which wings are affected by not only the expansion waves but also the compression waves. Flow choking occurs on the wing–body configuration with winglet of xw ¼3, where the wingtip region is widely exposed to the compression waves, and then thus causing high wave drag. On the other hand, for the configuration without a winglet of xw ¼3.5, the wing is less affected by those compression waves, and the high pressure regions near the wing tip causes the reduction of the wave drag of the wing. These phenomena can be explained by observing the reflection mechanism of the compression waves from the winglet. For the cases of without winglets, flow choking does not occur because the pressure increase caused by the reflection of the compression waves from the winglet does not exist. Drag reduction effects nar the wingtip are also not detected. 76 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Flat FlatPlate PlateAirfoil Airfoil (Theory) (Theory) Licher LicherBiplane Biplane Wing Wing 3D 3DDesigned Designed Wing Wing 0.22 Busemann Busemann Biplane Biplane Wing Wing 2D 2DDesigned Designed Wing 0.20 Lower Drag 0.18 0.16 CL 0.14 Required C L for Cruise 0.12 0.10 0.08 0.06 0.04 0.02 every 0.5deg plotted 0.00 0.000 0.002 0.004 0.006 0.008 0.010 0.012 0.014 CD Fig. 65. Drag polar curves of three-dimensional biplane wings and single flat-plate wing with an infinite span. Fig. 63. Span-wise Cl, Cd and l/d distributions of the ‘‘Final Wing’’. Fig. 66. Cp visualizations of the inner surfaces of the ‘‘Final Wing with Winglet’’. Cl Flat Plate (Theory) (t/c=0) Fig. 64. Cp visualizations of the inner surfaces of the ‘‘Final Wing’’. Table 8 Aerodynamic performance of three-dimensional biplane wings. Three-dimensional biplane wing CL CD L/D Busemann biplane wing (a ¼ 21) Licher biplane wing (a ¼1.51) Initial wing (a ffi11) Final wing (a ffi11) 0.110 0.107 0.111 0.125 0.00706 0.00642 0.00621 0.00678 15.6 16.7 17.9 18.4 In the cases of both w/wlt and w/o wlt at xw ¼4 and 4.5, at which point the wings are affected by only the expansion waves, flow chokings are not observed. Fig. 76 shows span-wise Cd distributions along the wing for both w/o wlt and w/wlt cases. In the cases w/o wlt at xw ¼3 and 3.5, we notice several high Cd regions, which are affected by the compression waves from the body, and low Cd regions, which are 0.22 0.20 0.18 0.16 0.14 0.12 0.10 0.08 0.06 0.04 0.02 0.00 0.000 0.002 0.004 0.006 Final Wing with Winglet 0.008 Cd 0.010 0.012 0.014 Fig. 67. Drag polar diagram of ‘‘Final Wing with Winglet’’. caused by the expansion waves. Similar trends are observed for the case w/wlt, with the exception of one unique characteristic for xw ¼3.5. The reflection of the compression waves from the winglet creates a thrust force (low drag) near the winglet. These same reflection waves are what cause the flow choking in the xw ¼3 case. In summary, for both cases w/o wlt and w/wlt, the areas affected by the compression waves will generate greater wave drag than the wing-alone cases, when there is reflection of the compression waves from the winglet. On the other hand, the areas affected by the expansion waves have better aerodynamic performance in all cases. Therefore, the configurations of xw ¼4 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 εn=tan-1 (0.125/0.5) 14.0[deg] εs=tan (0 1275/1 02) 7 1[deg] -1 Symmetry Plane 0.5C(root) 1.02C(root) Side view of Nose region 1.98C(root) Flow 2.5C(root) y 77 Table 9 Geometric parameters of wing planform. Parameters Conditions Taper ratio Aspect ratio Semi-span length Reference area Mid-chord line 0.25 5.12 1.6 1 Normal to the free-stream C(root) 0.505C(root) 0.2525C(root) x z Fig. 68. Wing–body configuration proposed by Odaka and Kusunose [27,28,31]. Fig. 71. Positions at which a wing is attached. Fig. 69. Cp visualization of a wing–body configuration proposed by Odaka and Kusunose at z ¼ 0 [27,28,31]. Flow C(root) S(ref) H(root) y ctip Fig. 72. Mesh visualization for analysis at cruise condition. H(tip) b/2 6.3. Application of designed wing to wing–fuselage combination x z Fig. 70. Configuration of tapered Busemann biplane wing. and xw ¼4.5 are recommended. Fig. 77 shows span-wise Cl, Cd and l/d distributions at an angle of attack of 21. It can be concluded the biplane wing is reasonably robust against disturbances generated by fuselage. Moreover, when the wing from the wing–body configuration is exposed to expansion waves, aerodynamic characteristics of the wing itself can be improved compared to those of the wing without the body (simple wing). In order to design practical flight carriers, the designed biplane wing discussed in the previous section was applied to a wing– body configuration. From the view point not only of aerodynamic performances at the cruise condition but also of avoiding the unstart problem near the cruise Mach number, the wing–body configuration w/wlt of xw ¼4 was selected. Under these conditions the designed biplane wing will be exposed only to expansion waves from the body. This wing–body configuration with the designed wing, defined as the ‘‘Final Wing with Winglet’’ in the previous section, will be referred to in this study as the ‘‘Final wing–body configuration’’. 78 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Fig. 73. Cp visualizations of the bodies with the winglets at z¼ 0 (zero angle of attacks). w/o wlt CL simple wing w/o wlt x=4 w/o wlt 0.22 0.20 0.18 0.16 0.14 0.12 0.10 0.08 0.06 0.04 0.02 0.00 0.000 0.004 0.002 x=3 w/o wlt x=4.5 w/o wlt 0.006 CD 0.008 Table 10 Aerodynamic performance of wings of the body without winglet. x=3.5 w/o wlt 0.010 0.012 0.014 w/ wlt CL simple wing w/ wlt 0.22 0.20 0.18 0.16 0.14 0.12 0.10 0.08 0.06 0.04 0.02 0.00 0.000 0.002 x=3.5 w/ wlt 0.004 0.006 CD x=4 w/ wlt 0.008 0.010 Fig. 74. Drag polar diagrams. x=4.5 w/ wlt 0.012 0.014 A.o.A 0 1 2 3 xw ¼ 3 CL CD L/D 0.0001 0.00397 0.0 0.0603 0.00511 11.8 0.1208 0.00852 14.2 0.1817 0.01426 12.7 xw ¼ 3.5 CL CD L/D 0.0000 0.00338 0.0 0.0587 0.00446 13.2 0.1173 0.00769 15.3 0.1758 0.01308 13.4 xw ¼ 4 CL CD L/D 0.0000 0.00313 0.0 0.0586 0.00421 13.9 0.1170 0.00743 15.7 0.1753 0.01280 13.7 xw ¼ 4.5 CL CD L/D 0.0000 0.00307 0.0 0.0582 0.00414 14.0 0.1163 0.00736 15.8 0.1747 0.01274 13.7 Simple wing CL CD L/D 0.0001 0.00326 0.0 0.0544 0.00425 12.8 0.1090 0.00725 15.0 0.1643 0.01229 13.4 At an angle of attack of 1.191, CL and CD were improved from 0.126 to 0.131 and from 0.00647 to 0.00631, respectively. L/D increased from 19.4 to 20.8. This value is very close to that of the 2-D designed biplane discussed in Section 4. Note that the lift-towave-drag ratio of the 2-D designed biplane is 21.7. Fig. 78 shows K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Table 11 Aerodynamic performance of wings of the body with winglet. A.o.A 0 xw ¼ 3 CL CD L/D 0.0002 0.02199 0.0 0.0630 0.02329 2.7 0.1263 0.02727 4.6 0.1904 0.03417 5.6 0.0001 0.00279 0.0 0.0591 0.00387 15.3 0.1180 0.00714 16.5 0.1769 0.01267 14.0 0.0001 0.00271 0.0 0.0584 0.00378 15.4 0.1167 0.00700 16.7 0.1750 0.01235 14.2 xw ¼ 4.5 CL CD L/D 0.0001 0.00274 0.0 0.0582 0.00381 15.3 0.1162 0.00702 16.6 0.1745 0.01238 14.1 Simple wing CL CD L/D 0.0001 0.00283 0.0 0.0543 0.00382 14.2 0.1089 0.00681 16.0 0.1642 0.01184 13.9 xw ¼ 3.5 CL CD L/D xw ¼ 4 CL CD L/D 1 2 3 79 the surface Cp contours of the ‘‘Final wing–body configuration’’. Fig. 79 shows drag polar diagrams and aerodynamic performance of the wing itself from ‘‘Final wing–body configuration’’ (designed X¼4 w/wlt) and the ‘‘Final Wing’’ without body (simple designed wing). The values of the aerodynamic performance of the wings are tabulated in Table 12. The wing from the ‘‘Final wing–body configuration’’ achieved the highest performance. In Fig. 79, a drag polar curve of the two-dimensional flat-plate airfoil obtained from the linear theory [8] is also included. In its aerodynamic performance, the wing of the ‘‘Final wing–body configuration’’ achieves the lowest drag at CL 40.14. Fig. 80 shows span-wise Cl, Cd and l/d distributions of the designed wing of the wing–body configuration and the designed wing itself (simple designed wing). The general trends in their aerodynamic characteristics are almost the same as those of the Busemann biplane wing shown in Fig. 76. It can be seen that the flow disturbances generated by a body will not deteriorate the performance of the biplane wing if the wing locations are carefully selected. Finally, the hinged slats and flaps were installed into the ‘‘Final wing–body configuration’’ to overcome the choked-flow problem at its off-design conditions [32]. Wing forms as well as its CL–MN, CD–MN curves are shown in Fig. 81. The hinged slats and flaps were equipped at the leading edge and trailing edge, respectively, along Fig. 75. (a) Surface Cp distributions of wing–body configurations and simple wing w/o wlt at zero lift conditions (zero angle of attacks). (b) Surface Cp distributions of wing– body configurations and simple wing w/wlt at zero lift conditions (zero angle of attacks). K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 w/o wlt 0.014 0.012 0.01 0.018 0.016 0.12 0.014 0.1 0.012 region affected by compression waves 0.08 0.01 0.06 0.008 Cd Cl 0.008 Cl , Cd w/o wlt 0.14 simple x=3 x=3 .5 x=4 x=4 .5 0.006 0.006 0.04 Cl simple Cl x=3.5 Cl=4.5 Cd x=3 Cd x=4 0.004 0.02 0.002 Cl x=3 Cl x=4 Cd simple Cd x=3.5 Cd x=4.5 0.004 0.002 0 0 0.2 0.4 0.6 0 0.8 1 1.2 1.4 0 1.6 1.4 1.6 y/croot 0 0.2 0.4 0.6 0.8 1 y/ croot 1.2 1.4 1.6 l/d w/o wlt 25 region affected by expansion waves 20 w/ wlt 0.008 15 l/d simple wing x=3.5 x=4 x=4.5 0.006 10 l / d simple l /d x=3 l/d x=3.5 l/d x=4 l/d x=4.5 5 0.004 Cd Cd 80 0 0.002 0 0.2 0.4 0.6 0.8 1 1.2 y/croot 0 w wlt Cl, Cd reflection effect of winglet - 0.004 0.2 0.4 0.6 0.8 1 1.2 1.4 1.6 y/ croot Fig. 76. Span-wise Cd distributions at zero-lift conditions (zero angle of attacks). 0.12 0.012 0.1 0.01 0.08 0.008 Cl 0 0.014 Cd - 0.002 0.14 0.06 0.006 Cl simple wing 0.04 0.02 Cl x= 4 Cl x=4.5 Cd simple Cd x=3.5 Cd x= 4 Cd x=4.5 0.004 0.002 0 0 0 0.2 0.4 0.6 0.8 1 1.2 1.4 1.6 1.2 1.4 1.6 y/croot w wlt 60 l/d l/d simple wing l/ d x=3.5 l/ d x= 4 l/ d x=4.5 50 40 l/d the wing span from 0.1 to 0.9. Fig. 82 shows surface Cp contours of the wing–body configurations equipped with the hinged slats near the starting Mach number [16,53]. It can be observed that when the Mach number is at 1.57 the detached shock waves around the wingtip are not swallowed due to the winglet effect. However, at the other span-wise locations the detached shock waves are already swallowed. It is clear that by installing hinged slats and flaps on to the wing–body configurations, choked-flow condition can be avoided at its design Mach number of 1.7. Cl x=3.5 30 20 7. Experiment 10 0 0 Shortly after A. Busemann proposed his supersonic-biplane concept in 1935 [7], experimental investigations began. In the early 1940s, fundamental experiments were already conducted by Ferri in order to measure aerodynamic forces acting on the Busemann biplanes near its design Mach number [14]. Based on his experiments he concluded that the supersonic biplane may, as predicted by theory, lead to notable advantages from the perspective of both reducing drag and increasing efficiency of the wing unit. He also observed flow choking phenomena and their related hysteresis problems by varying the wing gap. This operation was carried out by moving one of the wing elements parallel to itself. In this review paper we focus on the recent experimental investigations that are closely related to the current biplane developments. Supersonic and transonic flow fields around the Busemann biplane were examined by Kuratani et al. [34]. The purpose of this study was to demonstrate the shock-wave-interference and 0.2 0.4 0.6 0.8 1 y/croot Fig. 77. Span-wise Cl, Cd and l/d distributions at angle of attacks of 21. cancellation effects between the wing elements of the biplane. Wind-tunnel testing in supersonic and transonic flow regions was performed along with CFD analysis to investigate the flow characteristics around the 2-D supersonic-biplane model. Schlieren images and CFD analysis clarified the fundamental flow characteristics around the biplane at its design Mach number of 1.7. However, it was also observed in the transonic flow region that the supersonic biplane acted like a subsonic nozzle that was accompanied by choked flows. The experimental model for the ballistic range was designed and tested by Toyoda et al. [37] to examine the low-boom characteristic of the supersonic biplane. In their study winglets were attached to the ballistic-range models. Those winglets were designed with the help of CFD analysis in order to avoid the K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 81 Fig. 78. Surface Cp visualization of ‘‘Final wing–body configuration’’ at an angle of attack of 1.191. Flat Plate (Theory) simple wing w/ wlt simple designed wing x=4 w/ wlt designed x=4 w/ wlt 0.22 0.20 0.18 0.16 CL 0.14 Fig. 80. Span-wise Cl, Cd and l/d distributions of the wing of ‘‘Final wing–body configuration’’ and ‘‘Final Wing with Winglet’’. 0.12 0.10 0.08 0.06 0.04 0.02 0.00 0.000 0.002 0.004 0.006 0.008 0.010 0.012 0.014 CD Fig. 79. Drag polar curves of ‘‘Final Wing with Winglet’’ and the wing of ‘‘Final wing–body configuration’’. Table 12 Aerodynamic performance of the wings of ‘‘Final wing–body configuration’’ and ‘‘Final Wing with Winglet’’. A.o.A 0.19 0.69 1.19 1.69 2.19 2.69 Wing of ‘‘Final wing–body configuration’’ CL 0.0721 0.1017 0.1312 CD 0.00434 0.00506 0.00631 L/D 16.6 20.1 20.8 0.1607 0.00810 19.8 0.1900 0.01044 18.2 0.2192 0.01330 16.5 ‘‘Final wing with Winglet’’ 0.0710 0.0983 CL CD 0.00448 0.00523 L/D 15.8 18.8 0.1530 0.00821 18.6 0.1803 0.01046 17.2 0.2077 0.01323 15.7 0.1257 0.00647 19.4 un-start (choked flow) phenomena. As the CFD simulations indicated the designed experimental model was successfully launched without encountering the un-start problem. In their experiment, supersonic-biplane models were flown at a Mach number of 1.7 with zero angle of attack. The Schlieren images revealed presence of the shock-wave-cancellation mechanisms as expected from the CFD simulations. Using Pressure Sensitive Paint (PSP), Nagai et al. [36] measured the pressure distributions along the surfaces of the Busemann biplane in a small supersonic indraft wind tunnel. Due to the effects of the boundary layers developed along the wind-tunnel walls, the observed pressure distributions were considerably different from the values predicted through the simple 2-D supersonic thin-airfoil theory. They concluded that a further study was necessary to completely understand the interaction-flow mechanism of the 2-D Busemann biplane by carefully isolating the wind-tunnel wall effects. The low-speed aerodynamic characteristics of a baseline Busemann biplane (without high-lift devices) were investigated using experimental and CFD approaches by Kuratani et al. [35]. The purpose of the study was to analyze the fundamental low-speed aerodynamic and flow characteristics of the Busemann biplane. In this study, biplane stall characteristics were clarified. At the freestream velocity of 30 m/s, the Busemann airfoil stalled at an angle of attack of approximately 201. During the study, end plates were attached to a rectangular-shaped biplane wing to simulate 2-D flows. At high incidence angles, flow around the upper element of the biplane tended to separate earlier than that of the lower one. The stall of the lower element was suppressed due to the presence of the upper element and the total lift of the biplane system was, therefore, primarily generated by the lower element of the biplane. It was concluded that the fundamental aerodynamic characteristics of the Busemann biplane obtained through the wind-tunnel testing were in good agreement with those obtained from CFD analysis. At take-off and landing conditions, flow visualizations around the Busemann biplane airfoil equipped with leading-edge and tailingedge flaps (or slats and flaps) were performed by Kashitani et al. [38,39] in a low-speed smoke wind tunnel. The lift coefficient of the biplane airfoil was estimated by utilizing a method based on smokeline pattern measurements. With the help of hinged slats and flaps, the maximum lift coefficients of the Busemann biplane could reach to roughly 2.0 when the lift coefficient was normalized by using the baseline chord length of the wing element of the biplane. The drag coefficient was estimated by measuring the velocity defects in the 82 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 0.7 0.6 0.12 0.10 0.4 CD CL 0.5 0.3 0.08 0.06 0.2 0.04 0.1 0.02 0 Slat && Slat Flap Flap Slat Slat Simple Busemann (zero lift) Original Original 0.14 Slat && Slat Flap Flap Slat Slat Sufficient CL 0.5 1 1.5 0.00 0.5 2 1 1.5 M slat slat & flap 2.5 2 M 4deg original 1.19deg 0.8 1.0 M 1.19deg 1.57 1.7 Hysteresis of choking disappears Fig. 81. Wing form and CL–MN, CD–MN graphs. M ∞ =1.56 M ∞ =1. 57 M ∞ =1.60 Fig. 82. Surface Cp visualizations of ‘‘Final wing–body configuration’’ equipped with hinged slats near the starting Mach number. wake at a downstream station of the airfoil. The experimental data for the lift and drag coefficients at low speeds were in good agreement with the reference CFD data [6,32]. It was confirmed that the aerodynamic characteristics of the Busemann biplane equipped with slat and flap were similar to those of a conventional airfoil with high-lift devices. 8. Conclusions This paper reviewed the progresses on supersonic biplanes made by a group at Tohoku University since 2004 [1–6]. They have extended the classic Busemann biplane concept [7] to develop a practical supersonic biplane, that will generate sufficient lift at supersonic flights while making its shock-wave strength minimum. As a fundamental characterization the classic 2-D Busemann biplane and its extended biplane configurations such as the Licher biplane [10] were analyzed with CFD. The thickness–chord ratio of each element of those biplanes was approximately 0.05. The lift coefficient was set from 0.10 to 0.20 to generate sufficient lift at their cruise (design) Mach number of 1.7. It was confirmed that the Busemann biplane could eliminate almost all the wave drag caused by its airfoil thickness. At zero-lift condition wave drag generated was more than ninety percent smaller compared to that of a diamond airfoil, with the same thickness–chord ratio of 0.10 [6]. At a small angle of attack, the lift and wave drag of the Busemann biplane are identical to those of a flat-plate airfoil except for a small wave-drag penalty. This penalty is due to the entropy produced by shock waves, which exist between the biplane elements. The Licher biplane was able to further reduce lift-related wave drag by twothirds from that of the Busemann biplane. We then studied the aerodynamic characteristics of the Busemann biplane at off-design conditions. In the study flow choking and its concomitant hysteresis problems were found to occur at a wide range of free-stream Mach numbers, from 0.50 to 2.18, producing an unacceptable level of wave drag. As a countermeasure, hinged slats and flaps, which can also be used as high-lift devices during take-off and landing conditions, were utilized to control the area ratios of the inlet and the throat of biplanes. By applying these slats and flaps, the biplane was able to achieve the same level of wave drag (coefficient) as that of the diamond airfoil with equal thickness–chord ratio of 0.10, over a wide range of free-stream Mach numbers [20,22]. The Licher biplane was further improved by utilizing an inverse-design method with specified pressure distributions along the biplane surfaces [17,18]. The aerodynamic performance of the original Licher biplane was of Cl ¼ 0.0812, Cd ¼0.00449 with lift-to-(wave) drag ratio of 18.1 at an angle of attack of 11, based on Euler (inviscid) simulations. The designed biplane had a Cl of 0.115, Cd of 0.00531 and a lift-to-drag ratio of 21.7 at the same angle of attack. The designed biplane generated K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 less wave drag than the flat-plate airfoil at Cl 40.14 [18]. It is important to remember that the flat-plate airfoil has the lowest wave drag among the entire monoplane airfoils. The designed airfoil with slat and flap devices was also analyzed at flow conditions from take-off to cruise. It was confirmed that the designed airfoil with hinged slats and flaps could generate enough lift for its entire flying speeds, while nearly elminating flow choking and its related hysteresis problems [6,18]. To extend the previously discussed 2-D biplane to 3-D biplane wings, an additional study was conducted. For this study the tapered-wing planform with taper ratio of 0.25 and aspect ratio of 5.12 was selected [26,30]. Utilizing the previously discussed 2-D designed biplane configuration as the (initial) wing-section geometry of the tapered wing, the inverse design was simulated. The lift coefficient and lift-to-(wave) drag ratio of the designed wing were 0.125 and 18.4 (without viscous effect) at the angle of attack of approximately 11 [30,31]. In order to minimize pressure leaks at its wingtip regions, a winglet was introduced. When the winglet was applied to the designed wing, the lift-to(wave) drag ratio further improved to 19.6. For viscous flow analyses, the designed wing with the winglet achieved lift-to(total) drag ratios of 7.0–9.5 at a range of lift coefficients between 0.10 and 0.20 [32]. Flow choking and hysteresis also occurred for this designed wing. However the flow choking was milder than that of the 2-D designed biplane itself, and they were nearly eliminated, similar to the 2-D cases, with the use of slat and flap devices [30,32]. Finally, several wing–body configurations were simulated to investigate the wave-interference effects of the body on the tapered biplane wings [31,32]. In the study a body geometry that would generate strong shock waves at its nose region was selected to investigate the sensitivity of the biplane wing to nonuniform upstream flows. Preliminary parametric studies on the interference effects between the body and the supersonic-biplane wings were performed by choosing several different wing locations on the body. When the biplane wings were affected by compression waves from the body, the wings with winglets performed better than those without. It was, however, easier for flow choking to occur even with a small change in upstream flow condition when the biplane had winglets. When the wings were affected by expansion waves, the Mach number range at which flow choking would occur was reduced compared to that of the wing-alone (isolated wing) case. In general, supersonic-biplane wings performed better when they were exposed to expansion waves. The previously discussed designed wing with winglet was then applied to the wing–body configurations. Lift coefficient and lift-to-(wave) drag ratio of the wing were found to be 0.131 and 20.8, when the biplane wings were positioned in a region where they were exposed in the region of expansion waves. The aerodynamic performances of the designed biplane wing with body was able to approach those of the 2-D designed biplane by taking advantage of the interference effect of the body [32]. In summary, a 2-D designed supersonic biplane with approximately ten percent thickness–chord ratio can achieve lower wave drag better than a zero-thickness flat-plate airfoil at lift coefficient greater than 0.14. For both 2-D and 3-D biplane configurations, slats and flaps can be used to counteract flow choking that ocurs at off-design conditions. For wing–body configurations, when biplane wings are located in expansion-wave regions, the biplane wings perform better than the wing-alone configurations both at their design and at off-design conditions. These results indicate aerodynamic feasibility of supersonic biplanes for future supersonic transports. It is, however, clear that further fundamental studies incorporating viscous flow analysis to determine both biplane-wing and optimized fuselage geometries of these future transports are necessary. 83 Acknowledgments The authors are grateful for the help, information inputs and contributions provided by Professor S. Obayashi of Tohoku University, Professor A. Sasoh of Nagoya University, Dr. H. Yamashita, a post doctoral fellow of Tohoku University, Dr. M. Yonezawa of Honda R&D Co. Ltd, who finished the Ph.D course of Tohoku University, and Mr. Y. Utsumi, a Master course student of Tohoku University. We also would like to appreciate Ms. S. Tuchikado at University of Toyama for her efforts of supporting us to prepare the manuscript and Mr. J. Kusunose at the University of California at Davis for proofreading our manuscript. Appendix A A.1. Aerodynamic center Shock waves generated from a wing flying at supersonic speeds result in both of high wave drag and sonic-booms, which are disadvantageous for supersonic flight. In addition to these problems, issues concerning the shift in the wing’s aerodynamic center (A.C.) should be resolved for the practicality of supersonic flight. The authors would like to show some interesting results related to the A.C. of the biplane in this appendix. For considering a monoplane wing, the A.C. is at the 25% chord during subsonic flights (i.e. MN o1.0) and at the 50% chord during supersonic flights (i.e. MN 41.0). Therefore, the trim control of the supersonic airplanes becomes complex and difficult. In the case of the Concorde, eleven fuel tanks were equipped so that fuel could be transferred from tank to tank mid-flight. The fuel movement would accommodate for the change of the A.C. location due to change in speed. Thus, the shift of the A.C. location of a biplane should be investigated for a wide range of flight speeds. In this appendix, a two-dimensional analysis will be discussed [59,60]. A.1.1. Biplane and diamond airfoils To identify the A.C. location, CFD computation is applied to the models shown in Fig. 13 (Section 3.2). In order to compare the biplane’s A.C. with the monoplane’s, both the Busemann biplane and the diamond airfoil were numerically analyzed. Fig. A1 shows both airfoils and their coordinate axes. The chord length is 1.0, and the leading and trailing edge positions are 0.0 and 1.0, respectively. The airfoil thickness is 0.1; for the biplane, the thicknesses of both of upper and lower elements are 0.05, for a total thickness of 0.1. The distance between the upper and lower elements of the biplane is 0.505. This value was chosen to promote the favorable shock and expansion-wave interaction at the designed Mach number of 1.7 [60]. CFD flow simulations were conducted to obtain lift and pitching moment coefficients for the 27 cases for each of airfoil. Utilizing those lifts and pitching moments A.C. locations are Fig. A1. Airfoil models. 84 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Fig. A2. Surface Cp distributions along the chord. (For interpretation of the references to color in this figure legend, the reader is referred to the web version of this article). determined [59,60]. The 27 cases contain a subsonic range of Mach numbers from 0.2 to 0.6 and a supersonic range from 1.7 to 2.0 with angles of attack of 01, 11 and 21. To determine the A.C. location, we assumed that the A.C. would be located along the x-axis as shown in Fig. A1. From the study, it was found that the A.C. of biplanes remained within 25–27% chord at both in subsonic and in supersonic ranges. On the other hand, the A.C. location of diamond airfoils was 28% chord for flow speeds of Mach 0.2–0.6, and 44–45% chord for Mach 1.7–2.0. A.1.2. Relation between an A.C. location and load distributions Further investigation was conducted to relate the A.C. location and the load distribution (lift) along a chord axis. The surface Cp distributions are displayed with the airfoil geometry in Fig. A2. The figure includes four simulation cases: the diamond airfoil at the speed of MN ¼ 0.5 (a), the case of the biplane airfoil at the speed of MN ¼0.5 (b), the case of the diamond airfoil at the speed of MN ¼1.7 (c) and the case of the biplane airfoil at the speed of MN ¼1.7 (d). Each case has three different Cp distributions for the K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 85 Fig. A3. Load distributions along the chord. angle of attack of 01, 11 and 21, respectively. In the diagrams (a) and (c) of diamond airfoils the pink lines indicate the uppersurface Cps while the blue lines represents the lower surface Cps. In (b) and (d) of biplanes case the pink lines indicate the upperelement Cps while the blue ones indicate the lower element Cps. For the biplane cases, it can be seen that in subsonic flows, the lower element generates the lift whereas in supersonic flows, the upper element generates the lift. The load distributions along the chord were next studied. Using the Cp distributions, the load distribution graphs are produced and graphed in Fig. A3. Like Fig. A2, four different cases are compared here. Each case consists of their load distributions along the chord at angles of attack of 1.01 and 2.01 as well as their load variation distributions when the angle of attack increases from 0.01 to 1.01 and from 1.01 to 2.01. Focusing on the load variations, we note that in case (c) of diamond airfoil, the rear half of the airfoil generates lift, causing the A.C. location to shift to near 50% chord. However, in the other cases, the rear half of the airfoil does not generate lift, resulting in the A.C. location around 25% chord. 86 K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 Therefore, the A.C. of Busemann biplanes designed for MN ¼1.7 remains fairly constant, at 25% chord, both in subsonic flows of MN from 0.2 to 0.6 and in supersonic flows of MN from 1.7 to 2.0. References [1] Kusunose K, New A. Concept in the development of boomless supersonic transport. In: Proceedings of the first international conference on flow dynamics, Sendai, Japan, November 2004, p. 46–7. [2] Kusunose K, Matsushima K, Goto Y, Yamashita H, Yonezawa M, Maruyama D. et al. A fundamental study for the development of boomless supersonic transport aircraft. AIAA Paper, AIAA-2006-0654, January 2006. [3] Maruyama D, Matsushima K, Kusunose K, Nakahashi K. Aerodynamic design of biplane airfoils for low wave drag supersonic flight. AIAA Paper, AIAA2006-3323, June 2006. [4] Kusunose K, Matsushima KM, Maruyama D, Yamashita H, Yonezawa M. A study of 2-D supersonic biplanes for the development of nearly boomlesssupersonic transport. In: Proceedings of the 44th aircraft symposium, Omiya, Japan, October 2006 [in Japanese]. [5] Kusunose K, Matsushima K, Goto YM, Maruyama D, Yamashita H, Yonezawa M. A study in the supersonic biplane utilizing its shock wave cancellation effect. Journal of The Japan Society for Aeronautical and Space Sciences 2007;55(636):1–7. [in Japanese]. [6] Kusunose K, Matsushima K, Obayashi S, Furukawa T, Kuratani N, Goto Y, et al. Aerodynamic design of supersonic biplane, cutting edge and related topics. The 21st century COE Program. International COE of flow dynamics lecture series, vol. 5. Sendai: Tohoku University Press; 2007. [7] Busemann A. Aerodynamic lift at supersonic speeds. Luftfahrtforschung, Ed. 12, No. 6, October 3, 1935, p. 210–20. [8] Liepmann HW, Roshko A. Elements of gas dynamics. New York: John Wiley & Sons, Inc.; 1957 p. 107–123, 389. [9] Ferri A. Elements of aerodynamics of supersonic flows. New York: The Macmillan Company; 1949 (Also published from Dover Phoenix Edition, Dover Publications, Inc., New York, 2005), p. 154–160. [10] Licher RM. Optimum two-dimensional multiplanes in supersonic flow. Report No. SM-18688, Douglass Aircraft Co., 1955. [11] Japan Aerospace eXploration Agency (JAXA). Introduction to UPACS /http:// www.ista.jaxa.jp/res/c02/upacs/index.htmlS. [12] Nakahashi K, Ito Y, Togashi F. Some challenge of realistic flow simulations by unstructured grid CFD. International Journal for Numerical Methods in Fluids 2003;43:769–83. [13] Ito Y, Nakahashi K. Surface triangulation for polygonal models based on CFD data. International Journal for Numerical Methods in Fluids 2002;39(1): 75–96. [14] Ferri A. Experiments at supersonic speed on a biplane of the Busemann type. Translated by M. Flint, British R.T.P. Translation, No. 1407, Ministry of Aircraft Production, 1944, p. 1–40. [15] Furukawa T, Kumagai N, Oshiba S, Ogawa T, Saito K, Sasoh A. Measurement of pressure field near Busemann’s biplane in supersonic flow. In: Proceedings of the annual meeting and the seventh symposium on propulsion system for reusable launch vehicles, Northern Section of the Japan Society for Aeronautical and Space Sciences, vol. G-17, 2006 [in Japanese]. [16] Matsuo K. Compressive fluid dynamics. Rikogakusha, Tokyo, 1994, p. 70–96, 142–71 [in Japanese]. [17] Matsushima K, Kusunose K, Maruyama D, Matsuzawa T. Numerical design and assessment of a biplane as future supersonic transport. In: Proceedings of the 25th ICAS congress, ICAS 2006-3.7.1, 2006, p. 1–10. [18] Maruyama D, Kusunose K, Matsushima K. Aerodynamic characteristics of a two-dimensional supersonic biplane, covering its take-off to cruise conditions. International Journal on Shock Waves 2009;18(6):437–50. [19] Maruyama D, Matsushima K, Kusunose K, Nakashi K. Aerodynamic design of biplane airfoils for low wave dreg supersonic flight. In: Proceedings of the 24th AIAA applied aerodynamics conference, AIAA Paper, AIAA-2006-3323, 2006. [20] Maruyama D, Matsuzawa T, Kusunose K, Matsushima K, Nakahashi K. Consideration at off-design conditions of supersonic flows around biplane airfoils. In: Proceedings of the 45th AIAA aerospace sciences meeting and exhibit, AIAA Paper, AIAA-2007-0687, 2007. [21] Yamashita H, Yonezawa M, Obayashi S, Kusunose, K. A study of Busemanntype biplane for avoiding choked flow. AIAA Paper, AIAA 2007-0688, 2007. [22] Yamashita H, Obayashi S, Kusunose K. Reduction of drag penalty by means of plain flaps in boomless Busemann biplane. International Journal of Emerging. Multidisciplinary Fluid Sciences 2009;1(2):141–64. [23] Yamashita H, Obayashi S. Global variation of sonic boom intensity to seasonal atmospheric gradients. AIAA Paper, AIAA 2010-1389, 2010. [24] Yamashita H, Obayashi S. Sonic boom variability due to homogeneous atmospheric turbulence. Journal of Aircraft 2009;46(6):1886–93. [25] Yonezawa M, Yamashita H, Obayashi S, Kusunose K. Investigation of supersonic wing shape using Busemann airfoil. AIAA Paper, AIAA 20070688, 2007. [26] Yonezawa M, Obayashi S. Reducing drag penalty in the three-dimensional supersonic biplane. Journal of Aerospace Engineering 2009;223(7):891–9. [27] Odaka Y, Kusunose K. Interference effect of body on supersonic biplane wings. In: Proceedings of the 40th fluid dynamics conference/aerospace numerical simulation symposium, 2008, Paper 1B10, June 2008 [in Japanese]. [28] Odaka Y, Kusunose K. Effects of a bulky fuselage on aerodynamic characteristics of supersonic biplane wings. In: Proceedings of the 40th JSASS annual meeting, April 2009, p. 68–73 [in Japanese]. [29] Maruyama D, Nakahashi K, Kusunose K, Matsushima K. Aerodynamic design of supersonic biplane—toward efficient supersonic transport. In: Proceedings of the CEAS 2009, European Air and Space Conference, Manchester, UK, 2009. [30] Maruyama D, Matsushima K, Kusunose K, Nakahashi K. Three-dimensional aerodynamic design of low wave-drag supersonic biplane using inverse problem method. Journal of Aircraft 2009;46(6):1906–18. [31] Odaka Y, Kusunose K. A fundamental study for aerodynamic characteristics of supersonic biplane wing and wing–body configurations. Journal of Japan Society of Aeronautical Space Sciences 2009;57(664):217–24. [in Japanese]. [32] Maruyama D. Aerodynamic feasibility study of supersonic biplane. Dissertation for the degree of Doctor of Philosophy (Engineering), Aerospace Engineering, Tohoku University, July 2009. [33] Furukawa T, Kumagai N, Oshiba S, Ogawa T, Saito K, Sasoh A. Measurement of pressure field near Busemann’s biplane in supersonic flow. In: Proceedings of the annual meeting and the seventh symposium on propulsion system for reusable launch vehicles, Northern Section of the Japan Society for Aeronautical and Space Sciences, Sendai, Japan, March, 2006 [in Japanese]. [34] Kuratani N, Ogawa T, Yamashita H, Yonezawa M, Obayashi S. Experimental and computational fluid dynamics around supersonic biplane for sonic-boom reduction. AIAA Paper, AIAA 2007-3674, 2007. [35] Kuratani N, Ozaki S, Obayashi S, Orawa T, Matsuno T, Kawazoe H. Experimental and computational studies of low-speed aerodynamic performance and flow characteristics around a supersonic biplane. Transactions of the Japan Society for Aeronautical and Space Sciences 2009;52(176):89–97. [36] Nagai H, Oyama S, Ogawa T, Kuratani N, Asai K. Experimental study on interference flow of a supersonic biplane using pressure-sensitive paint technique. In: Proceedings of the ICAS 2008, 26th international congress of the aeronautical sciences ICAS/ATIO 2008, Anchorage, Alaska, USA, 2008, p. 1–9. [37] Toyoda A, Okubo M, Obayashi S, Shimizu K, Matsuda A, Sasoh A. Ballistic range experiment on the low sonic boom characteristics of supersonic biplane. AIAA Paper, AIAA 2010-0873, 2010. [38] Kashitani M, Yamaguchi Y, Kai Y Hirata K, Kusunose K. Preliminary study on lift coefficient of biplane airfoil in smoke wind tunnel. AIAA Paper, AIAA 2008-0349, 2008. [39] Kashitani M, Yamaguchi Y, Kai Y, Hirata K, Kusunose K. A study of the Busemann airfoil in smoke wind tunnel. Transactions of the Japan Society for Aeronautical and Space Sciences 2010;52(178):213–9. [40] Ferrari C. Recalling the Vth VOLTA congress: high speed in aviation. Annual Review of Fluid Mechanics 1996;28:1–9. [41] Busemann A. The relation between minimized drag and noise at supersonic speed. In: Proceedings of the Conference on high-speed aeronautics, Polytechnic Institute of Brooklyn, January 20–22, 1955, p. 133–44. [42] Walcher VO. Discussion on aerodynamic drag of wings at supersonic speed using biplane concept. Luftfahrtforshung 1937;14:55–62. [in German]. [43] Lighthill MJ. A note on supersonic biplane. British A.R.C. R.&M. No. 2002, 1944, p. 294–8. [44] Moeckel WE. Theoretical aerodynamic coefficients of two-dimensional supersonic biplane. NACA T.N. No. 1316, 1947. [45] Lomax H, Sluder L. A method for the calculation of wave drag on supersonicedge wing and biplane. NACA-TN no. 4232, 1958. [46] Bushnell DM. Shock wave drag reduction. Annual Review of Fluid Mechanics 2004;36:81–96. [47] Kulfan RM. Application of favorable aerodynamic interference to supersoinic airplane design. SAE Technical Paper Series 901988, 1990, p. 1–19. [48] Taylor RM. Variable wing position supersonic biplane. US Patent 4,405,102, US Navy, 1983. [49] Gerhardt HAA. Lifting shock wave cancellation module. US Patent 4,582,276, Northrop Corp., 1986. [50] White FM. Viscous fluid flow. 2nd ed. New York: McGraw-Hill, Inc.; 1991 p. 429–30. [51] Nakahashi K, Ito Y, Togashi F. Some challenge of realistic flow simulations by unstructured grid CFD. International Journal for Numerical Methods in Fluids 2003;43:769–83. [52] Ito Y, Nakahashi K. Surface triangulation for polygonal models based on CFD data. International Journal for Numerical Methods in Fluids 2002;39(1): 75–96. [53] Van Wie DM, Kwok FT, Walsh RF. Starting characteristics of supersonic inlets. In: 32nd AIAA/ASME/SAE/ASEE joint propulsion conference, AIAA 96-2914, July 1996. [54] Spalart PR, Allmaras SRA. One-equation turbulent model for aerodynamic flows. AIAA Paper, AIAA 92-0439, January 1992. [55] Ogoshi H, Shima E. Role of CFD in aeronautical engineering (15). Special Publication of National Aerospace Laboratory, SP-37. In: Proceedings of the 15th NAL symposium, Tokyo, Japan, June 1997, p. 81–6. [56] Matsushima K, Takanashi S. In: Fujii K, Dulikravich GS, editors. An inverse design method for wings using integral equations and its recent progress, Notes on numerical fluid mechanics, vol. 68. Wiesbaden: Vieweg & Sohn; 1999. p. 179–209. K. Kusunose et al. / Progress in Aerospace Sciences 47 (2011) 53–87 [57] Matsushima K, Maruyama D, Nakano T, Nakahashi K. Aerodynamic design of low boom and low drag supersonic transport using favorable wave interference. In: Proceeding of the 36th JSASS annual meeting, April 2005, p. 130–3 [in Japanese]. [58] Matsushima K, Maruyama D, Matsuzawa T. Numerical modeling for supersonic flow analysis and inverse design. In: Proceedings of the lectures and workshop international—recent advances in multidisciplinary technology and modeling, JAXA-SP-07-008E, 2008, p. 185–94. 87 [59] Noguchi R, Maruyama D, Matsushima K, Nakahashi K. Study of the aerodynamic center and aerodynamic characteristics of supersonic biplanes in a wide Mach number range. In: Proceedings of the 22nd CFD symposium by the Japan Society of Fluid Mechanics, vol. B6-1, December, 2008, p. 1–7 [in Japanese]. [60] Noguchi R. Study of aerodynamic center and low boom for realization of the next generation supersonic transport. Thesis for the degree of Master of Sciences (Aerospace Sciences), Department of Aerospace Engineering, Tohoku University, January 2010.
© Copyright 2025