Quick Review
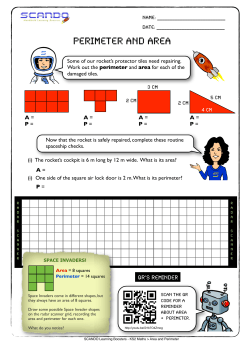
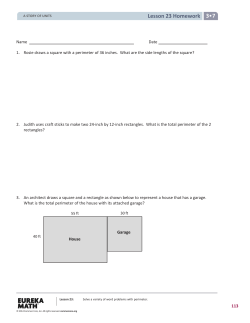
> We can find the perimeter of any polygon by adding the side lengths.
For this pentagon:
Perimeter = 4.0 + 1.5 + 2.0 + 2.5 + 2.0
= 12
4.0 cm
2.0 cm
1.5 cm
The perimeter is 12 cm. ' 2.0 cm^ ^ 2.5 cm
> We can use a formula to find the perimeter of some polygons.
Square Parallelogram Regular Hexagon
2 cm
2 cm
3 cm
P=SX4
P = (£ + w)X 2
P =2X4
P = (3 + 2) X 2
=8
= 5X2
= 10
The perimeters of the polygons are 8 cm, 10 cm, and 66 mm.
T&f TIdos®
1 o Find the perimeter of each polygon.
a)
b)
2.5 cm
io© HS- 2oB + 3o© 4- 3o@ = 14o3 23X 3 = 73
Tlh® periimeter ns 143 cmio Th® pdromdter is 7.5 cm.
©
3
—"i"
2
O
—h
fD
t-t.
—*
—.
3
CL
fD
3
vj
3 in
NJ
CL
fD
Q)
ID
i/T CD
cr fD
iQ
ID
5
f-t '^0
3
m
v
fD
T
CD
EL
CO
3
CD
n
in*
-t
ftT o O fD cr
¦~T
3s
fD fD
QJ
Q. n r-f1
m 3= fD C
m (D
tn
m 3 OL
fD
i-¥ £U
3
O
Q. fD
fD
fD
QJ
H CO
±Z U
O" CD
ET 5
OB
ST
m
Q
w
1&
&
E
w
m
0
&
E
IA
E
©
m
#ÿ
i
mi©
fD
\D
m
in
n>
O
—h
Q=
fD
<
CD
D"
n>
CD
rD
«o IQ^
a> CD
3
3' (D
n
fD rt
QJ
3
(D
-t
"D
in
3
3
Ul
O
^Sf
r+
3
0J
H
©
+
"-j ro
s^
o®
M3
¦u
r3 3
«o ^
ofD .
CB -o
^CLST
in
3
n
fD m
CD 3
fD
i-b»
D0 rp
1
CD
&
to
o
1 w
Ul
IM©
#
ftf
A
a
»
3
3
O
IT
®
^n®
^ D
^ ID
O 7+
fD
"o _
QJ
-a
a-
m
mm9
a
to
3
m
m
m
©
3
£5
„ o ro
A
m O^
©
ol
Q_
EL
O fD
|£
I?o
in
W
3 (D . ,
QJ €>
CD
3 ¦» s-
5 ¦o
| 0) q
in
•*o
3
CD
CD
in
e=&
Q
fD
fD
CD
Vi
XS
&
©
©
h$
x
S
ui
sr m
o
In
M
g*
CD
=3 o
CD
o
o
J*
o
o
o
n>
ol
i *
3a
31
^ ST
UD =ÿ
a 3"
3 fD
?
§
g o.
m§
^ — 1/1
<0 tn Q)
O ^3
© fD
m
o _
CD C _
o ¦a 3 aj
1!
3
yr
Io 5^
Hrt>^
II
b
+
M
•
w
+
a%
M
X
M
•
+
bJ
w
•
in
II
SI
3
n
b
+
b
KJ
in
+
KJ
+
M
b
in
SI
u
11
fD
(D
3
EHg
mil ®
¦u
•
b II
A
m
3 m
II
m
3
fD
EEM©
#
•
b
n
0®
3 o
00
II
©
ff.
3
A
-o
§9
CD
O
3
CQ
2=
r
"D
O
fD
m
fD
IP
fD
ZS
3s
fD
0=
3
oD
©
lp=t>
£=2
§§
TTli I
r
Exploring Rectangles
Quick Review
You can use formulas to find the perimeter
and the area of this rectangle.
25 mm
> P = (€ + w) X 2 ¦> A = (€ x w)
= (45 + 25) X 2 = (45 X 25)
= 70X2 =1125
45 mm
= 140
Perimeter = 140 mm Area = 1125 mm2
You can write the area in square centimetres:
Use: 1 mm = 0.1 cm
A= (45 = 10) X (25 = 10)
= 4.5 X 2.5
= 11.25
So, 1125 mm2 and 11.25 cm2 are equal areas.
Tir^ Tifesi
1 o Find the perimeter of each rectangle in centimetres and in millimetres.
Then find each area in square centimetres and in square millimetres.
Perimeter of A: 1§o4 gm ©r mm
Area of A: 14,.# cm2 ©r moirii2
A
Perimeter of B: f> em m 9@ mm
Area of B: 4oi sm2 or 4S@ BTnim2
4.2 cm
Perimeter of C: 1@.@ cm ©r 1@® mram
Area of C: cm2 @r 725 Bfim2
B
30 mm
C
2.9 cm
15 mm
©©
Pnefi©'
1 o The perimeter of a placemat is 1489 mm. How many centimetres is that?
Miofcmi 1 com = Mif 1@ = Miof)
2. Find the perimeter and area of each rectangle.
Record each measure in two different units.
si)
3.0 dm
3.3 km
2.0 km
4.1 dm
Sample hmwers
P = 1©oS km ®r 1© d©© m P = ICl dm ®r 142 cm
A - doi km2 m d d©© ©©© m2
A = 123 4m2 ®ir 123© em2
3o The area of a rectangular ballroom is 800 m2 and the perimeter is 120 m.
What are the dimensions of the ballroom? TGne ps iruii
2© m wM<g°„ 4© x 2© — i©@g (4© + 2@) x 2 = 12©
4o The area of a rug is 8000 cm2. How many square metres is that?
©o§ wA 1 rifi)2 = H ©© cm . 'il ©© CGTTJ = 1 © ©©© ecfan2; §@©© 1 © ©©© - ©3
Stretch four ThiiScIiig
The length of a rectangle is increased by 1 unit. Its width is decreased by 1 unit.
What happens to the area and the perimeter?
Use an example in your explanation.
Sampte Answer
The perimeter of this r<a€tancfe h 14 €TO
and its area is 12 cm2o
3 cm
(4 + 3) x 2 = 14; 4 X 3 = 12
New perimeter = 14 cm; S + 21 x 2 — 14
4 cm
New mm - 1© cm2g° S x 2 - 1©
Perimeter is the same and area dtecrgases.
2 cm
S cm
TP
/\ . " U(§u
Quick Review
,7
Pt
You can use a formula to find the volume of a rectangular prism.
The volume is the product of the prism's length, width, and height.
Volume = Length X Width X Height
This rectangular prism is 7.0 cm long,
3.5 cm wide, and 2.3 cm high.
Volume = 7,0 cm X 3.5 cm X 2.3 cm 2.3 cm
3.5 cm
= 24.5 cm2 X 2.3 cm 7.0 cm
= 56.35 cm3
The volume of the prism is 56.35 cm3.
Trv Tfcs®
1 o Find the volume of each rectangular prism,
a)
cm
0.5 cm
3.0 cm
4.0 cm
2.0 cm
cm3
1.2 cm
4 SEffi)3
1.5 cm
1.5 cm
1.5 cm
3o3T5 cm3
«fl)
1.5 cm
144 em3
102
3 cm3
1 o§ cm3
Praefi©'
1. Find the volume of each aquarium
a) rv=^ h)
1.0 m
60 cm
20 cm
50 cm
5.0 m
25 cm
40 cm
31 Si® em3
Dim3
©®© cm3
2. Work with a partner.
u) Find 4 small boxes. Label the boxes A, B, C, and D.
b) Measure the dimensions of each box. Estimate, then calculate,
each volume. Record your results in the table. Sample Answecrs
Estimated
W®lume
Actual
Volume
Box
Length
Width
A
13,5 cm
10,5 cm
<6.5 cm
75© cm3
921,4 cm3
B
9.5 cm
4.0 cm
12,5 cm
75© cm3
i3
C
cm
8.0 cm
6,5 em
75© cm3
728 cm3
D
10 cm
5 cm
10 cm
500 cm3
500 cm3
Mteight
3. Complete each table.
Lengtlh
(cm)
Width
6
9
3
8
5
4
5
(cm)
Widtlhi
(cm)
162
5.3
4.0
7.1
. J.52
2
80
6.0
3.2
5
96
3
4
48
10
2.0
1.1
22
5
5
125
12.0
2.5
4.0
120
(cm)
Height Volume
(cm3)
(cm)
Lengtslh
Height Volume
(cm3)
(cm)
Streteii T®ir Tiiiilciei|
Jocelyn built a rectangular prism with 36 centimetre cubes.
What might be the dimensions of the prism? Give as many answers as you can.
1 x 1 x P x 2 x li° n x 3 x 12g 1 x 4 x f)B°
1 x § X (§; 2X2X9; 1 X 3 X 3X3X4
coro
/W
The Cubic Metre
.OAs %
fe"
Quick Review
A line segment has only one dimension.
It is measured using linear units such as centimetres and metres.
A flat surface has two dimensions.
The area it covers is measured using square units such as square
centimetres or square metres.
An object has three dimensions.
The space it occupies is measured using cubic units such
as cubic centimetres or cubic metres.
A cube with edge length 1 m has volume
one cubic metre, or 1 m3.
1 m3 = 100 cm X 100 cm X 100 cm
= 1 000 000 cm3
1m
1m
1m
To find the volume of a rectangular prism in cubic metres, we find
the product of the length, width, and height in metres.
Volume = 3.0 m x 2.0 m X 2.5 m
= 6.0 m2 X 2 5 m
2.5 m
= 15.0 m3
2.0 m
The volume of this prism is 15 m3. 3 g m
Tcf ¥Gi@$(s
1 c Would you use a linear, square, or cubic unit to measure?
u) area of a desk pad square yroit b) space in an elevator cubic unit
c) length of a pen iliBiear unit d) perimeter of a dog run linear unit
104
n
OX
mo
SVJ U*
x 5*
iq
O
m
¦o
cr
o
X
c
i-n
#
u
i
A
©
A
»
m
&
o
&
m
u»
3
O
C
Q>
D"
>
IjU
in
in
s
W
c
(#1
fH
01
*®
0
0*
m
3
A
E1m3©
01
3
m
rD
QJ
o
Ul
m
01
0
01
#
<
1L
3
01
m
3
fD
f-f
rD
^
i/)
ro
3
m
i
"E
rD
3
^:a
o
m
m
<
o
c
A
fD*
5"
o
SVJ
ro
3
Q)
3w
o\
3
w
3
m
m
In
3
3
b
o\
3
b
UJ
In
O
§5
<
in
rD
ro
c-S*
3
91
pT
n
c
5°
O
CT
x
n
3"
QJ
rD
O
rD
3
o
£
m
O,
3
c=^
rm d
Q