How to Suppress Spurious
Signals in Oscillator Design
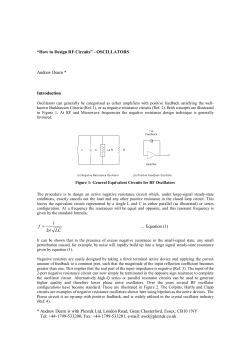
by
Norbert H.L. Koster
Bettina J. Koster
Adalbert Beyer, Fellow IEEE
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
1
Overview
Introduction
The Oscillator Circuit
CAD of the Oscillator
Experiments on the Oscillator
Spectral Lines
Improving of the Circuit and Results
Discussion
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
2
1
Introduction
A technique entitled
Fast Approximation Formulas Describing
the Non-linear Intrinsic Transistor
Equivalent Circuit Elements Speed Up and
Improve the CAD of Oscillator Circuits
was introduced in frame of the 2003 IEEE MTT
IMS workshop marked by WSI in Philadelphia.
That contribution has treated an important topic
of oscillator design.
During the last three years, new developments
on this field justify a continuation of the
presentation mentioned above.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
3
Introduction
Some Facts:
Unwanted spurious signals appear in the
oscillator’s spectrum when the oscillator circuit
produces at least one additional signal than
only the designed one.
These additional signals interfere with the
projected oscillation frequency or with its
harmonics and produce a number of disturbing
spurious signals.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
4
2
Introduction
What is to do?
To avoid this problem it is necessary to analyze
the reason for these additional oscillations and
to take such measures as inhibiting the
oscillator’ s circuit capability to produce
additional parasitic oscillations.
This presentation examines the problem of
exciting spurious signal in an oscillator circuit
and demonstrates, how an oscillator can be
CAD designed and assembled, producing the
desired frequency signal, only.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
5
The Oscillator Circuit
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
6
3
CAD of the Oscillator
In order to analyze the oscillator circuit, we use a program
package from Stephen A. Maas and Arthur Nichols entitled
„C/NL 2 for Windows 95, NTT and 3.1: Version 1.2 – Linear
and Nonlinear Circuit Analysis and Optimization, Artech
House Publishers, London, 1996. – ISBN 0-89006-899-2.”
Although, this CAD tool is very compact and easy to use, it
allows a very fast and effective implementation of all the
necessary equations to calculate the voltage-dependent
values of the intrinsic elements of both the JFETs during the
tuning and analyzing sequence.
Additionally, it is possible to insert easily a sufficient number
of own equations directly into the CAD program.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
7
CAD of the Oscillator
The program uses nodal analysis similar to SPICE.
The first task is to determine the variation of smallsignal parameters of the active elements as a
function of dc operating (quiescent) point.
Furthermore, it is necessary to predict the feasibility
for oscillation.
These problems were solved in the Philadelphia talk
by using simple algebraic expressions for
calculating those quantities.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
8
4
CAD of the Oscillator
DC Analysis (Source Coupled JFETs)
VC
RD
Id1
T1
Vs = RS (I d1 + I d2 )
Vr
Id2
T2
Vgs1 = Vgs2 = Vs
Vgs2
Vds1 = Vc RS (I d1 + I d2 )
Vds1 Vds2
Vgs1
VS
Vds2 = Vc RS (I d1 + I d2 ) Rd I d2
RS
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
9
CAD of the Oscillator
Drain-Source-Voltage of JFET 1
5
V
V
f
f
ds1 = 0.9613 c 1.0246 (1 e 01 )(1 e 02 ) V V
V
R
with the quantities f = 0.0303 S f02 = 1.385 VC
01
4
Rs
10
3
Id1
T1
Id2
Ur
T2
Uds1 Uds2
Ugs1
Ugs2
Us
15
22
Uds1 / V
Rd
Ub
33
47
56
68
2
82
100
1
RS
0
1
2
3
4
5
Ub / V
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
10
5
CAD of the Oscillator
Small Signal Equivalent Circuit of a JFET
CPGD
LG
1
RG
Cgd
g
G
ugs
i ds
Cgs
ZL e , e , l e
RD
d
Cds
ZL a , a , l a
s
CPG
2
D
Rds
Rgs
LD
CPD
RS
i ds = ugs gm e-j LS
1'
2'
S
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
11
CAD of the Oscillator
Drain-Source-Capacitor
C ds
fF
= 285,732 (1 e f13 ) 31,60106
Cgd
g
ugs
d
abbreviation:
i ds
Cgs
Cds
Rgs
Rds
s
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
i ds = ugs gm e-j f13 =
1,1569
U gs
V
Uni Gran Canaria, Las Palmas, 2007
+ 1,483
U ds
V
12
6
CAD of the Oscillator
Drain-Source-Capacitor
ugs
Cgd
d
i ds
Cgs
Cds
Rgs
Rds
s
200
Ugs / V
0,0
-0,2
-0,4
-0,6
Cds / fF
g
300
-0,8
-1,0
-1,2
-1,4
100
i ds = ugs gm e-j 0
0
1
2
3
4
5
Uds / V
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
13
CAD of the Oscillator
Remember, the JFETs used as active devices in
this oscillator are the type of
CFY30
from INFINEON Technologies AG.
From there, all results given in this presentation
refers solely to this type of JFET, but may be similar
if comparable types are used.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
14
7
CAD of the Oscillator
The simulated magnitude of the complex Sparameter s11 is considered for a wide
frequency range from dc up to 10 GHz.
The analysis of the oscillator circuit gives
some hints (parasitic elements) for additional
oscillations in the upper GHz region.
This seems to be the reason for the spurious
oscillation. Next view graph shows the
calculated course of the magnitude of the
reflection coefficient s11 from dc to 10 GHz.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
15
CAD of the Oscillator
(Simulation of s11 at 4.0 Vdc Biasing)
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
16
8
Experiments on the Oscillator Circuit
The Measurement Set Up
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
17
Experiments on the Oscillator Circuit
The Measurement at a Voltage of 3.9 VDC
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
18
9
Experiments on the Oscillator Circuit
The Measurement at a Voltage of 3.9 VDC
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
19
Spectral Lines
To give some explanation for the number of the
resulting spectral lines, we will regard the simple
mixture of two sinusoidal voltages v1(t) and v2(t)
with different frequencies f1 and f2.
The non-linear context between the current i(t)
and the signal voltage v(t) at an active element
can be described approximately by a polynomial
with the order of n = N
n= N
i (t ) = k n [v(t )]n .
n =1
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
20
10
Spectral Lines
If the signal voltage v(t) is a superposition of two
sinusoidal voltages with different frequencies and
different amplitudes, it is valid:
i =2
v(t ) = vi (t )
with
i =1
vi = vˆ sin (2f i t ) for i = 1, 2 .
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
21
Spectral Lines
After a little algebraic manipulation a result for the signal voltage
v(t) having the following components may be obtained:
1) the two frequencies f1 and f2,
2) the harmonics 2 f1 , 3 f1, 4 f1, 2 f2, 3 f2 and 4 f2,
3) the mixtures of second grade f1 ± f2
4) the mixtures of third grade 2 f1 ± f2 and f1 ± 2 f2
and
6) the mixtures of fourth grade 3 f1 ± f2, 2 f1 ± 2 f2, f1 ± 3 f2.
These are already the resulting frequencies assuming N = 4.
More realistic is an amount of N of above 18,
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
22
11
Improving of the Circuit and
Results
in MHz
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
23
Improving of the Circuit and
Results
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
24
12
Improving of the Circuit and
Results
in MHz
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
25
Improving of the Circuit and
Results
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
26
13
Improving of the Circuit and
Results
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
27
Discussion
This paper has been shown that a number of additional
signals can be excited by the oscillator’s active kernel due
to parasitic effects. These interfere with the projected
oscillation frequency or with its harmonics and produce a
number of disturbing spurious signals.
By means of a suitable CAD program, such as C/NL2, it
is possible to avoid this problem. The CAD tool can analyze
the reason for these additional oscillations and comfortably
give advice how to take measures in order to inhibit the
oscillator’s circuit capability to produce these unwanted
additional parasitic oscillations.
This talk examined the problem of exciting spurious
signals in an oscillator circuit and proves that an oscillator
can be easily CAD designed and assembled, producing the
designed frequency signal, only.
Prof. Dr.-Ing. A. Beyer
Uni Duisburg-Essen
Uni Gran Canaria, Las Palmas, 2007
28
14