Math 101 Sec S13N01 - Sample Test 3
2013
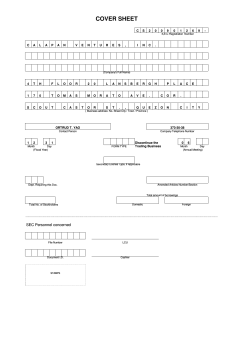
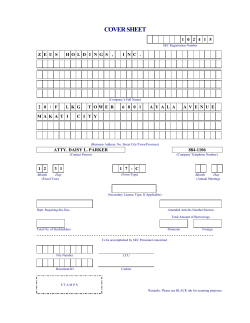
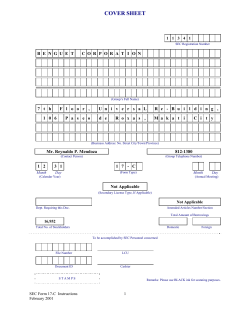
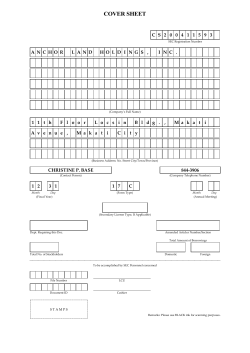
Question 1 Determine if the following series converge or diverge. State reasons for your conclusions.
P∞
arctan(n)
P∞
2π −n
P∞
(−1)n+1
√
n−2
n=0
n=0
n=4
P∞
√
n
n=1 n3 +1
Math 101 Sec S13N01 - Sample Test 3
2013
P∞ 2n
n=1 n!
Question 2 A 5-lb monkey is attached to the end of a 30-ft hanging rope that weighs 0.2 lb/ft.
The monkey climbs the rope to the top. How much work has it done?
Math 101 Sec S13N01 - Sample Test 3
2013
Question 3 Compute the volume generated by revolving x2 + (y − 2)2 = 1 about the x-axis. This
yields the volume of a doughnut.
Math 101 Sec S13N01 - Sample Test 3
2013
Question 4 Find the arc length of the curve x2/3 + y 2/3 = 4 from x = 1 to x = 8.
Math 101 Sec S13N01 - Sample Test 3
2013
Question 5:
The fish population in a lake is attacked by a disease at time t = 0,
with the result that the size P(t) of the population at time t
√
dP
= −k P
dt
where k is a positive constant. If there were initially 90,000 fish in the lake, and 40,000 were left
after 6 weeks, when will the fish population be reduced to 10,000?
Math 101 Sec S13N01 - Sample Test 3
2013
√
Question 6 Find first five terms of the Maclaurin series for f (x) = x 1 + x2 .