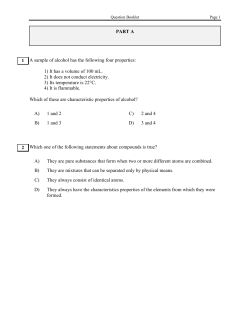
S SAMPLE QUESTION BOOKLET New Zealand Scholarship Calculus Time allowed: Three hours Total marks: 40 Question Booklet There are SIX questions in this booklet. Answer any FIVE of the six questions. Write your answers in the Answer Booklet. Use the Formulae and Tables Booklet provided. Show ALL working. Start your answer to each question on a new page. Carefully number each question. Answers developed using a CAS calculator require ALL commands to be shown. Correct answers only will not be sufficient. Check that this booklet has pages 2 –8 in the correct order and that none of these pages is blank. You may keep this booklet at the end of the examination. © New Zealand Qualifications Authority, 2012. All rights reserved. No part of this publication may be reproduced by any means without the prior permission of the New Zealand Qualifications Authority. 2 You have three hours to complete this examination. QUESTION ONE (a) Find all real solutions of ae x 2e x − 1 < 1 , where a is a positive constant. (b) A three-dimensional solid has a surface made of two types of triangles, types A and B. The area of a Type A triangle is AA = 2 k 2 − 2 k + 1 . The area of a Type B triangle is AB = ( ) 3 2 k − k +1 . 2 Find the value of k which minimises the total surface area, and show that this value gives a minimum. (c) Find all functions y = f (x) which satisfy Show that these functions also satisfy dy = y m+1 , where m is a non-zero constant. dx ( ) d n y = ny n + m . dx 3 QUESTION TWO (a) A circular pond is 5 metres in radius. The volume (in cubic metres) of water in the pond when the water is x metres from the top is V ( x ) = π 250π − 25π x + x 3 . 3 3 Rain falls at the rate of 15 millimetres per hour. How fast is the depth of water in the pond rising when it is 3 metres from the top? (b) Figure 1 below shows the function g ( x ) = 1 − x as a solid line. 1 0.8 0.6 y 0.4 0.2 0 0 0.4 x 0.6 0.8 Figure 1: Graph of y = g(x), and the curve rotated about the point 0.2 (i) Use differentiation to show that and find the value of A. ( g(x)dx = A 1 x 1 ( , ). 1 1 2 2 1.5 ) (2 + 3 x ) + C , (ii) Figure 1 shows the curve y = g(x) rotated 180 degrees around the point The two curves intersect only at the points (0,1) and (1,0). Find the area of the region enclosed by the two curves. ( , ) as a dotted line. 1 1 2 2 4 QUESTION THREE (a) Make use of trigonometric identities to find the exact value of cos 7 . 12 (b) Two half-angle formulae for trigonometry are given below. cos sin 2 =± 1+ cos 2 2 =± 1 cos 2 Given that tan = 20 6 and 0 < < , find an exact value of tan . 2 4 Simplify your answer. (c) Write half a page on the following, including diagrams or examples where appropriate. Describe the limitations of the second derivative test for maxima and minima of continuous functions. 5 QUESTION four (a) The gamma function Γ extends the factorial function to all of the real numbers except for the negative integers and zero. It takes values of the factorial function at positive integers: Γ(n + 1) = n! and has the property that for any values, Γ(x) = (x – 1)Γ(x – 1). A formula useful for finding other values of the gamma function is Euler’s reflection formula: π Γ ( z )Γ (1− z ) = . sin(π z ) Find the exact value of Γ ( ) , and hence the exact value of Γ ( ) . 5 2 1 2 (b) Consider the equation x6 + (2 – k)x4 + (25 – 2k)x2 – 25k = 0, where k is a real constant. Given that c = 2 + 3 i is a root of this equation, find the other five roots. (c) The vertices of a regular octagon, shown on the Argand diagram in Figure 2, represent the roots of a complex degree 8 polynomial. One root is shown at the point A, 1 + 0i. Write the polynomial in the form p(z) = (z – (a + bi))n + q. Im A (1 + 0i) Re Figure 2: Roots of a polynomial on an Argand diagram, including the point A, 1 + 0i. 6 question five (a) Find the positive values of k for which the curves y = k (5 x 2 − 9) and y = x 4 − x 2 meet at a common tangent. (b) Answer ONE of the following options. EITHER Consider the ellipse shown in Figure 3. It has the equation and is shown along with the line x + 2 y = 0 . Figure 3: A conic section and line. By making the substitution u = x + 2 y and an appropriate substitution for another variable v, write the conic section in the form u 2 v2 + =1 . c2 d 2 Hence, or otherwise, find the lengths of the major and minor axes of the ellipse. OR Figure 5 shows a completed critical path analysis of a construction project consisting of twelve related tasks. The task duration (TD) is shown in days at the top, with the estimated start time (EST) beneath. Only the two tasks in the centre (grey boxes) are sub-critical, and their float times are shown in brackets. Form a plan for the project manager to reduce the project completion time, with reasons for your choices. Write around half a page. 7 Figure 4: Completed critical path analysis of a construction project, with times in days. 8 question six (a) An elliptical frame is built with two equally bright light bulbs at the focal points of the ellipse, as shown in Figure 5. Figure 5: Elliptical frame with bulbs at focal points. At any point on the frame, the sum of the distances to the bulbs is a constant d. The brightness of a bulb at the frame is inversely proportional to the square of the distance to the bulb. An expression for the brightness at a point on the frame distance x from the left bulb is B(x) = I 1 1 + , where I and d are constants. x 2 (d x)2 Find the least brightly lit points on the frame, giving reasons for your answers. (b) Answer ONE of the following options. EITHER The system of linear equations below relates complex variables x, y and z. The variable k is a complex constant. Find the value or values of k for which the system of equations has no solution. OR There are three ways to score points in rugby: a converted try (7 points), an unconverted try (5 points) and a penalty or drop kick (3 points). If a team scores M converted tries, N unconverted tries and P penalties and drop kicks, then their score is S = 7 M + 5 N + 3P . If (m, n, p ) represents one way to score S points, then all ways to score S points are of the form M = m + 2x − y N = n − x + 2y P = p − 3x − y where x and y are integers. Use linear programming to find all ways to score 32 points.