1. No category

CHAB
Chapter Homework Assignment
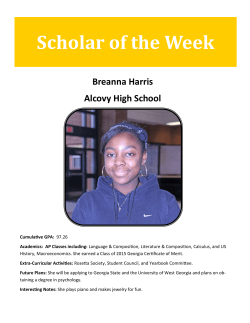
S.Hogan
Name: ____________________________________
CHAB AP2 HW
Due on Monday 4/27/15 by 5:45PM.
Directions: Use this sheet as a cover sheet for your homework assignment. If
you do not staple this cover sheet to the front of your assignment, you will receive
a zero. You may submit your HW early but NO LATE HW will be accepted.
Assignment:
EXAM
2011
2010 (Form B)
2007
2006 (Form B)
2004
1997 MC
NUMBER OF
PROBLEMS
6 problems
6 problems
6 problems
6 problems
6 problems
40 problems
Grading:
Item
Possible Points
All Problems Completed
2
Problems Labeled and in Numerical Order,
Page Numbers are Labeled
First Random Problem (correct with sufficient work)
2
Second Random Problem (correct with sufficient work)
4
Third Random Problem (correct with sufficient work)
4
Fourth Random Problem (correct with sufficient work)
4
TOTAL
20
4
Score
2011 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part A
Time— 30 minutes
Number of problems—2
A graphing calculator is required for these problems.
1. For 0 … t … 6, a particle is moving along the x-axis. The particle’s position, x t , is not explicitly given.
The velocity of the particle is given by vt a t 1 t
e
2
4
cos et
4
and x0
4 1. The acceleration of the particle is given by
2sin et
2.
(a) Is the speed of the particle increasing or decreasing at time t
5.5 ? Give a reason for your answer.
(b) Find the average velocity of the particle for the time period 0 … t … 6.
(c) Find the total distance traveled by the particle from time t
0 to t
6.
(d) For 0 … t … 6, the particle changes direction exactly once. Find the position of the particle at that time.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2011 The College Board.
Visit the College Board on the Web: www.collegeboard.org.
GO ON TO THE NEXT PAGE.
-2-
2011 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
t
(minutes)
0
2
5
9
10
H t (degrees Celsius)
66
60
52
44
43
2. As a pot of tea cools, the temperature of the tea is modeled by a differentiable function H for 0 … t … 10, where
time t is measured in minutes and temperature H t is measured in degrees Celsius. Values of H t at selected
values of time t are shown in the table above.
(a) Use the data in the table to approximate the rate at which the temperature of the tea is changing at time
t 3.5. Show the computations that lead to your answer.
1 10
H t dt in the context of this problem. Use a trapezoidal
10 Ô0
1 10
H t dt.
sum with the four subintervals indicated by the table to estimate
10 Ô0
(b) Using correct units, explain the meaning of
(c) Evaluate
10
Ô0
H „t dt. Using correct units, explain the meaning of the expression in the context of this
problem.
(d) At time t 0, biscuits with temperature 100’C were removed from an oven. The temperature of the
biscuits at time t is modeled by a differentiable function B for which it is known that
B „t 13.84e 0.173t . Using the given models, at time t
the tea?
10, how much cooler are the biscuits than
WRITE ALL WORK IN THE EXAM BOOKLET.
END OF PART A OF SECTION II
© 2011 The College Board.
Visit the College Board on the Web: www.collegeboard.org.
GO ON TO THE NEXT PAGE.
-3-
2011 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part B
Time— 60 minutes
Number of problems— 4
No calculator is allowed for these problems.
3. Let R be the region in the first quadrant enclosed by the graphs of f x in the figure above.
(a) Write an equation for the line tangent to the graph of f at x
8 x 3 and g x sin ! x , as shown
1
.
2
(b) Find the area of R.
(c) Write, but do not evaluate, an integral expression for the volume of the solid generated when R is rotated
about the horizontal line y 1.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2011 The College Board.
Visit the College Board on the Web: www.collegeboard.org.
GO ON TO THE NEXT PAGE.
-4-
2011 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
4. The continuous function f is defined on the interval 4 … x … 3. The graph of f consists of two quarter circles
and one line segment, as shown in the figure above. Let g x 2x x
Ô0 f t dt.
(a) Find g 3 . Find g „ x and evaluate g „ 3 .
(b) Determine the x-coordinate of the point at which g has an absolute maximum on the interval 4 … x … 3.
Justify your answer.
(c) Find all values of x on the interval 4 x 3 for which the graph of g has a point of inflection. Give a
reason for your answer.
(d) Find the average rate of change of f on the interval 4 … x … 3. There is no point c, 4 c 3, for
which f „c is equal to that average rate of change. Explain why this statement does not contradict the
Mean Value Theorem.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2011 The College Board.
Visit the College Board on the Web: www.collegeboard.org.
GO ON TO THE NEXT PAGE.
-5-
2011 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
5. At the beginning of 2010, a landfill contained 1400 tons of solid waste. The increasing function W models
the total amount of solid waste stored at the landfill. Planners estimate that W will satisfy the differential
dW
1
equation
W 300 for the next 20 years. W is measured in tons, and t is measured in years from
dt
25
the start of 2010.
0 to approximate the amount of solid waste that the landfill
1
).
contains at the end of the first 3 months of 2010 (time t
4
(a) Use the line tangent to the graph of W at t
d 2W
d 2W
in terms of W. Use
to determine whether your answer in part (a) is an underestimate or
2
dt
dt 2
1
.
an overestimate of the amount of solid waste that the landfill contains at time t
4
(b) Find
(c) Find the particular solution W
condition W 0 W t to the differential equation
dW
dt
1
W 300 with initial
25
1400.
6. Let f be a function defined by f x (a) Show that f is continuous at x
Î1 2sin x for x … 0
Ï 4x
for x ! 0.
Ðe
0.
(b) For x › 0, express f „ x as a piecewise-defined function. Find the value of x for which f „ x (c) Find the average value of f on the interval > 1, 1@.
WRITE ALL WORK IN THE EXAM BOOKLET.
END OF EXAM
© 2011 The College Board.
Visit the College Board on the Web: www.collegeboard.org.
-6-
3.
2010 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
CALCULUS AB
SECTION II, Part A
Time— 45 minutes
Number of problems— 3
A graphing calculator is required for some problems or parts of problems.
1. In the figure above, R is the shaded region in the first quadrant bounded by the graph of y
horizontal line y 6, and the vertical line x 2.
4 ln3 x , the
(a) Find the area of R.
(b) Find the volume of the solid generated when R is revolved about the horizontal line y
8.
(c) The region R is the base of a solid. For this solid, each cross section perpendicular to the x-axis is a square.
Find the volume of the solid.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2010 The College Board.
Visit the College Board on the Web: www.collegeboard.com.
GO ON TO THE NEXT PAGE.
-2-
2010 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
2. The function g is defined for x ! 0 with g1
2, g „ x 1x , and g„„x
sin x 1Ø
1
È
ÉÊ 1 2 ÙÚ cos x x .
x
(a) Find all values of x in the interval 0.12 … x … 1 at which the graph of g has a horizontal tangent line.
(b) On what subintervals of 0.12, 1 , if any, is the graph of g concave down? Justify your answer.
(c) Write an equation for the line tangent to the graph of g at x
(d) Does the line tangent to the graph of g at x
Why?
t
0
2
P(t)
0
46 53 57 60 62 63
4
6
8
0.3.
0.3 lie above or below the graph of g for 0.3 x 1 ?
10 12
3. The figure above shows an aboveground swimming pool in the shape of a cylinder with a radius of 12 feet and a
height of 4 feet. The pool contains 1000 cubic feet of water at time t 0. During the time interval 0 … t … 12
hours, water is pumped into the pool at the rate P t cubic feet per hour. The table above gives values of P t for selected values of t. During the same time interval, water is leaking from the pool at the rate Rt cubic feet
per hour, where Rt V
25e 0.05t . (Note: The volume V of a cylinder with radius r and height h is given by
! r 2 h. )
(a) Use a midpoint Riemann sum with three subintervals of equal length to approximate the total amount of
water that was pumped into the pool during the time interval 0 … t … 12 hours. Show the computations that
lead to your answer.
(b) Calculate the total amount of water that leaked out of the pool during the time interval 0 … t … 12 hours.
(c) Use the results from parts (a) and (b) to approximate the volume of water in the pool at time t
Round your answer to the nearest cubic foot.
12 hours.
(d) Find the rate at which the volume of water in the pool is increasing at time t 8 hours. How fast is the
water level in the pool rising at t 8 hours? Indicate units of measure in both answers.
WRITE ALL WORK IN THE EXAM BOOKLET.
END OF PART A OF SECTION II
© 2010 The College Board.
Visit the College Board on the Web: www.collegeboard.com.
-3-
2010 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
CALCULUS AB
SECTION II, Part B
Time— 45 minutes
Number of problems— 3
No calculator is allowed for these problems.
4. A squirrel starts at building A at time t 0 and travels along a straight, horizontal wire connected to
building B. For 0 … t … 18, the squirrel’s velocity is modeled by the piecewise-linear function defined
by the graph above.
(a) At what times in the interval 0 t 18, if any, does the squirrel change direction? Give a reason
for your answer.
(b) At what time in the interval 0 … t … 18 is the squirrel farthest from building A ? How far from
building A is the squirrel at that time?
(c) Find the total distance the squirrel travels during the time interval 0 … t … 18.
(d) Write expressions for the squirrel’s acceleration at , velocity vt , and distance x t from
building A that are valid for the time interval 7 t 10.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2010 The College Board.
Visit the College Board on the Web: www.collegeboard.com.
GO ON TO THE NEXT PAGE.
-4-
2010 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
5. Consider the differential equation
dy
dx
x 1
.
y
(a) On the axes provided, sketch a slope field for the given differential equation at the twelve points indicated,
and for 1 x 1, sketch the solution curve that passes through the point 0, 1 .
(Note: Use the axes provided in the exam booklet.)
(b) While the slope field in part (a) is drawn at only twelve points, it is defined at every point in the xy-plane for
dy
which y › 0. Describe all points in the xy-plane, y › 0, for which
1.
dx
(c) Find the particular solution y
f x to the given differential equation with the initial condition f 0 2.
6. Two particles move along the x-axis. For 0 … t … 6, the position of particle P at time t is given by
!
pt 2 cos t , while the position of particle R at time t is given by r t t 3 6t 2 9t 3.
4
(a) For 0 … t … 6, find all times t during which particle R is moving to the right.
(b) For 0 … t … 6, find all times t during which the two particles travel in opposite directions.
(c) Find the acceleration of particle P at time t
at time t 3 ? Explain your reasoning.
3. Is particle P speeding up, slowing down, or doing neither
(d) Write, but do not evaluate, an expression for the average distance between the two particles on the interval
1 … t … 3.
WRITE ALL WORK IN THE EXAM BOOKLET.
END OF EXAM
© 2010 The College Board.
Visit the College Board on the Web: www.collegeboard.com.
-5-
2007 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part A
Time—45 minutes
Number of problems—3
A graphing calculator is required for some problems or parts of problems.
1. Let R be the region in the first and second quadrants bounded above by the graph of y
by the horizontal line y
2.
20
and below
1 x2
(a) Find the area of R.
(b) Find the volume of the solid generated when R is rotated about the x-axis.
(c) The region R is the base of a solid. For this solid, the cross sections perpendicular to the x-axis are
semicircles. Find the volume of this solid.
WRITE ALL WORK IN THE PINK EXAM BOOKLET.
© 2007 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
-2-
GO ON TO THE NEXT PAGE.
2007 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
2. The amount of water in a storage tank, in gallons, is modeled by a continuous function on the time interval
0 … t … 7, where t is measured in hours. In this model, rates are given as follows:
(i) The rate at which water enters the tank is f t 100t 2 sin t gallons per hour for 0 … t … 7.
(ii) The rate at which water leaves the tank is
g t ^
250 for 0 … t 3
gallons per hour.
2000 for 3 t … 7
The graphs of f and g, which intersect at t 1.617 and t
t 0, the amount of water in the tank is 5000 gallons.
5.076, are shown in the figure above. At time
(a) How many gallons of water enter the tank during the time interval 0 … t … 7 ? Round your answer to the
nearest gallon.
(b) For 0 … t … 7, find the time intervals during which the amount of water in the tank is decreasing. Give a
reason for each answer.
(c) For 0 … t … 7, at what time t is the amount of water in the tank greatest? To the nearest gallon, compute the
amount of water at this time. Justify your answer.
WRITE ALL WORK IN THE PINK EXAM BOOKLET.
© 2007 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
-3-
GO ON TO THE NEXT PAGE.
2007 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
x
f x
f „ x g x g „ x 1
6
4
2
5
2
9
2
3
1
3
10
–4
4
2
4
–1
3
6
7
3. The functions f and g are differentiable for all real numbers, and g is strictly increasing. The table above
gives values of the functions and their first derivatives at selected values of x. The function h is given by
h x f g x 6.
(a) Explain why there must be a value r for 1 r 3 such that hr (b) Explain why there must be a value c for 1 c 3 such that h „c (c) Let w be the function given by w x gx
Ô1
5.
5.
f t dt. Find the value of w „3 .
(d) If g 1 is the inverse function of g, write an equation for the line tangent to the graph of y
at x 2.
g 1 x WRITE ALL WORK IN THE PINK EXAM BOOKLET.
END OF PART A OF SECTION II
© 2007 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
-4-
2007 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part B
Time—45 minutes
Number of problems—3
No calculator is allowed for these problems.
4. A particle moves along the x-axis with position at time t given by x t e t sin t for 0 … t … 2 ! .
(a) Find the time t at which the particle is farthest to the left. Justify your answer.
(b) Find the value of the constant A for which x t satisfies the equation Ax „„t x „t x t for 0 t 2 ! .
t
(minutes)
r „t (feet per minute)
0
2
5
7
11
12
5.7
4.0
2.0
1.2
0.6
0.5
0
5. The volume of a spherical hot air balloon expands as the air inside the balloon is heated. The radius of the
balloon, in feet, is modeled by a twice-differentiable function r of time t, where t is measured in minutes. For
0 t 12, the graph of r is concave down. The table above gives selected values of the rate of change, r „t ,
of the radius of the balloon over the time interval 0 … t … 12. The radius of the balloon is 30 feet when t 5.
4 3
(Note: The volume of a sphere of radius r is given by V
!r . )
3
(a) Estimate the radius of the balloon when t 5.4 using the tangent line approximation at t
estimate greater than or less than the true value? Give a reason for your answer.
(b) Find the rate of change of the volume of the balloon with respect to time when t
measure.
5. Is your
5. Indicate units of
(c) Use a right Riemann sum with the five subintervals indicated by the data in the table to approximate
12
Ô0
r „t dt. Using correct units, explain the meaning of
12
Ô0
(d) Is your approximation in part (c) greater than or less than
r „t dt in terms of the radius of the balloon.
12
Ô0
r „t dt ? Give a reason for your answer.
WRITE ALL WORK IN THE PINK EXAM BOOKLET.
© 2007 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
-5-
GO ON TO THE NEXT PAGE.
2007 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
6. Let f be the function defined by f x k x ln x for x ! 0, where k is a positive constant.
(a) Find f „ x and f „„ x .
(b) For what value of the constant k does f have a critical point at x 1 ? For this value of k, determine
whether f has a relative minimum, relative maximum, or neither at x 1. Justify your answer.
(c) For a certain value of the constant k, the graph of f has a point of inflection on the x-axis. Find this value
of k.
WRITE ALL WORK IN THE PINK EXAM BOOKLET.
END OF EXAM
© 2007 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
-6-
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
CALCULUS AB
SECTION II, Part A
Time— 45 minutes
Number of problems— 3
A graphing calculator is required for some problems or parts of problems.
x3
x2
x
3cos x. Let R be the shaded region in the second
4
3
2
quadrant bounded by the graph of f, and let S be the shaded region bounded by the graph of f and line A, the
1. Let f be the function given by f x line tangent to the graph of f at x
0, as shown above.
(a) Find the area of R.
(b) Find the volume of the solid generated when R is rotated about the horizontal line y
2.
(c) Write, but do not evaluate, an integral expression that can be used to find the area of S.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
2
GO ON TO THE NEXT PAGE.
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
2. Let f be the function defined for x • 0 with f 0 by f „ x e
x 4
2
sin x . The graph of y
5 and f „, the first derivative of f, given
f „ x is shown above.
(a) Use the graph of f „ to determine whether the graph of f is concave up, concave down, or neither on the
interval 1.7 x 1.9. Explain your reasoning.
(b) On the interval 0 … x … 3, find the value of x at which f has an absolute maximum. Justify your answer.
(c) Write an equation for the line tangent to the graph of f at x
2.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
3
GO ON TO THE NEXT PAGE.
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
3. The figure above is the graph of a function of x, which models the height of a skateboard ramp. The function
meets the following requirements.
(i) At x
0, the value of the function is 0, and the slope of the graph of the function is 0.
(ii) At x
4, the value of the function is 1, and the slope of the graph of the function is 1.
(iii) Between x
0 and x
4, the function is increasing.
(a) Let f x ax 2 , where a is a nonzero constant. Show that it is not possible to find a value for a so that f
meets requirement (ii) above.
x2
, where c is a nonzero constant. Find the value of c so that g meets requirement (ii)
16
above. Show the work that leads to your answer.
(b) Let g x cx3 (c) Using the function g and your value of c from part (b), show that g does not meet requirement (iii) above.
xn
, where k is a nonzero constant and n is a positive integer. Find the values of k and n so
k
that h meets requirement (ii) above. Show that h also meets requirements (i) and (iii) above.
(d) Let h x WRITE ALL WORK IN THE EXAM BOOKLET.
END OF PART A OF SECTION II
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
4
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
CALCULUS AB
SECTION II, Part B
Time— 45 minutes
Number of problems— 3
No calculator is allowed for these problems.
4. The rate, in calories per minute, at which a person using an exercise machine burns calories is modeled by the
1
3
function f. In the figure above, f t t 3 t 2 1 for 0 … t … 4 and f is piecewise linear for 4 … t … 24.
4
2
(a) Find f „22 . Indicate units of measure.
(b) For the time interval 0 … t … 24, at what time t is f increasing at its greatest rate? Show the reasoning that
supports your answer.
(c) Find the total number of calories burned over the time interval 6 … t … 18 minutes.
(d) The setting on the machine is now changed so that the person burns f t c calories per minute. For this
setting, find c so that an average of 15 calories per minute is burned during the time interval 6 … t … 18.
WRITE ALL WORK IN THE EXAM BOOKLET.
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
5
GO ON TO THE NEXT PAGE.
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
5. Consider the differential equation
dy
dx
y 12 cos ! x .
(a) On the axes provided, sketch a slope field for the given differential equation at the nine points indicated.
(Note: Use the axes provided in the exam booklet.)
(b) There is a horizontal line with equation y
(c) Find the particular solution y
c that satisfies this differential equation. Find the value of c.
f x to the differential equation with the initial condition f 1
0.
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
6
GO ON TO THE NEXT PAGE.
2006 AP® CALCULUS AB FREE-RESPONSE QUESTIONS (Form B)
t
(sec)
vt ft sec ft
at sec 2
0
15
25
30
35
50
60
–20
–30
–20
–14
–10
0
10
1
5
2
1
2
4
2
6. A car travels on a straight track. During the time interval 0 … t … 60 seconds, the car’s velocity v, measured in
feet per second, and acceleration a, measured in feet per second per second, are continuous functions. The table
above shows selected values of these functions.
(a) Using appropriate units, explain the meaning of
60
Ô30
60
Ô30
vt dt in terms of the car’s motion. Approximate
vt dt using a trapezoidal approximation with the three subintervals determined by the table.
(b) Using appropriate units, explain the meaning of
value of
30
Ô0
30
Ô0
at dt in terms of the car’s motion. Find the exact
at dt.
(c) For 0 t 60, must there be a time t when vt 5 ? Justify your answer.
(d) For 0 t 60, must there be a time t when at 0 ? Justify your answer.
WRITE ALL WORK IN THE EXAM BOOKLET.
END OF EXAM
© 2006 The College Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for students and parents).
7
2004 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part A
Time—45 minutes
Number of problems—3
A graphing calculator is required for some problems or parts of problems.
1. Traffic flow is defined as the rate at which cars pass through an intersection, measured in cars per minute. The
traffic flow at a particular intersection is modeled by the function F defined by
F t
82
4 sin
t
for 0
2
t
30,
where F t is measured in cars per minute and t is measured in minutes.
(a) To the nearest whole number, how many cars pass through the intersection over the 30-minute period?
(b) Is the traffic flow increasing or decreasing at t
7 ? Give a reason for your answer.
(c) What is the average value of the traffic flow over the time interval 10
t
15 ? Indicate units of measure.
(d) What is the average rate of change of the traffic flow over the time interval 10
measure.
t
15 ? Indicate units of
Copyright © 2004 by College Entrance Examination Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for AP students and parents).
2
GO ON TO THE NEXT PAGE.
2004 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
2. Let f and g be the functions given by f x
f and g are shown in the figure above.
2x 1
x and g x
3 x
1 x for 0
x
1. The graphs of
(a) Find the area of the shaded region enclosed by the graphs of f and g.
(b) Find the volume of the solid generated when the shaded region enclosed by the graphs of f and g is
revolved about the horizontal line y 2.
(c) Let h be the function given by h x
kx 1 x for 0 x 1. For each k 0, the region (not shown)
enclosed by the graphs of h and g is the base of a solid with square cross sections perpendicular to the
x-axis. There is a value of k for which the volume of this solid is equal to 15. Write, but do not solve, an
equation involving an integral expression that could be used to find the value of k.
Copyright © 2004 by College Entrance Examination Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for AP students and parents).
3
GO ON TO THE NEXT PAGE.
2004 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
3. A particle moves along the y-axis so that its velocity v at time t
t
0, the particle is at y
1. (Note: tan
1
x
(a) Find the acceleration of the particle at time t
1
tan
1
et . At time
arctan x )
2.
(b) Is the speed of the particle increasing or decreasing at time t
(c) Find the time t
0 is given by v t
2 ? Give a reason for your answer.
0 at which the particle reaches its highest point. Justify your answer.
(d) Find the position of the particle at time t 2. Is the particle moving toward the origin or away from the
origin at time t 2 ? Justify your answer.
END OF PART A OF SECTION II
Copyright © 2004 by College Entrance Examination Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for AP students and parents).
4
2004 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
CALCULUS AB
SECTION II, Part B
Time—45 minutes
Number of problems—3
No calculator is allowed for these problems.
4. Consider the curve given by x2
(a) Show that
3y
8y
dy
dx
4 y2
7
3 xy.
2x
.
3x
(b) Show that there is a point P with x-coordinate 3 at which the line tangent to the curve at P is horizontal.
Find the y-coordinate of P.
d2y
at the point P found in part (b). Does the curve have a local maximum, a local
dx2
minimum, or neither at the point P ? Justify your answer.
(c) Find the value of
5. The graph of the function f shown above consists of a semicircle and three line segments. Let g be the function
given by g x
x
3
f t dt.
(a) Find g 0 and g 0 .
(b) Find all values of x in the open interval
answer.
5, 4 at which g attains a relative maximum. Justify your
(c) Find the absolute minimum value of g on the closed interval
(d) Find all values of x in the open interval
5, 4 . Justify your answer.
5, 4 at which the graph of g has a point of inflection.
Copyright © 2004 by College Entrance Examination Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for AP students and parents).
5
GO ON TO THE NEXT PAGE.
2004 AP® CALCULUS AB FREE-RESPONSE QUESTIONS
6. Consider the differential equation
dy
dx
x2 y
1.
(a) On the axes provided, sketch a slope field for the given differential equation at the twelve points indicated.
(Note: Use the axes provided in the pink test booklet.)
(b) While the slope field in part (a) is drawn at only twelve points, it is defined at every point in the xy-plane.
Describe all points in the xy-plane for which the slopes are positive.
(c) Find the particular solution y
f x to the given differential equation with the initial condition f 0
END OF EXAMINATION
Copyright © 2004 by College Entrance Examination Board. All rights reserved.
Visit apcentral.collegeboard.com (for AP professionals) and www.collegeboard.com/apstudents (for AP students and parents).
6
3.
! !
!
1997 AP Calculus AB:
Section I, Part A
"#!$%&'()*+,-!./01'0/(-2!
!
!"#$"!!#$%&''!()*&+,-'&!'.&/-0-&12!)*&!1(34-$!(0!4!05$/)-($!%&!!-'!4''53&1!)(!6&!)*&!'&)!(0!4%%!+&4%!
$536&+'!!'!!0(+!,*-/*!!& 7'8!!-'!4!+&4%!$536&+9!
!
;
:9!
³ : 7= '
!
!
!
!
!
!
!
7?8!
7@8!
7A8!
7B8!
7C8!
<
> '8 (' !
;!
=!
>!
<>!
=;!
;9!
!
D0! & 7 '8
!
7?8!
!
7@8!
!
7A8!
!
7B8!
!
7C8!
' ; ' <2 !)*&$! & c7 '8 !
<' <
;' <
'
;' <
:
;' <
' <
;' <
!
!
!
!
E' >
; ;' <
!
!
)
)
<9!
D0! ³
!
!
!
7?8! * ;) E !
=9!
D0! & 7 '8
!
!
7?8! <!
*
& 7 '8 (' * ;)2 !)*&$! ³ 7 & 7 '8 E8 (' !
*
7@8! E) E* !
7A8!
F) = * !
7B8! F) E* !
7C8! F) >* !
7B8! G<!
7C8! GE!
:
'< ' 2 !)*&$! & c7:8 !
'
7@8! :!
!
AP Calculus Multiple-Choice Question Collection!
7A8! G:!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
100!
!!!!!!!
! !
1997 AP Calculus AB:
Section I, Part A
!
. " - 01 ". /- " / -2 !34!5+65)7&!8+96!,+(!!
"#!
!
!
$%&!'()*%!+,! !
!
:><! " ! = !
!
/
:?<! " / !!+(!! " ! !
.
!
:@<! " !
:A<!
:;<! " = !
/
!!+(!! " ! / !
.
/
" /!
.
!
#
1#!
!
!
B#!
0 /
$ %#
/³
!
#
:;<! $ & !
:><! $
#
/
&!
:?<!
#
/
$
&!
:@<!
#
/
/$
& !
:A<! $# & !
%
5+4 / : ". < !
%"
!
!
:;<! 1 " / 436: ". < 5+4: ". < !
!
:><!
1 " / 5+4: ". < !
!
:?<!
436 / : ". < !
!
:@<! 1 " / 436: ". < 5+4: ". < !
!
:A<! /436: ". < 5+4: ". < !
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
101!
!!!!!!!
! !
1997 AP Calculus AB:
Section I, Part A
!
"#$%&'()%!*+,!-$.$-!&(!&/$!.(00(1')2!%'&#3&'()4!
!
! !
!
!
!
5!6#2!6$2')%!&(!7-310!#8!3!9$-&'730!1'-$!3&!&':$! ! ; 4!</$!9$0(7'&=!"!(.!&/$!6#2!3&!&':$!!>!
; d ! d * >!'%!2'9$)!6=!&/$!.#)7&'()!1/(%$!2-38/!'%!%/(1)!36(9$4!
!
!
*4! 5&!1/3&!930#$!(.!#!!?($%!&/$!6#2!7/3)2$!?'-$7&'()@!
!
!
A5B! C!
ADB! E!
AFB! G!
AHB! I!
AJB! *!
!
,4!
!
!
!
K/3&!'%!&/$!&(&30!?'%&3)7$!&/$!6#2!&-39$0$?!.-(:! !
A5B! LE!
ADB! LM!
; !&(! !
AFB! LL!
L;4! 5)!$N#3&'()!(.!&/$!0')$!&3)2$)&!&(!&/$!2-38/!(.! $
* @!
AHB! *!
7(%AC %B !3&! %
AJB! G!
S
!'%!
E
!
!
S·
§
A5B! $ L ¨ % ¸ !
E¹
©
!
S·
§
ADB! $ L C ¨ % ¸ !
E¹
©
!
AFB! $
S·
§
C¨ % ¸ !
E¹
©
!
AHB! $
S·
§
¨ % ¸ !
E¹
©
!
AJB!
S·
§
C ¨ % ¸ !
E¹
©
$
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
102!
!!!!!!!
! !
!
1997 AP Calculus AB:
Section I, Part A
!
""#! $%&!'()*%!+,!-%&!.&(/0)-/0&!+,!!!!!/1!1%+23!/3!-%&!,/'4(&!)5+0&#!6%/7%!+,!-%&!,+88+2/3'!7+48.!5&!-%&!!
!
'()*%!+,!!!"9!
! !
!
!
!
!
!
":#! ;-!2%)-!*+/3-!+3!-%&!'()*%!+,! #
!
§" "·
>;?! ¨ @ ¸ !
©: :¹
!
!
§" "·
>A?! ¨ @ ¸ !
© : B¹
!
AP Calculus Multiple-Choice Question Collection!
" :
$ !/1!-%&!-)3'&3-!8/3&!*)()88&8!-+!-%&!8/3&! : $ < #
:
>C?!
"·
§
¨"@ ¸ !
<¹
©
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
§ "·
>D?! ¨"@ ¸ !
© :¹
>E?!
= 9!
:@ : !
103!
!!!!!!!
! !
!
"#$! %&'!!!!!(&!)!*+,-'./,!0&*.,&0!*/1!)22!1&)2!,+3(&14!"$!5*! ! c8 "9
1997 AP Calculus AB:
Section I, Part A
7 "6
"6
:!';&,!!!!!.4!0&-1&)4.,<!/,!';&!
.,'&1=)2!
!
8>9!
f: 6 !
8?9!
f: f !
8@9!
6: 7 !
8A9!
6: f !
8B9!
6: f !
!
"7$! %&'!#!!!(&!)!0.**&1&,'.)(2&!*+,-'./,!4+-;!';)'! ! 8#9 6 !),0! ! c8#9 C $!5*!';&!'),<&,'!2.,&!'/!';&!<1)D;!
/*!#!!!)'! " # !.4!+4&0!'/!*.,0!),!)DD1/E.3)'./,!'/!)!F&1/!/*!!!:!';)'!)DD1/E.3)'./,!.4!
!
!
8>9! G$7!
8?9! G$C!
8@9! 6$H!
8A9! #$7!
8B9! C$C!
!
!
!
"C$! I;&!<1)D;!/*!';&!*+,-'./,!!!!!.4!4;/J,!.,!';&!*.<+1&!)(/=&$!K;.-;!/*!';&!*/22/J.,<!4')'&3&,'4!)(/+'!#
!!!.4!'1+&L!
!
!
8>9! 2.3 ! 8 "9 2.3 ! 8 "9 !
" o$
"o%
!
8?9!
2.3 ! 8 "9
6!
!
8@9! 2.3 ! 8 "9
6!
!
8A9! 2.3 ! 8 "9 " !
!
8B9!
" o$
"o%
"o%
2.3 ! 8 "9 !0/&4!,/'!&E.4'$!
" o$
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
104!
!!!!!!!
! !
1997 AP Calculus AB:
Section I, Part A
!
"#$! %&'!()'(!*+!,&'!)'-.*/!'/01*2'3!45!,&'!-)(6&!*+! ! " 7 " !(/3!,&'!1./'! !
!
"<
"#
7@
=7
!
9>;!
!
9?;!
!
9A;!
!
!
9:;!
=
=
=
=
!
"C$! D+! " 7 ! 7
78 E!F&(,!.2!,&'!G(1H'!*+!
!
!
9:;! 78
!
7C
9>;! C
!
7C
#7!
#" 7
8 !.2!
9B;! @S !
!(,!,&'!6*./,! <E= I !
9?;!
C
!
7C
9A;!
=
!
<
9?;!
$ "!
9A;! $ !
9B;!
78
!
7C
!
S
<
J
$ ,(/ "
"@$!
³
!
!
!
9:;! J!
0*2 7 "
#" !.2!
9>;! "!
9B;! $ " !
"K$! D+! % 9 "; 1/ " 7 " E !,&'/! % c9 "; !
!
!
9:;!
!
9>;!
!
9?;!
!
9A;!
!
9B;!
!
7"
"7 "
7"
7
" "
7 "
"7 "
7"
7
" "
"
7
" "
!
!
!
!
!
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
105!
!!!!!!!
! !
!
1997 AP Calculus AB:
Section I, Part A
"#$! %&'!()'*(+'!)(,-'!./! 0.1 ! !.2!3&'!423'*)(,! > 567@ !41!
!
!
89:!
142 7 142 5
!
;
!
8<:!
142 7 142 5
!
"
!
8=:!
142 5 142 7
!
"
!
8>:!
142 5 142 7
!
"
!
8?:!
142 5 142 7
!
;
!
!
!41!
!o@ ,2 !
"@$! ,4A
!
!
89:! #!
8<:!
@
!
"
8=:! @!
8>:! " !
8?:! 2.2'B413'23!
!
""$! C&(3!(*'!(,,!)(,-'1!./!!!/.*!D&40&!3&'!/-2034.2!!#$$E'/42'E!FG! # 8 !: 8 ! " 5:" ! !41!420*'(142+H!!
!
!
89:! %&'*'!(*'!2.!1-0&!)(,-'1!./!!!$!
!
8<:!
! @!!(2E!!! ! 5 !
!
8=:!
5 ! @ !
!
8>:!
@ ! 5 !
!
!
8?:! 9,,!)(,-'1!./!!!
"5$! I/!3&'!*'+4.2!'20,.1'E!FG!3&'!%J(B416!3&'!,42'! % " 6!(2E!3&'!0-*)'! %
! !41!*').,)'E!(F.-3!3&'!!
!
%J(B416!3&'!).,-A'!./!3&'!1.,4E!+'2'*(3'E!41!
!
5"S
@KS
@KS
;S
!
8>:!
!
8?:! S !
!
!
89:!
!
8<:!
!
8=:!
7
5
7
5
!
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
106!
!!!!!!!
! !
!
"#$! %&'!'()*'++,-.!
/
/ § /
"
0
12 ·
˜˜˜ ¨¨
¸ !,+!3!4,'53..!+65!3))*-(,537,-.!8-*!
12 © 12
12
12
12 ¸¹
!
"! !
12
!
9:;!
³2
!
9<;!
³2
!
9=;!
/ / !
"! !
12 ³ 2 12
!
9>;!
/ /
! "! !
12 ³ 2
!
9?;!
/ 12
! "! !
12 ³ 2
/
1997 AP Calculus AB:
Section I, Part A
! "! !
!
!
"1$!
³ ! +,.9" !; "!
!
!
!
/
!
9:;! @-+9" !; +,.9" !; # !
"
#
!
/
!
9<;! @-+9" !; +,.9" !; # !
"
#
!
/
!
9=;! !!! @-+9" !; +,.9" !; # !
"
#
!
9>;! " ! @-+9" !; +,.9" !; # !
!
!
9?;! " ! @-+9" !; #+,.9" !; # !
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
107!
!!!!!!!
! !
1997 AP Calculus AB:
Section I, Part B
!
"#!$%&'()*+,-./0%&1!2.34'3.(5-!6)7'%-)8!
!
"#$%&"!!#$%!&'(!()*+,!-./(01+*2!3*2.(!45!,'(!+400(+,!*-67(0!84(6!-4,!*27*96!*::(*0!*/4-;!,'(!+'41+(6!
;13(-<!='(-!,'16!'*::(-6>!6(2(+,!504/!*/4-;!,'(!+'41+(6!,'(!-./?(0!,'*,!?(6,!*::04)1/*,(6!
,'(!()*+,!-./(01+*2!3*2.(<!
!
!
#@%!A-2(66!4,'(0716(!6:(+151(8>!,'(!84/*1-!45!*!5.-+,14-!!'!!16!*66./(8!,4!?(!,'(!6(,!45!*22!0(*2!
-./?(06!!(!!540!7'1+'!!' #(%!!16!*!0(*2!-./?(0<!
!
BC<! D5! ' # (%
%@ (
> !,'(-! ' c# (% !
@(
!
!
#E%! $!
!
#F%!
!
#G%! % @( !
!
#H%!
!
#I%!
!
% @ ( #$ @ (%
@ (@
% @ ( #@ ( $%
(@
% @ ( #@ ( $%
@ (@
!
!
!
BB<! &'(!;0*:'!45!,'(!5.-+,14-! ) (J C ( @ B ( @ +46 ( !+'*-;(6!+4-+*31,9!*,! ( !
!
!
#E%! K$<LM!
#F%! K$<CJ!
#G%! K$<CB!
#H%! K$<MN!
#I%! K@<JJ!
!
!
!
J
BM<! &'(!;0*:'!45!!'!!16!6'47-!1-!,'(!51;.0(!*?43(<!D5! ³ ' # (% *( @<J !*-8! + c# (%
$
!
!
!
+ #J% + #O% !
!
#E%! O<J!
#F%! $<J!
!
AP Calculus Multiple-Choice Question Collection!
#G%! J<J!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
#H%! P<J!
' # (%> !,'(-!
#I%! L<J!
108!
!!!!!!!
! !
"#$! %&'!!!!!(&!)!*+,-'./,!0+-1!'1)'! 6.7
" o2
!
!
!
!
!
!
!
1997 AP Calculus AB:
Section I, Part B
!
! 34 "5 ! 345
"
8 $!91.-1!/*!'1&!*/66/:.,;!7+0'!(&!'<+&=!
>$! !!!.0!-/,'.,+/+0!)'!#!?!4$!
>>$! !$!.0!@.**&<&,'.)(6&!)'!#!?!4$!
>>>$! A1&!@&<.B)'.B&!/*!$!$!.0!-/,'.,+/+0!)'! #
4 $!
3C5! >!/,6D!!!!!!!!3E5!!!>>!/,6D!!!!!!!!!!!3F5!!!>!),@!>>!/,6D!!!!!!!3G5!!!>!),@!>>>!/,6D!!!!!3H5!!!>>!),@!>>>!/,6D!
4
I2$! %&'!$!$$(&!'1&!*+,-'./,!;.B&,!(D! ! 3 #5 4% J # $!K/<!:1)'!B)6+&!/*!#!.0!'1&!06/L&!/*!'1&!6.,&!'),;&,'!'/!
'1&!;<)L1!/*!!!!!)'! #M ! 3 #5 !&N+)6!'/!O=!
!
!
!
3C5! 2$PQI!
3E5! 2$4"Q!
3F5! 2$OPI!
3G5! 2$OJ4!
3H5! 2$88P!
IP$! C!<).6</)@!'<)-R!),@!)!</)@!-</00!)'!<.;1'!),;6&0$!C,!/(0&<B&<!0'),@0!/,!'1&!</)@!"2!7&'&<0!0/+'1!/*!
'1&!-</00.,;!),@!:)'-1&0!),!&)0'(/+,@!'<).,!'<)B&6.,;!)'!Q2!7&'&<0!L&<!0&-/,@$!C'!1/:!7),D!
7&'&<0!L&<!0&-/,@!.0!'1&!'<).,!7/B.,;!):)D!*</7!'1&!/(0&<B&<!J!0&-/,@0!)*'&<!.'!L)00&0!'1</+;1!'1&!
.,'&<0&-'./,=!!
!
!
3C5! 8"$Q2!
3E5! 8"$II!
3F5! 8#$42!
3G5! Q2$22!
3H5! Q"$J2!
!
I4$! >*! & 4 # I M!:1)'!.0!'1&!7.,.7+7!B)6+&!/*!'1&!L</@+-'!#&!=!
!
!
3C5! SPQ!
3E5! SI!
3F5! SJ!
3G5! 2!
!
3H5! 4!
IO$! 91)'!.0!'1&!)<&)!/*!'1&!<&;./,!.,!'1&!*.<0'!N+)@<),'!&,-6/0&@!(D!'1&!;<)L10!/*! & -/0 #M !& #M !
!
),@!'1&!&T)U.0=!
!
!
3C5! 2$P4"!
3E5! 2$OI8!
3F5! 2$J22!
3G5! 2$Q22!
3H5! 2$#J"! !
!
IJ$! A1&!()0&!/*!)!0/6.@!!'!!.0!'1&!<&;./,!&,-6/0&@!(D!'1&!;<)L1!/*! &
6, # M !'1&!6.,&! # %M !),@!'1&!!
!
#T)U.0$!>*!'1&!-</00!0&-'./,0!/*!'!L&<L&,@.-+6)<!'/!'1&!#T)U.0!)<&!0N+)<&0M!'1&,!'1&!B/6+7&!/*!'!.0!
!
P
4
P O
!
3C5!
!
3E5!
!
3F5! P!
3G5! 4!
3H5!
3% P5 !
4
O
O
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
109!
!!!!!!!
! !
1997 AP Calculus AB:
Section I, Part B
!
"#$! %&!'()!*)+,-.',-)!/&!!!!!,0!1,-)2!34! ! c6 "7 # " 8 " 5 9!.'!:(,;(!/&!'()!&/<</:,21!-.<=)0!/&!"!*/)0!!!!!
(.-)!.!+)<.',-)!>.?,>=>!-.<=)@!
!
!
6A7! BC$DE!
6F7! C$5C!
6G7! C$HI!
6J7! C$H#!
6K7! 8$L8!
!
"E$! M)'! ! 6 "7
!
I
!
6A7!
!
D
!
" $!%&!'()!+.')!/&!;(.21)!/&!!!!!.'! "
6F7! I!
$ !,0!':,;)!,'0!+.')!/&!;(.21)!.'! " I 9!'()2! $
6G7! D!
6J7!
I
!
5
6K7!
I
5 5
"L$! A'!',>)! % t C 9!!'()!.;;)<)+.',/2!/&!.!N.+',;<)!>/-,21!/2!'()!"O.?,0!,0! & 6% 7 % 0,2 % $!A'! %
!
-)</;,'4!/&!'()!N.+',;<)!,0!B5$!P/+!:(.'!-.<=)!%!:,<<!'()!-)</;,'4!/&!'()!N.+',;<)!3)!Q)+/@!
!
!
6A7! I$C5!
6F7! I$D"!
!
AP Calculus Multiple-Choice Question Collection!
6G7! I$"#!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
6J7! 5$"I!
!
!
C 9!'()!!
6K7! 8$ID!
110!
!!!!!!!
! !
!
""#! $%&! # ' !(
!
³"
1997 AP Calculus AB:
Section I, Part B
!
$'% ( &% )!*+%,%!$!+-.!&+%!/,-0+!.+1*2!-314%#!5+67+!18!&+%!81991*62/!71:9;!3%!&+%!
/,-0+!18!!#!<!
!
!
!
!
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
111!
!!!!!!!
! !
!
1997 AP Calculus AB:
Section I, Part B
!
" "#$ %#" %#$ &#"
!
" ' !( ) )
$
* %)
!
*+#! ,!-./01!23!4.0516!327!.!829-:95256!3598-:29!!"!!:6!6;2<9!./241#!=3!3257!1>5.0!65/:9-174.06!23! > "? &@ !
.71!561@?!<;:8;!23!-;1!32002<:9A!:6!-;1!-7.B1C2:@.0!.BB72D:E.-:29!23! ³
!
!
!
',(! *!
'G(! %&!
'H(! %I!
'J(! &K!
&
"
" ' !( #! F!
'L(! )&!
+"#! M;:8;!23!-;1!32002<:9A!.71!.9-:@17:4.-:416!23! " ' !( 6:9 ! 826 ! F!
!
6:9 & !
!
=#! $ ' !(
!
&
!
==#! $ ' !(
!
===#! $ ' !(
!
!
!
!
!
!
!
!
!
!
826 & !
!
&
826'& !(
!
K
',(! =!290N!
'G(! ==!290N!
'H(! ===!290N!
'J(! =!.9@!===!290N!
'L(! ==!.9@!===!290N!
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
112!
!!!!!!!
!!
1997 Answer Key!
1997 AB
!
"#! $!
%#! &!
'#! $!
(#! )!
*#! +!
,#! $!
-#! )!
.#! $!
/#! 0!
"1#!+!
""#!+!
"%#!0!
"'#!&!
"(#!$!
"*#!0!
",#!)!
"-#!&!
".#!$!
"/#!)!
%1#!+!
1997 BC
!
!
%"#!+!
%%#!)!
%'#!&!
%(#!0!
%*#!&!
-,#!+!
--#!)!
-.#!)!
-/#!$!
.1#!&!
."#!&!
.%#!0!
.'#!$!
.(#!$!
.*#!$!
.,#!&!
.-#!0!
..#!+!
./#!0!
/1#!)!
!
AP Calculus Multiple-Choice Question Collection!
!
"#! $!
%#! +!
'#! &!
(#! $!
*#! $!
,#! &!
-#! $!
.#! +!
/#! &!
"1#!0!
""#!$!
"%#!&!
"'#!0!
"(#!$!
"*#!)!
",#!0!
"-#!0!
".#!$!
"/#!)!
%1#!+!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
!
!
%"#!&!
%%#!$!
%'#!+!
%(#!)!
%*#!&!
-,#!)!
--#!+!
-.#!&!
-/#!)!
.1#!0!
."#!)!
.%#!0!
.'#!+!
.(#!$!
.*#!)!
.,#!&!
.-#!0!
..#!$!
./#!)!
/1#!0!
158!
!!!!!!!
!!
"#!
1997 Calculus AB Solutions: Part A
$!
%
³"
(' !& ) !* "! ( ! ' & ! % *
%
"
(") "%* (" &* ) !
!
%#!
"
!(% ! &* % , !!!!# c( !*
# ( !*
+!
"
(% ! &* %
!(% ! &*
"
%
(% ! &*
"
% (& ! &*
(&! &*
!
%! &
!
$
$
³%
'#!
/!
# ( !*
!
-#!
0!
&
$!
" %
( "'
%³
.#!
/!
"
@5? % ( !& *
"!
§"
·
% @5?( !& * ¨ (@5?( !& * ¸
© "!
¹
!
!
1#!
!
2#!
!
!
% @5?( !& *( ?>A( !& *(& ! % * !
$!
E9:!FG;!@9<A;:!C>6:@8>5A!D9:A!*!@9<A;:?!?>;A#!E9>?!9<==:A?!<8! '
H!
I:8! +" !F:!89:!<6:<!F:8D::A!89:!;6<=9!<AC!'J<K>?!456! 3 d ' d ) 7!<AC!L:8! + % !F:!89:!<6:<!F:8D::A!
( # ( !* -* "!
³%
$
$!
&#!
# ( !* "! -³ " "! !% %$ -($ %* .$ '% !
%
!
"
!& ! , !!!# c( !*
!
& ! % " "
!
%
, !!# c("*
&("*% " "
("* %
& " " & !
& ! ' ") !& %' ! % '1, !!&c "% !& '1 ! % '1 !, !!&cc &) ! % 2) ! '1 "%(&! %*( ! %* !!
%
%
&cc 3!!456!! ! %7 !89:6:456:!89:!;6<=9!>?!@5A@<B:!C5DA!456 ! ! % !
&
&
!
'
)#!
'
%
(
) !
!
§"
·
% @5?( !& *( ?>A( !& * ¨ ( !& * ¸ !
© "!
¹
) #!
89:!;6<=9!<AC!89:!'J<K>?!456! ) d ' d 1 !E9:A! +" "%!!<AC!!+ % " #!!E9:!858<L!C>?8<A@:!>?!
+" + % "& #!
!
"3#! 0!
!
""#! 0!
&
§S·
@5?(% !*, !!&c %?>A(% !*, !!!&c ¨ ¸
©'¹
§S·
%!!<AC!!& ¨ ¸ 3,!!&
©'¹
S·
§
% ¨ ! ¸ !
'¹
©
M>A@:! # c !>?!=5?>8>B:!456!! % ! % !!<AC!A:;<8>B:!456! ! %!<AC!456!! ! %7 !D:!<6:!L55N>A;!
456!<!;6<=9!89<8!>?!>A@6:<?>A;!456! % ! % !<AC!C:@6:<?>A;!589:6D>?:#!OALP!5=8>5A!0#!
!
"%#! H!
&
" %
! , !!&c
%
!, !Q:!D<A8!!&c
AP Calculus Multiple-Choice Question Collection!
"
! Ÿ !!
%
"
§" "·
#!M5!89:!=5>A8!>?! ¨ 7 ¸ #!
%
© % 1¹
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
217!
!!!!!!!
!!
1997 Calculus AB Solutions: Part A
*!!##"!!+,!-./0.1,+23!45.2!! " c 6 $!!7+2/.!85.!29:.018;0!+,!2;2<2.318+=.>!85+,!+,!
!&
!;2?@!45.2!85.!-.2;:+218;0!+,!2.318+=.$!!A2?@!45.2!! ! & $!!
" c( !)
"#$! %!
!
!
!
"'$! B!
!
"C$! F!
' !&
!
" ( ! ) | $( !)
& C( ! #)* !!$( !) 6!!+D!!6 C ! "#!! Ÿ !!!
7818.:.28!F!+,!809.!G./19,.! ?+: " ( !)
&
! o%
&$E !
?+: " ( !) $!%?,;>! ?+: " ( !) !-;.,!2;8!.H+,8!
! o% !o&
G./19,.!85.!?.D8<!12-!0+358<,+-.-!?+:+8,!10.!2;8!.I91?>!,;!2.+85.0!(%)>!(B)>!2;0!(J)!10.!809.$!
!
"E$! J!
K5.!10.1!;D!85.!0.3+;2!+,!3+=.2!G@!! ³
&
&
"
&(' ! !# )
#
(C ( ! & "))'!
&
6
§ L·
&¨L ¸
© #¹
#&
!
#
!
"M$! %!
!
!
'
*! !
#
&C
§ '· § '·
!! ( ˜ (c 6! Ÿ !"N( ˜ (cc (c ˜ (c 6* !!" (#) (cc ¨ ¸ ˜ ¨ ¸ 6* !!(cc !
&M
© #¹ © #¹
!& ( &
&C* !!& ! & ( ˜ (c 6* !!! ( ˜ (c 6* !!(c('>#)
!
"L$! B!
³
S
'
6
³
S
'
6
!
"O$! J!
) 812 !
&
/;, !
) 812 !
&
/;, !
'! !!+,!;D!85.!D;0:!! ³ )* '* !45.0.! *
'!
) 812 !
S
'
6
)" )6
" ( !) ?2 ! & " !*!!!!" c( !)
!
&6$! P!
"
) "!
' &
( ! ")
! " '!
&
812 !$ $!!
˜
" C
"
/;, ! '!
(,+2 C ,+2(#))
³
L #
L
,+2(#) ,+2(#) $!
&!
&
! "
!
"
(,+2 C ,+2 #) *!!Q;8.R!!7+2/.!85.!,+2.!+,!12!;--!D92/8+;2>!
L
!
!
!!+,!2;2.H+,8.28!,+2/.! ?+:!?2 !
!o" ?2 !
!o"
6!!12-!! ?+: ! z 6 $!
&"$! P!
?+:
!
!
&&$! J!
!
" ( !) ( ! & #)) ! * !!" c( !) ) ! ( ! & & ! #)
&#$! %!
J+,S,!45.0.! +
! $!! ,
&
S³ ! & '(
6
!o"
&
S ³ ( ' '(
6
) ! ( ! #)( ! ")* !!" c( !) ! 6!!D;0!! " ! # !
S C
(
C
&
6
#&S
!
C
!
!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
218!
!!!!!!!
!!
1997 Calculus AB Solutions: Part A
"#$! %!
&'(! > )*+@ !,'!-./.-'-!.0(1!2)!34,.0('5/673$!! '!
!
:3.0;! " < !=
+
9 !!!+
2)
+
* !"
2)
"
* !8
2)
8
* ˜˜˜* !2) + !!
2)
2)
!
! *!(>'!5.;>(!?.'@600!34@! ¦ " < !# ='! !.3!60!6AA51B.@6(.10!C15! ³
# +
!
"2$! D!
+
)
! $! $!
:3'!(>'!('E>0.F4'!1C!60(.-'5./6(./'3!,G!A65(3*!H>.E>!H63!5'@1/'-!C51@!(>'!D%!I1453'!
J'3E5.A(.10!.0!+KKL$!
!
!
!
%
!
$%
$!
$& 3.0 " ! $!
!!!
+
& E13 " !
"
!
!
!
³ ! 3.0<" != $!
+
+
+
+
! E13<" != ³ E13<" != $! ! E13<" != 3.0<" != '
"
"
"
#
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
219!
!!!!!!!
!!
1997 Calculus AB Solutions: Part B
!
"#$! %!
!
""$! ,!
# ' !)
"& !
* !!# c' !)
&!
&" & ! ˜ & ! &" & !
" & ! '& ! ()
+!&
& !&
!
$ !- # ! & " ! & ./0 ! $!!1//2!34!456!78395!/:! $cc # ! (& & ./0 ! !;<!456!=;<>/=!!
?@-A@(B!0;<.6!4534!>/C3;<!./<43;<0!3DD!456!/94;/<!E3DF60$! $cc !.53<760!0;7<!34! ! ($GH $!!!
!
"G$! ,!
-
³I
% '-) % 'I)
(
-
³I
# ' !)&!
# ' !)&! ³ # ' !)&!
(
& &$- +$- !!
(
' J/F<4!0KF3860!:/8! ³ # ' !)&! )!
I
!
"H$! J!
L56!046C!/:!456!KF604;/<0!C63<0! # c'&) M $!L5F0!!#!!;0!>;::686<4;3ND6!34!!!O!&!3<>!45686:/86!
./<4;<F/F0!34!!!O!&$!P6!2</=!</45;<7!/:!456!./<4;<F;4Q!/:! # c $!R!3<>!RR!/<DQ$!
!
GI$! S!
!
G($! S!
!
!
G&$! U!
!
&
&
&
# ' !) &"+ ! * !# c' !) (# !" + ! * !P6!=3<4!!(# !" + !
:F<.4;/<!$!O!-A!456<!:;<>!456!;<46806.4;/<!4/!764! !
164!!!N6!456!>;043<.6!/:!456!483;<!:8/C!456!
&!
.8/00;<7$!L56<!
#I $!
&'
&(
&!
&( ! &!
( & ! & "I& Ÿ & (
&! Ÿ
$!
&'
&'
&' ( &'
S:468!+!06./<>0A! ! &+I !3<>!0/! ( &MI $!
&( &+I
'#I) M"$# !
L5686:/86!
&' &MI
! !
) ' !) & ! & G !* !)c' !) + ! G* !)c!.53<760!:8/C!<6734;E6!4/!9/0;4;E6!34!!
*
I$"-HIGM$!!V4/86!45;0!;<!!*$!! ³ './0 ! !) &!
G-$! J!
./0 !
!
G+$! J!
J8/00!06.4;/<0!386!0KF3860!=;45!0;>60!/:!D6<745!$$!
!
W/DFC6!O ³ $ & &!
!
!
GM$! J!
!
G#$! S!
-$ !T8395!456!>68;E34;E6!:F<.4;/<!3<>!456!
I$(#G $!
! !!34!!!
"
(
I
"
³( D< ! &!
'! D< ! !)
"
(
&$!)'&)
I$+II !
'" D< " ") 'I () ( !
1//2!34!456!78395!/:! # c !3<>!D/.346!=5686!456!$!.53<760!:8/C!9/0;4;E6!4/!<6734;E6$! !
# ' !)
! * !!# c' !)
(
& !
* !!
AP Calculus Multiple-Choice Question Collection!
(
&˜
& +
(
& (
G !
! Ÿ !+
I$H( !
(
!
+
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
220!
!!!!!!!
!!
1997 Calculus AB Solutions: Part B
"#$! %!
!(" ) " *+, " !!-,.!!#(/)
&! Ÿ !#(" )
' &
" 01* " '2 !!#(" ) /!-3!" '$4" !
&
!
""$! 5!
% ( $)
$
³ ! &( $)'$ Ÿ % (!)
/6 !37898:198!1,;<!(=)!19!(5)!-98!>1**+?;8$!%@3! % c( $ ) &( $ ) !-,.!
37898:198!!%!!+*!.+::898,3+-?;8!-3!$(A!)$!B7+*!+*!39@8!:19!378!C9->7!+,!1>3+1,!(5)!?@3!,13!+,!1>3+1,!
(=)!D7898!37898!->>8-9*!31!?8!-!019,89!+,!378!C9->7!-3!$!A!)$!=;*16!E+,08!&!+*!+,098-*+,C!-3!
:+9*36!378!C9->7!1:!!%!!F@*3!*3-93!1@3!01,0-G8!@>$!B7+*!+*!-;*1!39@8!+,!(5)!?@3!,13!(=)$!
!
"H$! %!
*
' '
˜ (I & ˜ I & ˜ J & ˜ " 'I) '& !
& &
!
H/$! K!
+ ( $)
!
!
+ ( $)
!
!
+ ( $)
' &
*+, $ !
&
'
01* & $ !
&
'
01*(& $) !
4
+ c( $) *+, $ 01* $ !
L8*!
+ c( $)
01* $ *+, $ !
M1!
+ c( $)
'
*+,(& $) *+, $ 01* $ !
&
L8*!
AP Calculus Multiple-Choice Question Collection!
!
Copyright © 2005 by College Board. All rights reserved. Available at apcentral.collegeboard.com.
221!
!!!!!!!