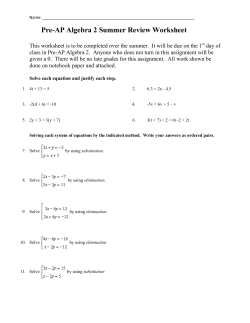
Quiz for Lessons 8-1 Through 8-4
CHAPTER
SECTION 8A
Quiz for Lessons 8-1 Through 8-4
8-1 Identifying Quadratic Functions
Tell whether each function is quadratic. Explain.
1. y + 2x 2 = 3x
2. x 2 + y = 4 + x 2
{(2, 12), (-1, 3), (0, 0), (1, 3)}
3.
Tell whether the graph of each quadratic function opens upward or downward and
whether the parabola has a maximum or a minimum.
4. y = -x 2 - 7x + 18
5. y - 2x 2 = 4x + 3
6. y = 5x - 0.5x 2
1 x 2 - 2 and give the domain and range.
7. Graph the function y = _
2
8-2 Characteristics of Quadratic Functions
Find the zeros of each quadratic function from its graph. Then find the axis of
symmetry.
8.
9.
Þ
10.
Þ
n
Ó
Ó
Ó
Ý
Ý
{
Ó
Ó
{
Ý
n
ä
{
Ó
{
ä
Ó
{
Ó
{
Fnd the vertex.
11. y = x 2 + 6x + 2
12. y = 3 + 4x - 2x 2
13. y = 3x 2 + 12x - 12
14. The height in feet of the curved roof of an aircraft hangar can be modeled by
y = -0.02x 2 + 1.6x, where x is the horizontal distance in feet from one wall at
ground level. What is the greatest height of the hangar?
8-3 Graphing Quadratic Functions
Graph each quadratic function.
15. y = x 2 + 3x + 9
16. y = x 2 - 2x - 15
17. y = x 2 - 2x - 8
18. y = 2x 2 - 6
19. y = 4x 2 + 8x - 2
20. y = 2x 2 + 10x + 1
8-4 Transforming Quadratic Functions
Compare the graph of each function with the graph of f (x) = x 2 .
2 x2
21. g (x) = x 2 - 2
22. g (x) = _
23. g (x) = 5x 2 + 3
3
24. g (x) = -x 2 + 4
25. The pilot of a hot-air balloon drops a sandbag onto a target from a height of 196 feet.
Later, he drops an identical sandbag from a height of 676 feet.
a. Write the two height functions and compare their graphs. Use h (t) = -16t 2 + c,
where c is the height of the balloon.
b. Use the graphs to tell when each sandbag will reach the ground.
Ready to Go On?
553
© Copyright 2025