1. finance
2. bank

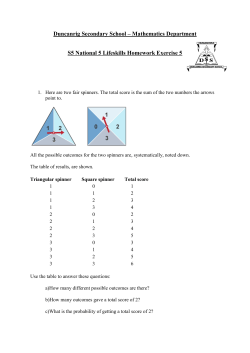
Currency Crises and the DR Shadow Exchange Rate
Federico Filippini∗
Universidad de los Andes
March 20, 2015
Download Newest Version
Abstract
This paper develops an analytical model of cross-listed asset (assets issued in the domestic country
and traded both on the domestic and a foreign market) and shows that these assets can be used to
compute a shadow exchange rate and forecast large devaluations. Among cross-listed assets, the focus on
the Depositary Receipts (DRs), which are claims priced in a foreign market of shares of a domestic asset.
Relative to its corresponding domestic assets, DRs (i) share the same risk factor except that the latter
are priced in foreign currency, and (ii) can be converted into shares of domestic asset (and viceversa).
Then, investors can use this cross-listed assets to send money abroad, which provides an alternative
technology to acquire dollars, rather than going to the central bank. The model extends the literature
of currency crises and speculative attacks by allowing trading in two different markets with cross-listed
assets and shows that the law of one price, between the domestic asset and the corresponding DR, fails to
hold in equilibrium prior to the outbreak of the crises, in line with the empirical evidence. Furthermore,
the model suggests that DRs can be used to compute a measure of shadow exchange rate, and forecast
devaluations.
∗ I am extremely grateful to Boyan Jovanovic for his invaluable guidance. I also want to thank Jess Behnabib, Klaus Hellwig,
David Kohn, John Leahy, Laurent Mathevet, Michal Szkup, Stijn Van Nieuwerburgh, Laura Veldkamp, Shengxing Zhang and
seminar participants at the NYU Stern Macro Lunch for useful suggestions and comments. Any remaining errors are my own.
Email: [email protected]
1
1
Introduction
In the proximity of currency crises, uncertainty critically rises. Moving from the status quo to a new
regime usually requires agents to drastically adjust their actions, while facing the challenging problem to
estimate when the change will take place, and what will happen in the new regime. This paper aims to
provide new tools for solving these statistical problems, by developing a theoretical model that captures
the behavior of asset prices around the outbreak of currency crises. The model extends the Morris-Shin
framework, where asymmetrically informed agents face the risk of an exchange rate regime change, to
consider the behavior of the price a domestic asset relative to its corresponding Depositary Receipt (DR).
DRs are claims, priced in a foreign market, of shares of a domestic asset upon which the DR is written1 .
In line with the empirical evidence, the model predicts that persistent deviations from the law of one price
(LooP) (that relates the price of the domestic asset and its corresponding Depositary Receipt) anticipate
currency crises. In particular, the model shows that informational (and market) frictions can generate these
deviations prior to the outbreak of the crises.
Before the outbreak of currency crises, asset prices are usually subject to massive fluctuation as a response to the sudden reversal of capital flows. Kaminsky and Reinhart [1999] show, for a large sample of
currency crises, that stock market indexes decline around 20% before the outbreak of the crises, highlighting
the potential predicting power of asset prices. However, they also show that these stock market indexes
corrections occur (on average) about a year before the beginning of the crises, and not every stock market
corrections predict devaluations. In this line, the empirical studies on forecasting currency crises has not
provided precise predictions on the timing and magnitude of the crises.
The challenge of using stock market data is to identify the currency crises risk from other factors affecting
asset prices. This paper solves this problem by using a particular set of asset: cross-listing assets, instead
of only considering the stock market index. Cross-listing assets are issued in the domestic country and are
traded both on the domestic and a foreign market. Within this class of assets, the focus is on DRs, given that
for such assets the domestic asset and the corresponding DR share the same risk factors with the exception
that the latter is also subject to the exchange rate risk. Therefore, DRs can be used as a tool to measure
investors expectations about the exchange rate, and provide a way to assess the currency crises risk.
This paper proposes two extensions to the second generation models of currency crises2 , and subsequent
1 American Depository Receipt (ADR) and Global Depository Receipt (GDR) are two common forms of depository receipts.
An ADR is listed and traded on exchanges based in the US, and dollar-denominated, while a GDR is traded non-U.S. markets
such as London, Frankfurt and Singapore.
2 Second generation models of currency crises, starting with Obstfeld [1986] and Flood and Garber [1984], allow for the
2
works of Morris and Shin [1998] and others. First, the main source of uncertainty is switched from the
characteristics (of the balance sheet) of the central bank to the performance of the domestic economy, about
which investors have imperfect and asymmetric information. In this way, the model resembles more closely
episodes of sudden reversal of capital flows, similar to the recent crises in East Asia (1997-98) and Russia
(1998), or even those studied by Eichengreen, Rose and Wyplosz [1995], in which the exchange rate regime
was not obviously unsustainable by simply considering the balance sheet of the central bank3 . In the current
paper, the currency crisis is triggered by investors unfolding their positions on domestic assets - which
happens when their expectations about the performance of domestic assets deteriorate - and increasing their
positions on dollars. When a sufficiently large mass of investors go to the central bank to exchange domestic
currency, the foreign reserves drop (below some commonly known threshold determined by the central bank,
as in Obstfeld [1986]), and a devaluation takes place. As in Angeletos and Werning [2006], asset prices
play a dual role simultaneously affecting the payoffs of the domestic asset and aggregating disperse private
information.
Second, the model introduces cross-listing assets. Cross-listing assets play an important role in the global
economy. On the one hand, they allow companies to access financing opportunities with greater liquidity
and transparency. On the other hand, they provide international investors with accessible tools to obtain
greater diversification. In terms of the model, cross-listing assets allow investors to transfer resources from
one market to other without the need of using dollars. Among the cross-listing instruments4 , the focus is on
Depositary Receipts (DRs). As discussed in Bailey, Chan and Chung [2000], DRs account for most of the
trading of cross-listed assets.
Since DRs can be converted into shares of the domestic asset at any point in time (and vice-versa), both
securities are nearly perfect substitutes. By comparing the price of the DR and the corresponding domestic
asset, is possible to obtain a measure of the exchange rate investors expect, in the event of a currency crisis.
In this paper, the ratio of the price of the DR and the domestic asset constitutes the DR shadow exchange
rate.
In a frictionless (and financially integrated) economy, arbitrage enforces the LooP between the domestic
existence of multiple equilibria and, in this way, are able to account for the unpredictability of crises. A more exhaustive
description of existing models of currency crises and the relationship between first and second generation models can be found
in Jeanne [2000] and Cavallari and Corsetti [2000].
3 In particular, Obstfeld [1996] shows that for the case of the European Monetary Union during 1992-94, in countries such
as France, Belgium or Denmark did not display signs of weak fundamentals.
4 Non-U.S. firms list shares in U.S. markets in several different ways: New York or Global registered shares, American
Depositary Receipts, or even direct listings ordinary shares of the domestic market. These instruments differ in term of their
fungibility (or, exchangeability) between the underlying stock and the cross-listed ones, affecting the investors ability to arbitrage
away cross-border differences.
3
asset and the corresponding DR. The LooP implies that (i) the DR shadow exchange rate should be equal
to the spot exchange rate, and that (ii) there is no relevant information contained in the prices. However,
empirical literature has documented events where the LooP fails to hold. Melvin [2003], Auguste, Dominguez,
Kamil and Tesar [2006] and Levy Yeyati, Schmukler and Van Horen [2009] study the behavior the DRs
securities during episodes with capital controls and show that market friction limit arbitrage and prevents
the LooP from holding, while Eichler, Karmann and Maltritz [2009] and Maltritz and Eichler [2010] study
the mis-pricing of American Depositary Recepts (ADRs) around currency crises, and show that these events
are associated with persistent and increasing deviations of the LooP.
Discusion: Main mechanism to break the LooP
Consider an investor holding a position in domestic currency in a country with fragile fundamentals
that could be subject to a run on the currency or capital flow reversals. Given that the domestic asset is
cross-listed, the investor has access to two alternative technologies to acquire dollars: the central bank or a
cross-market transaction (CMT). The CMT requires purchasing the domestic asset, converting it into DRs,
and selling those shares in the foreign market. As opposed to acquiring dollars at the central bank, the CMT
is (i) reversible (the investor can convert back the DRs into shares of the domestic asset) and (ii) allows
for observing new information enclosed in the price of the DR. Therefore, the domestic asset has embedded
the option to acquire foreign currency through the CMT. When the economy is close to its tipping point
(beyond which there is a devaluation), the value of this option increases and so does the price of the domestic
asset - particularly, relative to the price of the DR. In this way, price of the underlying and the exchange
rate-adjusted DR display a wedge which is decreasing in the strength of the fundamentals.
The paper also shows that while capital controls limit arbitrage, they fail to replicate the anticipatory
behavior of cross-listing assets to currency crises. The paper proposes an information friction in the context
global games, as the main mechanism that explains the behavior. The value of the domestic asset derives
from the expected dividend payments plus the option to use the CMT (which is increasing in the uncertainty),
helps assert whether a devaluation will take place or not, and what would be the post-devaluation level of
the exchange rate.
Outline
The rest of the paper is organized as follows. Section (2) shows evidence on the break of the LooP for
the case of Argentina and section (3) reviews the literature related to the paper. Section (4.1) presents the
4
setup of the model for the model with cross-listing assets. Section (4.3) solves the benchmark model when
agents have common knowledge. Section (4.4) allows traders to exchange a cross-listed asset and contrast
the empirical implications with the previous section. Finally, section (5) presents some final remarks.
2
Empirical Evidence
Figure (1) illustrates the evolution of the stock market index and the official exchange rate around the
currency crisis of Argentina in 2001. To give a brief background, after the hyperinflation episode in 1989,
Argentina adopted a fixed exchange rate that pegged the peso one to one to the dollar. During the Mexican
crisis, the Argentinean central bank was able to maintain the currency peg despite of the pressures generated
by the sudden capital reversals. However, the worsening external conditions in the early 2001 tied with the
incapacity of policy makers to use the exchange rate as a buffer to accommodate the shocks, exacerbated
the misalignment of the nominal exchange rate. Consequently, capital controls were introduced in December
2001 (until November 2002) and the peg collapsed one month after, on January 2002, generating an initial
devaluation of 40%. These devaluation was expected by investors who set the ADR shadow exchange rate
at around 1.5 ARS/USD in the week preceding the devaluation, as shown in figure (2).
In particular, figure (1) shows a steady decline in the stock market index around one year prior to the
collapse of the exchange rate regime, and even before international reserves declined. This evidence partially
contradicts the conclusion arrived by the literature, since the stock market index actually increased before
the outbreak of the crisis. According to the traditional view, there is no reason why the investor’s value of
domestic asset increased closer to the outbreak of the crisis. Furthermore, the figure shows that the level of
international reserves may not have been determinant over the timing of the crisis. Specifically, in August
2001 the level of international reserves fell below phycological threshold of $ 15.000 millon for the first time,
and was about the same level as at the time of the devaluation.
Figure (2) displays the price of the stock of BBVA Banco Frances, one of the most liquid cross-listed
Argentinean stocks, around the currency crisis in early 2002. The red-dashed line represents the price of
the domestic stock (FRAN.BA) traded in the Argentinean stock market, and denominated in pesos. The
blue line depicts the price of the ADR (BFR.US) traded in the NYSE, adjusted by the spot exchange rate.
When both lines coincide, there are no arbitrage opportunities given that the prices measured in the same
currency (in this case, in Argentinean pesos) are equal. The gray line marks the outbreak of the currency
crisis. Note that in the proximity of the outbreak, prices diverge creating potential arbitrage opportunities.
5
Figure 1: Argentina: Stock market index (Merval) and official exchange rate.
Note: This figure shows the evolution of the Merval Stock Index, the international reserves and the official exchange rate for
Argentina from November 1998 to September 2004. In January 2002, the Central Bank of Argentina devalued the currency by
40%. Source: Bloomberg.
The wedge between both prices, also referred as the cross-market premium, increases before the crisis and
remains positive even after a couple of some months.
Figure (3) displays the ADR shadow exchange rate and the official exchange rate for Argentina around
January 2002. The red line represents the official exchange rate, which was fixed to 1 until January 2002.
The blue line represents the ratio of the prices at the Argentinean stock market and the NYSE of BBVA
Banco Frances, which is referred as the ADR shadow exchange rate.
Figure (3) suggests that the ADR shadow exchange rate anticipates the collapse of the fixed exchange
rate regime. This anticipatory deviation from the LooP requires that investors value domestic asset relatively
more than the ADR adjusted by the prevailing exchange rate. This pre-crisis dynamic of the ADR shadow
exchange rate, is not be captured in a model with perfect information or even with capital controls, as shown
in the Appendix (by equation (28)).
Figure (4) illustrates the ADR shadow exchange rate and the official exchange rate in Argentina for the
recent past. After the collapse of the currency board in 2002, the central bank adopted a crawling peg
6
Figure 2: Argentina: BBVA Banco Frances. Underlying and ADR prices, in pesos.
Note: The red line represents the price of FRAN.BA (underlying) in pesos and the green line represents the price BFR.US
(ADR) traded in the NYSE, adjusted by the spot exchange rate. The blue line marks the outbreak of the currency crisis. The
top panel displays a sample from Nov/1998-Sep/2004, while the bottom panel only displays the sample Aug/2001-Jun/2002.
exchange rate. With the objective to reduce the exchange rate volatility, the government set a path for
the exchange rate with a constant depreciation rate. Since early 2011, the external conditions continuously
deteriorated increasing perceived probability of a devaluation. The government imposed capital controls,
restricting investors access for foreign exchange. The investors use the CMT in order to acquire dollars,
increasing the value of the underlying stocks. Dots are chronologically sorted, and linked by the dashed
line. Note that prior to the large movements of the official exchange rate, the ADR shadow exchange rate
increases dramatically highlighting its predicting power.
3
Literature Review
This paper is related to several strands in the literature. It fits within the second generation models of
currency attacks that considers heterogenous beliefs, going back to Morris and Shin [1998]. It is more closely
related to the models of Angeletos and Werning [2006] and Hellwig, Mukherji and Tsyvinski [2006] that
focus on the role of markets in providing useful information about the economic fundamentals in a context of
potential speculative attacks. The main difference with these papers is that investors can access cross-listing
7
Figure 3: Argentina: ADR shadow exchange rate and official exchange rate.
Note: This figure shows the ADR shadow exchange rate and the official exchange rate for Argentina around the currency crises.
On December 6, 2001 the exchange rate was realigned from 1 peso/dollar to 1.2 peso/dollar. For the next 3 year, the exchange
rate fluctuated around 3.8 and 4.2 peso/dollar. The ADR shadow exchange rate is calculated according to Eq. (4).
assets. As shown in the previous section, cross-listing are better predictors of the timing and the size of the
crisis, relative to stock market indexes.
The literature on speculative attacks and currency crises has assumed as one of the main source of uncertainty the characteristics of the central bank. Morris and Shin [1998] and Goldstein, Ozdenoren and Yuan
[2011] assume that agents are uncertain about the strength of the central bank to confront an speculative
attack or the its private value of maintaining the currency peg. Hellwig et al. [2006] assume uncertainty over
the highest tolerable level of interest rates for the central bank, in line with Obstfeld [1986]. These assumptions neglect some of the empirical evidence since, at times when the odds of a currency run increases, the
news’ coverage on the central bank account’s peaks, increasing the amount and the precision of the public
information available. This, in turn, makes it harder to achieve the uniqueness result that emerges in models
on global games5 .
This paper differs from most of the studies mentioned above, by assuming the main source of uncertainty
5 For example Angeletos and Werning [2006], who introduce exogenous and endogenous public information in a model of
speculative attacks, show that uniqueness is attained as long as the precision of the private signals exceeds some increasing
function of the public signal (agents based their decision more heavily in their own private signal)
8
Figure 4: Argentina: Official and Shadow Exchange Rate.
Note: This figure shows the ADR shadow exchange rate and the official exchange rate for Argentina during the capital control
period from 2001 to April 2014.
are the fundamentals driving the performance of the domestic assets. In this direction, the model focus
on the behavior of asset prices in an environment that allows for speculative attacks, in line with Drazen
[2000] and Krugman [2000] who suggest that the outcome of the speculative attack is not determined by the
characteristics of the central bank, but the interaction of these with the performance of the economy.
The paper also relates to an extensive empirical literature that studies the role of cross-listing assets.
According to Bailey et al. [2000], American Depositaty Receipts (ADRs) account for most of the equity
trading across borders, also suggests that foreign investors’ activity is consistent with “liquidity traders”
assumption that is made in the model. Auguste et al. [2006] describe a dramatic change in the trading
volume in cross-listed shares in Argentina during the financial crisis of 2001 and show that although the
aggregate trading volume of the local stock exchange (Merval) steadily declines, cross-listed stocks trading
volume increases dramatically at the time of the financial crises, from representing roughly 40 percent of the
total volume to over 80 percent.
Empirical literature has also focus on the role of ADRs in global financial integration (see, for example,
Melvin [2003], Auguste et al. [2006] and Levy Yeyati et al. [2009]). Empirical studies suggest that the returns
9
on ADRs are negatively affected by currency crises (Kim, Szakmary and Mathur [2000]; Bin, Blenman and
Chen [2004], Pasquariello [2008]), but more crucially, display a persistent violation of the law of one price
between ADRs and the corresponding underlying stocks. This paper develops a theoretical framework that
studies the effects of financial crises and the ADR pricing. Levy Yeyati et al. [2009] show that capital
controls can be easily evaded, and they do affect financial integration as measured by the cross-market
premium. Controls on capital inflows put downward pressure on domestic markets relative to international
ones, generating a negative premium. The opposite happens with controls on capital outflows. Levy Yeyati,
Schmukler and Van Horen [2004] argue that the most liquid stocks, which are also cross-listed, had the
largest increase in price during the financial crises in Argentina. They show that while liquidity played a
role in explaining Argentine stock returns, a dummy variable for ADR shares is significant and positive even
after controlling for liquidity. Cross-listed assets display a particular behavior during financial crises that
can help to predict the proximity of the outbreak.
Also closely related, Eichler et al. [2009] show there is a strong link between ADR shadow exchange
rate and currency crisis, as ADR investors discount higher currency crisis risks from a deterioration of
fundamentals such as a fall in commodity prices, a depreciation in the trading partners’ currencies, an
increase in the sovereign yield spreads, or interest rate spreads widen. They suggest that ADR spreads reflect
investors’ expectations of a devaluation, and represent the mean exchange rate investors expect following a
currency crisis or a realignment.
4
A Cross-listing Asset Model
4.1
Setup
The model has three stages, indexed by t ∈ {0, 1, 2}, and two countries. The first country is referred
as domestic, and the second country as foreign. To fix ideas, one may think of the domestic as a small
developing country, and the foreign as a financially developed country.
Each country has its own currency, referred as the peso and the dollar, respectively. The exchange rate,
measured in units of pesos per dollars, is initially fixed according to a currency peg and normalized to 1.
However, at t = 2 there are two possible exchange rate regimes: the status quo, or a devaluation.
There are three types of investors: a unit measure of informed domestic investors, referred as d -investors,
indexed by i and uniformly distributed over [0, 1], a representative informed foreign investor, referred as the
10
f -investor, and a stochastic measure of liquidity traders denoted by Φ(u), where Φ(·) represents the CDF of
the standard normal distribution, and u is normally distributed with mean 0 and variance αu−1 .
The domestic central bank (CB) is forced to abandon the peg if, and only if, the domestic aggregate
demand of dollars D surpasses the maximum tolerable loss of foreign reserves R. The exchange rate at t = 2,
denoted e, is given by
e=


 1+E
if D > R

 1
if
D≤R
where E > 0 captures the devaluation rate.
4.1.1
Assets
There are two risky assets: a domestic asset (also called underlying) and the corresponding depositary
receipt (DR). Both assets are in zero net supply and pay the liquidation values at t = 2. The liquidation
value of the underlying is denoted by θ units of pesos, and therefore the liquidation value of the DR is given
by θ/e units of dollars, where
θ ∼ N (0, αθ−1 )
and αθ−1 captures the prior precision of the liquidation value of the underlying. By definition, the
liquidation values of the underlying and the DR, measured in the same currency, are equal.
The underlying and the DR are traded in two segmented markets, the domestic and the foreign market
respectively, and are convertible, meaning that shares of the underlying can be exchanged 1-to-1 for shares
of DR, and viceversa. The conversion process takes place overnight: from t = 0 to t = 1, and is costless.
The segmentation is such that only trades denominated in local currency can take place in the local
market. In this way, the underlying (traded in the domestic market) can only be purchased using pesos and
therefore its market price p is denominated in pesos, and the DR (traded in the foreign market) can only be
purchased using dollars and has a market price p˜ denominated in dollars. For simplicity, and without loss
of generality, trading in the asset markets is assumed to be not overlapped, i.e. the domestic market opens
at t = 0, and the foreign market at t = 16 .
6 Many studies have analyzed the marginal effects of overlapping trading hours versus non-overlapping, see Hupperets and
Menkveld [2002], Grammig, Melvin and Schlag [2005] and Wang, Meng Rui and Firth [2002]. The later situation is often valid
for countries such as Taiwan, Japan and Korea, whose exchange market close well before the U.S. exchange open. In this model,
this assumption simplifies the portfolio decision of the informed domestic investors without affecting the basic results.
11
Table (1) summarizes the gross payoffs for the different investments opportunities available in the domestic
market (in the top panel, denominated in pesos) and the foreign market (in the bottom panel, denominated
in dollars). The left column presents the gross payoffs conditional on a devaluation taking place, while the
right column displays the payoffs for the opposite case. For simplicity, consider the case that pesos earn
an interest rate given by r, while dollars earn no interest. Also, assume that the gross payoff earned from
holding pesos is such that pesos are dominated by dollars only in the event of a devaluation: 1 < r < 1 + E.
Devaluation
No Devaluation
Domestic Market (in pesos)
Pesos
r
r
Risky Asset
θ
θ
1+E
1
Dollar
Foreign Market (in dollars)
DR
θ/(1 + E)
θ
Dollar
1
1
Table 1: Gross payoffs of investment opportunities.
Given this microstructure, an investor holding pesos at t = 0 has two ways to acquire dollars before the
regime changes: (i ) exchanging the pesos at the CB, or (ii ) using the cross-market transaction (CMT). The
CMT involves purchasing shares of the underlying at t = 0, converting them into shares of DR (overnight),
and selling them in the foreign market at t = 1.
4.1.2
Informed investors
Both, d - and f -investors have risk-neutral preferences over the terminal wealth denominated in their local
currency7 . However, while d -investors receive an endowment of one peso at t = 0, the f -investor is assumed
to have deep pockets of dollars8 .
Investors can trade in the first two stages facing short-selling constraints for every asset but, given that
the underlying is represented in the foreign market by the DR, the f -investor is indifferent between trading
at t = 0 and not, and for simplicity it is assumed that he only trades in the foreign market at t = 1. This
result is shown in lemma (7), in the appendix.
7 This assumption is without loss of generality. Generalizations of this assumption, when the domestic investor consumes a
basket of domestic and foreign currency, does not change the qualitative results.
8 This assumption helps to focus on the case of sudden capital reversals (or sudden stops), which is at the center of this
model.
12
Let wi denote the final wealth of the d -investor i, denominated in pesos. For a given realization of the
exchange rate e and the liquidation value θ, the final wealth is given by
wi = x0i θ + d0i e + mi r,
(1)
where {x0i , d0i , mi } represents i’s final positions of the risky asset (underlying or DR), dollars and pesos
respectively. Note that at t = 2 the d -investor is indifferent between holding a position of underlying or
DRs, given that measured in the same currency both assets have the same liquidation value.
The t = 0 budget constraint, denominated in pesos, for investor i is given by
1 ≥ xi p + di + mi
(2)
where {xi , di , mi } represents investor i’s demands of underlying, dollars and pesos, at t = 0 respectively.
Each investor i can also trade in the foreign market (at t = 1), if (i) he acquired dollars at t = 0: di > 0,
or (ii) had shares of the underlying converted into DRs: xi > 0. The restriction, that forbids peso holders
from acquiring DRs, is
xi p˜ + di ≥ x0i p˜ + d0i
(3)
Following Hellwig et al. [2006], the d -investor simultaneously solves the portfolio and coordination problems9 . A d -investor attacks when he takes a positive position in dollars, i.e. d > 0. In this way, when a
domestic investor purchases shares of underlying at t = 0, he is indeed sustaining the currency peg. Note
that, only dollars demanded at the CB at t = 0 effectively reduce the foreign reserves of the monetary
authority. If a domestic investor sell shares of the underlying in the foreign market in exchange of dollars,
d0 > 0, it has no effect over the CB’s decision.
4.1.3
Information
At the beginning of the game, each informed investor observes an idiosyncratic private signal about θ.
Let si and s˜ denote the investor i and f -investor private signals, respectively. Conditional on θ, private
signals are independent, and identically distributed according to a normal distribution with mean θ and
variance 1/αs and 1/αs˜, respectively,
9 This assumption opposes to Angeletos and Werning [2006] who assume a two-stage process, first choosing the asset
allocation and later determining whether to attack the regime.
13
si ∼ N (θ, 1/αs );
s˜ ∼ N (θ, 1/αs˜)
Given that each d -investor is ex-ante identical, d -investor i can be characterized by the realization of his
private signal si . For this reason, as long as it remains clear, subindexes are omitted and each d -investor is
completely characterized by the realization of his private signal s.
In line with models on noisy rational expectations, asset prices have a dual function: market clearing
and information aggregation. While a higher asset price reduces the expected return of the asset through
the market clearing channel, it also provides a positive (noisy) signal to the investor which increases the
expected return.
In particular, an informed investor also makes rational inferences from the realization of the market
prices p and p˜. The information set of the d -investor at t = 0 is given by I0 ≡ {s, p}, and at t = 1 is given
by I1 ≡ {s, p, p˜}, while the information set of the f -investor at t = 1 is given by I˜1 ≡ {˜
s, p, p˜}. Informed
investors trading strategies are mappings from signal-price tuples into bounded asset holdings.
4.1.4
Liquidity traders
Liquidity traders are endowed with shares of the underlying that they supply inelastically at t = 0. In line
with Grossman and Stiglitz [1980], liquidity traders prevent asset prices from fully revealing the fundamentals.
As suggested by Easley and O’hara [2004], they can also be motivated by that fact that liquidity traders
represent the number of shares that are genuinely available for trade, rather than the number of shares that
exist. Each liquidity trader supplies shares of the underlying worth 1 peso, and therefore the aggregate
stochastic supply of the underlying is given by,
S(p, u) =
4.1.5
Φ(u)
p
Discussion
Let {X, D, M } ∈ [0, p1 ] × [0, 1]2 represent the t = 0 aggregate demands10 for the underlying, dollars11 and
pesos, where X is given by
10 Aggregate demands can also be interpreted as the expectation of the individual demands, conditional on the true realization
of θ, i.e. X(θ, p) = E[x(s, p) | θ]. Therefore, aggregate demands are also equal to the measure of informed investor demanding
each particular asset times the upper bound of that asset’s individual demand.
R
11 Short-selling constraints bound both the d-investor dollar demand d ∈ [0, 1], and the the size of the attack, D = 1 d di ∈
0 i
[0, 1].
14
Z
∞
X(θ, p) =
√
x(s, p)dΦ ( αs (s − θ))
−∞
and D and M are defined analogously.
Assuming that the price of the underlying is affected by the realization of liquidity traders, which is
demonstrated below, assets demands respond to the two sources of aggregate risk {θ, u}. The equation
below displays the aggregate budget constraint at t = 0, obtained by integrating the d -investors’ budget
constraints at t = 0,
1 = pX(θ, p) + D(θ, p) + M (θ, p)
A devaluation requires a sufficiently high (low) aggregate demand for dollar- (peso-)denominated assets,
i.e. pX(θ, p) + M (θ, p) < 1 − R. According to the previous equation, there are at least two intervals for R
that uniquely determined the outcome of the game; even with perfect information, when multiplicity seems
to be more prominent in the literature. First, if R ≤ 0 the regime collapses with certainty, and the payoffs
are reflected in the left column of table (1). Second, if R > 1, which is the upper bound of D, the regime
survives with certainty, independently of the outcome of the coordination game. In the latter case, dollars
are strictly dominated by pesos and payoffs are represented by the right column of (1). These two sets are
also described by the literature with unique equilibria in the coordination game, and for that reason they
are not at the center of the studies. Following the literature, the level of foreign reserves is assumed to be
such that R ∈ (0, 1], where the CB is vulnerable to a large speculative attack.
From the previous equation is also clear that the fate of the exchange regime depends on the realization
of the measure of liquidity traders. This can be noted by imposing the market clearing condition for the
domestic market, X = Φ(u)/p, into the previous equation, which yields
1 = Φ(u) + D(θ, p) + M (θ, p)
4.2
Timing of the game
The timing of the game is as follows. At the beginning of the game, nature draws the pair {θ, u} that
determines the aggregate state which remains unknown to all agents until t = 2. At t = 0, the d -investor
trades with the liquidity trader in the domestic market, and submits a demand for dollars to the CB. At
stage t = 1, the foreign market opens and the d -investors and the f -investor exchange shares of DR. At
15
t = 2, the CB determines the exchange rate regime and assets pay off. Figure (5) summarizes the timing of
the game.
Stage 0
Stage 1
Stage 2
Stage 3
{θ, u} realized
s˜’s obseved
CB sets e0
Assets pay off
{{si }, s˜} are obseved
Dom. Mkt. opens: p
For. Mkt. opens: p˜
Figure 5: Timing of the game.
4.3
Common knowledge
Before solving the model with asymmetric information, the common knowledge framework is considered.
In this case, it is assumed that informed investors and the CB have common knowledge about θ, but u remains
unknown. Notably, as oppose to Obstfeld [1996], the coordination problems are resolved by realization of
the asset price p, which provides a relevant signal about u12 , and eliminates the uncertainty with respect to
the realization of the exchange rate e. Therefore, the DR is subject to no further risks, the law of one price
holds: p˜ = p/e.
Under the common knowledge assumption, the individual and the aggregate demands from d -investors
coincide. The following proposition describes the equilibrium in this framework. Conditional on θ, p takes
one of two values: either θ/r or θ/(1 + E). The former, and higher, value is observed upon no devaluation,
and as mentioned before requires large liquidity shock13 . Also, p reveals all relevant information about u.
Proposition 1 (Eq. under common knowledge about θ:). Under common knowledge about θ, the price
of the underlying p coordinates d-investors. The price of the DR reflects that at t = 0 all uncertainty is
resolved. In particular, p and p˜ are such that
12 Although there still remains some aggregate risk, the introduction of the asset markets into the Morris and Shin [1998]
framework helps to avoid the coordination failure as long as the aggregate supply is sufficiently inelastic.
13 The domestic market clearing condition: X = S(u, p), and the short-selling constraints, imply that if there is a large supply
of underlying, in equilibrium the domestic aggregate demand for dollars should remain small.
16
p(θ, u)
=


 θ/(1 + E)
if
0 ≤ Φ(u) ≤ 1 − R

 θ/r
if
1 − R < Φ(u) ≤ 1
;
(4)
p˜(θ, u)
=


 p/(1 + E)
if
p = θ/(1 + E)

 p
if
p = θ/r
This and all the proofs are presented in the Appendix. The main idea behind the proof is that if there is
no devaluation, in equilibrium dollars are strictly dominated and the d -investor has to be indifferent between
holding pesos and the underlying, i.e. p = θ/r, in which case the d -investor does not have incentives to
deviate from this strategy (demanding either pesos or the underlying). In particular, there is no devaluation
if there is a large supply of underlying: Φ(u) > 1−R. On the other hand, a devaluation requires Φ(u) < 1−R
and, in equilibrium, p = θ/(1 + E) so that the investor is indifferent between the underlying and dollars, and
pesos are strictly dominated.
In equilibrium the market price p reveals all the relevant information about u, and the posterior probability of devaluation (conditional on p), denoted by π(p), is given by,
π(p) =


 0
if p = θ/r

 1
if p = θ/E
where π(p) depends on the measure of liquidity traders u and the stock of international reserves R. Both,
a lower liquidity in the domestic market or a weaker position of international reserves increase the probability
of a devaluation. In particular, a stronger position of reserves is able to completely avoid the devaluation:
limR→1 π(p) = 0, regardless of u.
Figure (6) illustrates the domestic and foreign asset markets. In the top panel, the blue line depicts the
aggregate demand for the underlying, captured by the measure of d -investors demanding the asset pX(θ, p),
defined by equation (30). Conditional on θ, a higher price increases the demand of the underlying as it
signals that the no devaluation state takes place. If pX(θ, p) < 1 − R, the demand for dollars is high enough
to generate a successful attack. In this case, p = θ/(1 + E) leaves the d -investor indifferent between the
underlying and dollars. Analogously, if pX(θ, p) > 1 − R there is no devaluation and p = θ/r satisfies the
indifference condition. The green line represents a possible realization of the measure of liquidity traders,
Φ(u). A large realization of the measure of liquidity traders reduces the probability of a devaluation. In the
bottom panel, the blue line shows the supply of DRs conditional on p = θ/r, while the orange line represents
17
the supply of DRs conditional on p = θ/(1 + E). In this case, any measure of d -investors supplying the DR
would be consistent with no-arbitrage opportunities.
4.3.1
Capital controls
The next corollary investigates the effects of capital controls on the equilibrium under common knowledge
about θ. Capital controls are modelled as Tobin-like taxes on capital outflows and distort the domestic price
of dollars, which increases to (1 + τ ), where τ captures the size of the tax. The indifference condition for the
d -investor determines that p should be higher in case of a devaluation,
p(θ, u) =


 θ/r


θ(1+τ )
(1+E)
if
1 − R(1 + τ ) < Φ(u) ≤ 1
if
0 ≤ Φ(u) ≤ 1 − R(1 + τ )
(5)
Abstracting from the signalling problem faced by the government when imposing the tax, capital controls
effectively reduce the unconditional probability of a devaluation14 is given by
π(p) = Pr(
1 − R(1 + τ ) > Φ(u))
which is monotonically decreasing in τ .
Corollary 1 (Capital controls). Under common knowledge about θ, the probability of a devaluation is
monotonically decreasing on the level of capital controls (on outflows) τ . Moreover, higher capital controls
increase the over-valuation of the underlying, captured by p − θ.
Given that the f -investor is not subject to any friction, the price of the DR is not affected by the capital
controls, and is still given by equation (4). These results can be extended to the asymmetric information
framework.
4.3.2
Discussion
Finally, consider the case where the exchange rate can take two possible values in the case of a devaluation:
e ∈ {1, 1 + E1 , 1 + E2 }. In particular, conditional on a devaluation taking place, the exchange rate is equal
to e = E1 with probability ρ. The new indifference condition for the d -investor, if the exchange rate reached
after the devaluation is randomly determined, is
14 This corollary abstracts from the signaling problem of the government when establishing this capital controls. This signaling
problem is further studied in Angeletos, Hellwig and Pavan [2006] and Filippini [2013].
18
θ/p = (1 + ρE1 + (1 − ρ)E2 )
Therefore, the new equilibrium asset price takes a value p = θ/(1 + ρE1 + (1 − ρ)E2 ) if 1 − R(1 + τ ) ≤
Φ(u) ≤ 1. Note that uniqueness prevails in this case. Moreover, if the post-attack level of exchange rate
depends on the size of the attack, uniqueness is also preserved along with the structure of the asset price
function from equation (4).
4.4
Asymmetric information
This section describes the equilibrium under asymmetric information. The results are intended to shed
some light into three key features of currency crises. First, what are the drivers of the deviation from the
law of one price of cross-listed assets observed in the data prior to the outbreak of the crises? Second, what
is the predictive power of assets prices, and in particular of depositary receipts, for currency crises? Finally,
what is the role of liquidity in preventing or exacerbating failures of coordination?
This section makes two initial simplifications, along with the result from lemma (7). First, following
the literature on noisy rational expectations, it is assumed that asset prices are monotone with respect
to the endogenous public signals. The public signals embedded in the prices of underlying and the DR
are respectively denoted by z and z˜, and each combines the two sources of aggregate risk θ and u in the
economy15 ,
˜
z˜ = θ + ξu
z = θ + ξu,
where the conditional precision of the public signals are αz = αu /ξ 2 and αz˜ = αu /ξ˜2 for the domestic
˜ are endogenously determined.
and foreign public signals, respectively. The coefficients {ξ, ξ}
Second, in line with the literature on global games, the analysis focuses on monotone equilibria defined
as a perfect Bayesian equilibria such that, at t = 0, a d -investor attacks if, and only if, his private signal s
is below some lower threshold s(z), and demands the underlying if, and only if, s > s(z) where s(z) is some
upper threshold. Furthermore, at t = 1 the d -investor demands dollars if, and only if, his private signal is
below some threshold s0 (z, z˜). Thresholds are identical across d -investors.
Given the information sets, beliefs are updated according to the Bayes rule. Denote {µ, µ0 } ∈ [0, 1]2 the
d -investor’s posterior beliefs at t = 0 and at t = 1, respectively, and µ
˜ the f -investor’s posterior, which are
15 The
equilibrium asset prices p(z) and p˜(z, z˜) depend on the public signals, not separately on θ and u.
19
given by,
d -investor beliefs at t = 1:
f -investor beliefs at t = 0:
√
αs s + αz z
α θ−
,
α
√
αs s + αz z + αz˜z˜
α0 θ −
µ0 (θ | s, z, z˜) = φ
,
α0
√
αs˜s˜ + αz z + αz˜z˜
α
˜ θ−
µ
˜(θ | s˜, z, z˜) = φ
,
α
˜
d -investor beliefs at t = 0:
µ(θ | s, z) = φ
(6)
(7)
(8)
where φ(·) represents the PDF of the standard normal distribution, and the posterior precisions are given
by α = αs + αz + αθ , α0 = α + αz˜, and α
˜ = αs˜ + αz + αz˜ + αθ .
The following definition describes the equilibrium with asymmetric information.
Definition 1. Given {θ, u}, an equilibrium is a set of threshold strategies for: the d-investor at t = 0 given
by {s, s} and at t = 1 given by s0 and a threshold for the liquidation value θ∗ , an optimal portfolio strategy
for the f-investor at t = 1 given by y ∗ , a set of beliefs {µ, µ0 , µ
˜}, and asset prices {p, p˜} such that,
1. Given prices {p, p˜} and thresholds {θ∗ , s˜∗ }, each d-investor chooses the optimum portfolio at t = 0 to
maximize expected final wealth (1) subject to the budget constraint (2) and posterior beliefs (6), and at
t = 1 re-balances his portfolio whenever possible, subject to (3) and posterior beliefs (7).
2. Given prices {p, p˜} and thresholds {θ∗ , s, s s∗ }, the f-investor chooses the optimum portfolio at t = 1 to
maximize expected final wealth subject to the posterior beliefs (8).
3. The domestic and foreign asset markets clear.
4. A devaluation occurs if, and only if, θ < θ∗ .
5. Asset prices p and p˜ depend monotonically on the public signals z and z˜.
6. Beliefs are updated according to the Bayes’ rules (6-8), whenever possible.
The remainder of this section characterizes the equilibrium with asymmetric information, which is solved
using the guess and verify method, and is organized as follows. First, the equilibrium outcome in foreign
market at t = 1 is solved. Second, the optimal portfolio for the d -investor at t = 0 is found. Finally, it is
verified that the equilibrium condition (5) holds at t = 0 and t = 1.
20
4.5
Equilibrium in the Foreign Market (t = 1)
In a monotone equilibrium, any d -investor trading at t = 1 is such that s ∈
/ [s, s]: arrived either with
dollars or shares of DR. A monotone equilibrium in the foreign market is identified by s0 , y ∗ and p˜. The
equilibrium is solved in three steps. First, the problem of the d -investor is considered. Then, the problem
of the f -investor is solved. And finally, the equilibrium in the foreign market is described.
4.5.1
d -investor
Each d -investor arrives to the foreign market with a portfolio given by {x, d} and signals {s, z}. In light
of the new public signal, z˜, embedded in the price of the DR, each d -investor can re-balance his portfolio
by reallocating resources into dollars or DRs. The investor’s risk-neutrality yields a corner solution for the
portfolio problem, and the optimal policy for the d -investor at t = 1 is,



1



κ(s, z, z˜) =
∈ [0, 1]




 0
if
E[θ | s, z, z˜] > p˜E[e | s, z, z˜]
if E[θ | s, z, z˜] = p˜E[e | s, z, z˜]
(9)
otherwise
where κ = 1 represents the decision to keep (buy) the shares of DR, and κ = 0 captures the decision to
sell (hold) the shares, for a d -investor initially holding shares of DR (dollars). Furthermore, κ = 1 implies
{x0 = x + d/˜
p, d0 = 0} and κ = 0 implies {x0 = 0, d0 = x˜
p + d}.
For a given realization of p˜, there is a unique d -investor s0 indifferent between unfolding his portfolio
position and not, which follows from the fact that conditional expected returns of θ and e are monotonic.
The identity of s0 is implicitly determined by
E[θ | s0 , z, z˜] = p˜E[e | s0 , z, z˜]
(10)
and sets the threshold strategy as follows. Each d -investor whose private signal is such that s > s0
chooses κ = 1. The following lemma asserts the existence and uniqueness of the threshold strategy s0 .
Lemma 1 (Existence and uniqueness of s0 ). Given the realization of the asset prices {p, p˜}, the t = 0
threshold strategies {s, s} and threshold θ∗ , there is a unique d-investor indifferent, between holding and
selling the shares of DR, at t = 1 defined by s0 (z, z˜), which solves equation (10)
Note that the marginal d -investor seems to be independent of t = 0 strategies, but only depends of
the realization of the public signals. To illustrate the intuition, figure (7) displays the expected returns for
21
investing in DRs and dollars, conditional on p˜. The blue line depicts the LHS of equation (10), which is
monotonically increasing in s, while the orange line displays the RHS of the equation, which is monotonically
decreasing in s16 .
The next corollary establishes that s0 (z, z˜) is increasing in the realization of the public signals. The
intuition is as follows. Higher realizations of the public signal increases the conditional beliefs about θ
which, in turn, increases the expectation of the liquidation value of the DR and reduces the expected payoff
of dollars. Therefore, it takes a lower signal s to be indifferent between dollars and the DR.
Corollary 2 (Monotonicity of s0 ). The threshold strategy s0 (z, z˜) is strictly monotonically decreasing with
respect to the realizations of the public signals {z, z˜}.
This result has implications in terms of the volume traded in the foreign market given that the supply
of DRs is given by
√
√
max {Φ ( αs (s0 − θ)) − Φ ( αs (s − θ)) , 0}
which is decreasing in θ: lower traded volumes should be observed as the asset’s fundamentals improve.
4.5.2
f -investor
Endowed with dollars and his signals {˜
s, z}, at t = 1 the f -investor decides whether to maintain his
endowment or invest it in DRs. Therefore, the f -investor considers the conditional expected liquidation
value of the DR, where its distribution is given by the convolution of the distributions of θ and e. Figure (8)
illustrates the distribution of the ratio θ/e, for two realizations of the threshold such that θ1∗ < 0 < θ2∗ . The
analytical expression of the distribution of θ/e is provided in the Appendix. In order to provide some intuition
about the distribution, it is useful to consider these two cases. First, if θ∗ > 0, the PDF of θ/e is equal to
that of θ if θ > θ∗ , and given by the PDF of θ/(1 + E) is θ ≤ θ∗ /(1 + E). The interval θ∗ /(1 + E) < θ < θ∗
has zero probability. Similarly, if θ∗ < 0, the PDF of θ/e coincides that of θ/(1 + E) for realization of the
fundamentals such that θ < θ∗ ; and coincides with that of θ if θ > θ∗ /(1 + E). However, in this case, the
probability that θ∗ /(1 + E) > θ > θ∗ is given by the sum of Prob(θ > θ∗ ) + Prob(θ < θ∗ /(1 + E) | θ < θ∗ ),
and therefore the PDF of θ/e is equal to the sum of the PDFs of θ and θ/(1 + E).
The conditional expected return on the DR is given by
16 The
RHS of equation (10) converges to p˜ as s → ∞, independently of the realizations of the public signal or the threshold
θ∗ .
22
θ
π
˜ (˜
s, z, z˜)
E
| s˜, z, z˜ =
E[θ | θ < θ∗ , s˜, z, z˜] + (1 − π
˜ (˜
s, z, z˜))E[θ | θ > θ∗ , s˜, z, z˜]
e
1+E
(11)
where π
˜ (˜
s, z, z˜) denotes the posterior probability of devaluation for the f -investor, computed using beliefs
described by equation (8), and decreasing in s˜. While the second term of the RHS is monotonic in s˜, the
first term works in the opposite direction - monotonicity is useful to facilitate the signal extraction process
from prices. Then, the following lemma describes the sufficient condition for the conditional expected return
on the DR to be monotonically increasing in s˜.
Lemma 2 (Monotonicity of the expected liq. value of the DR). Given the precisions {αs , αz , αs˜, αu }, there
is E such that if, and only if, E < E the expected return of the DR for the f-investor is monotonically
increasing in s˜.
The intuition of the proof is as follows. While a devaluation is undesirable for the f -investor for high
realizations of θ, the opposite is true when θ is low enough, i.e. a devaluation increases the return of the
DR for negative realizations of θ. However, for any given set of parameters, there is a devaluation rate E
close enough to 0 such that the previous effect is depreciatively small, and the expected return on the DR is
monotonically increasing for every s˜.
The optimal portfolio choice for the f -investor is given by



∞



y˜(˜
s, z) =
∈ [0, ∞)




 0
if E[ θe | s˜, z] > p˜
if E[ θe | s˜, z] = p˜
(12)
otherwise
and the market clearing condition for the foreign market requires the f -investor to be indifferent. This
follows from the fact that with strictly positive probability there are some d -investors supplying the asset in
the foreign market. The indifferent condition for the f -investor pins down the price of the DR, which (under
the condition described in lemma (2)) is monotonically increasing in s˜17 . The next lemma describes the the
equilibrium price of the DR.
Lemma 3 (Existence and uniqueness of p˜). Given {p, s, s, θ∗ }, the price of the DR is such that the indifference condition for the f-investor, and solves the following equation
17 Note that the monotonicity condition is important to facilitate the signal extraction from the price of the DR. While, under
weaker conditions that yield a non-monotonic the price of the DR for an interval of realizations of s˜, and the signal extraction
problem becomes algebraically challenging, it remains numerically solvable.
23
θ
p˜ = E
| s˜, z, z˜
e
(13)
Assuming the condition in lemma (2) holds, the RHS from equation (13) is monotonically increasing in
s˜ and the price p˜ is informationally equivalent to the public signal z˜.
Figure (9) displays the price of the DR for two different realizations of the threshold on the liquidation
value, such that θ1∗ < 0 < θ2∗ , and is intended to shed some light over the importance of lemma (2). The
solid line depicts the price of the DR given by equation (13) for the case in which θ2∗ > 0. If the private
signal is high enough, s˜ → ∞, the devaluation risk vanishes and the f -investor prices the DR consequently
(equivalently to the underlying, E[θ | ·]). Analogously, for low enough realization of s˜, the f -investor price
the DR as if a devaluation takes place with certainty: p˜(·, z˜) = E[θ/(1 + E) | ·, z˜]. The dashed line represents
the price of the DR for the case θ1∗ < 0. Note that, without imposing additional structure, the price of the
DR can be non-monotonic with respect to the signal of the f -investor. The intuition behind this results
is as follows. The f -investor wants to avoid a devaluation unless he expects θ to be negative. For some
intermediate (relative) low values of s˜, a devaluation is rather wanted. However, as pointed before, as
s˜ → −∞ the f -investor prices the devaluation scenario: E[θ | ·]/(1 + E), which is decreasing in s˜.
4.5.3
Foreign market equilibrium
Lemmas (1) and (3) characterize the strategies for each d -investor and the f -investor at t = 1, respectively.
The following proposition describes the equilibrium in the foreign market, where investors exchange shares
of DRs for dollars, and indirectly exchange information.
Proposition 2 (Foreign market equilibrium). Given {p, s, s, θ∗ },
1. the price of the DR is given by equation (13), and under the condition stated in lemma (2), and it
perfectly reveals s˜,
2. the threshold strategy for the d-investors, s0 , is given by equation (9),
3. and the portfolio strategy of the f-investor is determined equation (12).
Figure (10) shows the price of the DR for different parametrizations. The top panel displays p˜ under two
possible realizations of the devaluation rate E. A lower E reduces the difference between the liquidation
values of the underlying and DR. Furthermore, as E → 0 the price of the DR converges to the expected
24
value of θ. On the other hand, the bottom panel shows p˜ under two possible values of the precision of the
private signals αs˜. A higher precision of the signal increases the expected value of θ, which also increases
the price of the DR.
4.6
Equilibrium in the Domestic Market (t = 0)
At t = 0, the d -investor trades in the domestic market to allocate his 1 peso endowment into shares of
the underlying or dollars. According to the Bayes rule, the posterior belief at t = 0 is given by equation (6).
Risk-neutrality yields a non-diversified portfolio, where the investor allocates his entire endowment in
the asset with the highest conditional expected return. Note that if E[θˆ | s, p]/p > max{E[e | s, p], r}, both
dollars and pesos are strictly dominated by the underlying for the d -investor with signal s. In this case, he
allocates all his endowment in the underlying, x(s, p) = 1/p, while d(s, p) = m(s, p) = 0. The d -investor’s
optimal demand for the underlying is given by
x(s, p) =






if E[θˆ | x, p]/p > max{E[e | x, p], r}
1
p
h
∈ 0, p1




 0
i
if E[θˆ | x, p]/p = max{E[e | x, p], r}
otherwise
Analogously, the investor’s optimal demand for dollars is given by



1



d(s, p) =
∈ [0, 1]





0
if
E[e | x, p] > max{r, E[θˆ | x, p]/p}
if E[e | x, p] = max{r, E[θˆ | x, p]/p}
otherwise
The monotonicity result of the expected return on dollars from lemma (1) is extended to the narrower
information set at t = 0. Given {p, θ∗ }, the expected payoff of dollars is given by
E[e | s, p] = 1 + π(s, p)E
(14)
where π(s, p) ≡ Prob(θ < θ∗ | s, p) captures the conditional probability of a devaluation. The expected
payoff of dollars is monotonically decreasing in the private signal s. This result follows from the fact the
probability π(s, p) is decreasing in private signal s. Furthermore, dollars are more attractive for lower-signal
d -investors and for cases in which the threshold θ∗ is higher.
25
4.6.1
Discussion: Non-DR Benchmark
Before moving forward, it is worth discussing how this model compares to one in which the underlying
asset lacks of an DR. In this case, there is only one relevant trading round and θˆ simplifies to the payoff
of the underlying θ. Similar to Angeletos and Werning [2006], if the precision of the private signal is high
enough, the uniqueness of the equilibrium is preserved. Building on the result from lemma (1), is clear that
the conditional expected payoff of the underlying is monotonically increasing in the private signal s.
Let q denote the price of the underlying, lacking of a DR, measured in pesos. The price q has to be such
that the marginal investor sq - which is defined below - is indifferent between demanding the underlying and
the alternative investments, as described by the following equation,
E[θ | sq , q]/q = max{E[e | sq , q], r}
where the LHS captures the expected return of the underlying, and the RHS captures the expected return
of the alternative investments.
The price of the underlying q is implicitly defined by the previous equation, and is monotonically increasing in the private signal of the marginal investor sq . This result guarantees that the information embedded
in the asset price q reveals the realization of the private signal of the marginal investor, and conditioning in
q or sq is equivalent. The following lemma formalize this result.
Lemma 4 (No-DR underlying price). Assuming that the underlying asset lacks an DR, and given thresholds
{θ∗ , sq , sq }, the price of the underlying is such that the marginal d-investor is indifferent between acquiring
the asset or the alternatives, and is given by
q=
E[θ | sq ]
max{E[e | sq ], r}
(15)
which is monotonically increasing in sq , and therefore sq is perfectly revealed by the realization of q.
The proof builds on results from previous lemmas, and shows that the price of the underlying reveals the
beliefs of the marginal investor acquiring the asset. Figure (11) shows the price q for different realization
of the marginal investor sq , under two possible realizations of the threshold such that θ1∗ < θ2∗ . The dashed
line represents the price of the underlying assuming a lower threshold θ1∗ , which implies that the for a given
sq the probability of a devaluation is relatively smaller, reducing the expected return on dollars. For that
reason, when considering intermediate realizations of sq the price q(sq , θ1∗ ) lies strictly above q(sq , θ2∗ ). For
26
either small (large) enough realizations of the private signal, the marginal effect of the private signal is small
and for any θ∗ ∈ {θ1∗ , θ2∗ } the investor is pricing a (no) devaluation case. As the marginal investor increases,
the price of the underlying q reflects his ”certainty” there will be no devaluation: E[θ | sq ]/r.
4.6.2
Pricing the Underlying with an DR
Compared to the Non-DR benchmark, the expected payoff of the underlying holding a DR includes the
value of the option to reselling the shares as DRs in the foreign market, at t = 1. The expected continuation
value of the underlying θˆ is given by equation (16), which can be alternatively written as the sum of the
expected liquidation value θ and the value of the option to access the foreign market and sell the shares at
the value of the DR.




pe − θ | s, z, z˜]} | s, z 
E[θˆ | s, p] = E[θ | s, z] + E max {0, E[˜
|
{z
}
(16)
option value: O(s,z,˜
z)
The first term of the RHS of equation (16) coincides to that of the Non-DR asset an reflects the expected
liquidation value θ. The second term captures the value generated from the opportunity to trade the asset
in the foreign market, which is reflected by the t = 0 expected value of the option.
To simplify notation, denote O the t = 1 value of the option to sell the risky asset in the foreign market
for dollars,
O(s, z, z˜) = max {0, υ(s, z, z˜)}
(17)
where υ(s, z, z˜) ≡ E[˜
pe − θ | s, z, z˜] denotes the t = 1 expected value of acquiring p˜ units of dollars while
selling the unit of underlying. Fixing p˜, the option O resembles a put option with respect to s: a higher
s reduces the conditional probability of a devaluation and increases the conditional value of θ. However,
according to equation (13), the value of the DR is tied to the realization of s in such a way that a low
realizations of the marginal’s d -investor reduces p˜, and also decreases the expected value of the option. For
these reasons, the value of the option O is non-monotonic in s.
Figure (12) shows the price of the DR (solid-orange line) and the expected payoff of dollars (dashed-blue
line). This figure assumes a fixed realization of the f -investor signal. A higher realization of the investor s,
increases the price of the DR through the information channel. Lemma (6), below, shows that the price of
the underlying is monotone in s, and therefore the f -investor can extract this signal from observing p.
27
Figure (13) displays the value of the two components of υ as described by equation (16), which reflects the
excess payoff of acquiring dollars at t = 1 (solid-blue line) with respect to holding the underlying (dashedblack line). For low (high) realizations of s (relative to z˜), the marginal d -investor prices a (no) devaluation
event. As shown in figure (12), the price of the DR also reflects some certainty upon this event, and in
that way p˜ converges to E[θ | s, ·]/E[e | s, ·]. For intermediate values, the price of the DR decreases only
marginally relative to the reduction of the posterior of the marginal d -investor, and therefore selling the
underlying is relatively more attractive (the d -investor is pricing a devaluation with a higher probability
that the f -investor). In particular, note that p˜E[e | s, ·] − E[θ | s, ·] ≥ 0 for every s. The payoff difference
between holding the shares of the DR and selling then for dollars, reaches it maximum when the Jensen
inequality is more prominent.
Given thresholds {θ∗ }, and asset prices {p, p˜}, the expected value of acquiring p˜ units of dollars while
selling one unit of the underlying, at t = 1, is given by
υ(˜
s, z, z˜) = p˜E[e | s, z, z˜] − E[θ | s, z, z˜]
(18)
which is positive for every s. Therefore, the value of the option is simple given by O = υ. Further results
on this point are shown in lemma (5).
Figure (14) depicts the value of the option O, as a function of the marginal d -investor s, for two realizations
of the threshold such that θ1∗ < 0 < θ2∗ . The bell-shaped option value highlights the informative role of prices,
i.e. a lower s reduces the price of the DR p˜, only because it reveals a low signal of θ. The option value reaches
its maximum when the uncertainty, measured by the disagreement of beliefs priced in the underlying and
the DR, is higher. The disagreement increases due to the non-linearity of posterior beliefs and the payoff of
the DR. The following lemma describes how the Jensen’s inequality plays a central role in driven the value
of the option.
Lemma 5 (Jensen’s inequality and DR price). Given the threshold θ∗ and strategies {s, s, s∗ , s˜∗ }, the price
of the DR is always as least as high as the ratio of the expected payoffs of the underlying relative to the dollar,
θ
E[θ | s, z, z˜]
p˜ ≡ E
| s, z, z˜ ≥
e
E[e | s, z, z˜]
Therefore, the value of υ is (non-strictly) positive, and the value of the option O simplifies to
O(s, z, z˜) ≡ max{0, p˜E[e | s, z, z˜] − E[θ | s, z, z˜]} = p˜E[e | s, z, z˜] − E[θ | s, z, z˜]
28
(19)
The proof follows from the Jensen’s inequality. This result is key as the value of the option simplifies to
expected excess return from the un-hedged position of purchasing dollars with the proceedings from selling
the underlying. Given this result, the expected continuation value of the underlying θˆ expressed in equation
(16) simplifies to,
E[θˆ | s, z] = E[˜
pe | s, z]
(20)
which represents the value of selling the underlying in the foreign market. The following lemma shows
that the continuation value of the underlying is monotonic in the signal of the marginal investor, which
implies that the information embedded in the price of the underlying has to equal the beliefs of the marginal
investor.
ˆ Given {θ∗ , s, s} and parameters {α}, there is αs > αs such that the
Lemma 6 (Monotonic expectation of θ).
expected value of the payoff of the underlying asset is monotonically increasing in the beliefs of the marginal
d-investor
∂E[θˆ | s, z]
>0
∂s
(21)
which implies that the investors can perfectly learn the realization of s.
The idea behind the proof is that as long as the precision of the private signal of the d -investor is high
enough, the covariance of p˜ and e converges to zero, and therefore the expectation of θˆ reflects product of
E[˜
p | s, z]E[e | s, z] which turns to be monotonically increasing in s.
Using the results from corollary (2) and lemma (2), the conditional expected returns of dollars and
the underlying are monotonic, with the opposite sign. Figure (15) displays the cross-sectional conditional
expected returns of the investment opportunities at t = 0. The intersections between the returns of dollar
and pesos, and pesos and the underlying determine the thresholds strategies {s, s}. The lower threshold is
uniquely determined by the following condition,
E[e | s, z] = max{
E[θˆ | s, z]
, r}
p
(22)
Analogously, there is a unique marginal d -investor s who is indifferent between acquiring the underlying
and the alternatives. This investor has to be such that the domestic market clears, and the following condition
has to be satisfied,
29
1−Φ
|
√
αs (s − θ
=
p
{z
}
Ag. Dem. of Underlying
Φ(u)
p
| {z }
Supply of Underlying
which implies that
s = θ − αs−1/2 u
(23)
As long as s < s, the marginal investor demanding dollars and the underlying do not agree on their
beliefs. The price of the underlying, is determined by the indifference condition for the investor s, and is
implicitly defined in the following equation
E[θˆ | s, z]
= max{E[e | s, z], r}
p
(24)
Finally, the aggregate demand for dollars, which is determined by the marginal d -investor s, is given
√
by D = Φ αs (s − θ . The threshold value for θ is such that the economies just turns to a devaluation,
D = R. The following equation determines θ∗ as a function of the aggregate states of the economy,
θ∗ = s − αs−1/2 Φ−1 (R)
(25)
The following proposition characterize the monotone equilibrium. As stated before, the price of the
ˆ
underlying not only reflects the expected dividend payments but also the value of the option embedded in θ.
Proposition 3. Under asymmetric information, if the condition in lemma (2) holds, there is a unique
equilibrium in which,
1. p is determined by equation (24) and the price of the DR p˜ is given by equation (13),
2. the d-investor’s threshold strategies at t = 0 {s, s} are given by equations (22) and (23), respectively,
3. the strategies at t = 1 for the d-investor s0 is given by equation (9),
4. the strategies at t = 1 for the f-investor s∗ is given by condition (12),
5. and threshold for the regime status θ∗ is given by equation (25)
Figure (16) replicates the evidence shown in section 2. The figure displays the official exchange rate
and the DR shadow exchange rate as functions of the difference between the realization of theta and the
30
threshold level θ∗ . For realizations of θ well above the threshold, the currency peg is sustained and the DR
shadow exchange rate coincides with the official exchange rate. However, as the aggregate state θ approaches
to the threshold, a increasing mass of d -investors are uncertain about the outcome of the game, and for that
reason, prefer to acquire the underlying in an attempt to wait for the next period until new information is
revealed in order to take an irreversible action.
5
Conclusion
Currency crisis are usually associated with large output declines and fiscal cost and have drastic effects
on firms with balance sheet mismatches. Notably, high uncertainty increases the hedging cost against the
effects devaluations exposing agents to the currency crises risk. For this reason, being able to accurately
predict the outbreak of a crises is critical.
In this paper, I have shown how information frictions can contribute in the understanding of the anatomy
of currency crises, studying the behavior of asset prices in the proximity of a currency crisis. Although the
second generation model of currency crises have been able to shed some light in this respect, the empirical
implications of these models are narrow and, in some case, not validated by empirical evidence. The challenge
is to identity the currency crises risk from other factors affecting the asset pricing. I show evidence that
cross-listing assets are good instruments to pin down this risk. I focus on American Depositary Receipts
(ADRs), as a tool to measure investors’ expectations with respect to the post-devaluation exchange rate.
The breakdown of the law of one price (LooP) imposed by arbitrage can account for the currency crises risk.
I develop a theoretical model that captures the impact of currency crises on the pricing of the ADR
market. This paper proposes two extensions to the second generation models of currency crises. First, I
replace the main source of the uncertainty from the balance sheet of the central bank to the performance of
the domestic asset. In this way, the model resembles more closely to sudden reversal of capital flows when
investors unfold the position on local currency. Second, I introduce cross-listing assets into the model.
Using ADRs, investors in the local economy make a cross-market trade to acquire dollars. Note that this
provides investor with an alternative technology to access dollars, other than going to the central bank. In
this way, the price of the underlying asset encompasses not only the expectations of future dividend payments
but also the value of the option to convert the underlying and get dollars. The hedging opportunity makes
the price of the underlying to increase in more fragile states. In turn, this causes the value of the option
embedded in the underlying to increase. The price of the underlying cross-listed stock increases relatively
31
to non-cross-listed stocks, and to its corresponding ADR. The model is able to replicate quantitatively the
breakdown of the LooP in the proximity of a currency crisis.
References
Alexander, Gordon J., Cheol S. Eun, and S. Janakiramanan, “Asset Pricing and Dual Listing on
Foreign Capital Markets: A Note,” The Journal of Finance, March 1987, 42 (1), 151–158.
Angeletos, George-Marios and Iv´
an Werning, “Crises and Prices: Information Aggregation, Multiplicity, and Volatility,” American Economic Review, December 2006, 96 (5), 1720–1736.
, Christian Hellwig, and Alessandro Pavan, “Signaling in a Global Game: Coordination and Policy
Traps,” Journal of Political Economy, June 2006, 114 (3), 452–484.
Auguste, Sebastian, Kathryn M.E. Dominguez, Herman Kamil, and Linda L. Tesar, “Crossborder trading as a mechanism for implicit capital flight: ADRs and the Argentine crisis,” Journal of
Monetary Economics, October 2006, 53 (7), 1259–1295.
Bailey, Warren, Kalok Chan, and Y. Peter Chung, “Depositary Receipts, Country Funds, and the
Peso Crash: The Intraday Evidence,” Journal of Finance, December 2000, 55 (6), 2693–2717.
Bin, Feng-Shun, Lloyd P. Blenman, and Dar-Hsin Chen, “Valuation impact of currency crises:
Evidence from the ADR market,” International Review of Financial Analysis, 2004, 13 (4), 411–432.
Cavallari, Lilia and Giancarlo Corsetti, “Shadow rates and multiple equilibria in the theory of currency
crises,” Journal of International Economics, 2000, 51 (2), 275–286.
Drazen, Allan, “Political Contagion in Currency Crises,” in “Currency Crises” NBER Chapters, National
Bureau of Economic Research, Inc, 2000, pp. 47–70.
Easley, David and Maureen O’hara, “Information and the Cost of Capital,” Journal of Finance, August
2004, 59 (4), 1553–1583.
Eichengreen, Barry, Andrew K. Rose, and Charles Wyplosz, “Speculative Attacks on Pegged Exchange Rates: An Empirical Exploration with Special Reference to the European Monetary System,”
Center for International and Development Economics Research (CIDER) Working Papers C95-046, University of California at Berkeley January 1995.
32
Eichler, Stefan, Alexander Karmann, and Dominik Maltritz, “The ADR shadow exchange rate as
an early warning indicator for currency crises,” Journal of Banking & Finance, November 2009, 33 (11),
1983–1995.
Filippini, Federico, “Optimal Capital Control and Speculative Attacks,” Working Paper, New York University December 2013.
Flood, Robert P. and Peter M. Garber, “Collapsing exchange-rate regimes : Some linear examples,”
Journal of International Economics, August 1984, 17 (1-2), 1–13.
Gagnon, Louis and Andrew G. Karolyi, “Multi-market trading and arbitrage,” Journal of Financial
Economics, July 2010, 97 (1), 53–80.
Goldstein, Itay, Emre Ozdenoren, and Kathy Yuan, “Learning and Complementarities in Speculative
Attacks,” Review of Economic Studies, 2011, 78 (1), 263–292.
Grammig, Joachim, Michael Melvin, and Christian Schlag, “Internationally cross-listed stock prices
during overlapping trading hours: price discovery and exchange rate effects,” Journal of Empirical Finance,
January 2005, 12 (1), 139–164.
Grossman, Sanford J. and Joseph E. Stiglitz, “On the Impossibility of Informationally Efficient Markets,” American Economic Review, June 1980, 70 (3), 393–408.
Hellwig, Christian, Arijit Mukherji, and Aleh Tsyvinski, “Self-Fulfilling Currency Crises: The Role
of Interest Rates,” American Economic Review, December 2006, 96 (5), 1769–1787.
Hupperets, Erik C. J. and Albert J. Menkveld, “Intraday analysis of market integration: Dutch blue
chips traded in Amsterdam and New York,” Journal of Financial Markets, January 2002, 5 (1), 57–82.
Jeanne, Olivier, Currency crises: a perspective on recent theoretical developments, Vol. 20, International
Finance Section, Department of Economics, Princeton University, 2000.
Kaminsky, Graciela L. and Carmen M. Reinhart, “The Twin Crises: The Causes of Banking and
Balance-of-Payments Problems,” American Economic Review, June 1999, 89 (3), 473–500.
Kim, Minho, Andrew C. Szakmary, and Ike Mathur, “Price transmission dynamics between ADRs
and their underlying foreign securities,” Journal of Banking & Finance, August 2000, 24 (8), 1359–1382.
33
Krugman, Paul R., “Introduction to ”Currency Crises”,” in “Currency Crises” NBER Chapters, National
Bureau of Economic Research, Inc, 2000, pp. 1–6.
Maltritz, Dominik and Stefan Eichler, “Currency crisis prediction using ADR market data: An optionsbased approach,” International Journal of Forecasting, October 2010, 26 (4), 858–884.
Melvin, Michael, “A stock market boom during a financial crisis?: ADRs and capital outflows in Argentina,” Economics Letters, October 2003, 81 (1), 129–136.
Morris, Stephen and Hyun Song Shin, “Unique Equilibrium in a Model of Self-Fulfilling Currency
Attacks,” American Economic Review, June 1998, 88 (3), 587–97.
Obstfeld, Maurice, “Rational and Self-fulfilling Balance-of-Payments Crises,” American Economic Review,
March 1986, 76 (1), 72–81.
, “Models of currency crises with self-fulfilling features,” European Economic Review, April 1996, 40 (3-5),
1037–1047.
Pasquariello, Paolo, “The anatomy of financial crises: Evidence from the emerging ADR market,” Journal
of International Economics, December 2008, 76 (2), 193–207.
Wang, Steven Shuye, Oliver Meng Rui, and Michael Firth, “Return and volatility behavior of
dually-traded stocks: the case of Hong Kong,” Journal of International Money and Finance, April 2002,
21 (2), 265–293.
Yeyati, Eduardo Levy, Sergio L. Schmukler, and Neeltje Van Horen, “The price of inconvertible
deposits: the stock market boom during the Argentine crisis,” Economics Letters, April 2004, 83 (1), 7–13.
,
, and
, “International financial integration through the law of one price: The role of liquidity and
capital controls,” Journal of Financial Intermediation, July 2009, 18 (3), 432–463.
Appendices
A
An Introduction to Depositary Receipts
Almost all non-U.S. companies that list their shares on U.S. exchanges use Depositary Receipts (DRs) as
one of their main instruments. DRs traded in U.S. exchanges are referred as American Depositary Receipts
34
(ADRs). Each ADR represents a specific number of shares of the underlying security issued in a domestic
market. ADRs were initially developed to provide U.S. investors with instruments to better diversify their
portfolios. While ADRs have several advantages over other cross-listing shares (dollar-denominated, U.S.
clearance and settlement), they are not fully fungible18 .
From the perspective of the firms, issuing ADRs allow them to access to a broader, and more liquid,
investor base as well as it gives the opportunity to raise new capital. However, non-U.S. companies are
required to comply with the U.S. Securities and Exchange Commission (SEC) requirements: filing a registration statement and furnish an annual report with a reconciliation of financial accounts. In this way, U.S.
investors find more transparent conditions for investing in firms overseas.
The issuance of a DR requires a foreign depositary banks to purchase shares of the underlying stock
and place them on its account at the custodian bank in the local country. A broker can also initiate the
creation of DRs by following the same procedure, and placing the share of underlying on the depositary
bank’s account at the custodian bank. Cancellations or redemptions of DRs simply require reversing the
process. As Gagnon and Karolyi [2010] points out, the convertibility is definitively not seamlessly, since it
requires many intermediaries and usually takes 1 to 2 business days to be completed.
As early noted by Alexander, Eun and Janakiramanan [1987], cross-listing securities can be used to
surpass barriers on capital flows such as capital controls. In this way, DRs provide an alternative technology
to acquire foreign currency in the domestic country (other than going to directly to the central bank).
The cross-market transaction (CMT) requires (i) purchasing the underlying stock in the domestic markets
(denominated in domestic currency), (ii) converting the underlying into (corresponding number of shares
of) DRs, and finally (iii) selling the DRs (denominated in foreign currency) in the foreign exchange.
This convertibility feature, allows to compute a measure of the shadow exchange rate, as the ratio of the
price of the underlying stock (denominated in domestic currency) to the price of the DR (denominated in
foreign currency). The ratio is called the DR shadow exchange rate, and rather reflects investors’ expectations
of the exchange rate - even after a potential currency crisis.
Frictionless models (in particular, with respect to the information) find limitations in assessing the
deviations of the DR shadow exchange rate to the spot exchange rate. In order to show the limitations,
consider first an economy with perfect information. Focus on an investor looking for arbitrage opportunities
between both technologies for purchasing dollars: the central bank (spot exchange rate) and the CMT.
Assume the initial exchange rate is 1. The (gross) returns on the CMT and the dollar investments are,
18 For a definition a full fungibility see Gagnon and Karolyi [2010]. Briefly, full fungibility requires among other conditions
that both claims are identical and there are no legal restrictions on cross-border ownership and trading.
35
Returns on the CMT:
e˜
p
p
Returns on dollars: e
(26)
where e denotes the end-of-period exchange rate (in terms of domestic currency to US dollars), p is the
price of the underlying stock (denominated in pesos), and p˜ represents the price of the ADR (denominated
in dollars). No arbitrage implies that the return from both investments should be equal,
⇒
No arbitrage
p
=1
p˜
(27)
which represents the law of one price in this model19 .
The introduction of capital controls as a step towards restoring independent monetary policy with a
fixed exchange rate regime20 generate persistent deviations of the measures of the exchange rate. Similar to
increasing domestic interest rates, raising the Tobin-tax on capital outflows reduces the opportunity cost of
holding domestic assets and reduces the dollar demand. The policy makers’ rationale for imposing capital
controls is to increase the attractiveness of domestic currency–denominated assets while maintaining the
fixed exchange rate and the level of international reserves untouched.
Consider now the case where capital controls are in place. For simplicity, controls on capital outflows are
modelled as a Tobin-tax proportional to the level of exchange rate. Denote the level of capital controls by
τ . The taxes affects sudden dollar withdraws from the central bank. In this case, the price of a dollar at the
central bank increases to (1 + τ ) and the return is reduced by the same factor. Since the ADR conversion
provides a legal option to surpass the controls, the returns on the cross-market transaction are unaffected.
The no arbitrage condition in this case is
No arbitrage (under capital controls)
⇒
p
= (1 + τ )
p˜
(28)
One of the empirical regularities found in the literature is the persistent relative discount (premium)
taken on ADR after the introduction of controls capital on outflows (inflows). Melvin [2003], Auguste et
al. [2006] and Levy Yeyati et al. [2009] study the behavior the cross-listing securities during episodes with
19 With
common knowledge:
• If p/˜
p > (1 + τ ), no agent demand dollars → No Devaluation.
• If p/˜
p < (1 + τ ), agents do not use cross-listing assets.
• If p/˜
p = (1 + τ ), agents are indifferent between both technologies.
20 According to the Mundell’s trilemma, a country cannot simultaneously maintain a fixed exchange rate and an independent
monetary policy, in a context of free capital flows.
36
capital controls. They propose this market friction as the mechanism that limits arbitrage and prevents
the law of one price from holding. However, there is not interesting dynamics on the DR shadow exchange
rate, as capital controls would increase it by the measure of the tax. From equation (28) it is clear that
capital controls introduce a constant wedge from the law of one price without market frictions, represented
in equation (27).
Pasquariello [2008] empirically shows that the law of one price ceases to hold in proximity to financial
crises. In particular, finds that between ADR returns and the dollar returns of their perfect substitutes
weakened considerably (by 54% on average). Moreover, the wedge between the alternative measures of the
exchange rate increases as the crises approaches.
The DR shadow exchange rate, st , the price ratio of both securities is defined as follows,
s=
p
p˜
(29)
The DR shadow exchange rate represents a measured of the implied exchange rate discounted by DR
investors. It is clear that capital controls only create a constant wedge between the price of both securities21 ,
an a persistent deviation of the DR shadow exchange rate from the official exchange rate22 , but are unable
to capture the dynamics described in section (2).
B
Proofs
Lemma 7 (f -investor trading only in the foreign market). The f investor is at most indifferent between
trading and not-trading in the domestic market.
Proof of Lemma (7). First, consider the case of common knowledge about θ. In this case, the price of the
underlying is determined by the indifferent condition of the d -investor, and is given by equation (4). From
the result of proposition (1), the LooP holds and therefore, the returns of the DR is equal to that of the
underlying.
Second, under asymmetric information about θ the LooP may not hold. This result is given by proposition
(3). In particular, whenever the LooP ceases to hold, price of the underlying is higher that the price of the
DR (adjusted by the exchange rate): p > p˜e. Therefore, the return of the underlying is at most equal to
21 This analysis abstracs from the signalling component of the government actions which might trigger a sudden change in
the investor’s beliefs.
22 Auguste et al. [2006], Levy Yeyati et al. [2009], Eichler et al. [2009] among others find that controls on capital outflows
lead to a higher ADR shadow exchange rate than the official: controls lead to a price premium of the underlying over the ADR
stock.
37
that of the DR. For these reasons, the f -investor is at most indifferent between trading in the domestic and
foreign markets.
Proof of Proposition (1). Under common knowledge about θ, the individual and aggregate demands coincide.
The (gross) return on the underlying is known with certainty, and given by θ/p. The return on the dollar,
however, is uncertain and depends on the regime status.
First, consider the case where the d -investor is certain that a devaluation takes place. In this case, (i)
pesos are strictly dominated (m = M = 0) and (ii) the demand for the underlying asset (and therefore the
underlying liquidity) has to be sufficiently low, pX(θ, p) = Φ(u) ≤ 1 − R. The indifference condition for the
d -investor implies that p = θ/(1 + E). Second, consider the case where Φ(u) > 1 − R. In this case, there is
no devaluation, and therefore dollars are weakly dominated. The indifference condition between acquiring
the underlying and pesos implies that: p = θ/r. Equation (4) describes the equilibrium asset price function.
In this way, the price of the underlying reveals whether the liquidity shock is large enough to avoid the
devaluation or not.
Moreover, given the result in the domestic market, the f -investor also perfectly learn the realization of u
which determines the price of the DR.
The individual and aggregate underlying demands are given by
X(θ, p) = x(θ, p) =



1





1  ∈ [1 − R, 1]
p

∈ [0, 1 − R]





 0
if
θ
r
>p
if
θ
r
=p
if
θ
1+E
(30)
=p
otherwise
where 1/p denotes the upper bound for the underlying demand implied by the short-selling constraints.
Under common knowledge about θ, the information revealed through the prices is such that higher prices
clearly signal a better realization of the fundamentals, generating an increasing asset demand.
Proof of Corollary (1). The probability of a devaluation, given by Pr(
1 − R(1 + τ ) > Φ(u)) is decreasing
in τ , and furthermore, the price of the underlying determined by the indifference condition of the d -investor
is given by 5. The increase of the price of dollars at the CB also increases the price of the underlying, in the
case of a devaluation, with respect to the case of no capital controls.
Proof of Lemma (1). The condition expectation of θ, given by
38
E[θ | s0 , z, z˜] =
αs s + αz z + αz˜z˜
αs + αz + αz˜ + αθ
is monotonically increasing in s, while the conditional expectation of the exchange rate, given by
E[e | s0 , z, z˜] = π(s, z, z˜)E + 1
is monotonically decreasing in s, where π(s, z, z˜) = Φ
√
α0 θ ∗ −
αs s+αz z+αz˜ z˜
α0
, and α0 = αs + αz +
αz˜ + αθ .
Therefore, given the realization of p˜, there exists a unique s0 (z, z˜) such that equation (10) holds.
Proof of Corollary (2). The proof follows from applying the Implicit Function Theorem to equation (10).
The intuition is that higher realizations of the public signal increases the conditional expectation of θ, and
reduces that of the exchange rate. Therefore, it takes a lower s for the indifference in equation (10) to
hold.
Proof of Lemma (2). From equation (11), the expected value of the liquidation value of the DR can be
rewritten as follows,
θ
−E
E
| s˜, z = E [θ | s˜, z] + π
˜ (˜
s, z)
E [θ | θ < θ∗ , s˜, z]
e
1+E
where the conditional expectation E [θ | θ < θ∗ , s˜, z], as well as the conditional probability of devaluation
π
˜ (˜
s, z) are decreasing with respect to s˜. It is easy to show that, the marginal effect of the second term of
the previous equation vanishes as E → 0. Then, there is E such that the marginal effect of the first term
dominates to the one of the second term, regardless of the parameters {αs , αz , αs˜, αθ }.
Proof of Lemma (3). Given that some d -investor sell shares of DR in the foreign market with strictly positive
probability, the f -investor has to be at least indifferent between acquiring shares of the DR and holding the
dollar endowment. If the price of the DR is given by equation (13), the f -investor is indifferent.
The distribution of the liquidation value of the DR, θ/e is given by the convolution of distributions
of θ and e. The PDF of θ/e, denoted by h(θ/e | θ∗ ), depends on the threshold θ∗ which determines the
fundamental level below which there is a devaluation, and is given as follows,
39
h
θ ∗
|θ
e
=










θ∗
1+E
f (θ)
θ∗
1−F (θ ∗ )+G( 1+E
)+1
if
g(θ)
θ∗
1−F (θ ∗ )+G( 1+E
)+1
if θ ≤ θ∗ ≤ 0 ∨ (θ∗ > 0 ∧ θ <

f (θ)+g(θ)


θ∗

1−F (θ ∗ )+G( 1+E
)+1




 0
(θ∗ < 0 ∧
if θ∗ < 0 ∧
θ∗
1+E
< θ) ∨ (θ > θ∗ ≥ 0)
θ∗
1+E )
(31)
> θ > θ∗
otherwise
where f (θ) represents the PDF of θ conditional on the information set {˜
s, z, z˜}, and F (θ) denotes the
corresponding CDF, and g(θ/(1 + E)) represents the PDF of θ/(1 + E) conditional on the same information
set and G(θ/(1 + E)) denotes the corresponding CDF.
The expected value of θ/e is given by
(αs˜s˜+αz z+αz˜ z˜)2
exp − 2(α
2E(αs˜ + αz + αz˜ + αθ )2
4
+α
+α
+α
)
s
˜
z
z
˜
θ
√
+
E[θ/e | s˜, z, z˜] =
2 π(1 + E)(αs˜ + αz + αz˜ + αθ )
h
i
√
˜−θ ∗ (αs˜+αz +αz˜ +αθ )
z
˜z
2π(αs˜s˜ + αz z + αz˜z˜) 2 + E + E Erf αs˜s˜+αz√z+α
2(αs˜+αz +αz˜ +αθ )2
√
+
2 π(1 + E)(αs˜ + αz + αz˜ + αθ )
which is monotonically increasing wherever the condition in lemma (2) holds.
Proof of Proposition (2). The proposition follows from the results from lemmas (1), (3) and (2).
Proof of Lemma (4). Given that the stochastic supply of shares of underlying, provided by the liquidity
traders, has to be absorbed by the d -investors, there is a marginal d -investor, sq , indifferent between acquiring
the underlying and the alternatives. The identity of the marginal investor is determined by the domestic
market clearing condition as shown by equation (23).
The monotonicity of the price q, determined by equation (15), follows from the monotonicity of θ and e,
positive and negative respectively.
Proof of Lemma (5). From the result of lemma (3), the information embedded in the price of the DR is
given by the private information of the f -investor. Furthermore, assuming that the price of the underlying is
monotonically increasing in marginal investor s implies that the information set of the marginal d -investor
at t = 1 is given by {s, s˜}. Applying the Jensen’s inequality to equation (19) yields the result.
40
Proof of Lemma (6). Equation (20) can be rewritten as follows,
E[θˆ | s, z] = E[˜
p | s, z](1 − π(s, z)) + E[˜
p | θ > θ∗ , s, z](1 − Eπ(s, z))
which is strictly increasing is s.
Proof of Proposition (3). The proposition follows from the results from lemmas (5), (6) and (2).
41
Domestic Market
p
FHuL
Θ
r
pX
Θ
1+E
Q
1-R
Ž
p
1
Foreign Market
p
Ž Ž
pX
p
1+E
Ž
Q
1
Figure 6: Asset markets under common knowledge about θ
Note: The market clearing condition for the underlying is given by X(θ, p) = S(u, p). In the top panel, the blue line depicts
the measure of d-investors demanding the underlying, captured by pX(θ, p), at different prices. The green line represents a
realizations of the measure of noise traders, Φ(u). In the bottom panel, the lines represent the measure of d-investor supplying
the DR in the foreign market.
42
Ž
Ž
p EHe s, z, zL
Ž
EHΘ s, z, zL
Ž
s'Hz,zL
s
Figure 7: Expected Return for the d -investor at t = 1.
Note: Considering the d-investor at t = 1, the orange line depicts the conditional expected return for acquiring dollars, and
the blue line depicts that for acquiring the DR. d-investors whose private signal are below (above) s∗ unfold (increase) their
positions in the asset, in exchange for dollars.
pdfHȐeL
Θ2
Θ*1
Θ*1
Θ*2
Θ
Figure 8: Distribution of θ/e
Note: The blue curve depicts the distribution of θ/e for a negative threshold θ1∗ < 0, while the dashed-red curve captures the
distribution for a positive threshold θ2∗ > 0.
43
Ž
pH×,Θ2* L
Ž
E@Θ ×, sD
1+E
Ž
pH×,Θ1* L
Ž
s
Ž
E@ΘÈ×,sD
Figure 9: p˜ as a function of the f -investor’s private information.
Note: The blue curve depicts the price of the DR for a negative threshold θ1∗ < 0, while the dashed-red curve captures the price
of the DR for a positive threshold θ2∗ > 0.
44
Figure 10: Sensitivity of p˜.
Note: The top panel displays the price of the DR under two possible realizations of the devaluation rate such that E 0 > E.
The bottom panel shows the price of the DR considering two possible realizations of the precision of the private signal αs˜.
45
q
qHsq ,Θ1* L
qHsq ,Θ2* L
E@ΘÈ×,sq D
E@Θ ×, sq D
1+E
sq
Figure 11: No DR benchmark: Price of the underlying q
Note: The price of the underlying q as a function of the the marginal investor sq and the threshold θ∗ is described by equation
(15). The only difference between the solid and the dashed lines is that the latter considers a lower threshold: θ1∗ < θ2∗ , which
reduces the probability of a devaluation and therefore increases the (relative) valuation of the underlying. As the marginal
investor increases, the price of the underlying q reflects his ”certainty” there will be no devaluation: E[θ | sq ]/r − 1.
Ž
E@eÈ×,sD
Ž
p
E@Θ ×, sD
E@e ×, sD
s
Figure 12: Value of υ at t = 1
Note: The value of υ reflects the price of the DR and the expected payoff of dollars, with respect to the expected liquidation
value θ. The solid-orange line represents the price of the DR as a function of the the marginal investor s, as described by
equation (13). The dashed-blue line represents the expected payoff of dollar. The dashed-red line represents the ratio of the
payoffs of θ and e.
46
Ž
pE@eÈ×,sD
E@ΘÈ×,sD
s
Figure 13: Value of υ at t = 1
Note: The value of υ is given by the difference of the expected liquidation value and the payoff of acquiring dollars. The
solid-blue line represents the price of the DR times the expected payoff of dollars. The dashed line represents the expected
liquidation value of the underlying.
OH×,Θ2* L
OH×,Θ1* L
s
Figure 14: Option value: O
Note: The option value as a function of the the marginal investor s, as described by equation (17). The only difference between
the solid and the dashed lines is that the latter considers a lower threshold: θ1∗ < 0 < θ2∗ , which reduces the DR price unless s
is large enough.
47
q
E@eÈ×D
`
E@ΘÈ×D
r
s
s
s
Figure 15: Conditional expected returns at t = 0.
Note:
Ž
p
,e
p
1+E
Ž
p
p
e
1
Θ
Θ*
Figure 16: Official and DR shadow exchange rate.
Note: The dashed line represents the exchange rate e for different realizations of θ − θ∗ . The solid line depicts the DR shadow
exchange rate, computed as the ratio of the price of the DR to the price of the underlying.
48