1. No category

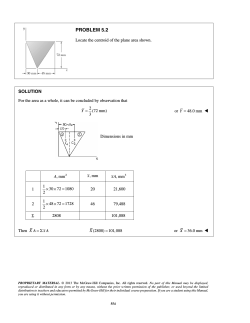
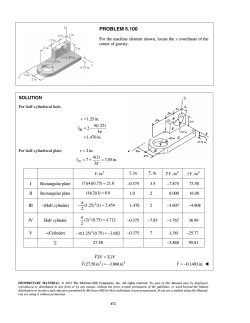
PROBLEM 17.1
The rotor of an electric motor has an angular velocity of 3600 rpm when the load and power are cut off. The
50-kg rotor then coasts to rest after 5000 revolutions. Knowing that the kinetic friction of the rotor produces a
couple of magnitude 4 N · m, determine the centroidal radius of gyration of the rotor.
SOLUTION
ω1 = 3600
Angular velocities:
rev 1min 2π rad
⋅
⋅
= 120π rad/s
min 60 s
rev
ω2 = 0
Angular displacement:
5000 rev = 10000 π rad
Principle of work and energy: T1 + U1→2 = T2 :
1
1
I ω12 = I (120π ) 2 = 71.061 × 103 I
2
2
1
T2 = I ω22 = 0
2
U1→2 = − M θ = −(4 N ⋅ m)(10000π rad) = −40000π N ⋅ m
T1 =
71.061 × 103 I − 40000π = 0
I = 1.76839 kg ⋅ m 2
I = mk 2
Centroidal radius of gyration.
k =
I
1.76839 kg ⋅ m 2
=
= 0.1881 m
m
50 kg
k = 188.1 mm 
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1700
PROBLEM 17.19
A slender 9 lb rod can rotate in a vertical plane about a pivot at B. A spring of
constant k = 30 lb/ft and of unstretched length 6 in. is attached to the rod as
shown. Knowing that the rod is released from rest in the position shown,
determine its angular velocity after it has rotated through 90°.
SOLUTION
Position 1:
CD = 142 + 52 = 14.866 in.
Unstretched
Length
Spring:

x1 = CD − ( 6 in. ) = 14.866 − 6 = 8.8661 in. = 0.73884 ft
Ve =
Gravity:
Kinetic energy:
1 2 1
kx1 = (30 lb/ft)(0.73884)2 = 8.1882 lb ⋅ ft
2
2
 7 
Vg = Wh = 9 lb =  − ft  = −5.25 lb ⋅ ft
 12 
V1 = Ve + Vg = 8.1882 lb ⋅ ft − 5.25 lb ⋅ ft = 2.9382 lb ⋅ ft
T1 = 0
Position 2:
Spring:
x2 = 9 in. − 6 in. = 3 in. = 0.25 ft
Ve =
1 2 1
kx2 = (30 lb/ft)(0.25 ft) 2 = 0.9375 lb ⋅ ft
2
2
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1727
PROBLEM 17.19 (Continued)
Gravity:
Vg = Wh = 0
V2 = Ve + Vg
= 0.9375 lb ⋅ ft
Kinetic energy:
 7 
v2 = rω2 =  ft  ω2
 12 
1
1  9 lb 
I = mL2 = 
(2 ft)2 = 0.093168 slug ⋅ ft 2
12
12  32.2 
1
1
T2 = mv22 + I ω22
2
2
2
=
1  9 lb    7  
1
2

   ft  ω2  + (0.093168)ω2
2  32.2    12  
2
T2 = 0.094138ω22
Conservation of energy:
T1 + V1 = T2 + V2
0 + 2.9382 = 0.094138ω22 + 0.9375
ω22 = 21.253
ω2 = 4.6101 rad/s
ω2 = 4.61 rad/s

PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1728
PROBLEM 17.25
A rope is wrapped around a cylinder of radius r and mass m as shown. Knowing that
the cylinder is released from rest, determine the velocity of the center of the cylinder
after it has moved downward a distance s.
SOLUTION
Point C is the instantaneous center.
v = rω
Position 1. At rest.
ω=
v
r
T1 = 0
Position 2. Cylinder has fallen through distance s.
T2 =
1
1
mv 2 + I ω 2
2
2
1
11
 v 
mv 2 +  mr 2  
2
2 2
 r 
3
= mv 2
4
2
=
Work.
U1→2 = mgs
Principle of work and energy.
3
mv 2
4
4
gs

v2 =
3
T1 + U1→ 2 = T2: 0 + mgs =
v=
4 gs

3
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1737
PROBLEM 17.55
Two disks of the same thickness and same material are attached to a
shaft as shown. The 8-lb disk A has a radius rA = 3 in., and disk B has a
radius rB = 4.5 in. Knowing that a couple M of magnitude 20 lb ⋅ in. is
applied to disk A when the system is at rest, determine the time required
for the angular velocity of the system to reach 960 rpm.
SOLUTION
2
r 
WB =  B  WB
 rA 
Weight of disk B.
2
 4.5 in. 
=
 (8 lb)
 3 in. 
= 18 lb
I = I A + IB
Moment of inertia.
2
1 8 lb  3 
1 18 lb  4.5 
ft  +
ft
=

2 32.2  12 
2 32.2  12 
2
= 0.04707 lb ⋅ ft ⋅ s 2
Angular velocity.
ω2 = 960 rpm = 100.53 rad/s
Moment.
M = 20 lb ⋅ in. = 1.667 lb ⋅ ft
Principle of impulse and momentum.
Syst. Momenta1 + Syst. Ext. Imp.1→2 =
Moments about C:
Required time.
Syst. Momenta2
0 + Mt = I ω2
I ω2
M
(0.04707 lb ⋅ ft ⋅ s2 )(100.53 rad/s)
=
1.667 lb ⋅ ft
t=
t = 2.839 s
t = 2.84 s 
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1791
PROBLEM 17.71
The double pulley shown has a mass of 3 kg and a radius of gyration of 100 mm.
Knowing that when the pulley is at rest, a force P of magnitude 24 N is
applied to cord B, determine (a) the velocity of the center of the pulley after
1.5 s, (b) the tension in cord C.
SOLUTION
rC = 0.150 m
For the double pulley,
rB = 0.080 m
k = 0.100 m
Principle of impulse and momentum.
Syst. Momenta1
+
Syst. Momenta 2
v = rC ω
Kinematics. Point C is the instantaneous center.
Moments about C:
=
Syst. Ext. Imp. 1→ 2
0 + Pt (rC + rB ) − mgtrC = I ω + mvrC
= mk 2ω + m(rC ω )rC
ω=
Pt (rC + rB ) − mgtrC
(
m k 2 + rC2
)
(24)(1.5)(0.230) − (3)(9.81)(1.5)(0.150)
3(0.1002 + 0.1502 )
= 17.0077 rad/s
=
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1813
PROBLEM 17.71 (Continued)
(a)
v = (0.150) (17.0077) = 2.55115 m/s
Linear components:
v = 2.55 m/s 
0 + Pt − mgt + Qt = mv
mv
+ mg − P
t
(3)(2.55115)
=
+ (3)(9.81) − 24
1.5
Q=
(b)
Q = 10.53 N 
Tension in cord C.
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1814
PROBLEM 17.80
A 2.5-lb disk of radius 4 in. is attached to the yoke BCD by means
of short shafts fitted in bearings at B and D. The 1.5-lb yoke has a
radius of gyration of 3 in. about the x axis. Initially the assembly is
rotating at 120 rpm with the disk in the plane of the yoke (θ = 0).
If the disk is slightly disturbed and rotates with respect to the yoke
until θ = 90°, where it is stopped by a small bar at D, determine
the final angular velocity of the assembly.
SOLUTION
2
 1.5   3 
−3
2
I C = mkC2 = 
  12  = 2.9115 × 10 lb ⋅ s ⋅ ft
32.2

 
Moment of inertia of yoke:
Moment of inertia of disk:
1
θ = 0: I A = mr 2
4
=
1  2.5  4 

4  32.2 
 12 
2
= 2.15666 × 10−3 lb ⋅ s 2 ⋅ ft
1
θ = 90°: I A = mr 2
2
=
1  2.5  4 

2  32.2 
 12 
2
= 4.3133 × 10−3 lb ⋅ s 2 ⋅ ft
Total moment of inertia about the x axis:
θ = 0: ( I x )1 = I C + I A
= 5.0682 × 10−3 lb ⋅ s 2 ⋅ ft
θ = 90°: ( I x )2 = I C + I A
= 7.2248 × 10−3 lb ⋅ s 2 ⋅ ft
Angular momentum about the x axis:
θ = 0: H1 = ( I x )1ω1
= 5.0682 × 10−3ω 1
θ = 90°: H 2 = ( I x ) 2 ω2
= 7.2248 × 10−3ω 2
Conservation of angular momentum.
H1 = H 2 : 5.0682 × 10−3 ω1 = 7.2248 × 10−3 ω2
ω2 = 0.7015ω1 = (0.7015)(120 rpm)
ω2 = 84.2 rpm 
PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed,
reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited
distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual,
you are using it without permission.
1831