1. No category

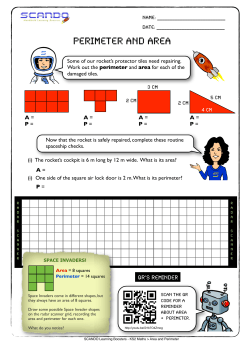
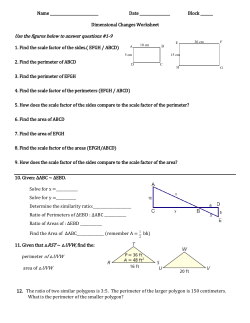
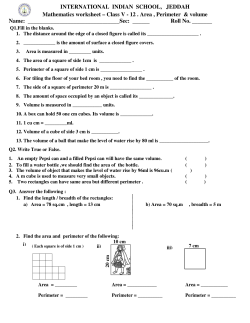
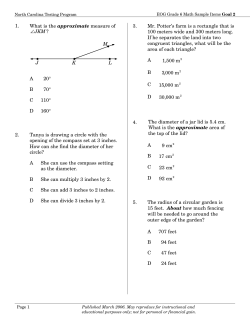
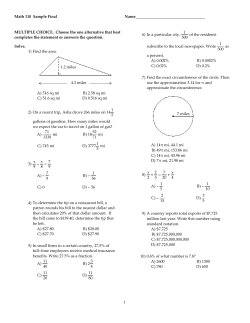
Your Task:
Why this
problem?
GETTING READY
1. Go to the “Area Explorer” at the Project Interactivate Site.
(http://www.shodor.org/interactivate/activities/perm/index.html)
•
Provides the
learners with
experience with
constant perimeter
and changing
area;
•
Illustrates multiple
shapes that have
the same
perimeter.
The “Area Explorer” applet is designed to generate figures of
random shapes on a grid with given perimeters. This tool can be
used to examine the relationships between the areas and the
perimeters of shapes when the perimeters remain constant.
2. Click the How button at the top of the screen for a description of
how to use the tool.
A new window appears in front of the tool.
3. Scroll through the instructions.
4. After reading the instructions, click Return to Activity to return to the tool.
Holding the Perimeter Constant
You will use the tool to complete a table of perimeters, numbers of possible shapes, and areas.
1. Begin by setting the perimeter slider to 4.
2.
Click the Draw New Shape button.
3. Calculate the area of the shape and record it in a table. If desired, you can use this table
provided.
4. Continue to click the Draw New Shape button until you have found all the possible shapes
with that perimeter.
5. Record all of the possible shapes and their areas in the table.
6. Go to the next perimeter in the table. Set the perimeter slider to the new value and repeat the
process until you have filled in the entire the table.
7. Describe any patterns in the areas as the perimeters increase.
8. Can two shapes have the same perimeters but have different areas? Explain your answer
with words and drawings.
* Answers on page 3.
© 2002, PBS. All rights reserved.
Page 1 of 3
Fill in the table:
Perimeter Draw all of the possible shapes. Record the area next to each shape.
4
6
8
© 2002, PBS. All rights reserved.
Page 2 of 3
Answers
7.
Perimeter
4
6
8
Draw all of the possible shapes. Record the area next to each shape.
Area=1 sq. unit
Area=2 sq. units
Area=3 sq. units,
Area=3 sq. units
Area=4 sq. units
8. As the perimeters increase, the number of possible areas increases.
9. Yes. For example, these two figures both have perimeters of 8 units:
However, the first figure has an area of 3 square units and the second figure has an area of 4
square units.
© 2002, PBS. All rights reserved.
Page 3 of 3