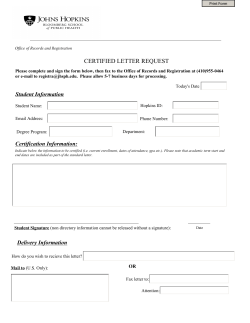
NYU Polytechnic School Of Engineering MA 2222 WORKSHEET # 3
NYU Polytechnic School Of Engineering
MA 2222
WORKSHEET # 3
Date:
Print Name:
Signature:
Section:
Instructor: Dr. Manocha
ID #:
Directions: Complete all questions clearly and neatly. You must show all work to have credit.
Unclear work will not be graded. THIS IS A CRUCIAL HOMEWORK UNDERSTAND IT
WELL FOR YOUR NEXT EXAM.
Problem Possible
1
16
2
16
3
16
4
16
5
18
6
18
Total
100
Points
Your signature:
Z
, where Z is a random variable that is N (0, 1),
(1) (Student’s t-distribution) Let T = p
U/r
U is a random variable that is χ2 (r), and Z and U are independent. Show that T has
a t- distribution with p.d.f.
Γ((r + 1)/2)
1
f (t) = √
,
πrΓ(r/2) (1 + t2 /r)(r+1)/2
−∞ < t < ∞.
Your signature:
(2) Let the distribution of T be t(23). Find
(a) t0.05 (23)
(b) t0.90 (23)
(c) P{−2.069 ≤ T ≤ 2.500}
Your signature:
(3) Let X1 , X2 , ..., Xn be a random sample from distributions with the given probability
ˆ
density functions. In each case, find the maximum likelihood estimator θ.
(a) f (x; θ) = (1/θ2 )xe−x/θ ,
(b) f (x; θ) = (1/2θ3 )x2 e−x/θ ,
0 < x < ∞, 0 < θ < ∞.
0 < x < ∞, 0 < θ < ∞.
Your signature:
(4) Let f (x; θ) = (1/θ)x(1−θ)/θ ,
0 < x < 1, 0 < θ < ∞.
P
(a) Show that the maximum likelihood estimator of θ is θˆ = −(1/n) ni=1 ln Xi .
ˆ = θ and thus that θˆ is an unbiased estimator of θ.
(b) Show that E(θ)
Your signature:
(5) An electric scale gives a reading equal to the true weight plus a random error that is normally distributed with mean 0 and standard deviation σ = 0.1 mg. Suppose that the
results of five successive weighings of the same object are as follows: 3.142, 3.163, 3.155,
3.150, 3.141
(a) Determine a 95 percent confidence interval estimate of the true weight.
(b) Determine a 99 percent confidence interval estimate of the true weight.
Your signature:
(6) The PCB concentration of a fish caught in Lake Michigan was measured by a technique that is known to result in an error of measurement that is normally distributed
with a standard deviation of 0.08 ppm (parts per million). Suppose the results of 10
independent measurements of this fish are
11.2, 12.4, 10.8, 11.6, 12.5, 10.1, 11.0, 12.2, 12.4, 10.6
(a) Give a 95 percent confidence interval for the PCB level of this fish.
(b) Give a 95 percent lower confidence interval.
(c) Give a 95 percent upper confidence interval.
© Copyright 2025