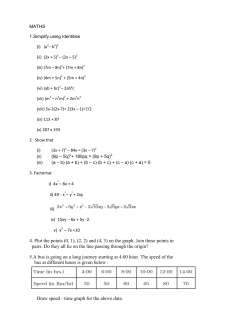
Geometry Chapter 5 REVIEW Problems Name: _____________________________ 2/3/2015 NOTE: back page has a helpful table! 1. Points B, D, and F are midpoints of the sides of EC = 30, AC = 40, and AE = 48. Find the perimeter of ΔBDF. The diagram is not to scale. A B F C E D 2. A triangular side of the Transamerica Pyramid Building in San Francisco, California, is 149 feet at its base. If a climber measures the distance from a base corner of the building to its peak is 859 feet, how far from the ground will the climber have to be to reach the point where the triangular side is 74.5 feet wide? Geometry Chapter 5 REVIEW Problems 3. The length of 2/3/2015 is shown. What other length(s) can you determine for this diagram? D G 4.2 F E 4. bisects Find the value of x and the length of FG. The diagram is not to scale. E 16x – 9 F 13x ) D G 5. Q is equidistant from the sides of | | | T (3 1 x+ 8)° 24° S | Q R Find the value of x. The diagram is not to scale. Geometry Chapter 5 REVIEW Problems 6. Find the circumcenter of 7. Find the length of 2/3/2015 with E(4, 4), F(4, 2), and G(8, 2). , given that is a median of the triangle and AC = 59. D A B C 8. Angle ADB is a right angle. For ∆ABC, name: (a) a median; (b) an altitude; (c) a perpendicular bisector, and (d) an angle bisector. | A E ) | D ) C F B Geometry Chapter 5 REVIEW Problems 2/3/2015 9. What is the orthocenter of the triangle with two altitudes described by the lines and ? y 5 (–1, 3) –5 5 x (4, –2) (–3, –2) –5 ____10. What is the negation of this statement? Miguel has three cats. A. Miguel has more than three cats. B. The cat has three owners. C. Miguel does not have three cats. D. Miguel has no cats. 11. Complete the indirect proof. Given: A quadrilateral and a fact: the sum of the angles of a quadrilateral = 360 degrees. Prove: A quadrilateral can have no more than 3 obtuse angles. Geometry Chapter 5 REVIEW Problems 2/3/2015 12. Identify all sets of parallel segments in the diagram. C 8 6 B D 8 6 A 5 5 F E 13. AC and BD are perpendicular bisectors of each other. Find AE, DB, BC and DC. Justify your answers. A 17 15 D E B 8 C 14. T is the midpoint of QR. U is the midpoint of QS. TU = 36 and m‹QUT = 85. What are RS and m‹QSR? Explain. Q T R U S Geometry Chapter 5 REVIEW Problems 2/3/2015 15. Lisa, Bree, and Caleb are meeting at an amusement park. They each enter at a different gate. On this diagram of the park, show where the friends can meet so that each walks the same distance from the gate to their meeting point. Explain your approach. Gate 2 Gate 1 Gate 3 16. If AC = 18 and BD = 21, find the perimeter of the small “dotted-line” quadrilateral (inside quadrilateral ABCD). Explain. Based on your work, make a conjecture about the relationship between the “midsegment quadrilateral” and the diagonals of the large quadrilateral. B | | | | C || || || || A D Geometry Chapter 5 REVIEW Problems ____17. If 2/3/2015 what is the relationship between D A A. B. B C C. D. not enough information ____18. Which three lengths could be the lengths of the sides of a triangle? A. 12 cm, 5 cm, 17 cm C. 9 cm, 22 cm, 11 cm B. 10 cm, 15 cm, 24 cm D. 21 cm, 7 cm, 6 cm ____19. Two sides of a triangle have lengths 6 and 17. Which expression describes the length of the third side? A. at least 11 and less than 23 C. greater than 11 and at most 23 B. at least 11 and at most 23 D. greater than 11 and less than 23 20. Three security cameras were mounted at the corners of a triangular parking lot. Camera 1 was 156 ft from camera 2, which was 101 ft from camera 3. Cameras 1 and 3 were 130 ft apart. Which camera had to cover the greatest angle? DRAW A SKETCH to support your answer. Geometry Chapter 5 REVIEW Problems Resources: 2/3/2015 Geometry Chapter 5 REVIEW Problems 2/3/2015