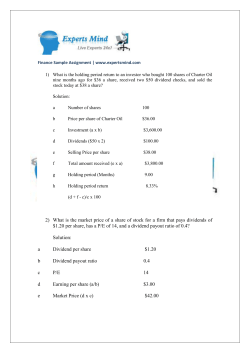
Mar 3NOTES Compound Interest.notebook Today' Objective(s): Monday March 3rd computing compound interest Homework: -- and -Continuous Interest Classwork: ½ WS February 28, 2014 • Interest is a charge you pay for borrowing money or that the bank pays you for leng them invest the money you keep in the bank. Key Vocabulary • Simple interest is a percentage paid on the principal, or inial balance, over a period of me. • Interest • Principal • If you leave the interest in your account, then you will receive interest on both the principal and the interest that is in your account. This is called compound interest because you are receiving interest on the interest. • Compound Interest Look familiar???? Times compounded n s to Thing . know.. nt of ou The am is added t s e r te l in rincipa to the p and then t amoun rest is the inte d on this te compu rincipal. p r e h ig h Compound interest accumulates at a faster rate than simple interest The process is repeated e depending on th number of compounding periods. Annually Semi ‐ annually Quarterly n = 1 n = 2 n = 4 Monthly Bi ‐ Monthly Semi ‐Monthly n = 12 n = 6 n = 24 Weekly Bi‐Weekly Daily n = 52 n = 26 n = 365 "Semi" vs "Bi"....What can you conclude? Mar 3NOTES Compound Interest.notebook February 28, 2014 Example 1 A = Equation Example 2 Suppose that $800 is deposited into an account that pays 9% compounded annually. Determine the amount of interest earned on a $100,000 investment if it is invested at 5.2% annual interest compounded quarterly for 12 years. Find the balance after 4 years? Example 4 Example 3 A coin had a value of $1.17 in 2005. It's value has been increasing at 9.2% each year. What is the value today? A = Continuos Growth Formula 2.718281828 4590452353602874 7135266249757247093699 95957496696762772407663035 mathematical 35475945713821785251664274663919320030592181 constant, e 7413596629043572900334295260595630738132328627. . . Continuously Compounded Interest is a great thing when you are earning it! Continuously compounded interest means that your principal is constantly earning interest and the interest keeps earning on the interest earned! Like the constant π, e is irrational, it never ends and it will never repeat. Mar 3NOTES Compound Interest.notebook #5 If you invest $1,000 at an annual interest rate of 5% compounded continuously, calculate the final amount you will have in the account after five years. If you invest $500 at an annual interest rate of 10% compounded #6 continuously, calculate the final amount you will have in the account after five years. Class Work: #1 How much money will you have at the end of 5 years if you invest $5000 at 8% annual interest compounded quarterly? #2 Calculate how much a 5year loan of $20,000 with annual interest of 5% compounded semiannually will cost you. Cost to you = A P = _______________ #3 #4 An investment of $1425 earns 6.75% and compounds annually. What is the total amount after 8 years? How many compounding periods are there in an investment that compounds... a) quarterly: ______ d) semi annually: _______ b) annually: ______ e) weekly: _______ c) monthly: _______ f) bimonthly: _______ February 28, 2014 #7 If you invest $2,000 at an annual interest rate of 13% compounded continuously, calculate the final amount you will have in the account after 20 years. #8 If you invest $20,000 at an annual interest rate of 1% compounded continuously, calculate the final amount you will have in the account after 20 years. Attachments Clipboard Content.url