Factoring
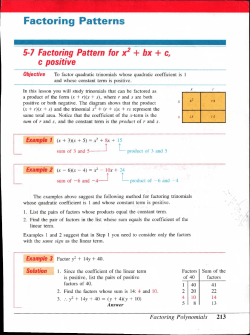
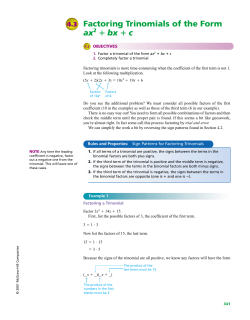
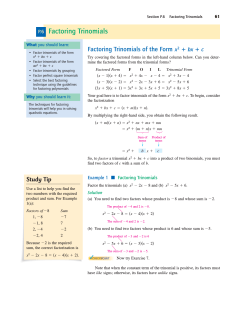
Factoring Polynomial Equations
Ms. Laster
Algebra II

General trinomial - 2x2-5x-12 = (2x + 3)(x - 4)
Perfect Square Trinomial - x2+10x+25=(x + 5)2
Difference of squares - 4x2 - 9 =(2x + 3)(2x - 3)
Common monomial factor - 6x2+15x=3x(2x+5)
Algebra II
Factoring
• Earlier, you learned to factor several types
of quadratic expressions:

• Earlier, you learned to factor several types
of quadratic expressions:
General trinomial - 2x2-5x-12 = (2x + 3)(x - 4)
Perfect Square Trinomial - x2+10x+25=(x + 5)2
Difference of squares - 4x2 - 9 =(2x + 3)(2x - 3)
Common monomial factor - 6x2+15x=3x(2x+5)
Special Factoring Patterns
• Two common patterns are the
difference and sum of two cubes.
• Now, we’ll look at some other types of
polynomials.
Algebra II
Special Factoring Patterns
• Two common patterns are the
difference and sum of two cubes.
• Sum of two cubes
a3 + b3 = (a + b)(a2 - ab + b2)
Algebra II
Algebra II
Special Factoring Patterns
• Two common patterns are the
difference and sum of two cubes.
• Sum of two cubes
a3 + b3 = (a + b)(a2 - ab + b2)
x3 + 8 = (x + 2)(x2 - 2x + 4)
Algebra II
1
Special Factoring Patterns
• Two common patterns are the
difference and sum of two cubes.
• Difference of two cubes
a3 - b3 = (a - b)(a2 + ab + b2)
Algebra II
Special Factoring Patterns
• Factor 64a4 - 27a
Algebra II
Special Factoring Patterns
• 64a4 - 27a Factor common monomial
• a(64a3 - 27)
Algebra II
Special Factoring Patterns
• Two common patterns are the
difference and sum of two cubes.
• Difference of two cubes
a3 - b3 = (a - b)(a2 + ab + b2)
8x3 - 1 = (2x - 1)(2x2 + 2x + 1)
Algebra II
Special Factoring Patterns
• 64a4 - 27a
Factor common monomial
Algebra II
Special Factoring Patterns
• 64a4 - 27a Factor common monomial
• a(64a3 - 27) Difference of cubes
Algebra II
2
Special Factoring Patterns
• 64a4 - 27a Factor common monomial
• a(64a3 - 27) Difference of cubes
• a((4a)3 - 33)
Algebra II
Special Factoring Patterns
•
•
•
•
64a4 - 27a Factor common monomial
a(64a3 - 27) Difference of cubes
a((4a)3 - 33)
a(4a - 3)(16a2 +12a + 9)
Algebra II
Factoring by Grouping
Factoring by Grouping
• Sometimes, you can factor a
polynomial by grouping pairs of
terms that have a common monomial
factor.
• Sometimes, you can factor a
polynomial by grouping pairs of
terms that have a common monomial
factor.
• The pattern for this is:
ra + rb + sa + sb = r(a + b) + s(a + b)

=(r + s)(a + b)
Algebra II
Factoring by Grouping
• Factor x2y2 - 3x2 - 4y2 + 12
Algebra II
Factoring by Grouping
• x2y2 - 3x2 - 4y2 + 12 x2 is common to the
first two terms, and 4 is common to the
second two.
Algebra II
Algebra II
3
Factoring by Grouping
• x2y2 - 3x2 - 4y2 + 12
• x2(y2 - 3) - 4(y2 - 3) remember, you
factored out a -4
Factoring by Grouping
• x2y2 - 3x2 - 4y2 + 12
• x2(y2 - 3) - 4(y2 - 3)
• (x2 - 4)(y2 - 3) 1st term is a difference of
squares
Algebra II
Factoring by Grouping
•
•
•
•
x2y2 - 3x2 - 4y2 + 12
x2(y2 - 3) - 4(y2 - 3)
(x2 - 4)(y2 - 3)
(x - 2)(x + 2)(y2 - 3)
Algebra II
Algebra II
Factoring Polynomials in Quadratic Form
• Sometimes an expression will be in
quadratic form, but not obviously.
Any expression in the form au2 + bu +
c, where u is some expression of x, is
quadratic.
Algebra II
Factoring Polynomials in Quadratic Form
Factoring Polynomials in Quadratic Form
• Sometimes an expression will be in
quadratic form, but not obviously.
Any expression in the form au2 + bu +
c, where u is some expression of x, is
quadratic.
• 81x4 - 16 This is not obviously
quadratic, since 81x4 doesn’t look like
a perfect square, but let’s look again.
• 81x4 - 16
• (9x2)2 - 42 Now, this is a difference of
Algebra II
squares
Algebra II
4
Factoring Polynomials in Quadratic Form
Factoring Polynomials in Quadratic Form
• 81x4 - 16
• (9x2)2 - 42 Now, this is a difference of
• 81x4 - 16
• (9x2)2 - 42 Now, this is a difference of
squares
•
(9x2 -
4)(9x2 +
squares
4)
• (9x2 - 4)(9x2 + 4) That first term is another
difference of squares
Algebra II
Algebra II
Factoring Polynomials in Quadratic Form
Factoring Polynomials in Quadratic Form
• 81x4 - 16
• (9x2)2 - 42 Now, this is a difference of
• Try factoring a2b2 - 8ab3 + 16b4
squares
• (9x2 - 4)(9x2 + 4)
• (3x - 2)(3x + 2)(9x2 + 4)
Algebra II
Algebra II
Factoring Polynomials in Quadratic Form
Factoring Polynomials in Quadratic Form
• a2b2 - 8ab3 + 16b4 Common factor
• a2b2 - 8ab3 + 16b4
• b2(a2 - 8ab + 16b2)
Algebra II
Algebra II
5
Factoring Polynomials in Quadratic Form
Factoring Polynomials in Quadratic Form
• a2b2 - 8ab3 + 16b4
• b2(a2 - 8ab + 16b2) Perfect Square
• a2b2 - 8ab3 + 16b4
• b2(a2 - 8ab + 16b2)
• b2(a2 - 4b)(a2 - 4b) or
Trinomial
Algebra II
b2(a2 - 4b)2
Algebra II
Summary of Methods for Factoring
1) Take out any common factors.
2) Recognize if polynomial is (or isn't) a perfect square, a difference of
squares, a difference of cubes or a sum of cubes.
3) If quadratic, try decomposition method.
4) If polynomial is higher than degree 2, find factors of the form (hx k) or (x - k) by substituting x = k/h and or
x = k into the polynomial and then using long division.
4) Continue process until polynomial is fully factored.
5) Check the factors by multiplying them together - you should get the
original polynomial if the factors are correct.
Algebra II
6