15. (II) A small rubber wheel is used to drive a large pottery wheel, and they are mounted so that their circular edges touch. If the small wheel has a radius of 2.0 cm and accelerates at the rate of 7.2 rad/s2, and it is in contact with the pottery wheel (radius 25.0 cm) without slipping, calculate (a) the angular acceleration of the pottery wheel, and (b) the time it takes the pottery wheel to reach its required speed of 65 rpm. ' l y ,re!,ÿ :.:j" &-- v'd,_ °" & C - "ÿ t ¢% j' #, .... 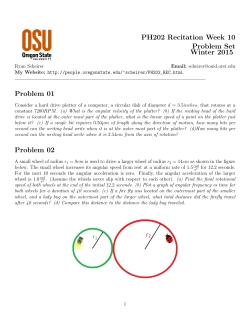"OS 23. (II) Calculate the net torque about the axle of the wheel shown in Fig. 10 52. Assume that a friction torque of 0.30 m. N opposes the motion. 20Nÿ30N FIGURE 10-52 Problem 23. i¸ ÿ v ÿ =,9ÿ , :4 a , I ..... ......... :a;: ,,1 , 50N / 24. (Iÿ) Determine the net torque on the 2.0-m-long beam shown in Fig. 10-53. Calculate about (a) point C, the cÿ, and (b) point P at one end. 60 N C P / • I 50 N , ÿ]' J ':z .... FIGURE 10-53 Problem24. Ptÿ >, j ................... ( I# iii: ........ 7. & >,'#/ c ÿ-/ # 2 : z 34. (IIÿ Four equal masses M are spaced at equal intervals, I, /'along a horizontal straight rod whose mass can be ignored. ./- The system is to be rotated about a vertical axis passing through the mass at the left end of the rod and perpendicu- lar to it. (a) What is the moment of inertia of the system about this axis? (b) What minimum force, applied to the farthest mass, will impart an angular acceleration a? (c) What is the direction of this force? / Y S-%ÿ%: ! g 59. (1I) A 4.8-m-diameter merry-goÿround is rotating ÿeely with an angular ve!ocity ol 0.80 rad/s. Its total moment of inertia is 1950 kg. m2. Four people standing on the ground, each of 65-kg mass, suddenly step onto the edge of the merry-goround. What is the angular velocity of the merry-go-round i now? What if the people were on it iÿitially and then jumped off in a radial direction (relative to the merry-go-round)? l"¸ ? ......... / \ / iÿ, 'ÿ'ÿ ...... ,.,{r / ,¢ ..... lÿ'¸ ,,ÿ ÿ'ÿ ,ÿ:• ÿ :/'ÿ Hÿ••• :•uÿ¸ÿ¸ •:ÿ:ÿ¸ 9ÿ. A uniform disk turns at 7.0 rev/s around a frictionless spinJ die. A nonrotating rod, of the same mass as the disk and length equal to the disk's diameter, is dropped onto the reety spirming disk. They then both mrn around the spindle with their centers superposed, Fig. 10-67. What is the angu- lar velocity in rev/s of the combination? FIGURE 10-67 Problem 96. i J 2¸ ÿ h/t ÿ'ÿ "2 J ,i'¸ ]_ ,f / 6.3 A ring of mass M and radius R lies on its side on a fMct[onless table. It is pivoted to the table at its rim. A bug of mass nÿ walks around the ring with speed v, starting at the pivot. What is the rotational velocity of the ring when the bug is (a) hÿ31fway around and (b) back at the pkot, Ans. clue. (a) If tzÿ = M, ¢ÿ = v/'31ÿ < .... O14 A :;=3 coJ/ , / r Mÿÿ ÿ .- 2. ÿ4ÿÿ m (ÿ r "ÿ-a ) /L(.ÿ.z..,) B " 1 6.14 A uniform stick of massl]! and length lis suspended horizontally with end B on the edge of a table, and the other end, 4 is held by hand, PointA issuddeniy released, At the instant after release: a. What [s the torque about B? b, What is the angular acceleration about B? c, What is the vertical acceTeration of the center of mass? d, From part c, find by inspection the vertical force at ]?, ,ÿzJ'- .L olt:tÿ.,. t4Z 2L )