1. No category

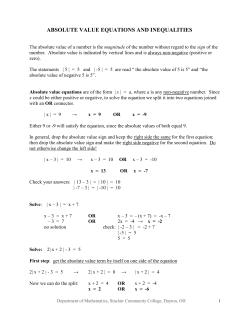
Algebra Final Exam
Solving Linear Equations Topical Review
Procedures
Solve for x:
4x + 3 = 11
4x + 3 = 11
-3 -3
4x = 8
4 4
x=2
Box VARIABLE TERM and Solve for variable


Get BOXED TERM by itself on one side of
the = sign
Solve for VARIABLE BOXED TERM:
Equations with Fractions
*6
*6
*6 5
x  17  102
6
Type 1:

Fraction with no ( ) in problem
Multiply EVERY term by the bottom # to get
rid of the fraction
5x  102  612
+102 +102
5x = 714
5
5
x = 142.8
This will cancel the Fraction out

Then solve basic equation
*4
*4 3
(3x  8)  15
4
Type 2:

Fractions with ( ) in problem
Multiply both sides by bottom # to get rid of
the fraction
This will cancel the Fraction out


3(3x  8)  60
Distribute remaining # in front of ( )
Then solve basic equation
9x  24  60
-24 -24
9x = 36
9 9
x=4
SOLVING INEQUALITY EQUATIONS

Solve the inequality equation using
procedures from above

Graph the solution on a number line using
correct endpoints (open or closed)
< or > have open circles
–2(x – 3) > 5(x – 9)
 2x  6  5x  45
-6
-6
-2x > 5x – 51
-5x -5x
-5x
-7x > -51
-7 -7
51
x
7
 or  have closed circles

Shade -Pick a test point to determine truth
value. Shade where value is TRUE

Write answer using
Interval Notation ( ) or [ ]
Solution Set < or > and 
or 

51
7
0
51
7
(,
REMEMBER: When you DIVIVDE by a NEGATIVE number you MUST FLIP the inequality sign
51
]
7
Solve the following:
3
(3x  8)  15
4
1.
8y – (5y + 2) = 16
2.
3.
9x – 6 = 5x – 15 + x
4.
3x
 7x  7  3(2x  1)
2
6.
3x  2 
8.
x3
4
 (x  1) 
2
5
5
5
x
8
8
5.
2x 
7.
1
7
5x  (3x  8)  4  x
2
2
9.
Solve and graph the inequality:
2
x  1  15
3
1 x

2 3
10.
Solve and graph the inequality:
4(2 – x)  -2x
11.
What is the value of x in the equation
x2 1 5
 
3
6 6
(1) 4
(2) 6
12.
13.
(3) 8
(4) 11
Which value of x satisfies the equation:
(1)
8.25
(3)
19.25
(2)
8.89
(4)
44.92
The inequality 7 
(1) x > 9
(2) x  
14.
?
3
5
7
9 
x 
  20 ?
3
28 
2
x  x  8 is equivalent to
3
(3) x < 9
3
(4) x  
5
Which of the following represents an expression?
(1) x  7  4
(3) 3x 2  5
(2) 5X  8  12
(4) 2 X  2  30
15.
Given the equation ax + b = c, then x can be expressed as
cb
c
(1) x 
(3) x   b
a
a
bc
c b
(2) x 
(4) x 
a
a
16.
The length of a rectangle is 15 and its width is w. The perimeter of the rectangle is, at
most, 50. Which of the inequalities could be used to find the longest possible width?
(1) 15  w  50
(2) 30  2w  50
(3) 30  2w  50
(4) 15  w  50
17.
18.
Given 2x + ax – 7 > -12, determine the largest integer value of a when x = -1.
Solve the inequality below to determine and state the smallest possible value for x in
the solution set. Write your answer in interval notation.
3(x + 3) ≤ 5x – 3
19.
At the Smith’s wedding, the bride and groom paid $11,374 for their guests to eat and
rent the ballroom for dancing after dinner. The venue charges $79 per adult, and $45
per child. If there were 122 more adults than children, write an equation to represent
this situation. You do not have to solve.
20.
Val and Max work at a dance store. Val is paid $210 per week plus 5% of his total sales
in x dollars, which is represented by V(x)  210  0.05x . Max is paid $360 per week plus
1% of his total sales in x dollars, which is represented by M(x)  360  0.01x . Determine
the value of x dollars that will make their weekly pay the same.