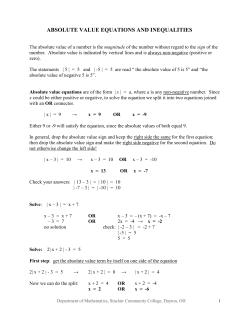
Subject: Mathematics Date: September 29, 2014 Topic: Inequations Subtopics: Introduction to Linear Programming Level: Fourth Form Time: 40 minutes (9:30-10:10) References: Toolsie Raymond (2004) : “Mathematics A Complete Course with CXC Questions” Volume 1. Pgs. 301-304 Greer A. & Layne C. E. (1994): Certificate Mathematics-A Revision Course for the Caribbean, Pg. 77-83 Previous Knowledge: Students know deduce inequality provided with graphical representation and number line illustration. In addition, they know the four inequalities signs and when to use each depending on the key word used in verbal sentences. Furthermore, they know to construct simple linear inequality graph. Objectives: Through guided practice and cooperative learning, students will be able to: 1. Model real-life situation using a linear inequality in two variables (C-A,S) 2. Appreciate the importance of linear programming in solving real-life situation (A) Concepts: Linear programming is the process of taking various linear inequalities relating to some situation, and finding the "best" value obtainable under those conditions. A typical example would be taking the limitations of materials and labor, and then determining the "best" production levels for maximal profits under those conditions. In "real life", linear programming is part of a very important area of mathematics called "optimization techniques". This field of study (or at least the applied results of it) is used every day in the organization and allocation of resources. These "real life" systems can have dozens or hundreds of variables, or more. In algebra, though, you'll only work with the simple (and graphable) two-variable linear case. The general process for solving linear-programming exercises is to graph the inequalities (called the "constraints") to form a walled-off area on the x,y-plane (called the "feasibility region"). Then you figure out the coordinates of the corners of this feasibility region (that is, you find the intersection points of the various pairs of lines), and test these corner points in the formula (called the "optimization equation") for which you're trying to find the highest or lowest value. Skills:     Identify which inequality signs to use Derive and graph the inequality Identify the feasible region Deduce the ordered pairs that satisfies the inequality Attitudes:  Willingness to participate in class activity  Work cooperatively to reach a common goal Materials: worksheet (application problem) Introduction: 1. Teacher will provide students a brain teaser on inequalities: 2. Students will be given one minute to think about it on their own and then one minute to discuss with a shoulder partner. 3. Students will then share their solutions with the rest of the class and their approach to finding the only ordered pairs. 4. Students will be randomly chosen to provide the inequality from the assignment given and teacher will randomly select students to agree or disagree with the result giving a logical justification. Development: 1. In pairs, teacher will provide each student with an example of a question modeling linear inequality. 2. Through questioning, the teacher will guide students to deriving the appropriate inequality. 3. Using a scale of 1cm to represent 400 coins on each axis, students will construct an appropriate graph to model the inequality. 4. Students will then identify the feasible region and shade it. Then they will identify the ordered pairs of integers in the graph that are possible solutions. 5. Teacher will clarify any misconceptions. Closure: Teacher will ask students, what the objectives for the day’s lesson were. Conclusion: Extended Activity: Students will research other applications of linear programming in real life. Evaluation: ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________