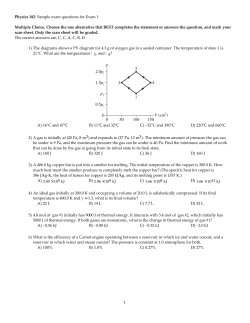
In-Class Exercise 4-01: A perfect heat engine with = 0.4 is used as a refrigerator (the heat reservoirs Th and Tc remain the same). How much heat Qc can be transferred in one cycle from the cold reservoir to the hot one if the supplied work in one cycle is W = 10 kJ? 1 For one cycle: = W Q − Qc = h Qh Qh Qc =1− Qh Qc Qc Qc Qh COP = = = Qc W Qh − Qc 1−Q h (1) (2) (3) 1− 1− = (4) 1 − (1 − ) 1 − 0.4 COP = = 1.5 (5) 0.4 So, if the supplied in one cycle work is W = 10 kJ, the heat that be transferred from the cold reservoir to the hot one is Qc = COP × W = 1.5 × 10 = 15 kJ. COP = In-Class Exercise 4-02 (textbook): Prove that if one can had a heat engine whose efficiency was better than the ideal value 1 − TTc , you could hook it up to an h ordinary Carnot refrigerator to make a refrigerator that requires no work input. 2 Hot reservoir, Th Qh,r Qh,e Magic engine Carnot refrige W Qc,e Qc,r Cold reservoir, Th Step-1: Ideal engine, the heat input and output are in the same ratio as the reservoir temperatures Qh,e Qc,e = Th,e Tc,e (6) Step-2: The work to be generated W , Qh,e − Qc,e = W (7) Step-3: Ideal Carnot refrigerator, the heat input and output are in the same ration as the reservoir temperatures Qh,r Qc,r = Th,r Tc,r (8) Step-4: For a given work W , Qh,r − Qc,r = W (9) T T h,e Step-5: Using equations above, and Th,r = Tc,e , we can get c,r Qh,e = Qh,r =α Qc,e Qc,r Qh,e − Qc,e = Qh,r − Qc,r (10) (11) From this two equations, we can get: Qh,r = Qh,e (12) Qc,r = Qc,e (13) Step-6: If the engine is better than ideal, Q0h,e < Qh,e = Qh,r . For the same W , we also have Q0c,e < Qc,e = Qc,r Step-7: The net heat flow from high T (to low T ) is: Q0h,e −Qh,r < 0. So, heat flow from low T to high T . This is against the Second Law of Thermodynamics.