1. No category

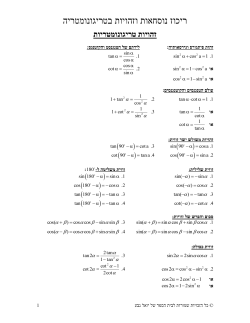
‫הוכן ע"י צחי אבנור‬
/ ‫טבלת אינטגרלים‬
‫ אך מטעמי‬,‫ מאחר ומדובר בפונקציות קדומות לכל תוצאה צריך להוסיף קבוע אינטגרציה‬:‫הערה חשובה‬
. ∫ f '(x)dx = f (x) +C ‫ ∫ הכוונה היא ל‬f '( x)dx = f ( x) ‫ כאשר רשום‬,‫ לכן זכרו‬.‫חיסכון החלטתי לוותר על זה‬
:‫אינטגרלים יסודיים‬
∫ 0 ⋅ dx = C
∫ dx = x
∫ e dx = e
∫ sin( x )dx = − cos x
x
x
∫ sinh( x )dx = cosh x
∫ cosh( x )dx = sinh x
∀λ ≠ −1.∫ x λ dx =
x λ +1
λ +1
∫ cos( x)dx = sin x
n
n
k =0
k =0
k
k +1
∫ ∑α k x = ∑α k x /(k + 1)
1
∫ x dx = ln (| x |)
1
∫x
2
dx =
−1
x
dx
= x
x
∫2
:‫אינטגרלים טריגונומטריים‬
dx
dx
∫ cos2 x = tan x ∫ sin2 x = − cot x ∫ tan( x )dx = − ln cos x ∫ cot( x)dx = ln sin x
dx
dx
∫ cosh 2 x = tanh x ∫ sinh 2 x = − coth x ∫ tanh( x)dx = ln cosh x ∫ coth( x)dx = ln sinh x
x sin2x
x sin2x
sinh2x x
x sinh2x
2
2
2
2
∫ sin (x)dx = 2 − 4 ∫ cos (x)dx = 2 + 4 ∫ sinh (x)dx = 4 − 2 ∫ cosh (x)dx = 2 + 4
∫
:‫אינטגרלים של הנגזרות של פונקציות טריגונומטריות והיפרבוליות הפוכות‬
dx
−1 ⋅ dx
dx
dx
arctan( x / a )
∫ 1 − x 2 = arcsin x ∫ 1 − x 2 = arccos x ∫ 1 + x 2 = arctan x ∫ x 2 + a 2 =
a
−1
dx
dx
dx
dx
tanh ( x / a )
= sinh −1 x ∫
= cosh −1 x ∫
= tanh −1 ( x ) ∫ 2
=−
2
2
2
2
1− x
x −a
a
x +1
x −1
∫e
λx
dx =
e
λx
λ
:‫אינטגרלים של פונקציות מעריכיות ולוגריתמיות‬
ax
ln( x )
x ln x − x
x
a
dx
ln( x )dx = x ln x − x ∫ log a ( x ) dx = ∫
dx =
=
∫
∫
ln a
ln a
ln a
‫אינטגרלים של סק וקוסק‬
1
1
1
: ‫הגדרות‬
csc θ =
sec h(θ ) =
csc h(θ ) =
sin θ
cosh θ
sinh θ
dx
dx
−1 x
−1 x
∫ sec(x)tan(x)dx = sec(x) ∫ csc(x)cot(x)dx = −csc(x) ∫ x x2 − a2 = sec ( a ) ∫ x x2 + a2 = csch ( a )
1
. sec θ =
cos θ
UnderWarrior Project http://underwar.livedns.co.il